Variable Tailing Nonlinear Transformation Design Based on Exponential Function in Impulsive Noise
-
摘要:
针对脉冲噪声中的信号检测问题,该文提出一种基于指数函数的非线性变换函数设计与优化方法。该方法利用指数函数衰减速度可调的优点,适用于脉冲噪声的各种分布模型。通过引入效能函数,将非线性函数设计问题转化为以效能最大化为目标的阈值与底数参数优化问题。由于效能是关于待优化参数的连续可导且单峰函数,该优化问题可采用数值优化方法如单纯形法快速稳健地求解。性能分析表明,针对脉冲噪声常用的对称α稳定分布、Class A分布和高斯混合分布,该文方法均能取得基本最优检测性能,基于实测大气噪声仿真的通信误码率也明显优于传统的削波器和置零器。因此,该文为各种分布的脉冲噪声提供了一个统一的最优抑制解决方法。
Abstract:A novel design of nonlinear transformation function for the signal detection in impulsive noise is proposed. The proposed method takes the advantage of adjustable fading factors of the exponential function, it can be effective for different models of impulsive noise. By introducing the efficacy as the objective function, nonlinear design is converted into the problem of optimizing the threshold and bottom parameters to maximize the efficacy. Since the efficacy is continuous, derivative, and unimodal, the optimization problem can be easily solved by the traditional optimization methods, such as the Nelder-Mead simplex method. Analysis shows that the proposed design can obtain the optimal performance in the widely-used models of impulsive noise, including the symmetric α-stable model, the Class A model, and the Gaussian mixture model. Simulation on real atmospheric noise demonstrates that the proposed design is obviously better than the traditional clipper and blanker. Thus, this paper proposes an optimal and uniform solution for suppressing impulsive noise of various models.
-
表 1 高斯混合噪声中非线性变换的效能
$(\varepsilon ,\sigma _2^2)$= (0.3 10) (0.3 100) (0.3 1000) (0.1 10) (0.1 100) (0.1 1000) (0.01 10) (0.01 100) (0.01 1000) 局部最优检测 0.5198 0.5709 0.6338 0.7935 0.8316 0.8678 0.9695 0.9796 0.9846 最优置零器 0.4637 0.5421 0.6196 0.7624 0.8160 0.8611 0.9647 0.9752 0.9837 最优削波器 0.4592 0.3906 0.3662 0.7407 0.6958 0.6793 0.9568 0.9453 0.9409 GZMNL 0.5056 0.5674 0.6328 0.7883 0.8300 0.8672 0.9689 0.9774 0.9846 GGM 0.4540 0.4982 0.5791 0.7576 0.7924 0.8311 0.9620 0.9691 0.9773 X 轴平移模式 0.5079 0.5652 0.6313 0.7880 0.8286 0.8665 0.9686 0.9772 0.9845 Y 轴平移模式 0.4939 0.5044 0.5512 0.7626 0.7614 0.7858 0.9599 0.9557 0.9589 定点平移模式 0.5091 0.5282 0.5697 0.7776 0.7837 0.8032 0.9636 0.9618 0.9641 -
NIKIAS C L and SHAO Min. Signal Processing with Alpha-Stable Distributions and Applications[M]. New York: Wiley, 1995: 67–73. 吕新荣, 李有明, 余明宸. OFDM系统的信道与脉冲噪声的联合估计方法[J]. 通信学报, 2018, 39(3): 191–198. doi: 10.11959/j.issn.1000-436x.2018047LYU Xinrong, LI Youming, and YU Mingchen. Joint channel and impulsive noise estimation method for OFDM systems[J]. Journal on Communications, 2018, 39(3): 191–198. doi: 10.11959/j.issn.1000-436x.2018047 OH H and NAM H. Design and performance analysis of nonlinearity preprocessors in an impulsive noise environment[J]. IEEE Transactions on Vehicular Technology, 2017, 66(1): 364–376. doi: 10.1109/TVT.2016.2547889 LU Lu, ZHAO Haiquan, and CHAMPAGNE B. Distributed nonlinear systern identification in α-stable noise[J]. IEEE Signal Processing Letters, 2018, 25(7): 979–983. doi: 10.1109/LSP.2018.2835763 罗忠涛, 卢鹏, 张杨勇, 等. 大气噪声幅度分布与抑制处理分析[J]. 系统工程与电子技术, 2018, 40(7): 1443–1448. doi: 10.3969/j.issn.1001-506X.2018.07.05LUO Zhongtao, LU Peng, ZHANG Yangyong, et al. Analysis on amplitude distribution and suppression techniques of atmospheric noise[J]. Systems Engineering and Electronics, 2018, 40(7): 1443–1448. doi: 10.3969/j.issn.1001-506X.2018.07.05 MIDDLETON D. Non-Gaussian noise models in signal processing for telecommunications: New methods an results for class A and class B noise models[J]. IEEE Transactions on Information Theory, 1999, 45(4): 1129–1149. doi: 10.1109/18.761256 SAAIFAN K A and HENKEL W. Decision boundary evaluation of optimum and suboptimum detectors in class-A interference[J]. IEEE Transactions on Communications, 2013, 61(1): 197–205. doi: 10.1109/TCOMM.2012.100812.110565 RABIE K M, ADEBISI B, TONELLO A M, et al. For more energy-efficient Dual-hop DF relaying power-line communication systems[J]. IEEE Systems Journal, 2018, 12(2): 2005–2016. doi: 10.1109/JSYST.2016.2639321 ZHIDKOV S V. Performance analysis and optimization of OFDM receiver with blanking nonlinearity in impulsive noise environment[J]. IEEE Transactions on Vehicular Technology, 2006, 55(1): 234–242. doi: 10.1109/tvt.2005.858191 ROŽIĆ N, BANELLI P, BEGUŠIĆ D, et al. Multiple-threshold estimators for impulsive noise suppression in multicarrier communications[J]. IEEE Transactions on Signal Processing, 2018, 66(6): 1619–1633. doi: 10.1109/TSP.2018.2793895 KAY S M. Fundamentals of Statistical Signal Processing, Volume Ⅱ: Detection Theory[M]. Prentice-Hall, 1998: 626–645. LI Xutao, SUN Jun, WANG Shouyong, et al. Near-optimal detection with constant false alarm ratio in varying impulsive interference[J]. IET Signal Processing, 2013, 7(9): 824–832. doi: 10.1049/iet-spr.2013.0024 ZHANG Guoyong, WANG Jun, YANG Guosheng, et al. Nonlinear processing for correlation detection in symmetric alpha-stable noise[J]. IEEE Signal Processing Letters, 2018, 25(1): 120–124. doi: 10.1109/LSP.2017.2776317 RABIE K M and ALSUSAE E. On improving communication robustness in PLC systems for more reliable smart grid applications[J]. IEEE Transactions on Smart Grid, 2015, 6(6): 2746–2756. doi: 10.1109/TSG.2015.2430528 罗忠涛, 卢鹏, 张杨勇, 等. 抑制脉冲型噪声的限幅器自适应设计[J]. 电子与信息学报, 2019, 41(5): 1160–1166. doi: 10.11999/JEIT180609LUO Zhongtao, LU Peng, ZHANG Yangyong, et al. Adaptive design of limiters for impulsive noise suppression[J]. Journal of Electronics &Information Technology, 2019, 41(5): 1160–1166. doi: 10.11999/JEIT180609 LUO Zhongtao, LU Peng, and ZHANG Gang. Locally optimal detector design in impulsive noise with unknown distribution[J]. EURASIP Journal on Advances in Signal Processing, 2018, No. 34: 1–12. doi: 10.1186/s13634-018-0560-x. VASTOLA K. Threshold detection in narrow-band non-Gaussian noise[J]. IEEE Transactions on Communications, 1984, 32(2): 134–139. doi: 10.1109/TCOM.1984.1096037 NELDER J A and MEAD R. A simplex method for function minimization[J]. The Computer Journal, 1965, 7(4): 308–313. doi: 10.1093/comjnl/7.4.308 WRIGHT M H. Direct search methods: Once scorned, now respectable[C]. 1995 Dundee Biennial Conference in Numerical Analysis, Dundee, UK, 1996: 191–208. POWELL M J D. An efficient method for finding the minimum of a function of several variables without calculating derivatives[J]. The Computer Journal, 1964, 7(2): 155–162. doi: 10.1093/comjnl/7.2.155 PRESS W H, TEUKOLSKY S A, VETTERLING W T, et al. Section 10.7. Direction Set (Powell's) Methods in Multidimensions[M]. 3rd ed. New York: Cambridge University Press, 2007: 123–134. CONN A R, SCHEINBERG K, and VICENTE L N. Introduction to Derivative-free Optimization[M]. Philadelphia: SIAM, 2009: 155–164. 罗忠涛, 卢鹏, 张杨勇, 等. 基于高斯化-广义匹配的脉冲型噪声处理方法研究[J]. 电子与信息学报, 2018, 40(12): 2928–2935. doi: 10.11999/JEIT180191LUO Zhongtao, LU Peng, ZHANG Yangyong, et al. A novel method for nonlinear processing in impulsive noise based on Gaussianization and generalized matching[J]. Journal of Electronics &Information Technology, 2018, 40(12): 2928–2935. doi: 10.11999/JEIT180191 LUO Zhongtao, GUO Renming, ZHANG Xinshu, et al. Optimal and efficient designs of Gaussian-tailed non-linearity in symmetric α-stable noise[J]. Electronics Letters, 2019, 55(6): 353–355. doi: 10.1049/el.2018.7347 ARIF M, NASEEM I, MOINUDDIN M, et al. Design of optimum error nonlinearity for channel estimation in the presence of Class-A impulsive noise[C]. The 6th International Conference on Intelligent and Advanced Systems, Kuala Lumpur, Malaysia, 2016: 1–6. doi: 10.1109/ICIAS.2016.7824137. IKPEHAI A, ADEBISI B, RABIE K M, et al. Energy-efficient vector OFDM PLC systems with dynamic peak-based threshold estimation[J]. IEEE Access, 2017, 5: 10723–10733. doi: 10.1109/ACCESS.2017.2709254 PENG Siyuan, CHEN Badong, SUN Lei, et al. Constrained maximum correntropy adaptive filtering[J]. Signal Processing, 2017, 140: 116–126. doi: 10.1016/j.sigpro.2017.05.009 邱天爽, 张旭秀, 李小兵, 等. 统计信号处理: 非高斯信号处理及其应用[M]. 北京: 电子工业出版社, 2004: 165–166.QIU Tianshuang, ZHANG Xuxiu, LI Xiaobing, et al. Statistical Signal Processing: Non-Gaussian Signal Processing and Its Application[M]. Beijing: Publishing House of Electronics Industry, 2004: 165–166. -






 下载:
下载:
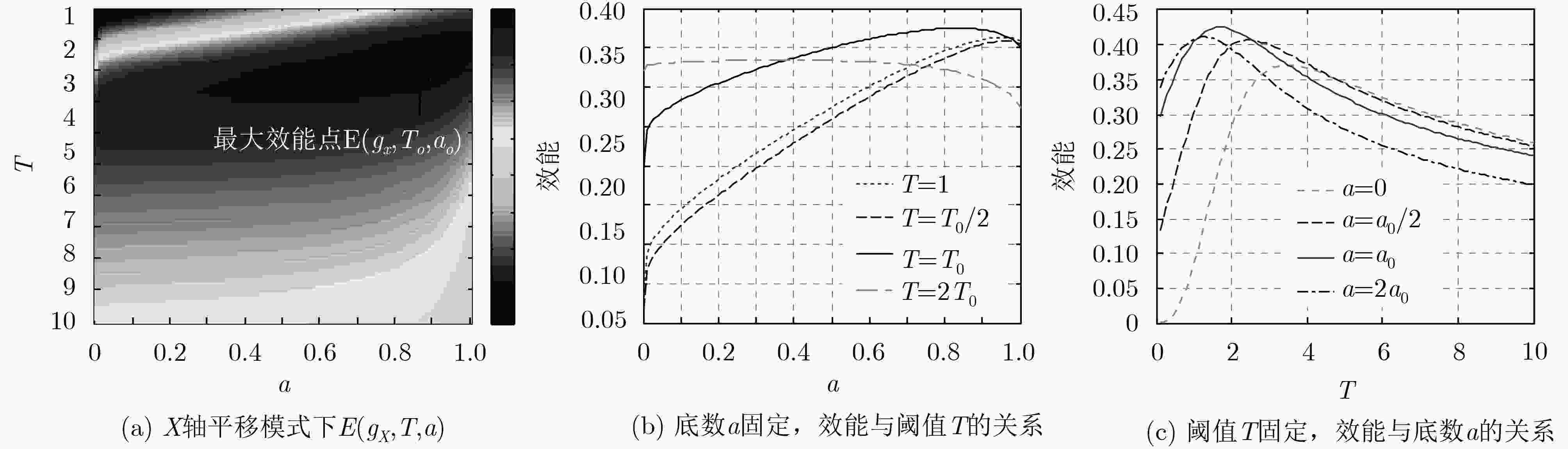






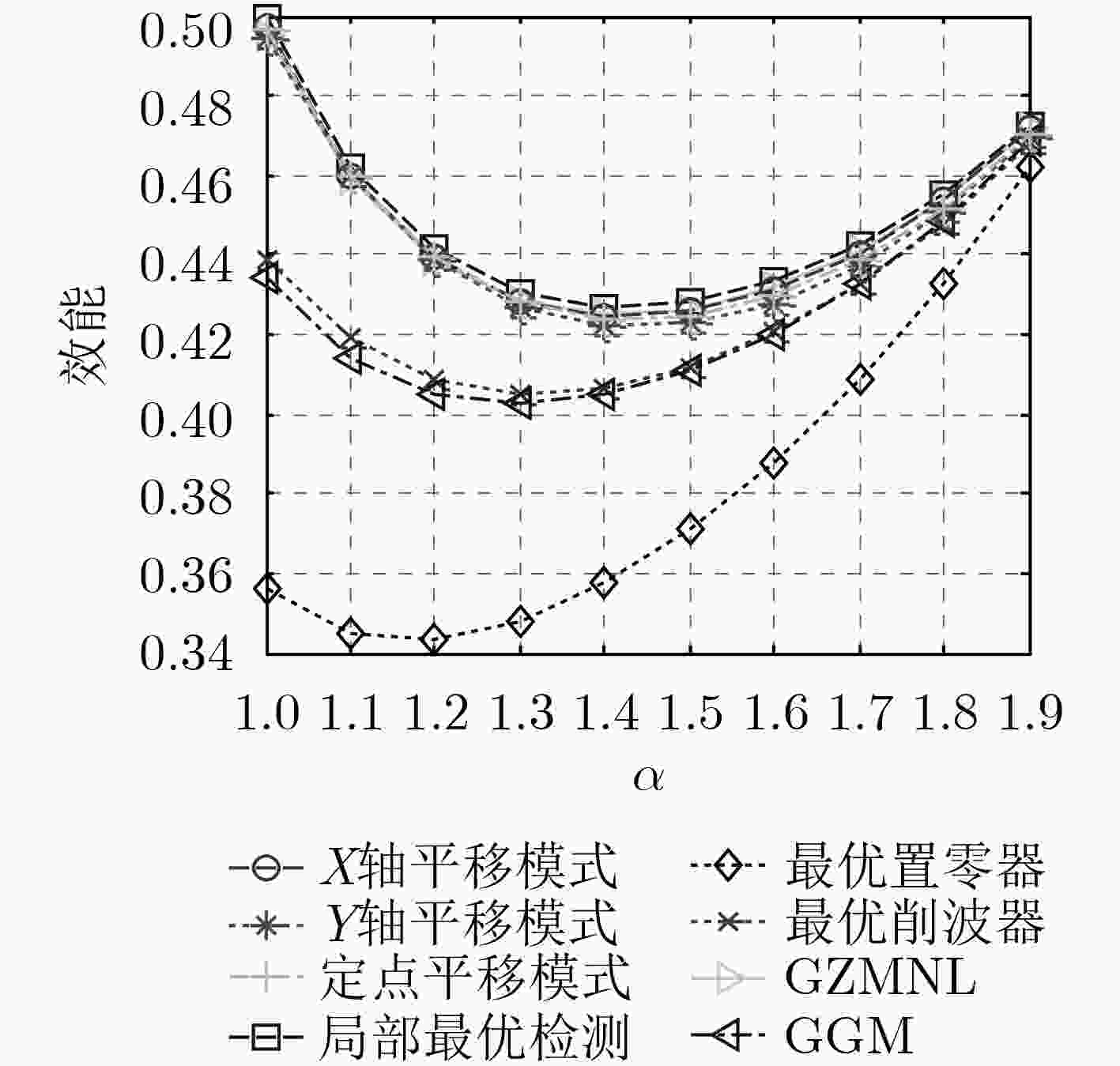

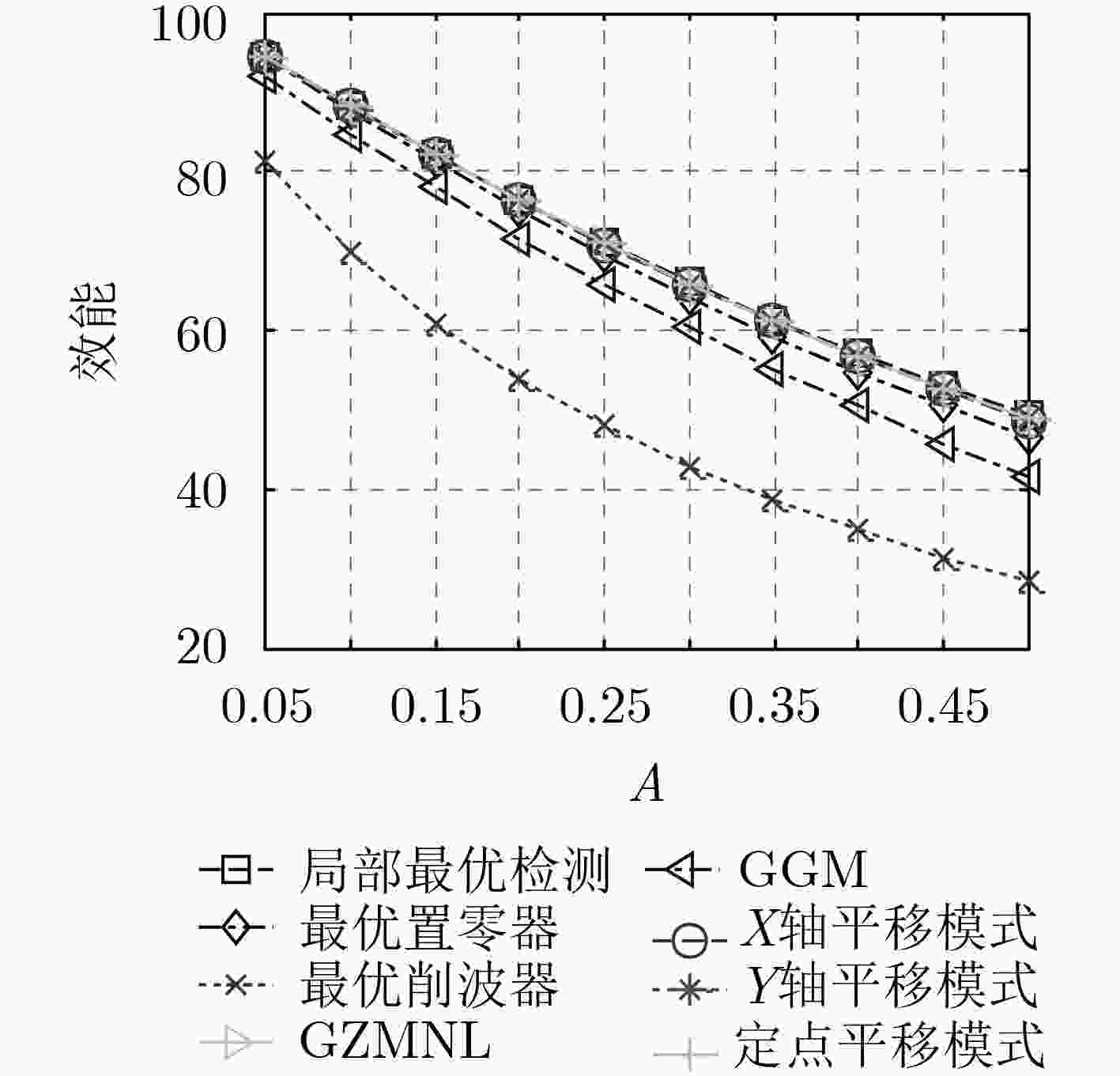
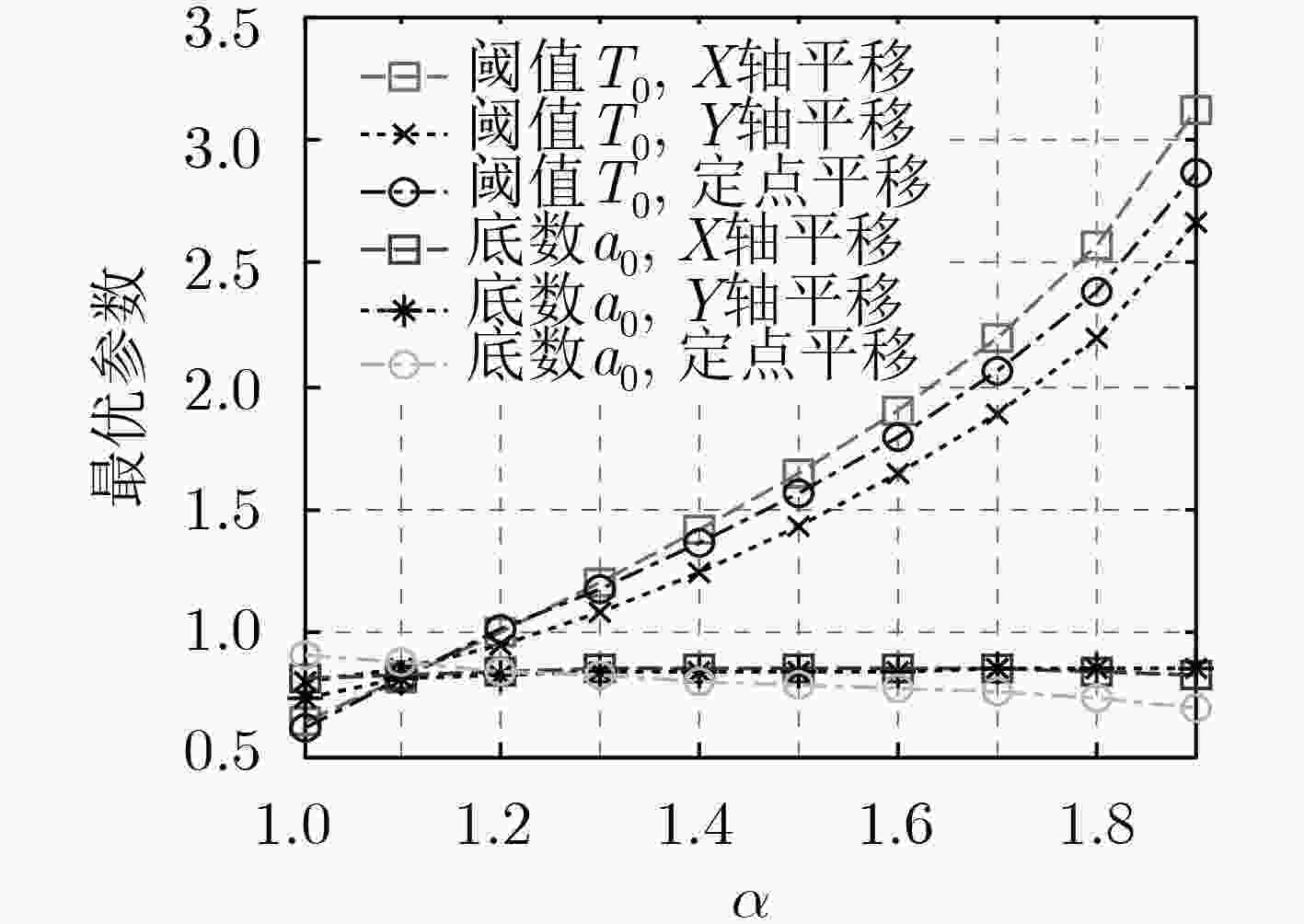





 下载:
下载:
