One-step Calculation Circuit of Blind Signal Detection using Complex-valued Hopfield Neural Network
-
摘要: 信号盲检测在大规模通信网络中具有重要的意义并得到了广泛的应用,如何快速得到信号盲检测结果是新一代实时通信网络的迫切需求。为此,该文从模拟电路的角度设计了一种能加速信号盲检测的复值Hopfield神经网络(CHNN)电路,该电路可一步完成大规模并行计算,提高信号盲检测速度,同时该电路可以通过调整忆阻器的电导和输入电压来实现可编程功能。Pspice仿真结果表明,该电路的计算精度可达99%以上,运行时间比Matlab软件仿真快3个数量级,此外,该电路具有良好的鲁棒性,即使在20%的噪声干扰下,仍能保持99%以上的计算精度。
-
关键词:
- 电路设计 /
- 忆阻器 /
- 复值Hopfield神经网络 /
- 信号盲检测
Abstract: Blind signal detection is of great significance in large-scale communication networks and has been widely used. How to quickly obtain blind signal detection results is an urgent need for the new generation of real-time communication networks. Considering this demand, a Complex-valued Hopfield Neural Network (CHNN) circuit is designed that can accelerate blind signal detection from an analog circuit perspective, the proposed circuit can accelerate the blind signal detection by rapidly performing massively parallel calculation in one step. At the same time, the circuit can be programmable by adjusting the conductance and input voltage of the memristor. The Pspice simulation results show that the computing accuracy of the proposed circuit can exceed 99%. Compared with Matlab software simulation, the proposed circuit is three orders of magnitude faster in terms of computing time. And the accuracy can be maintained at more than 99% even under the interference of 20% noise. -
表 1 电路和软件计算时间比较(ms)
输入信号数量 计算时间 Pspice Matlab 5 阶 0.001 9.5 10 阶 0.03 10.8 20 阶 0.04 11.2 40 阶 0.07 13.3 80 阶 0.16 15.2 -
[1] SATO Y. A method of self-recovering equalization for multilevel amplitude-modulation systems[J]. IEEE Transactions on Communications, 1975, 23(6): 679–682. doi: 10.1109/TCOM.1975.1092854. [2] TONG Lang, XU Guanghan, and KAILATH T. Blind identification and equalization based on second-order statistics: A time domain approach[J]. IEEE Transactions on Information Theory, 1994, 40(2): 340–349. doi: 10.1109/18.312157. [3] LI Jin, FENG Dazheng, and LI Bingbing. Space-time blind equalization of dispersive MIMO systems driven by QAM signals[J]. IEEE Transactions on Vehicular Technology, 2018, 67(5): 4136–4148. doi: 10.1109/TVT.2018.2790432. [4] HU Rong, YU Shujuan, ZHANG Yun, et al. WSN blind detection based on SOS[C]. The 11th World Congress on Intelligent Control and Automation, Shenyang, China, 2014: 5763–5766. doi: 10.1109/WCICA.2014.7053704. [5] LV Zhisheng, FENG Bin, and TAN Li. An improved dual-mode blind equalization algorithm for QAM signals[C]. 2022 6th International Conference on Robotics and Automation Sciences (ICRAS), Wuhan, China, 2022: 283–287. doi: 10.1109/ICRAS55217.2022.9842274. [6] BLOCHBERGER M, ELVANDER F, ALI R, et al. Distributed cross-relation-based frequency-domain blind system identification using online-admm[C]. 2022 International Workshop on Acoustic Signal Enhancement (IWAENC), Bamberg, Germany, 2022: 1–5. doi: 10.1109/IWAENC53105.2022.9914714. [7] YUAN Kang, ZHUO Junnan, GAO Wei, et al. Diffusion constant modulus algorithm for blind equalization[C]. 2022 IEEE International Conference on Signal Processing, Communications and Computing (ICSPCC), Xi’an, China, 2022: 1–6. doi: 10.1109/ICSPCC55723.2022.9984630. [8] XIAO Ying and DONG Yuhua. Blind equalization based on neural network under LS criterion by gradient iteration algorithm[C]. 2009 Fifth International Conference on Natural Computation, Tianjian, China, 2009: 91–94. doi: 10.1109/ICNC.2009.134. [9] ZHANG Yun and ZHANG Zhiyong. Blind 8PSK signals detection using discrete complex-valued Hopfield network[C]. 2010 IEEE 12th International Conference on Communication Technology, Nanjing, China, 2010: 983–986. doi: 10.1109/ICCT.2010.5688787. [10] WU Jinwen, JIN Haihong, YU Shujuan, et al. A novel blind detection algorithm based on multi-clustering complex neural network[C]. Proceedings of 2021 IEEE 4th Advanced Information Management, Communicates, Electronic and Automation Control Conference (IMCEC), Chongqing, China, 2021: 1017–1021. doi: 10.1109/IMCEC51613.2021.9482354. [11] MEI Ruru, WANG Zhugang, and HU Wanru. Robust blind equalization algorithm using convolutional neural network[J]. IEEE Signal Processing Letters, 2022, 29: 1569–1573. doi: 10.1109/LSP.2022.3189319. [12] XIE Xihai, FAN Chenzhao, WANG Shuai, et al. Varible step size multi-layer neural network blind equalization algorithm[C]. 2022 4th International Conference on Natural Language Processing (ICNLP), Xi’an, China, 2022: 477–481. doi: 10.1109/ICNLP55136.2022.00087. [13] ZHANG Hui, GU M, JIANG Xudong, et al. An optical neural chip for implementing complex-valued neural network[J]. Nature Communications, 2021, 12(1): 457. doi: 10.1038/s41467-020-20719-7. [14] YU Yaning and ZHANG Ziye. State estimation for complex-valued inertial neural networks with multiple time delays[J]. Mathematics, 2022, 10(10): 1725. doi: 10.3390/math10101725. [15] HONG Qinghui, FU Haotian, LIU Yiyang, et al. In-memory computing circuit implementation of complex-valued hopfield neural network for efficient portrait restoration[J]. IEEE Transactions on Computer-Aided Design of Integrated Circuits and Systems, 2023, 42(10): 3338–3351. doi: 10.1109/TCAD.2023.3242858. [16] NISHIKAWA I, IRITANI T, and SAKAKIBARA K. Improvements of the traffic signal control by complex-valued Hopfield networks[C]. The 2006 IEEE International Joint Conference on Neural Network Proceedings, Vancouver, Canada, 2006: 459–464. doi: 10.1109/IJCNN.2006.246717. [17] 王雷敏, 程佳俊, 胡成, 等. 基于改进的忆阻器在字符联想记忆中的应用[J]. 电子与信息学报, 2023, 45(7): 2667–2674. doi: 10.11999/JEIT220709.WANG Leimin, CHENG Jiajun, HU Cheng, et al. Application of improved memristor in character associative memory[J]. Journal of Electronics & Information Technology, 2023, 45(7): 2667–2674. doi: 10.11999/JEIT220709. [18] UYKAN Z. Shadow-Cuts minimization/maximization and complex Hopfield neural networks[J]. IEEE Transactions on Neural Networks and Learning Systems, 2021, 32(3): 1096–1109. doi: 10.1109/TNNLS.2020.2980237. [19] HONG Qinghui, LI Ya, and WANG Xiaoping. Memristive continuous Hopfield neural network circuit for image restoration[J]. Neural Computing and Applications, 2020, 32(12): 8175–8185. doi: 10.1007/s00521-019-04305-7. [20] YAN Renao, HONG Qinghui, WANG Chunhua, et al. Multilayer memristive neural network circuit based on online learning for license plate detection[J]. IEEE Transactions on Computer-Aided Design of Integrated Circuits and Systems, 2022, 41(9): 3000–3011. doi: 10.1109/TCAD.2021.3121347. [21] 肖平旦, 洪庆辉, 杜四春, 等. 基于忆阻器的可编程矩阵行列式求解电路设计及应用[J]. 中国科学: 信息科学, 2023, 53(5): 1008–1025. doi: 10.1360/SSI-2022-0229.XIAO Pingdan, HONG Qinghui, DU Sichun, et al. Design and application of a programmable matrix determinant-solving circuit based on memristors[J]. SCIENTIA SINICA Informationis, 2023, 53(5): 1008–1025. doi: 10.1360/SSI-2022-0229. [22] YI Qian. FPGA implementation of neural network accelerator[C]. 2018 2nd IEEE Advanced Information Management, Communicates, Electronic and Automation Control Conference (IMCEC), Xi’an, China, 2018: 1903–1906. doi: 10.1109/IMCEC.2018.8469659. -






 下载:
下载:


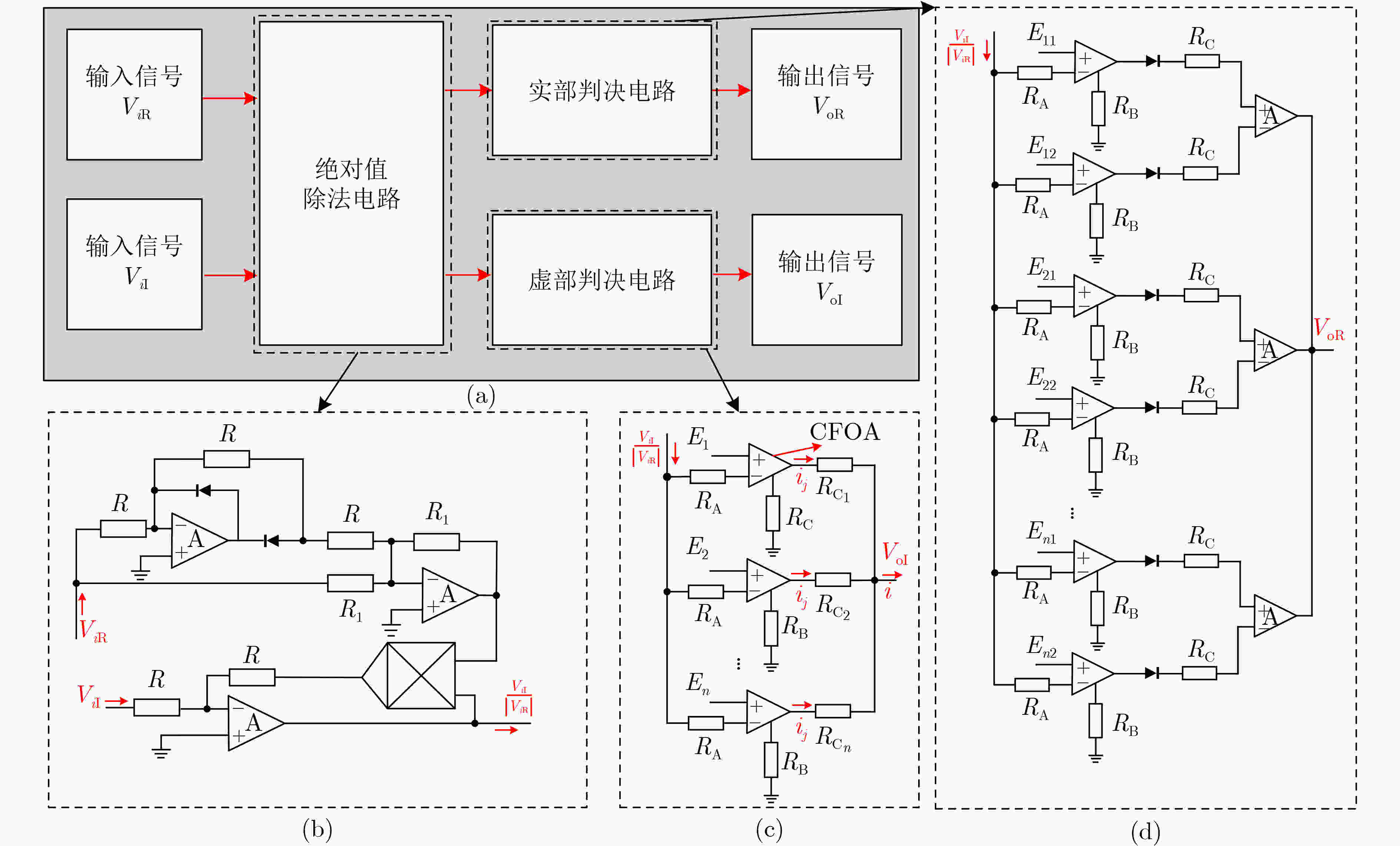



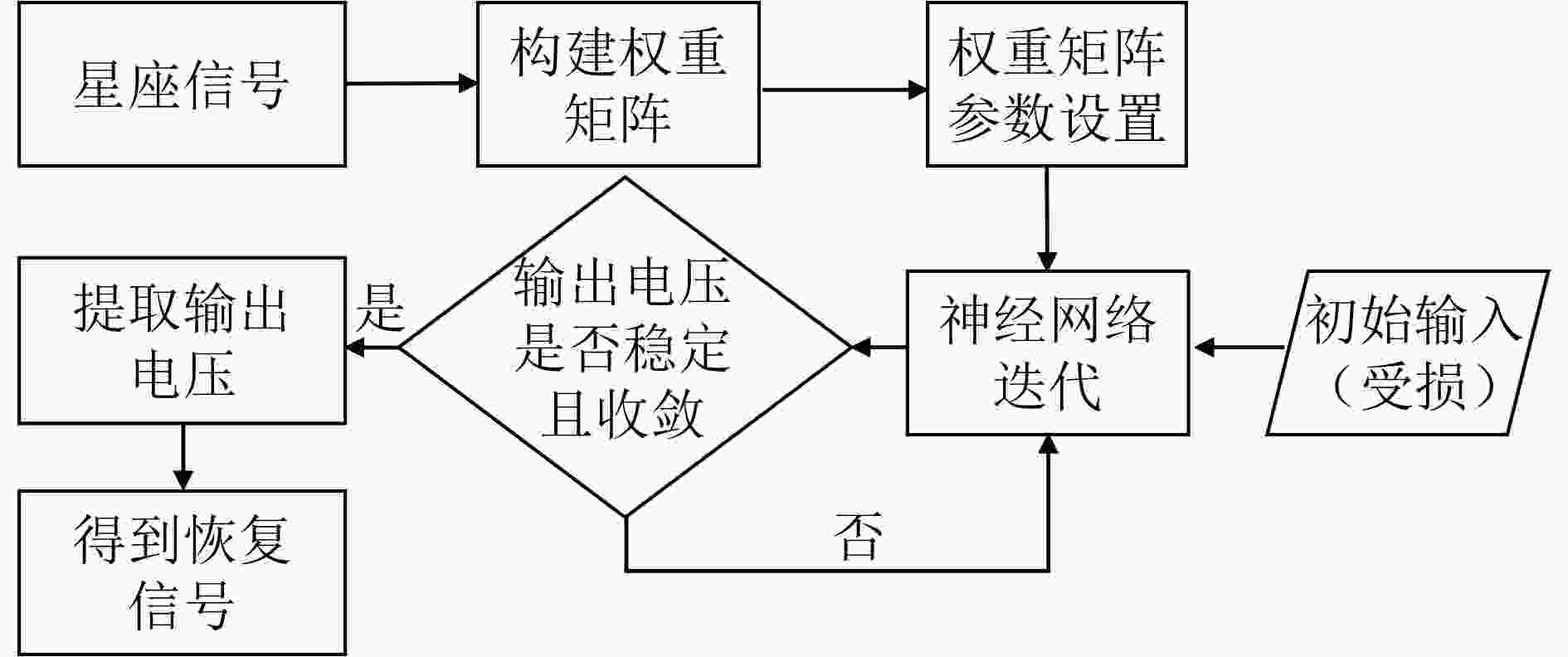

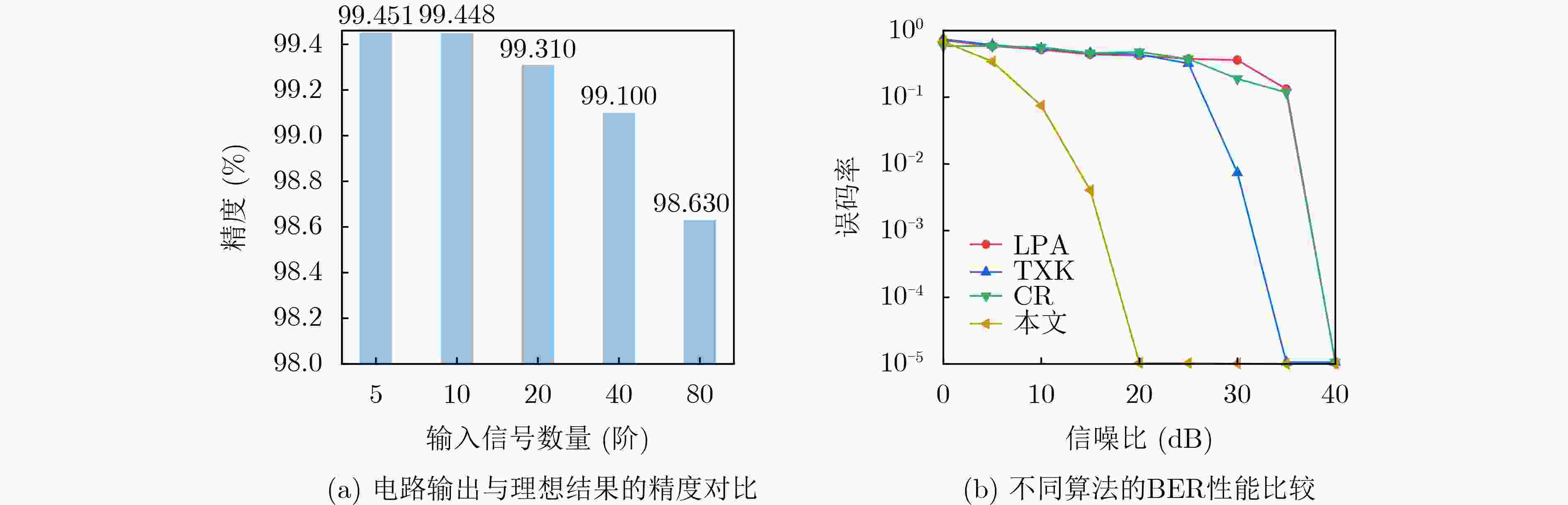






 下载:
下载:
