A Fast Carrier Frequency Offset Position Detection Algorithm for Passive Backscatter Communication System
-
摘要: 近来无源反向散射通信技术作为绿色物联网的关键技术，引起了广泛的关注。在无源反向散射通信系统中，收发节点与反射节点的振荡器差异、相对运动以及环境变化，导致接收端和发送端存在载波频率偏移(CFO)。CFO对信号检测和系统性能有重要影响，而当前多数无源反向散射通信系统研究忽略了CFO。该文设计了一种适用于频移键控调制(FSK)的CFO快速检测方法，不需要导频就能快速有效检测出CFO是否存在并找出存在的位置。首先，根据信号在CFO存在与否的不同，采用去直流后取模方法对信号进行处理，而后根据处理后的信号的特点，基于累积和(CUSUM)设计快速检测算法对载波频偏出现的位置进行检测，并对理论分析结果进行仿真验证，仿真结果表明取模检测可以有效检测出CFO出现的位置。Abstract: Recently, passive backscatter communication technology has attracted extensive attention as one key technology for green Internet of Things. In one typical backscatter communication system, there may exist Carrier Frequency Offset (CFO) between the receiver and the transmitter due to the relative motion or the difference in oscillators or environmental changes. CFO has an important impact on signal detection and system performance, but most current studies ignore the CFO. In this paper, a fast CFO detection method suitable for Frequency Shift Keying (FSK) modulation is designed, which can quickly and effectively detect without pilots whether there exists CFO and find out the location where the CFO begins. Specifically, this paper designs one detector amplitude-taking method. Next, based on CUmulative SUM (CUSUM) algorithm a fast detection algorithm is designed to detect the location of CFO. Finally, simulation results are provided to corroborate the proposed studies. The simulation results show that the designed detector can effectively locate the position where CFO appears.
-
[1] 张晓茜, 徐勇军. 面向零功耗物联网的反向散射通信综述[J]. 通信学报, 2022, 43(11): 199–212. doi: 10.11959/j.issn.1000-436x.2022199ZHANG Xiaoxi and XU Yongjun. Survey on backscatter communication for zero-power IoT[J]. Journal on Communications, 2022, 43(11): 199–212. doi: 10.11959/j.issn.1000-436x.2022199 [2] XING Chengwen, JING Yindi, WANG Shuai, et al. New viewpoint and algorithms for water-filling solutions in wireless communications[J]. IEEE Transactions on Signal Processing, 2020, 68: 1618–1634. doi: 10.1109/TSP.2020.2973488 [3] CAO Yinwen, YU Song, SHEN Jing, et al. Frequency estimation for optical coherent MPSK system without removing modulated data phase[J]. IEEE Photonics Technology Letters, 2010, 22(10): 691–693. doi: 10.1109/LPT.2010.2044170 [4] LEVEN A, KANEDA N, KOC U V, et al. Frequency estimation in intradyne reception[J]. IEEE Photonics Technology Letters, 2007, 19(6): 366–368. doi: 10.1109/LPT.2007.891893 [5] FATADIN I and SAVORY S J. Compensation of frequency offset for 16-QAM optical coherent systems using QPSK partitioning[J]. IEEE Photonics Technology Letters, 2011, 23(17): 1246–1248. doi: 10.1109/LPT.2011.2158994 [6] HUANG Dezhao, CHENG T H, and YU Changyuan. Accurate two-stage frequency offset estimation for coherent optical systems[J]. IEEE Photonics Technology Letters, 2013, 25(2): 179–182. doi: 10.1109/LPT.2012.2232288 [7] YANG Tao, SHI Chen, CHEN Xue, et al. Hardware-efficient multi-format frequency offset estimation for M-QAM coherent optical receivers[J]. IEEE Photonics Technology Letters, 2018, 30(18): 1605–1608. doi: 10.1109/LPT.2018.2863739 [8] KIM J W, LEE Y S, JIN M Y, et al. Carrier frequency offset estimation for OFDM system with large oscillator phase noise[C]. 2021 International Conference on Information and Communication Technology Convergence (ICTC), Jeju Island, Korea, 2021: 368–370. [9] ZHENG Shuai, CHEN Jian, KUO Yonghong, et al. Statistical histogram-based blind frequency offset estimation for MPSK and MQAM signal[C]. 2022 International Conference on Machine Learning and Knowledge Engineering (MLKE), Guilin, China, 2022: 125–129. [10] JAYAPRAKASH A and REDDY G R. Covariance-fitting-based blind carrier frequency offset estimation method for OFDM systems[J]. IEEE Transactions on Vehicular Technology, 2016, 65(12): 10101–10105. doi: 10.1109/TVT.2016.2542181 [11] PAGE E S. A test for a change in a parameter occurring at an unknown point[J]. Biometrika, 1955, 42(3/4): 523–527. doi: 10.2307/2333401 [12] KESHAVARZ H, SCOTT C, and NGUYEN X. Optimal change point detection in Gaussian processes[J]. Journal of Statistical Planning and Inference, 2018, 193: 151–178. doi: 10.1016/j.jspi.2017.09.003 [13] KO S I M, CHONG TT L, and GHOSH P. Dirichlet process hidden Markov multiple change-point model[J]. Bayesian Analysis, 2015, 10(2): 275–296. doi: 10.1214/14-BA910 [14] KOKOSZKA P and LEIPUS R. Change-point in the mean of dependent observations[J]. Statistics & Probability Letters, 1998, 40(4): 385–393. doi: 10.1016/S0167-7152(98)00145-X [15] NA O, LEE Y, and LEE S. Monitoring parameter change in time series models[J]. Statistical Methods & Applications, 2011, 20(2): 171–199. doi: 10.1007/s10260-011-0162-3 [16] LING Jin, LI Xiaoqin, YANG Wenzhi, et al. The CUSUM statistic of change point under NA sequences[J]. Applied Mathematics-A Journal of Chinese Universities, 2021, 36(4): 512–520. doi: 10.1007/s11766-021-4015-z [17] YU Yuncai and CHEN Zhicheng. Strong convergence rates of multiple change-point estimator for ρ-mixing sequence[J]. Communications in Statistics - Theory and Methods, 2023, 52(13): 4605–4621. doi: 10.1080/03610926.2021.1998532 [18] KIM K, PARK J H, LEE M, et al. Unsupervised change point detection and trend prediction for financial time-series using a new CUSUM-based approach[J]. IEEE Access, 2022, 10: 34690–34705. doi: 10.1109/ACCESS.2022.3162399 -
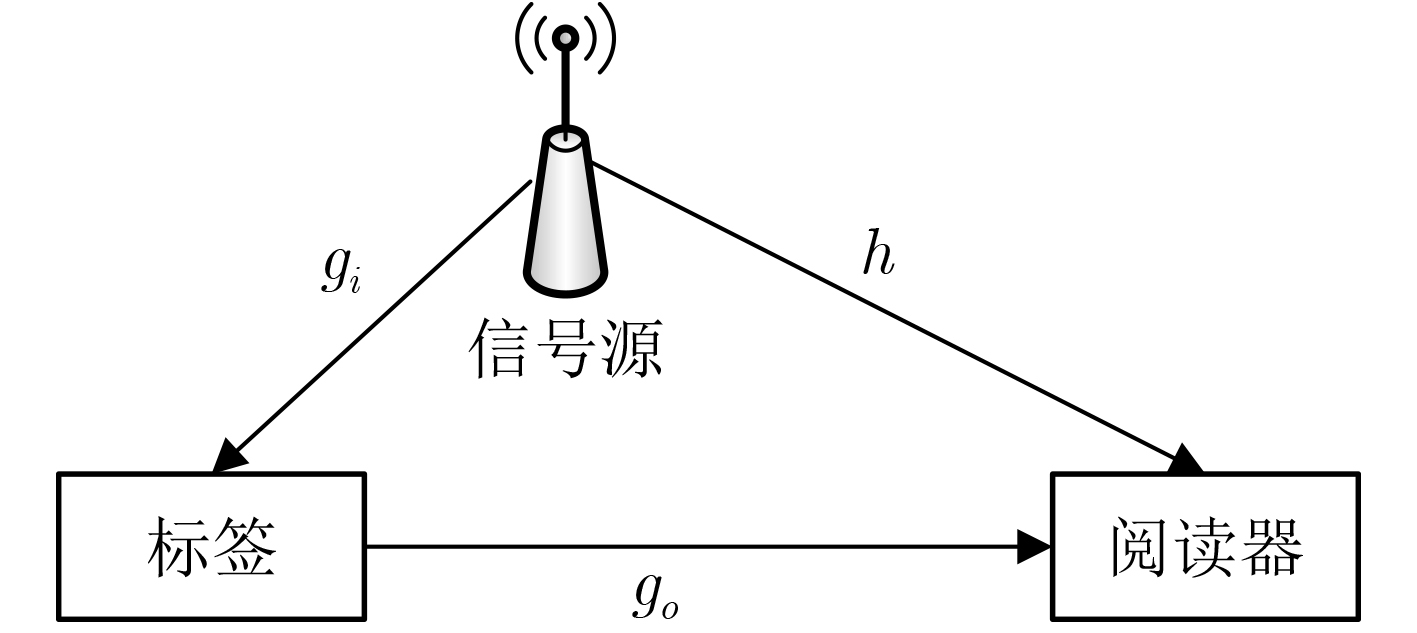
下载:
图(5)
计量
- 文章访问数: 616
- HTML全文浏览量: 180
- PDF下载量: 153
- 被引次数: 0
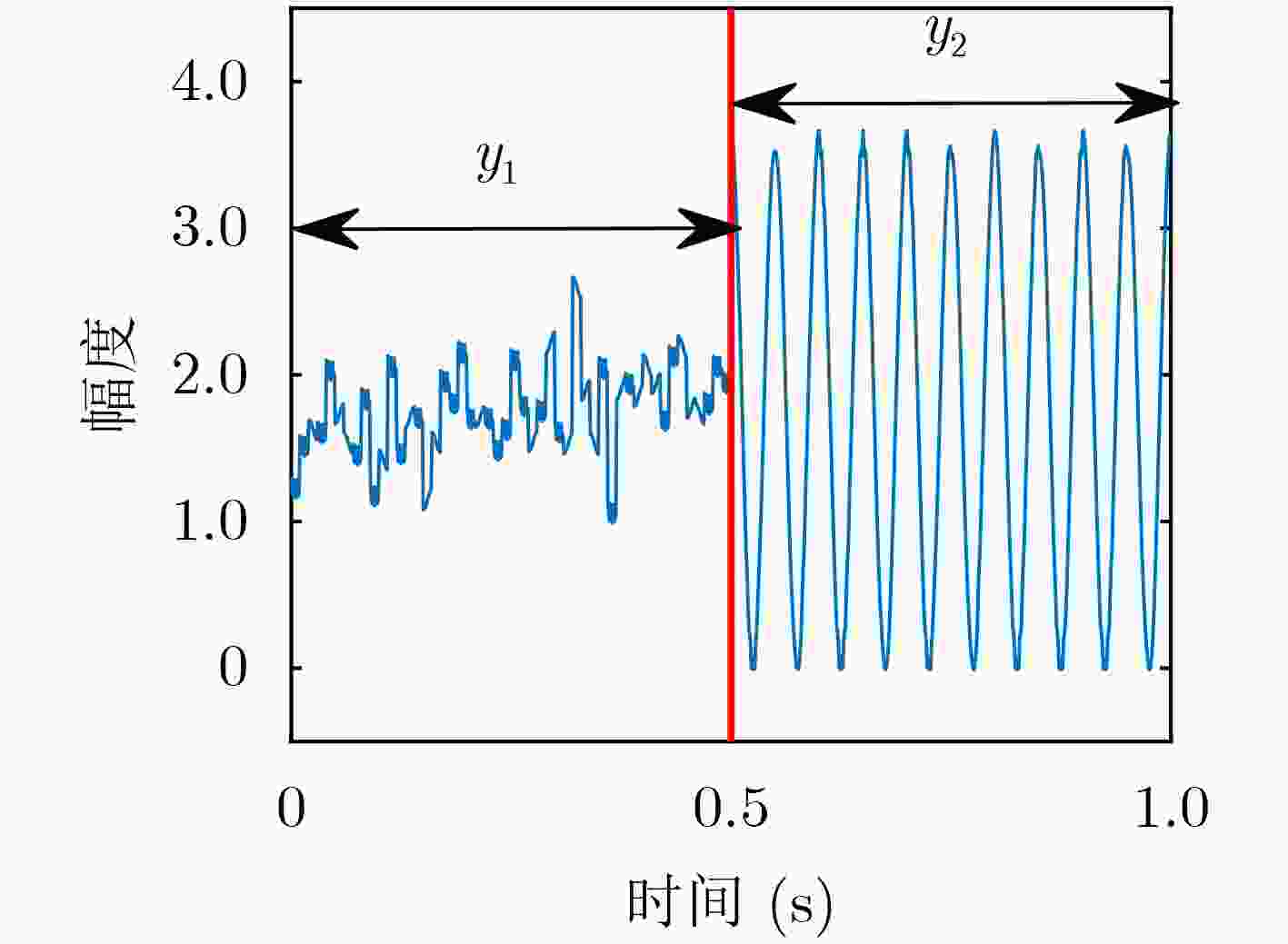
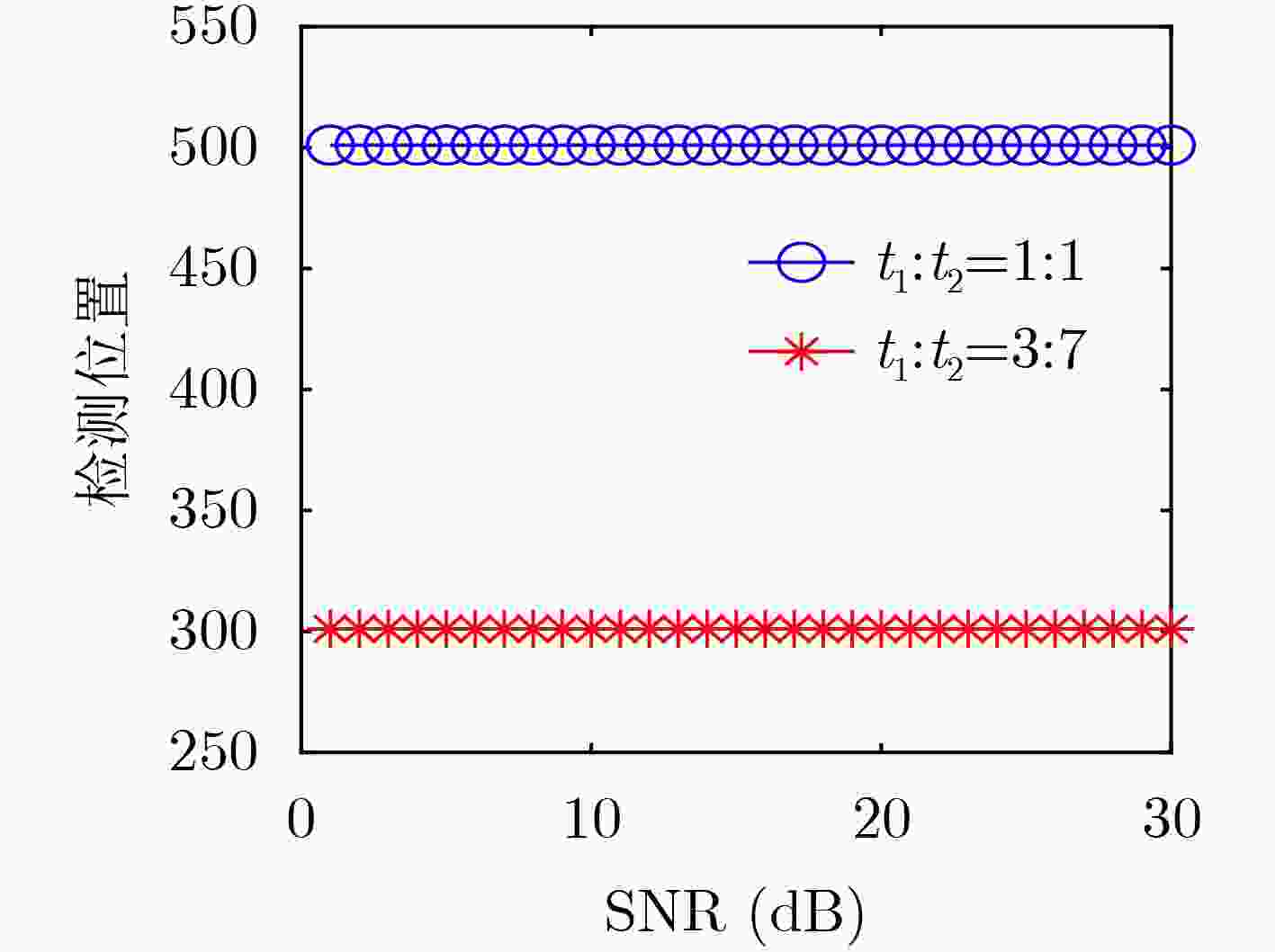
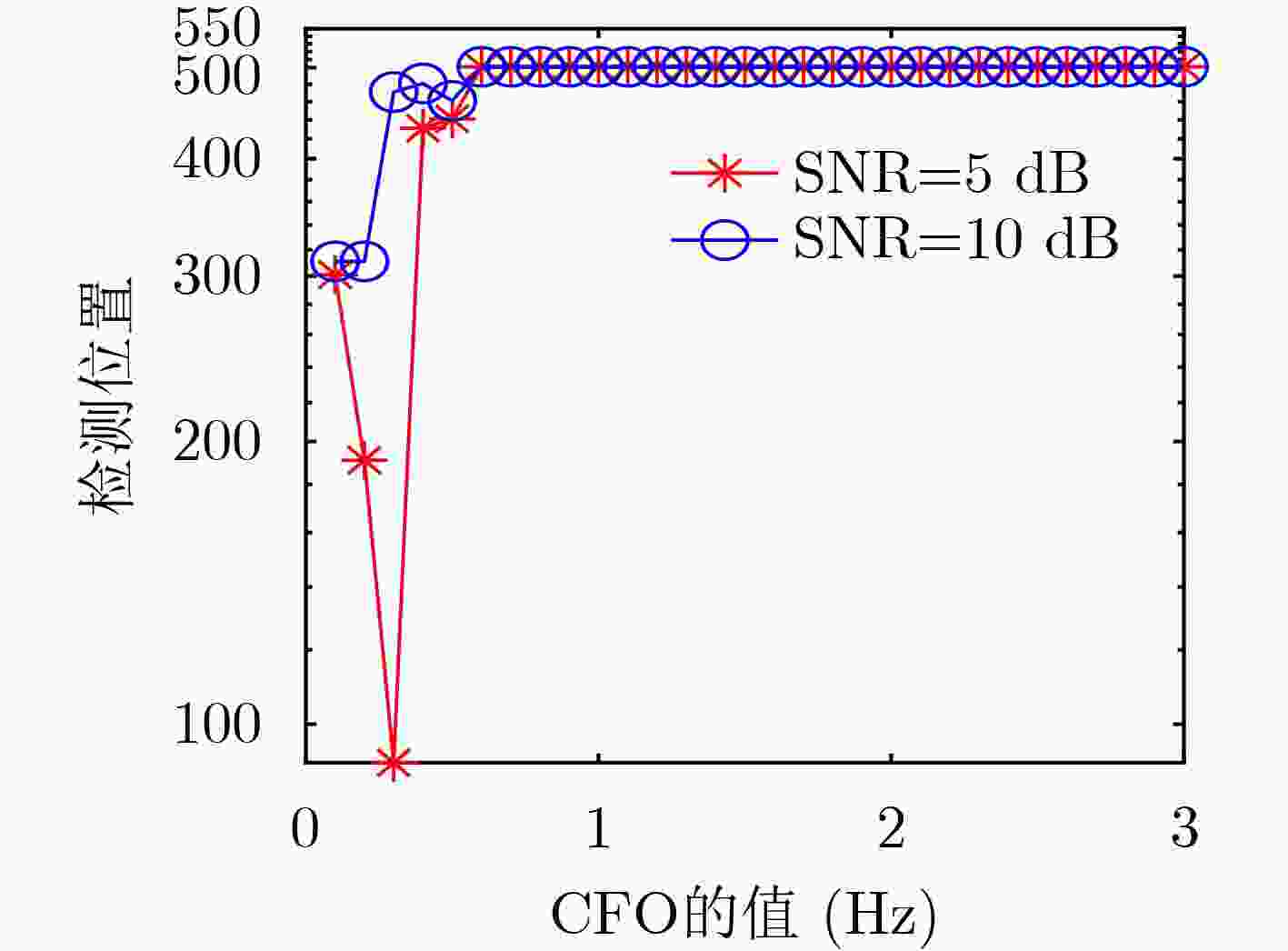
下载:
