Robust Beamforming Algorithm Based on Double-layer Estimation of Steering Vector and Covariance Matrix Reconstruction
-
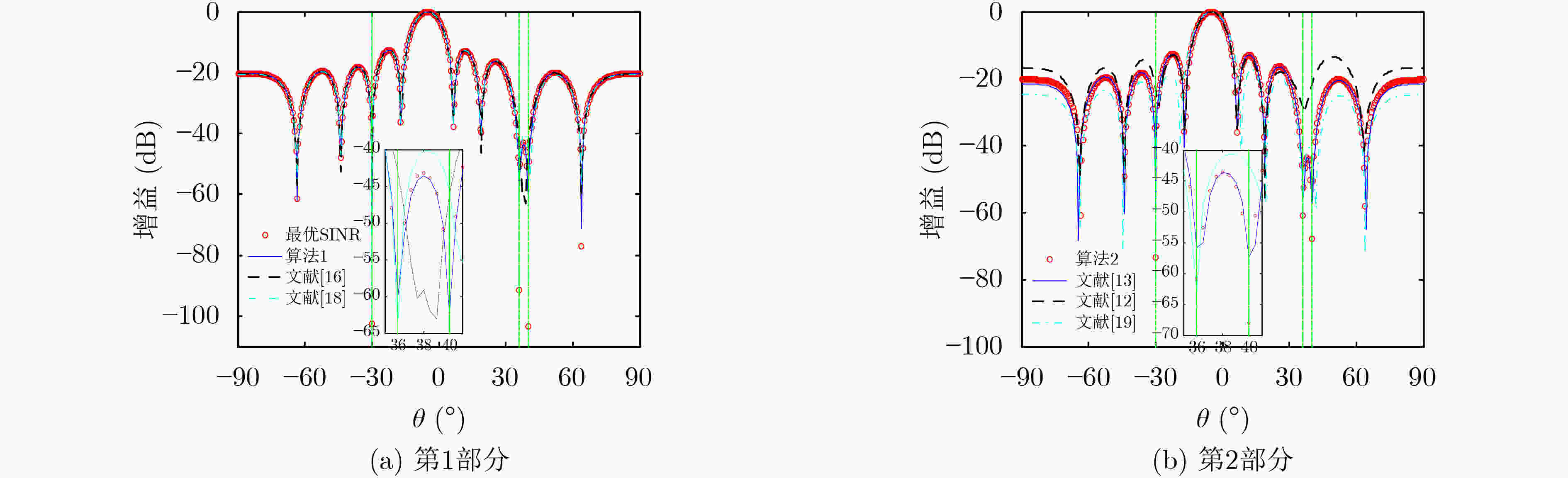
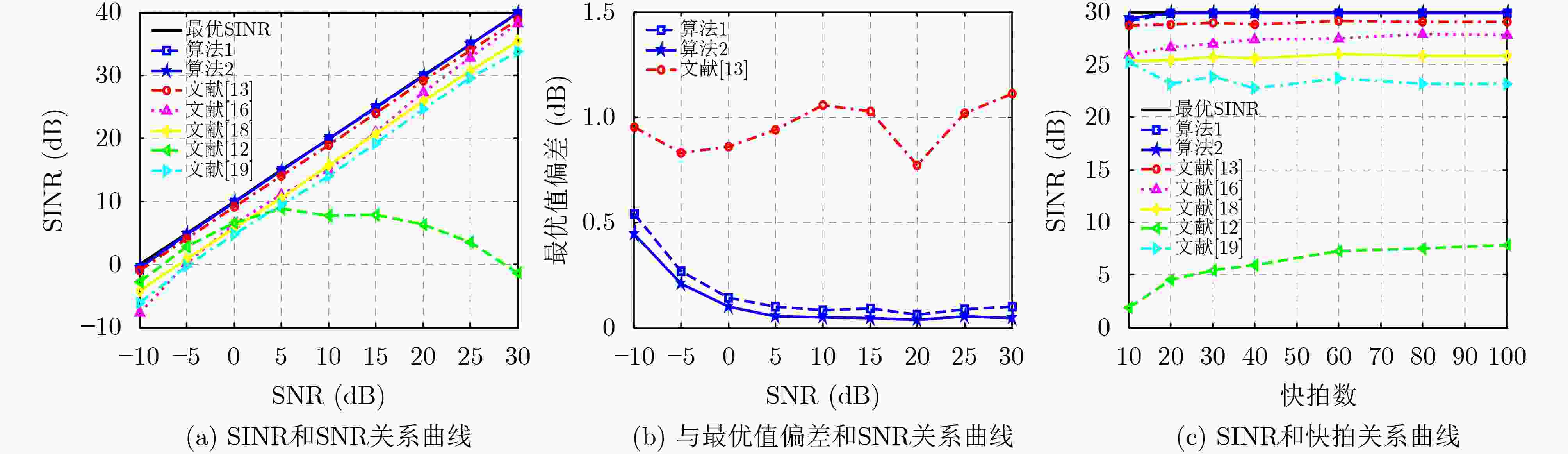
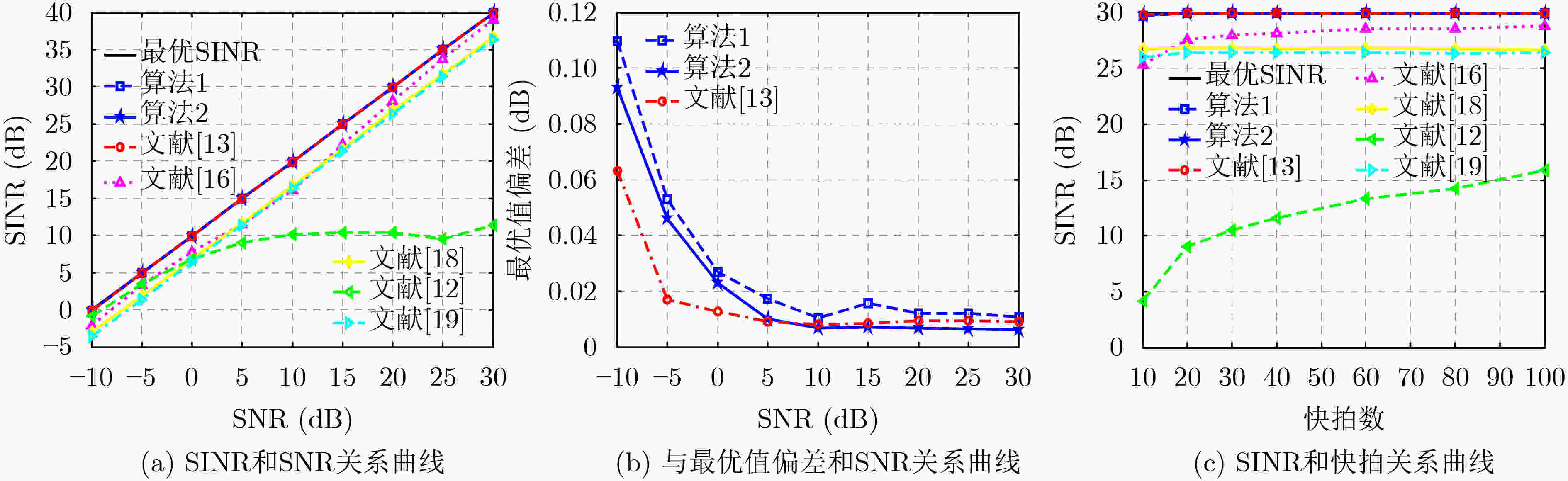
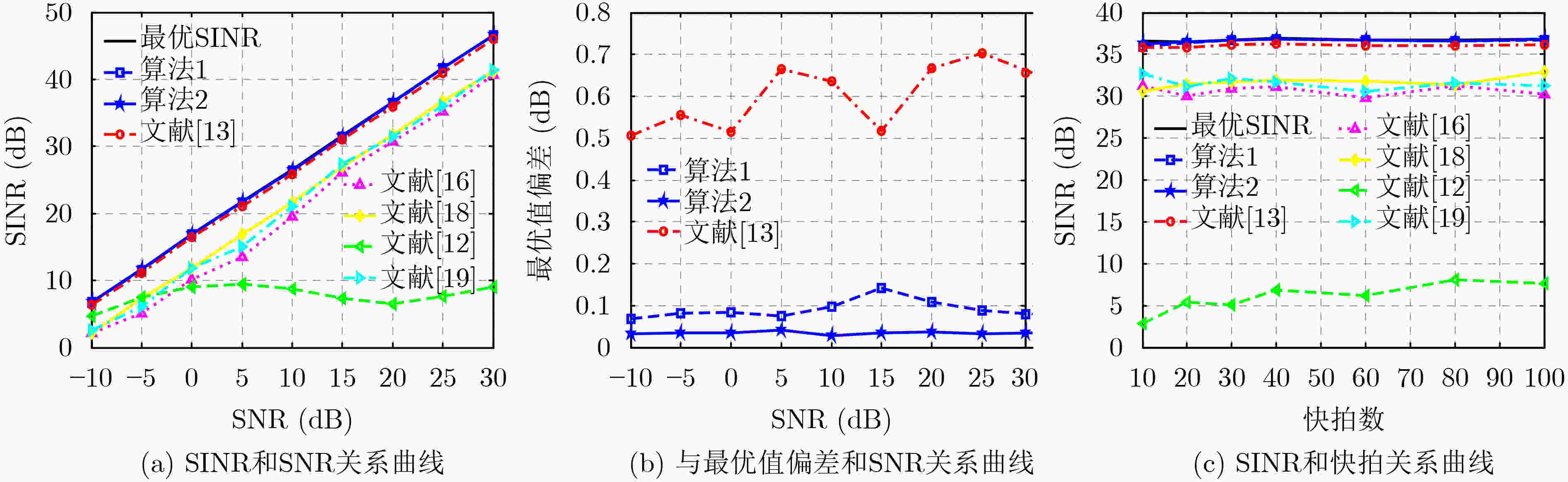
摘要: 针对干扰加噪声协方差矩阵(INCM)重构过程中Capon功率谱(CPS)估计分辨率低的问题,该文提出两种稳健自适应波束形成(RAB)算法。该算法首先通过搜索CPS的峰值确定积分区间,然后对各区间积分所得的协方差矩阵进行特征值分解。通过合理设置判定门限确定区间内所含的入射信源数量,并将较大特征值所对应的特征向量作为信源导向矢量(SV)的初步估计。而后通过最大化估计功率的方法,在初步估计SV的正交空间内搜索其与真实SV之间的误差。该算法1利用最小特征值所对应的特征向量,向初步估计的SV中添加正交比例梯度,得到双层估计的SV。与算法1不同,算法2通过求解2次优化(QP)问题得到修正的SV。最后通过重构INCM获得阵列最优权值矢量。通过计算机仿真实验,验证了所提算法有效解决了CPS估计分辨率低的问题,较其他算法综合性能更优,具备更高的稳健性。
-
关键词:
- 稳健自适应波束形成 /
- 协方差矩阵重构 /
- 导向矢量估计 /
- Capon功率谱估计
Abstract: Considering the problem of the low resolution of the Capon Power Spectrum (CPS) in the reconstruction of Interference plus Noise Covariance Matrix (INCM), two Robust Adaptive Beamforming (RAB) algorithms are proposed. The proposed algorithm first searches the peaks of CPS to determine the integration intervals and then eigen-decomposes the covariance matrixes obtained from the integration of each interval. The number of incident sources in the interval is determined by reasonably setting the decision threshold, and the eigenvectors corresponding to the larger eigenvalues are used as the preliminary estimation of the Steering Vectors (SV). Then, by maximizing the estimated power, the gap between the nominal SV and the real SV is searched in the orthogonal space of the nominal SV. The first proposed algorithm uses the eigenvector corresponding to the minimum eigenvalue to add the orthogonal proportional gradient to the initial estimated SV to obtain the double-layer estimated SV. The second proposed algorithm obtains the modified SV by solving a Quadratic Programming (QP) problem. Finally, the optimal weight vector of the array is obtained by reconstructing the INCM. Simulation results demonstrate that the proposed algorithm solves effectively the problem of the low resolution of the CPS estimation and is superior to other algorithms. -
表 1 所提算法步骤
序号 内容 步骤 1 利用式(13)计算CPS,并搜索CPS的峰值; 步骤 2 利用峰值确定积分区间,并使用式(17)计算区间积分; 步骤 3 利用式(18)特征值分解积分所得的矩阵,使用式(19)确定入射信号数量; 步骤 4 算法1利用式(20)构造一组SV,并计算最优SV;
算法2利用式(23)求解误差向量,并计算最优SV;步骤 5 利用式(21)和式(22)重构INCM,使用式(24)计算阵列权值矢量。 -
[1] BISWAS R N, MITRA S K, and NASKAR M K. Wireless node localization under hostile radio environment using smart antenna[J]. Wireless Personal Communications, 2021, 116(3): 1815–1836. doi: 10.1007/s11277-020-07763-8 [2] JAYAKRISHNAN V M and VIJAYAN D M. Performance analysis of smart antenna for marine communication[C]. The 2nd International Conference on Innovative Mechanisms for Industry Applications (ICIMIA), Bangalore, India, 2020: 88–91. [3] GROSS F. Smart Antennas with MATLAB[M]. 2nd ed. New York: McGraw-Hill Education, 2015: 148–149. [4] KUHN E V, PITZ C A, MATSUO M V, et al. A kronecker product CLMS algorithm for adaptive beamforming[J]. Digital Signal Processing, 2021, 111: 102968. doi: 10.1016/j.dsp.2021.102968 [5] 杨志伟, 张攀, 陈颖, 等. 导向矢量和协方差矩阵联合迭代估计的稳健波束形成算法[J]. 电子与信息学报, 2018, 40(12): 2874–2880. doi: 10.11999/JEIT180225YANG Zhiwei, ZHANG Pan, CHEN Ying, et al. Steering vector and covariance matrix joint iterative estimations for robust beamforming[J]. Journal of Electronics &Information Technology, 2018, 40(12): 2874–2880. doi: 10.11999/JEIT180225 [6] BYRNE D and CRADDOCK I J. Time-domain wideband adaptive beamforming for radar breast imaging[J]. IEEE Transactions on Antennas and Propagation, 2015, 63(4): 1725–1735. doi: 10.1109/TAP.2015.2398125 [7] FUKUE T, FUJITA A, and HAMADA N. Estimation of target position by the combination of MUSIC and adaptive beamforming in stepped-FM array radar[J]. IEICE Transactions on Information and Systems, 2015, E88-D(7): 1453–1456. doi: 10.1093/ietisy/e88-d.7.1453 [8] 唐敏, 齐栋, 刘成城, 等. 基于多级阻塞的稳健相干自适应波束形成[J]. 电子与信息学报, 2019, 41(7): 1705–1711. doi: 10.11999/JEIT180332TANG Min, QI Dong, LIU Chengcheng, et al. New adaptive beamformer for coherent interference based on multistage blocking[J]. Journal of Electronics &Information Technology, 2019, 41(7): 1705–1711. doi: 10.11999/JEIT180332 [9] CHEN Pei, ZHAO Yongjun, and LIU Chengcheng. Robust adaptive beamforming using a low-complexity steering vector estimation and covariance matrix reconstruction algorithm[J]. International Journal of Antennas and Propagation, 2016, 2016: 2438183. doi: 10.1155/2016/2438183 [10] ZHANG Ming, ZHANG Anxue, and YANG Qingqing. Robust adaptive beamforming based on conjugate gradient algorithms[J]. IEEE Transactions on Signal Processing, 2016, 64(22): 6046–6057. doi: 10.1109/TSP.2016.2605075 [11] 刘福来, 陈萍萍, 汪晋宽, 等. 基于多参数二次规划的零陷展宽和旁瓣控制方法[J]. 东北大学学报:自然科学版, 2012, 33(11): 1559–1562.LIU Fulai, CHEN Pingping, WANG Jinkuan, et al. Null broadening and sidelobe control method based on multiparametric quadratic programming[J]. Journal of Northeastern University:Natural Science, 2012, 33(11): 1559–1562. [12] LEE C C and LEE J H. Eigenspace-based adaptive array beamforming with robust capabilities[J]. IEEE Transactions on Antennas and Propagation, 1997, 45(12): 1711–1716. doi: 10.1109/8.650188 [13] GU Yujie and LESHEM A. Robust adaptive beamforming based on interference covariance matrix reconstruction and steering vector estimation[J]. IEEE Transactions on Signal Processing, 2012, 60(7): 3881–3885. doi: 10.1109/TSP.2012.2194289 [14] GU Yujie, GOODMAN N A, HONG Shaohua, et al. Robust adaptive beamforming based on interference covariance matrix sparse reconstruction[J]. Signal Processing, 2014, 96: 375–381. doi: 10.1016/j.sigpro.2013.10.009 [15] YUAN Xiaolei and GAN Lu. Robust adaptive beamforming via a novel subspace method for interference covariance matrix reconstruction[J]. Signal Processing, 2017, 130: 233–242. doi: 10.1016/j.sigpro.2016.07.008 [16] ZHENG Zhi, ZHENG Yan, WANG Wenqin, et al. Covariance matrix reconstruction with interference steering vector and power estimation for robust adaptive beamforming[J]. IEEE Transactions on Vehicular Technology, 2018, 67(9): 8495–8503. doi: 10.1109/TVT.2018.2849646 [17] ZHANG Zhenyu, LIU Wei, LENG Wen, et al. Interference-plus-noise covariance matrix reconstruction via spatial power spectrum sampling for robust adaptive beamforming[J]. IEEE Signal Processing Letters, 2016, 23(1): 121–125. doi: 10.1109/LSP.2015.2504954 [18] SUN Sicong and YE Zhongfu. Robust adaptive beamforming based on a method for steering vector estimation and interference covariance matrix reconstruction[J]. Signal Processing, 2021, 182: 107939. doi: 10.1016/j.sigpro.2020.107939 [19] ZHU Xingyu, YE Zhongfu, XU Xu, et al. Covariance matrix reconstruction via residual noise elimination and interference powers estimation for robust adaptive beamforming[J]. IEEE Access, 2019, 7: 53262–53272. doi: 10.1109/ACCESS.2019.2912402 [20] YANG Jian, LU Jian, LIU Xinxin, et al. Robust null broadening beamforming based on covariance matrix reconstruction via virtual interference sources[J]. Sensors, 2020, 20(7): 1865. doi: 10.3390/s20071865 [21] GRANT M, BOYD S, and YE Y. CVX: Matlab software for disciplined convex programming, version 2.2[EB/OL]. http://cvxr.com/cvx, 2020. -





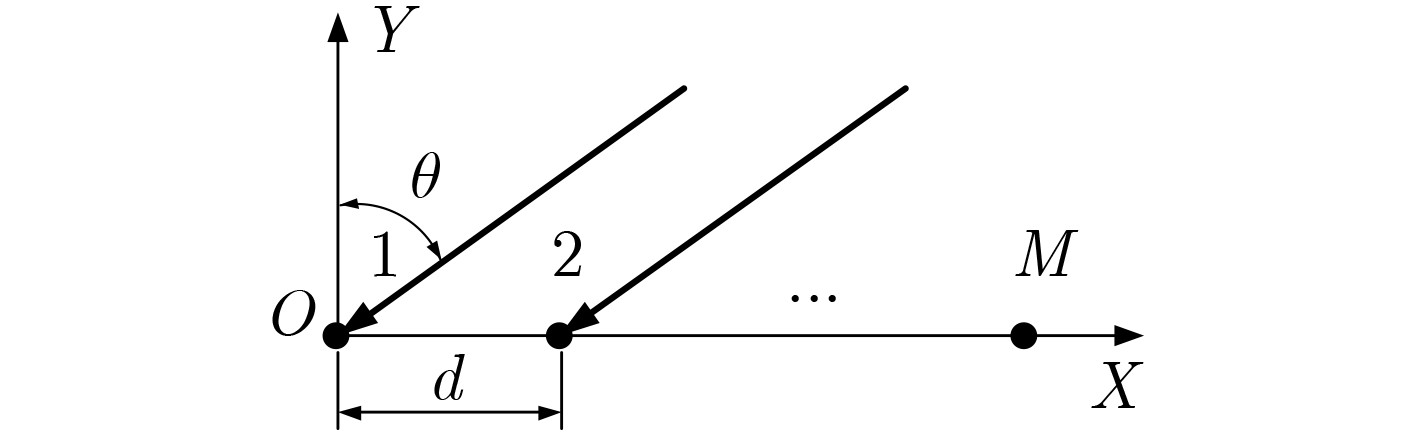
 下载:
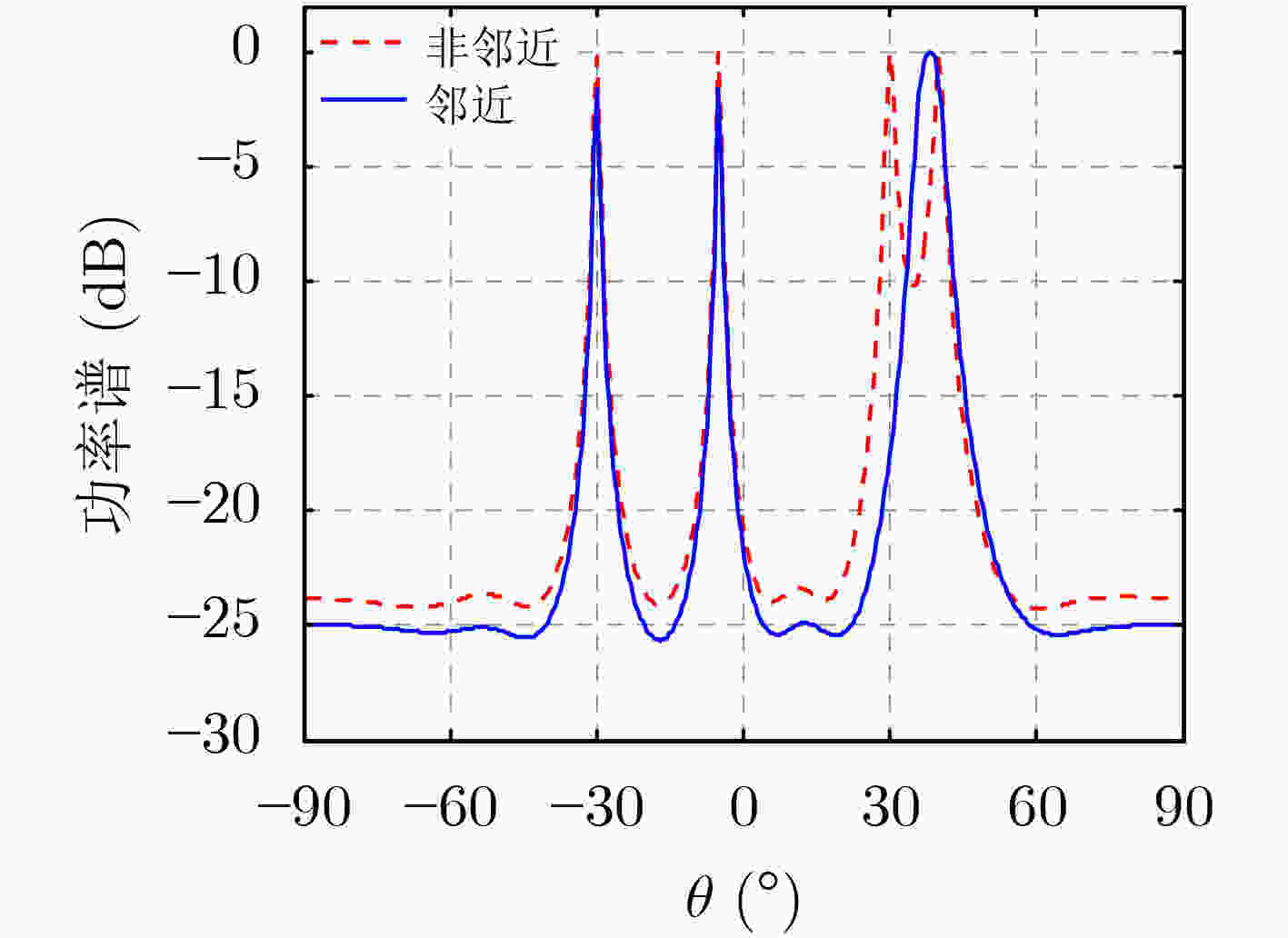
下载:
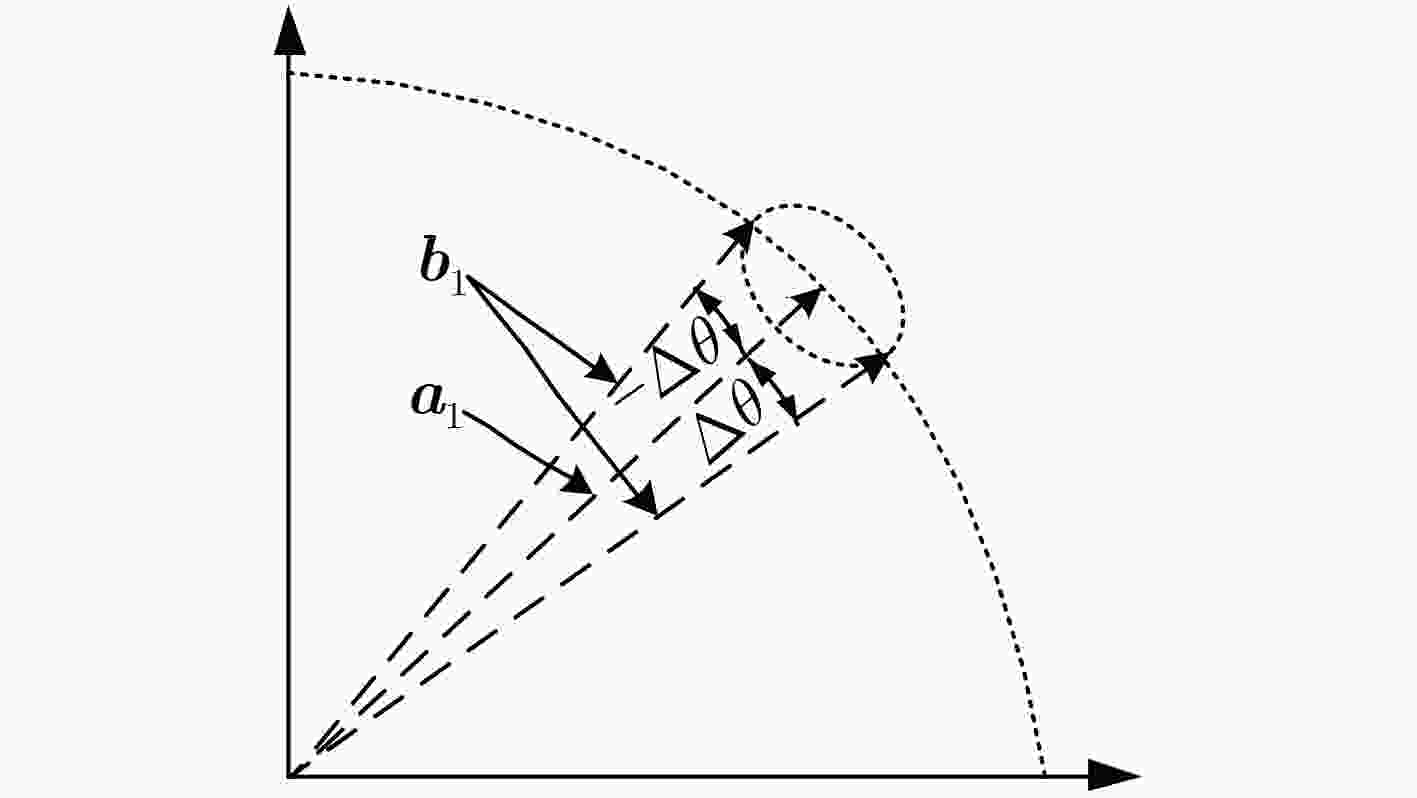



 下载:
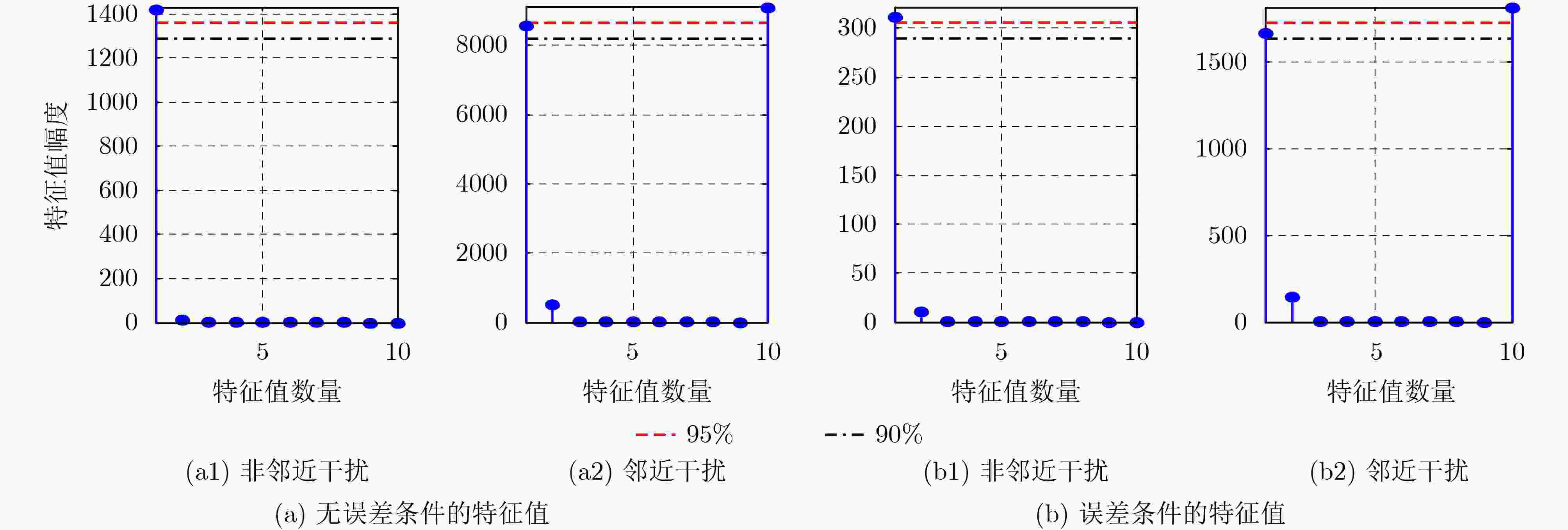
下载:
