D2D通信中一种基于非数据辅助误差适量幅度的同信道干扰控制方法及其性能分析

曾孝平; 李诗琪; 杨凡; 简鑫; 吴继森

doi:10.11999/JEIT200473

D2D通信中一种基于非数据辅助误差适量幅度的同信道干扰控制方法及其性能分析

doi: 10.11999/JEIT200473

1.
重庆大学通信工程学院重庆 400044
2.
重庆理工大学电气与电子工程学院重庆 400054
3.
重庆通用航空集团有限公司重庆 401135

基金项目: 国家自然科学基金(61501065, 61571069, 61601067, 61701054)；重庆市基础科学与前沿技术研究专项一般基金资助项目(cstc2016jcyjA0021)；中央高校基本科研业务费(106112016CDJXY160001)；重庆市重大主题专项(cstc2019jscx-zdztzxX0045, cstc2019jscx-zdztzxX0029, cstc2019jscx-zdztzxX0051)

详细信息

作者简介:
曾孝平：男，1965年生，教授，研究方向为下一代移动通信、无线通信、空间信息网

李诗琪：女，1996年生，硕士生，研究方向为下一代移动通信、干扰检测

杨凡：男，1983年生，教授，研究方向为无线宽带自适应传输、无线通信网络

简鑫：男，1987年生，副教授，研究方向为下一代移动通信、多入多出网络

吴继森：男，1966年生，高级工程师，研究方向为飞行器设计、航空通信导航设计

通讯作者:
曾孝平　zxp@cqu.edu.cn

中图分类号: TN929.5
计量
- 文章访问数: 743
- HTML全文浏览量: 387
- PDF下载量: 42
- 被引次数: 0
出版历程
- 收稿日期: 2020-06-11
- 修回日期: 2020-12-31
- 网络出版日期: 2021-02-26
- 刊出日期: 2021-09-16

NDA-EVM Based Co-channel Interference Control Method and Performance Analysis in D2D Communication

1.
College of Communication Engineering, Chongqing University, Chongqing 400044, China
2.
School of Electrical and Electronic Engineering, Chongqing University of Technology, Chongqing 400054, China
3.
Chongqing General Aviation Co., Ltd (CQGA), Chongqing 401135, China

Funds: The National Natural Science Foundation of China (61501065, 61571069, 61601067, 61701054), The Chongqing Research Program of Basic Research and Frontier Technology (cstc2016jcyjA0021), The Fundamental Research Funds for the Central Universities (106112016CDJXY160001), The Chongqing Technology Innovation and Application Development Key Project (cstc2019jscx-zdztzxX0045, cstc2019jscx-zdztzxX0029, cstc2019jscx-zdztzxX0051)

摘要

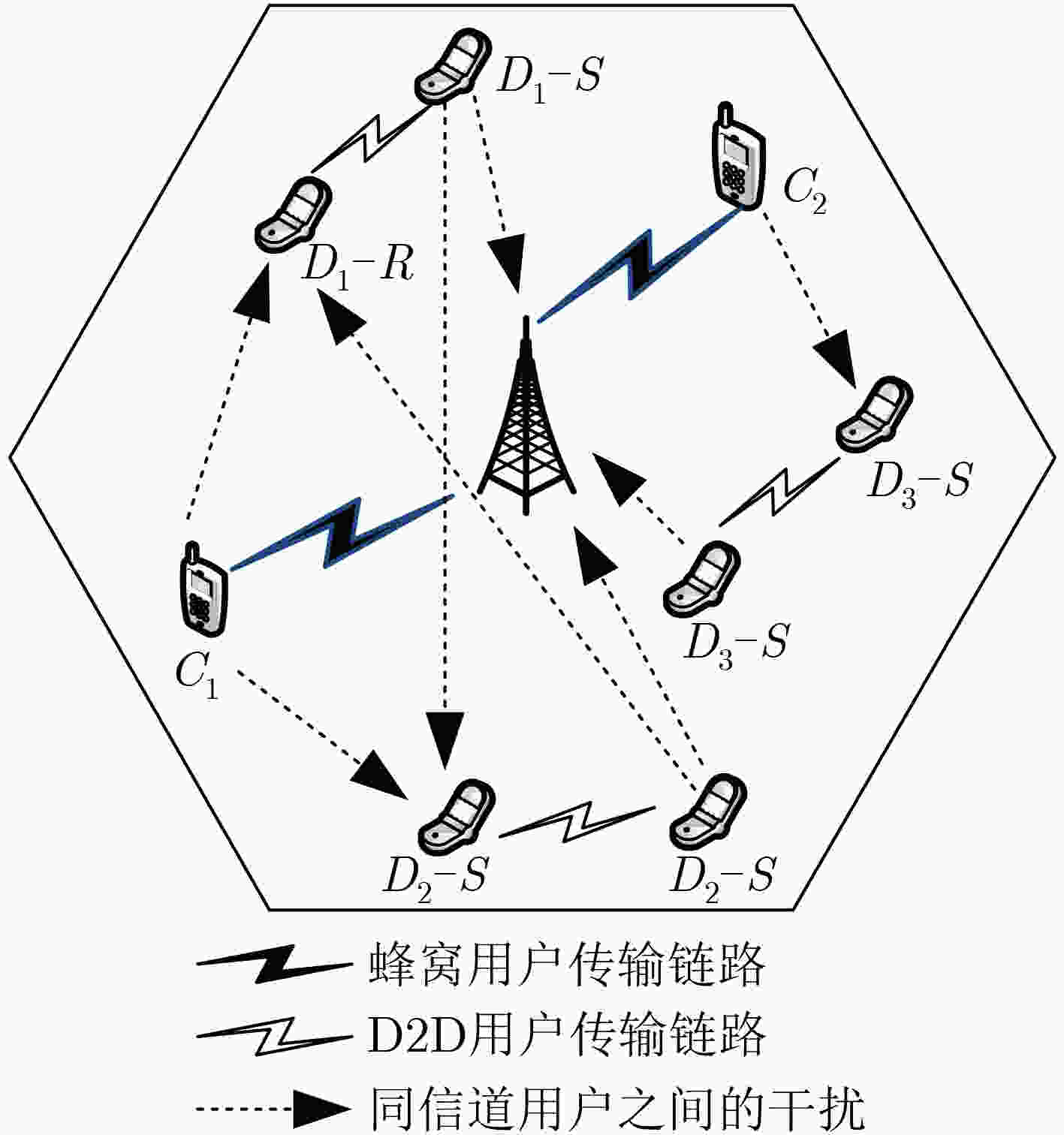
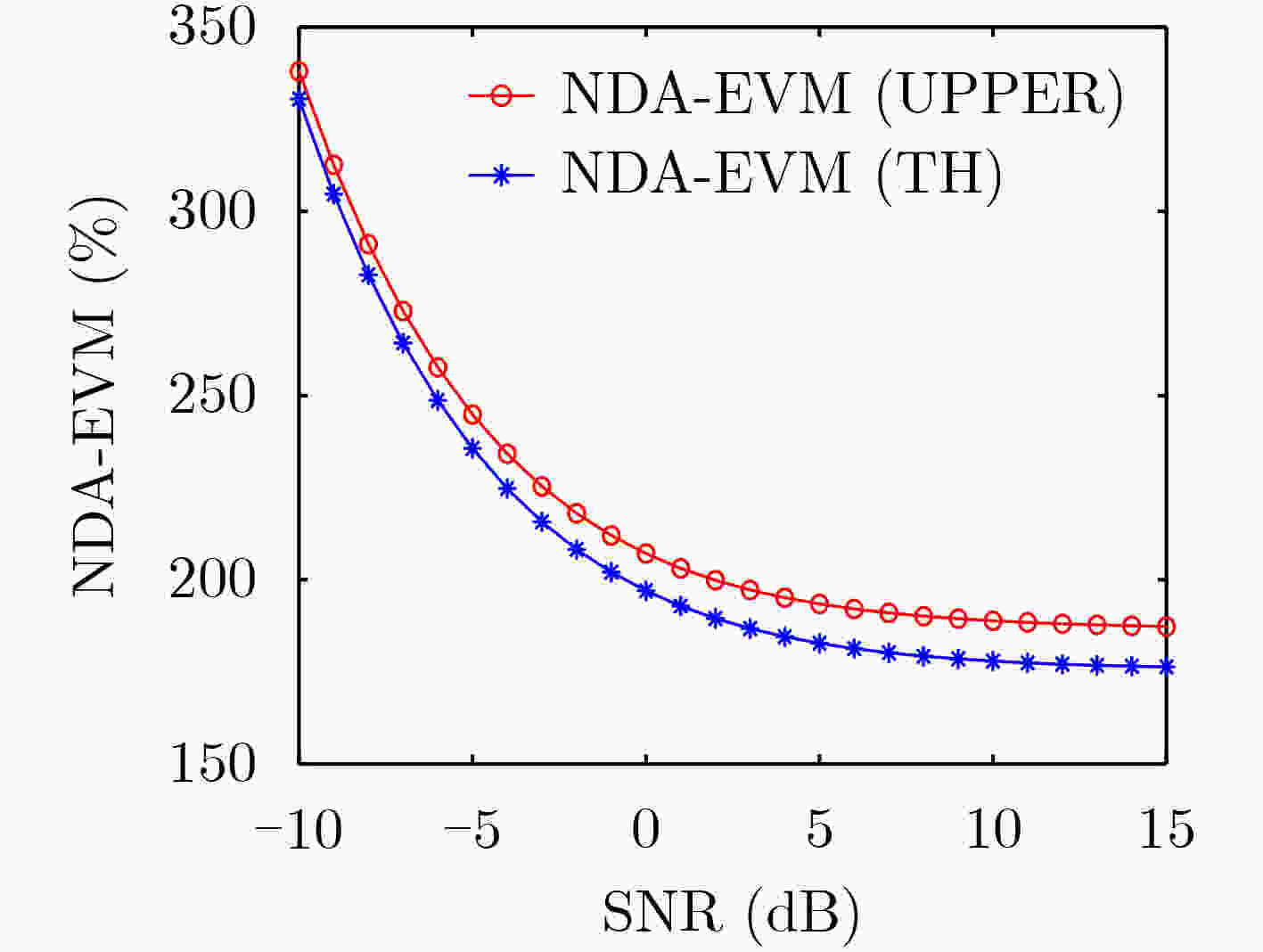
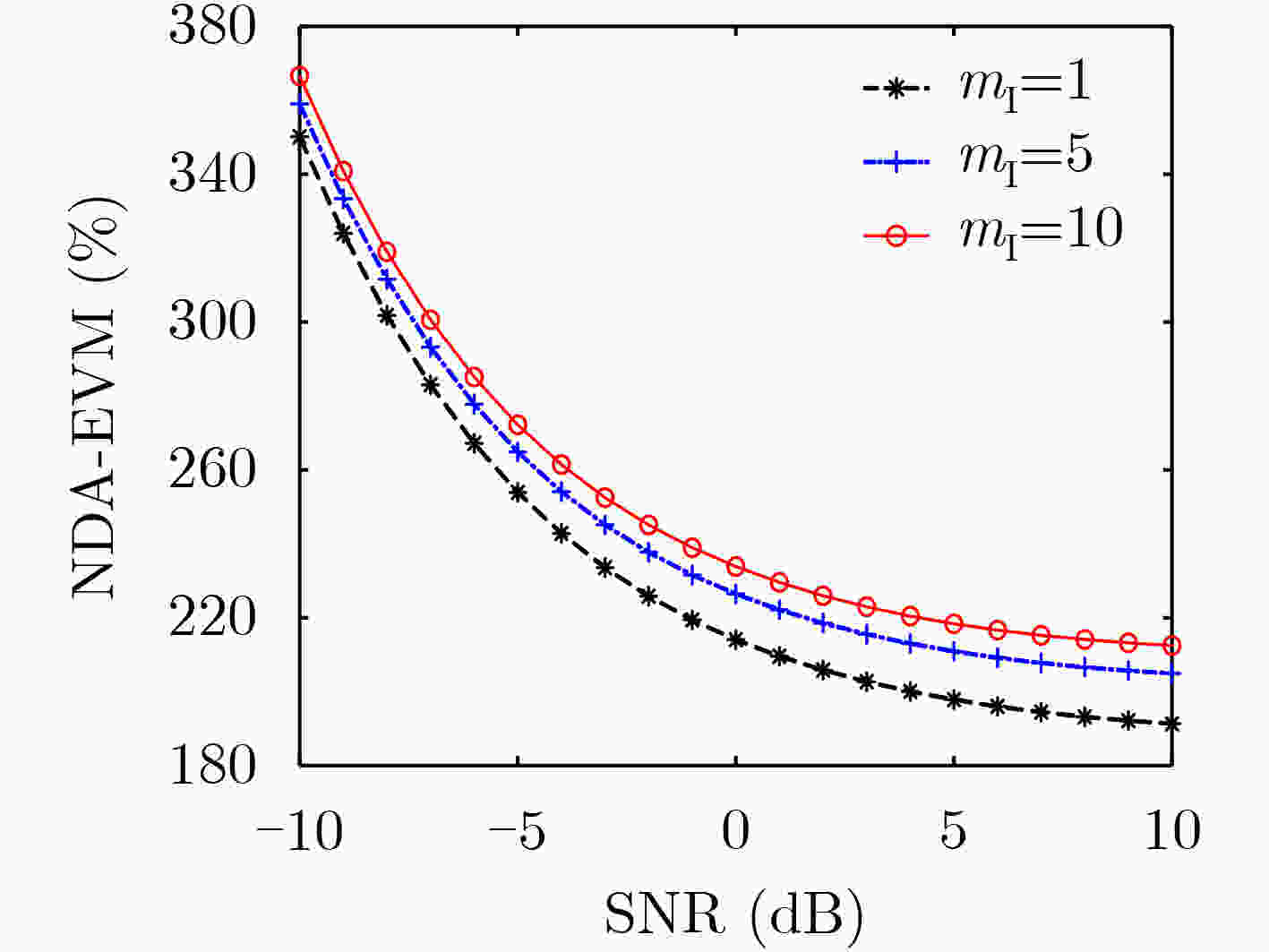
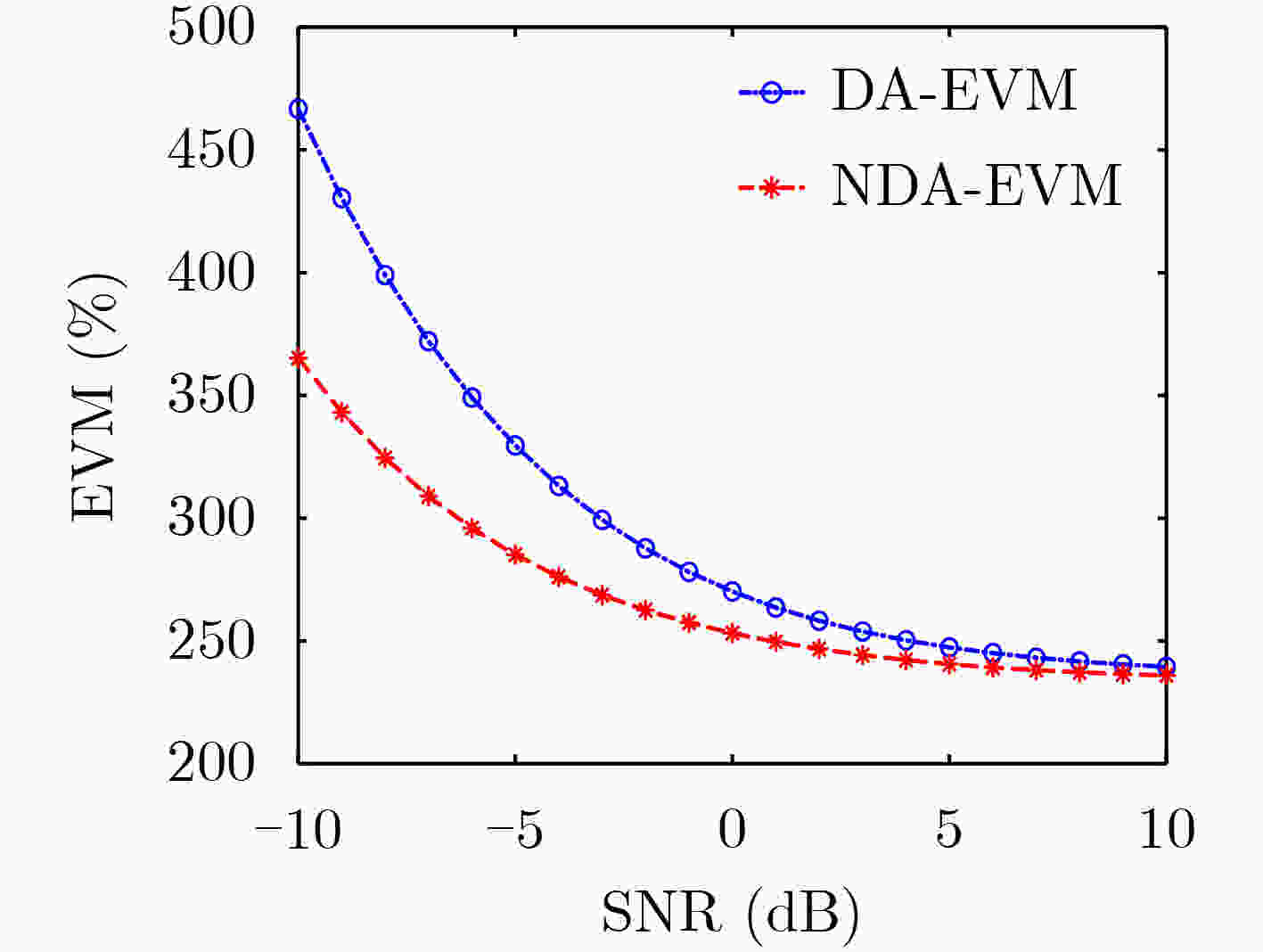
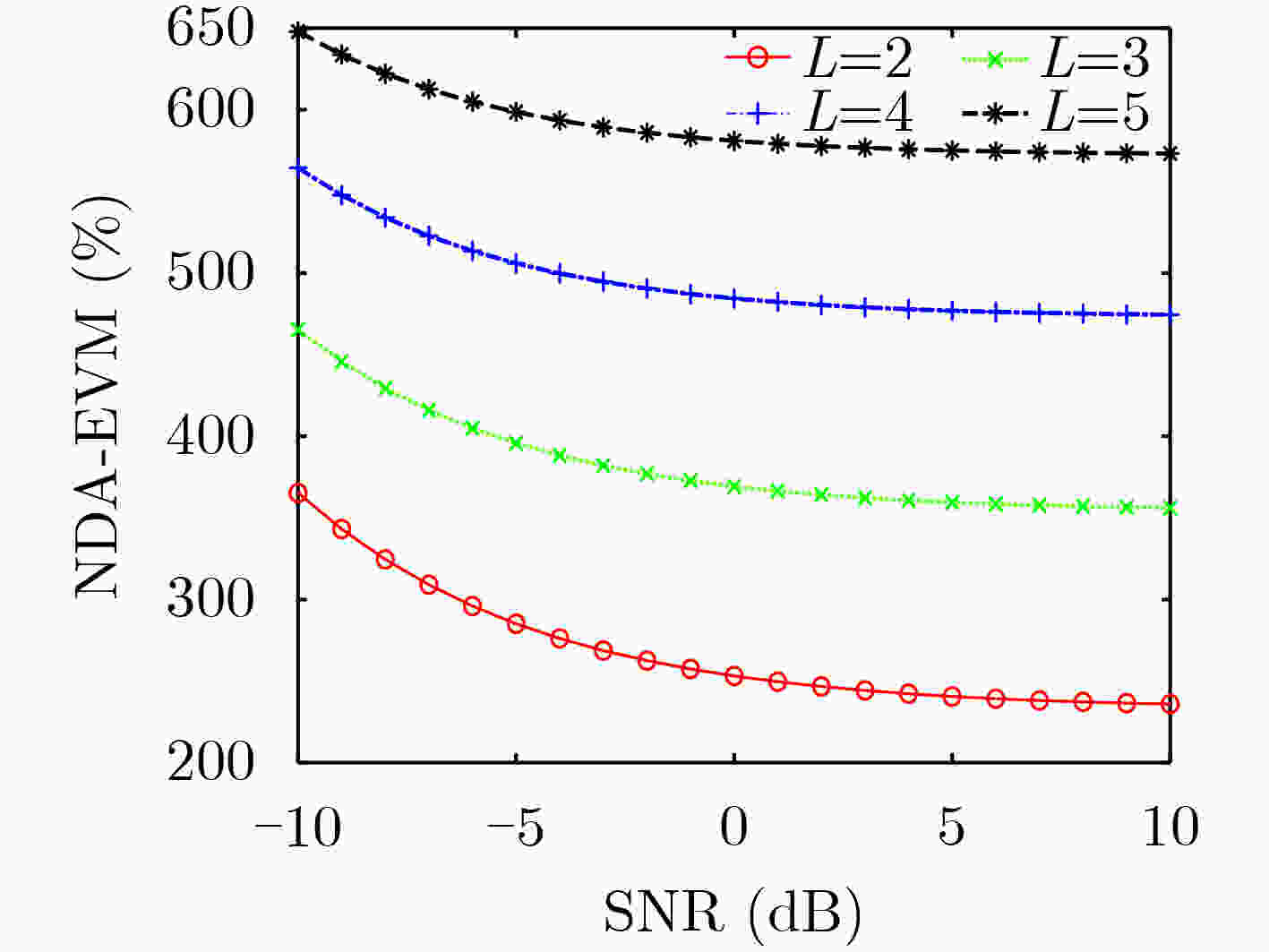
摘要: 针对D2D通信系统中广泛存在的同信道干扰问题，该文提出一种基于非数据辅助误差矢量幅度(NDA-EVM)进行同信道干扰分析的方法。以NDA-EVM作为信道质量评估参量，推导信号在M-QAM调制下的NDA-EVM统一计算模型，利用信道增益建立NDA-EVM同信道干扰分布模型，并进一步求解该模型的性能上限，从而量化同信道干扰。理论分析和仿真实验表明，相对于传统算法，该文所提上限的计算时间复杂度由$O({M^2})$降为$O({M})$，提高了信道评估时效性；推导性能上限与理论值吻合度高，特别是在低SNR时为紧上界，两者最小均方根误差RMSE低至0.2615。
- 同信道干扰 /
- D2D通信 /
- 非数据辅助 /
- 误差矢量幅度
Abstract: In view of the widely exist co-channel interference in D2D communication system, a novel method to quantify the co-channel interference based on NonDate Aided Error Vector Magnitude (NDA-EVM) is proposed. NDA-EVM is considered as a new metric to evaluate the change of the channels. The NDA-EVM of M-QAM modulated signal is analytically derived. Moreover, the model of NDA-EVM under co-channel interference is established. Specifically, the upper bound of NDA-EVM is calculated when co-channel interferences exist, so co-channel interferences can be quantified. Theoretical analysis and simulation experiments indicate that, when compared with traditional NDA-EVM algorithm, the proposed upper bound of NDA-EVM reduces the time complexity of algorithm from O(M²) to O(M), the effectiveness of channel estimation is improved. Besides, the derived upper bound closely matches with the theoretical value, especially at low SNR, the RMSE is as low as 0.2615.
- Co-channel interference /
- D2D communication /
- NonDate-Aided (NDA) /
- Error Vector Magnitude (EVM)

HTML全文

图 1 D2D通信系统模型

下载: 全尺寸图片幻灯片

图 2 $\alpha {\rm{ = 1}}$时NDA-EVM理论值与上限、下限值对比(16QAM)

下载: 全尺寸图片幻灯片

图 3 L=2时的NDA-EVM上限(4QAM)

下载: 全尺寸图片幻灯片

图 4 L=2时的NDA-EVM上限(256QAM)

下载: 全尺寸图片幻灯片

图 5 L=2时不同${m_{\rm{I}}}$的NDA-EVM上限

下载: 全尺寸图片幻灯片

图 6 L=2时NDA-EVM与DA-EVM对比

下载: 全尺寸图片幻灯片

图 7 不同干扰信道数量时的NDA-EVM上限

下载: 全尺寸图片幻灯片

表 1 算法时间复杂度

计算式	循环次数	时间复杂度
$\begin{aligned} \frac{2}{{k + 1}}&\bigg\{ \sum\limits_{j = 0}^k {\bigg[\sigma ( - b + {\lambda _{jk,{\rm{R}}}})\varphi \bigg(\frac{{ - b - {\lambda _{jk,{\rm{R}}}}}}{\sigma }\bigg) + ({\lambda _{jk,R}}^2 + {\sigma ^2})Q\bigg(\frac{{ - b - {\lambda _{jk,{\rm{R}}}}}}{\sigma }\bigg)} \bigg] \\ & + \sum\limits_{j = 0}^k {\bigg[\sigma (b + {\lambda _{jk,{\rm{R}}}})\varphi \bigg(\frac{{b - {\lambda _{jk,{\rm{R}}}}}}{\sigma }\bigg) + \bigg({\lambda _{jk,{\rm{R}}}}^2 + {\sigma ^2}\bigg)Q\bigg(\frac{{b - {\lambda _{jk,{\rm{R}}}}}}{\sigma }\bigg)} \bigg] \\ & + \sum\limits_{i = 1}^{k - 1} {\sum\limits_{j = 0}^k {\bigg[\sigma ( - b + {\lambda _{ji,{\rm{R}}}})\varphi \bigg(\frac{{ - b - {\lambda _{ji,{\rm{R}}}}}}{\sigma }\bigg) + ({\lambda _{ji,{\rm{R}}}}^2 + {\sigma ^2})Q\bigg(\frac{{ - b - {\lambda _{ji,{\rm{R}}}}}}{\sigma }\bigg)} \bigg]} {\rm{ }} \\ & - \sum\limits_{i = 1}^{k - 1} \sum\limits_{j = 0}^k \bigg[\sigma (b + {\lambda _{ji,{\rm{R}}}})\varphi \bigg(\frac{{b - {\lambda _{ji,{\rm{R}}}}}}{\sigma }\bigg) + ({\lambda _{ji,{\rm{R}}}}^2 + {\sigma ^2})Q\bigg(\frac{{b - {\lambda _{ji,{\rm{R}}}}}}{\sigma }\bigg) \bigg] \bigg\} \end{aligned} $	$2{M_m} + ({M_m} - 2) \times {M_m}$	$O(M_m^2)$
$\dfrac{{\rm{4}}}{{k + 1}}\left\{ C + 4kb\sigma \displaystyle\sum\limits_{j = 0}^{\dfrac{{k - 1}}{2}} {\left[\alpha {\beta _j} - \sqrt {\dfrac{1}{{2\pi }}} \exp \left(\sqrt {\dfrac{\pi }{2}} \alpha {\beta _j} - \dfrac{{{\alpha ^2}{\beta _j}^2}}{2}\right) \right]}\right\} $	$\dfrac{{{M_m}}}{2}$	$O({M_m})$

下载: 导出CSV

表 2 仿真参数

仿真参数	数值
载频${f_c}$(GHz)	2.2
发送功率${P_{\rm{0}}}$(W)	1
符号周期${T_{{\rm{sym}}}}$(μs)	66.7
循环前缀(CP)类型	${\rm{Normal}}$
${\rm{MQAM}}$星座类型	${\rm{QAM/16QAM/64QAM/256QAM}}$

下载: 导出CSV

参考文献(25)

[1]	LEE J and LEE J H. Performance analysis and resource allocation for cooperative D2D Communication in cellular networks with multiple D2D Pairs[J]. IEEE Communications Letters, 2019, 23(5): 909–912. doi: 10.1109/LCOMM.2019.2907252
[2]	TEHRANI M N, UYSAL M, and YANIKOMEROGLU H. Device-to-device communication in 5G cellular networks: Challenges, solutions, and future directions[J]. IEEE Communications Magazine, 2014, 52(5): 86–92. doi: 10.1109/MCOM.2014.6815897
[3]	钱志鸿, 王雪. 面向5G通信网的D2D技术综述[J]. 通信学报, 2016, 37(7): 1–14. doi: 10.11959/j.issn.1000-436x.2016129 QIAN Zhihong and WANG Xue. Reviews of D2D technology for 5G communication networks[J]. Journal on Communications, 2016, 37(7): 1–14. doi: 10.11959/j.issn.1000-436x.2016129
[4]	曾孝平, 毛海伟, 杨凡, 等. Nakagami-m衰落信道下D2D通信自适应调制算法研究[J]. 通信学报, 2018, 39(9): 31–42. doi: 10.11959/j.issn.1000-436x.2018151 ZENG Xiaoping, MAO Haiwei, YANG Fan, et al. Study on adaptive modulation in D2D communications over Nakagami-m fading channel[J]. Journal on Communications, 2018, 39(9): 31–42. doi: 10.11959/j.issn.1000-436x.2018151
[5]	钱志鸿, 王义君. 面向物联网的无线传感器网络综述[J]. 电子与信息学报, 2013, 35(1): 215–227. doi: 10.3724/SP.J.1146.2012.00876 QIAN Zhihong and WANG Yijun. Internet of Things-oriented wireless sensor networks review[J]. Journal of Electronics &Information Technology, 2013, 35(1): 215–227. doi: 10.3724/SP.J.1146.2012.00876
[6]	张平, 陶运铮, 张治. 5G若干关键技术评述[J]. 通信学报, 2016, 37(7): 15–29. doi: 10.11959/j.issn.1000-436x.2016130 ZHANG Ping, TAO Yunzheng, and ZHANG Zhi. Survey of several key technologies for 5G[J]. Journal on Communications, 2016, 37(7): 15–29. doi: 10.11959/j.issn.1000-436x.2016130
[7]	张达敏, 张绘娟, 闫威, 等. 异构网络中基于能效优化的D2D资源分配机制[J]. 电子与信息学报, 2020, 42(2): 480–487. doi: 10.11999/JEIT190042 ZHANG Damin, ZHANG Huijuan, YAN Wei, et al. D2D resource allocation mechanism based on energy efficiency optimization in heterogeneous networks[J]. Journal of Electronics &Information Technology, 2020, 42(2): 480–487. doi: 10.11999/JEIT190042
[8]	DUDAK C and KAHYAOGLU N D. A descriptive study on AM-AM and AM-PM conversion phenomena through EVM-SNR relation[C]. 2012 IEEE Topical Conference on Power Amplifiers for Wireless and Radio Applications, Santa Clara, USA, 2012: 69–72. doi: 10.1109/PAWR.2012.6174945.
[9]	THOMAS V A, KUMAR S, KALYANI S, et al. Error vector magnitude analysis of fading SIMO channels relying on MRC reception[J]. IEEE Transactions on Communications, 2016, 64(4): 1786–1797. doi: 10.1109/TCOMM.2016.2530797
[10]	YU Qinghua, QIU Bin, CONG Bo, et al. Research on real-time BER estimation in satellite downlink[C]. 2019 18th International Conference on Optical Communications and Networks (ICOCN), Huangshan, China, 2019: 1–3. doi: 10.1109/ICOCN.2019.8934721.
[11]	YANG Fan, MAO Haiwei, ZENG Xiaoping, et al. Nondata-aided error vector magnitude analysis of η-μ fading channels in device-to-device communications[J]. IEEE Access, 2019, 7: 82101–82109. doi: 10.1109/ACCESS.2019.2923584
[12]	TIWARI A, SAKHARDANDE K, and SHAH G. Non-data-aided SNR estimation for Nakagami-m fading channel[C]. 2018 IEEE International Students’ Conference on Electrical, Electronics and Computer Science (SCEECS), Bhopal, India, 2018: 1–4. doi: 10.1109/SCEECS.2018.8546853.
[13]	崔浩. 无线通信中的无缝自适应技术[D]. [博士论文], 中国科学技术大学, 2014. CUI Hao. Seamless adaptation for wireless communication[D]. [Ph. D. dissertation], University of Science and Technology of China, 2014.
[14]	张然然. 下一代无线通信系统的自适应传输技术研究[D]. [博士论文], 北京邮电大学, 2009. ZHANG Ranran. Research on adaptive transmission technologies for next generation wireless communication systems[D]. [Ph. D. dissertation], Beijing University of Posts and Telecommunications, 2009.
[15]	SHAFIK R A, RAHMAN M S, and ISLAM A R. On the extended relationships among EVM, BER and SNR as performance metrics[C]. 2006 International Conference on Electrical and Computer Engineering, Dhaka, Bangladesh, 2006: 408–411. doi: 10.1109/ICECE.2006.355657.
[16]	PARTHASARATHY S, KUMAR S, GANTI R K, et al. Error vector magnitude analysis in generalized fading with Co-channel Interference[J]. IEEE Transactions on Communications, 2018, 66(1): 345–354. doi: 10.1109/TCOMM.2017.2748127
[17]	SEN S, SANTHAPURI N, CHOUDHURY R R, et al. AccuRate: Constellation based rate estimation in wireless networks[C]. The 7th USENIX Conference on Networked Systems Design and Implementation, San Jose, USA, 2010: 28–30.
[18]	MAHMOUD H A and ARSLAN H. Error vector magnitude to SNR conversion for nondata-aided receivers[J]. IEEE Transactions on Wireless Communications, 2009, 8(5): 2694–2704. doi: 10.1109/TWC.2009.080862
[19]	杨凡, 曾孝平, 毛海伟, 等. κ-μ阴影衰落信道下非数据辅助的误差矢量幅度性能分析[J]. 通信学报, 2018, 39(5): 177–188. doi: 10.11959/j.issn.1000-436x.2018088 YANG Fan, ZENG Xiaoping, MAO Haiwei, et al. Nondata-aided error vector magnitude performance analysis over κ-μ shadowed fading channel[J]. Journal on Communications, 2018, 39(5): 177–188. doi: 10.11959/j.issn.1000-436x.2018088
[20]	LAI Weikuang, WANG Y C, LIN H C, et al. Efficient resource allocation and power control for LTE-A D2D communication with pure D2D model[J]. IEEE Transactions on Vehicular Technology, 2020, 69(3): 3202–3216. doi: 10.1109/TVT.2020.2964286
[21]	JANG W M. A simple upper bound of the Gaussian Q-Function with closed-form error bound[J]. IEEE Communications Letters, 2011, 15(2): 157–159. doi: 10.1109/LCOMM.2011.011011.102207
[22]	SHAHINI A, BAGHERI A, and SHAHZADI A. A unified approach to performance analysis of energy detection with diversity receivers over Nakagami-m fading channels[C]. 2013 International Conference on Connected Vehicles and Expo (ICCVE), Las Vegas, USA, 2013: 707–712. doi: 10.1109/ICCVE.2013.6799881.
[23]	3GPP. Small cell enhancements for E-UTRA and E-UTRAN-physical layer aspects[R]. Technical Report: 36.872, 2013.
[24]	RAHMAN M A and HARADA H. New exact closed-form PDF of the sum of Nakagami-m random variables with applications[J]. IEEE Transactions on Communications, 2011, 59(2): 395–401. doi: 10.1109/TCOMM.2010.112310.090212
[25]	KRSTIC D, GŁABOWSKI M, STEFANOVIC M, et al. Level crossing rate of ratio of product of two Rayleigh and one Nakagami-m random variable and of ratio of Rayleigh and product of two Nakagami-m random variables[C]. 2018 11th International Symposium on Communication Systems, Networks & Digital Signal Processing (CSNDSP), Budapest, Hungary, 2018: 1–6. doi: 10.1109/CSNDSP.2018.8471843.