Security Analysis of Block Cipher CFE
-
摘要: 该文对基于混沌的双模块Feistel结构(CFE)高安全性高速分组算法的安全性进行了分析。分析结果表明,算法不适合用积分攻击、中间相遇攻击、不变量攻击、插值攻击和循环移位攻击分析其安全性;可以抵抗相关密钥攻击;更进一步地构造出了5轮不可能差分特征链,并利用其进行区分攻击;求得算法的活性S盒下界为6,概率约为2–21;算法存在5轮零相关线性特征。Abstract: The security of high security and high speed block cipher algorithm of two-module FEistel structure based on Chaos (CFE) is analyzed. The results show that the cipher is not suitable to use integral attack, meat-in-the-middle attack, invariant attack, interpolation attack and circle shift attack to analyze its security. And it can resist the related-key attack. Furthermore, 5 rounds of impossible differential characteristic are constructed and used to distinguish attacks. The lower bound of the active S-box is 6, and the probability is about 2–21. There are 5 rounds of linear characteristic with zero-correlation.
-
表 1 差分活性S盒个数下界
轮数 1 2 3 4 5 128/256 bit 0 2 3 5 6 -
[1] BIHAM E and SHAMIR A. Differential cryptanalysis of DES-like cryptosystems[J]. Journal of Cryptology, 1991, 4(1): 3–72. doi: 10.1007/BF00630563 [2] 杨伟伟, 刘光杰, 戴跃伟. 基于交叉耦合映像格子时空混沌的S盒设计[J]. 应用科学学报, 2015, 33(4): 438–448. doi: 10.3969/j.issn.0255-8297.2015.04.010YANG Weiwei, LIU Guangjie, and DAI Yuewei. Design of S-boxes based on spatiotemporal chaotic systems of cross coupled map lattices[J]. Journal of Applied Sciences—Electronics and Information Engineering, 2015, 33(4): 438–448. doi: 10.3969/j.issn.0255-8297.2015.04.010 [3] 贾平, 徐洪, 戚文峰. 轻量S盒密码性质研究[J]. 密码学报, 2015, 2(6): 497–504. doi: 10.13868/j.cnki.jcr.000096JIA Ping, XU Hong, and QI Wenfeng. Research on cryptographic properties of lightweight S-boxes[J]. Journal of Cryptologic Research, 2015, 2(6): 497–504. doi: 10.13868/j.cnki.jcr.000096 [4] 杨萍. 基于MILP方法的轻量级分组密码的安全性分析[D]. [硕士论文], 山东师范大学, 2018.YANG Ping. Security analysis of lightweight block cipher based on MILP method[D]. [Master dissertation], Shandong Normal University, 2018. [5] 吴文玲, 张蕾. 不可能差分密码分析研究进展[J]. 系统科学与数学, 2008, 28(8): 971–983.WU Wenling and ZHANG Lei. The state-of-the-art of research on impossible differential cryptanalysis[J]. Journal of Systems Science and Mathematical Sciences, 2008, 28(8): 971–983. [6] 韦永壮, 史佳利, 李灵琛. LiCi分组密码算法的不可能差分分析[J]. 电子与信息学报, 2019, 41(7): 1610–1617. doi: 10.11999/JEIT180729WEI Yongzhuang, SHI Jiali, and LI Lingchen. Impossible differential cryptanalysis of LiCi block cipher[J]. Journal of Electronics &Information Technology, 2019, 41(7): 1610–1617. doi: 10.11999/JEIT180729 [7] 张仕伟, 陈少真. SIMON不可能差分及零相关路径自动化搜索算法[J]. 软件学报, 2018, 29(11): 3544–3553. doi: 10.13328/j.cnki.jos.005296ZHANG Shiwei and CHEN Shaozhen. Automatic search algorithm for impossible differential trials and zero-correlation linear trials in SIMON[J]. Journal of Software, 2018, 29(11): 3544–3553. doi: 10.13328/j.cnki.jos.005296 [8] 马楚焱, 刘国强, 李超. 对PICO和RECTANGLE的零相关线性分析[J]. 密码学报, 2017, 4(5): 413–422. doi: 10.13868/j.cnki.jcr.000193MA Chuyan, LIU Guoqiang, and LI Chao. Zero-correlation linear cryptanalysis on PICO and RECTANGLE[J]. Journal of Cryptologic Research, 2017, 4(5): 413–422. doi: 10.13868/j.cnki.jcr.000193 [9] DUO Lei, LI Chao, and FENG Keqin. Square like attack on camellia[C]. The International Conference on Information and Communications Security, Zhengzhou, China, 2007: 269–283. doi: 10.1007/978-3-540-77048-0_21. [10] 任炯炯, 李航, 陈少真. 减轮Simeck算法的积分攻击[J]. 电子与信息学报, 2019, 41(9): 2156–2163. doi: 10.11999/JEIT180849REN Jiongjiong, LI Hang, and CHEN Shaozhen. Integral attack on reduced-round simeck algorithm[J]. Journal of Electronics &Information Technology, 2019, 41(9): 2156–2163. doi: 10.11999/JEIT180849 [11] 邓元豪, 金晨辉, 赵杰卿. Type-3型广义Feistel结构的中间相遇攻击[J]. 密码学报, 2019, 6(1): 27–36. doi: 10.13868/j.cnki.jcr.000280DENG Yuanhao, JIN Chenhui, and ZHAO Jieqing. Meet-in-the-middle attack on Type-3 Feistel structure[J]. Journal of Cryptologic Research, 2019, 6(1): 27–36. doi: 10.13868/j.cnki.jcr.000280 [12] 汪艳凤, 吴文玲. 分组密码TWINE的中间相遇攻击[J]. 软件学报, 2015, 26(10): 2684–2695. doi: 10.13328/j.cnki.jos.004805WANG Yanfeng and WU Wenling. Meet-in-the-Middle attack on TWINE block cipher[J]. Journal of Software, 2015, 26(10): 2684–2695. doi: 10.13328/j.cnki.jos.004805 [13] JAKOBSEN T and KNUDSEN L R. The interpolation attack on block ciphers[C]. The International Workshop on Fast Software Encryption, Haifa, Israel, 1997: 28–40. doi: 10.1007/BFb0052332. [14] 金晨辉, 杨阳, 祁传达. 对混沌序列密码的相关密钥攻击[J]. 电子与信息学报, 2006, 28(3): 410–414.JIN Chenhui, YANG Yang, and QI Chuanda. A related-key attack on chaotic stream ciphers[J]. Journal of Electronics &Information Technology, 2006, 28(3): 410–414. [15] TODO Y, LEANDER G, and SASAKI Y. Nonlinear invariant attack: Practical attack on full SCREAM, iSCREAM, and Midori64[J]. Journal of Cryptology, 2019, 32(4): 1383–1422. doi: 10.1007/s00145-018-9285-0 [16] XIE E Y, LI Chengqing YU Simin, et al. On the cryptanalysis of Fridrich’s chaotic image encryption scheme[J]. Signal Processing, 2017, 132: 150–154. doi: 10.1016/j.sigpro.2016.10.002 -





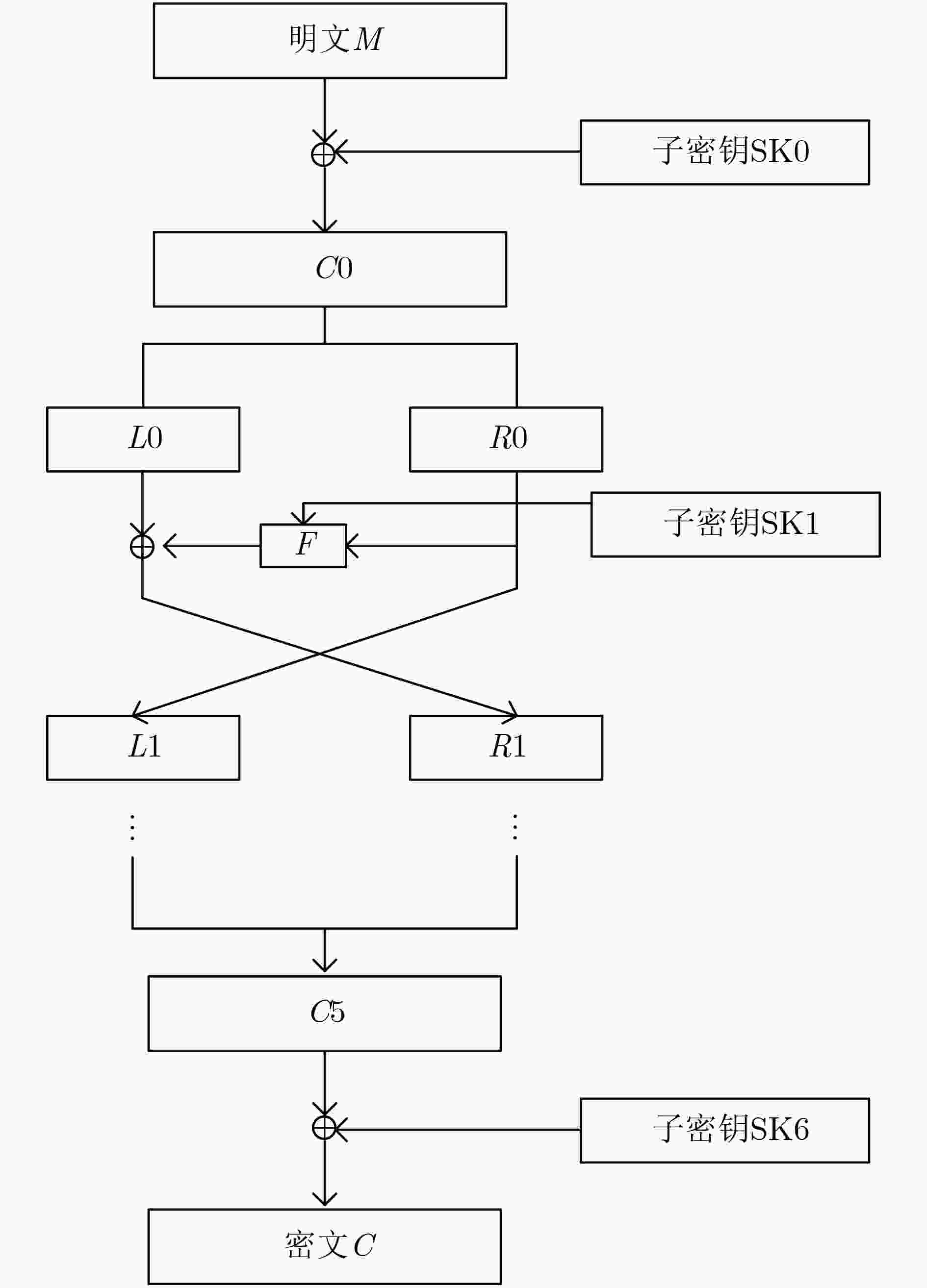
 下载:
下载:
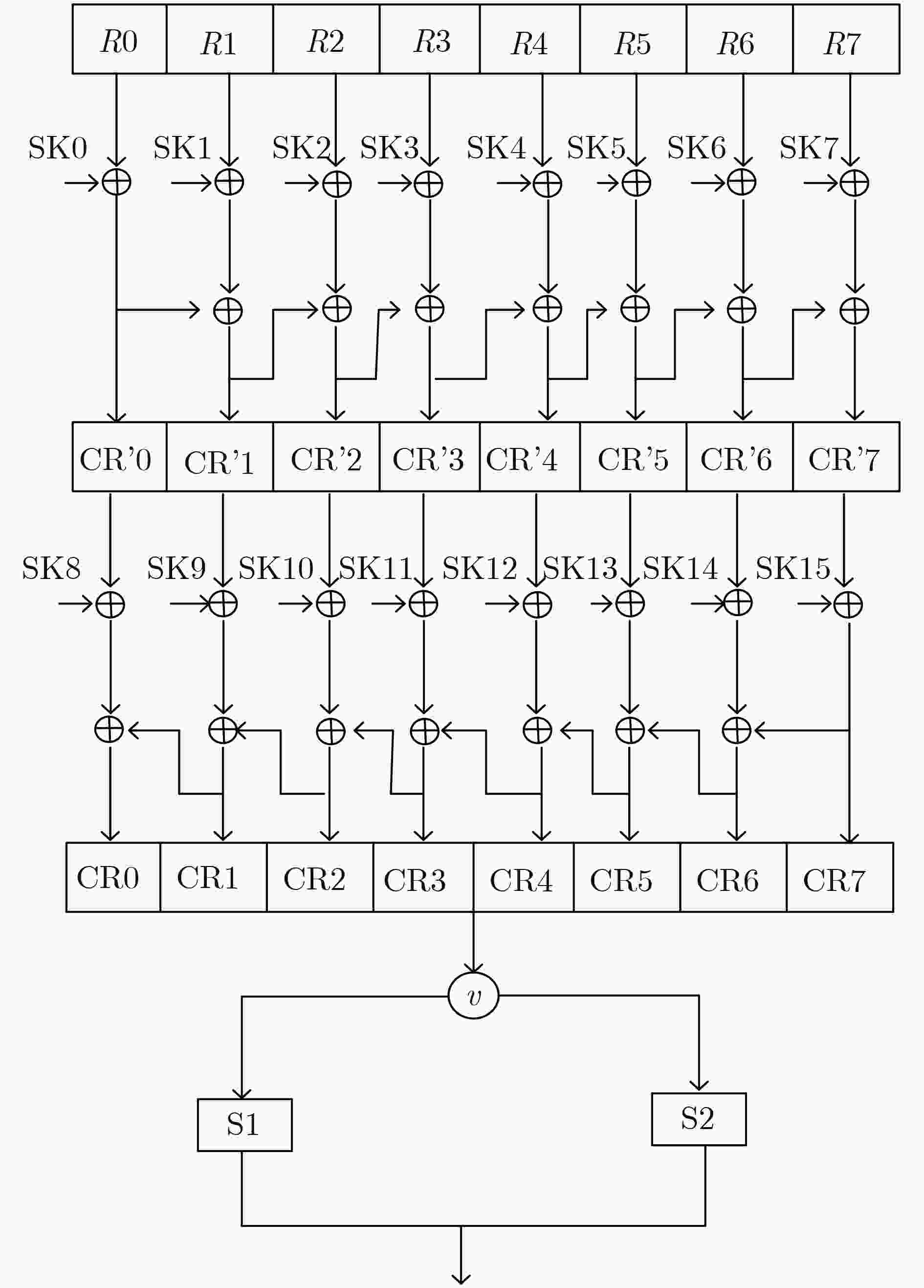



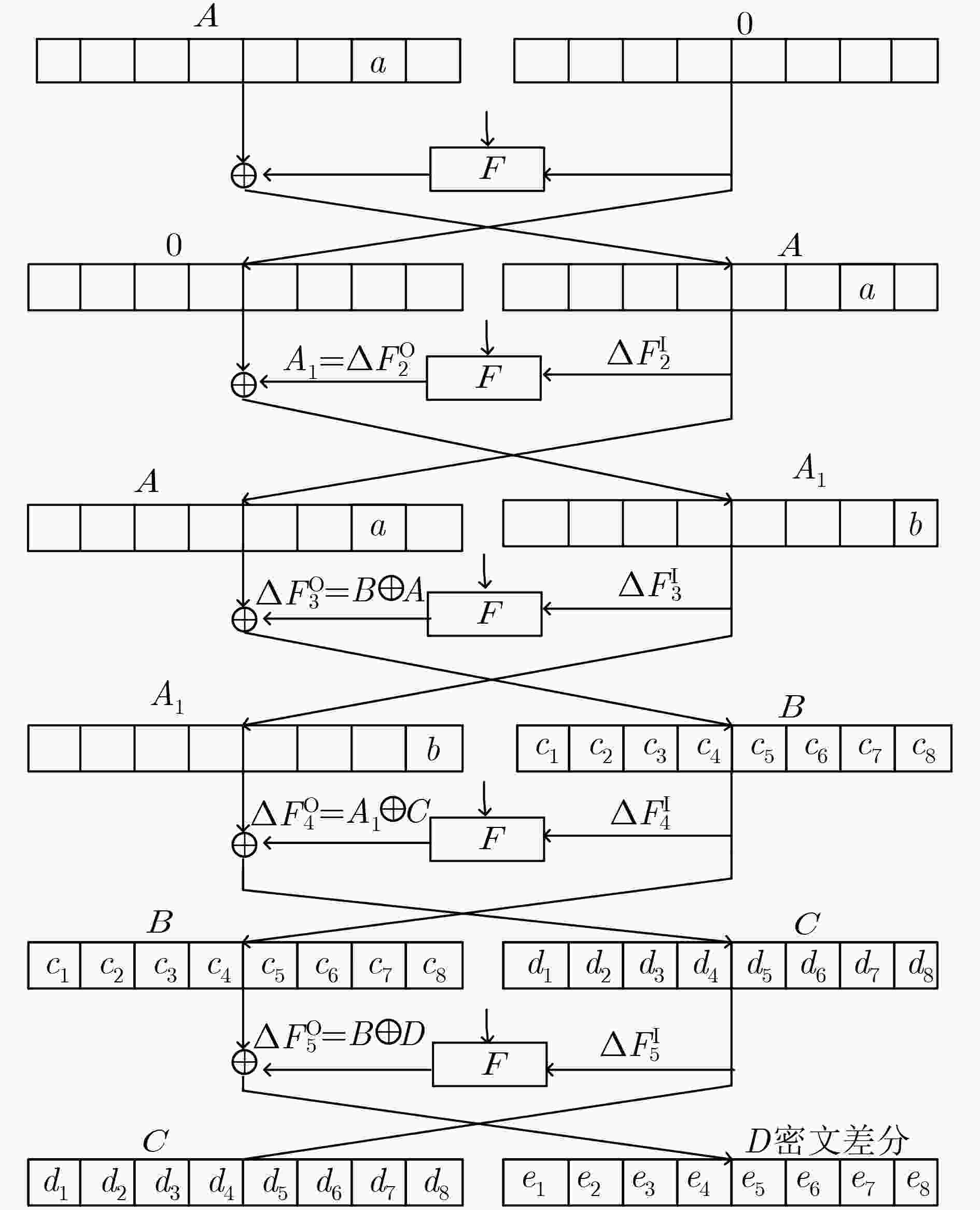


 下载:
下载:
