一种类箕舌线函数的变步长归一化自适应滤波算法

火元莲; 龙小强; 连培君; 王丹凤

doi:10.11999/JEIT191003

一种类箕舌线函数的变步长归一化自适应滤波算法

doi: 10.11999/JEIT191003

西北师范大学物理与电子工程学院兰州 730070

基金项目: 国家自然科学基金(61561044)

详细信息

作者简介:
火元莲：女，1973年生，博士，副教授，研究方向为信号与信息处理、数字图像处理

龙小强：男，1995年生，硕士生，研究方向为自适应滤波技术

连培君：女，1995年生，硕士生，研究方向为自适应滤波技术

王丹凤：女，1996年生，硕士生，研究方向为自适应滤波技术

通讯作者:
龙小强　lxqchj@163.com

中图分类号: TN713
计量
- 文章访问数: 1273
- HTML全文浏览量: 553
- PDF下载量: 111
- 被引次数: 0
出版历程
- 收稿日期: 2019-12-16
- 修回日期: 2020-10-24
- 网络出版日期: 2020-12-07
- 刊出日期: 2021-02-23

A Kind of Versoria Function Normalized Adaptive Filtering Algorithm

College of Physics and Electronic Engineering, Northwest Normal University, Lanzhou 730070, China

Funds: The National Natural Science Foundation of China (61561044)

摘要

摘要:
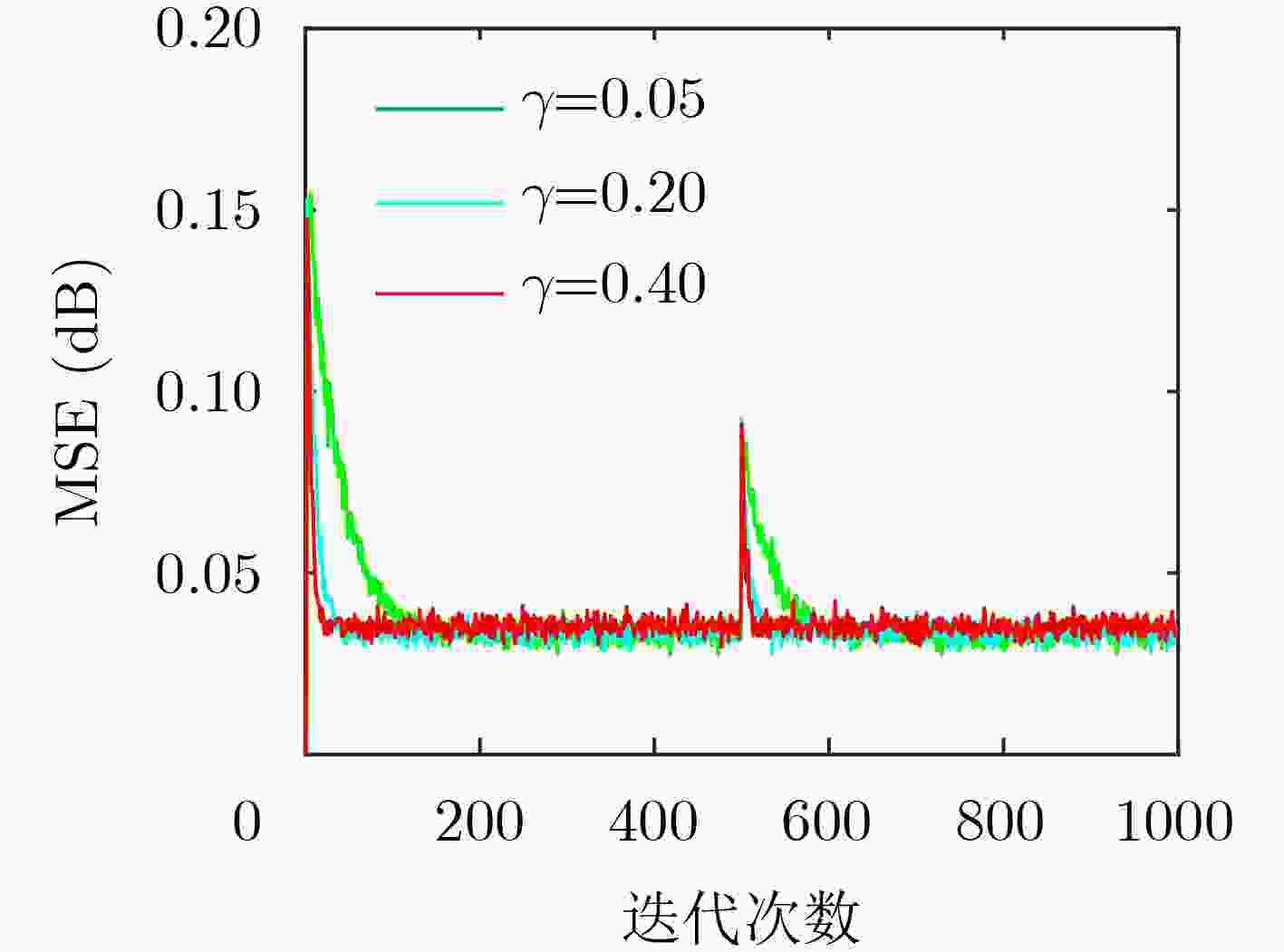
在综合考虑自适应滤波算法设计中收敛速度、稳态误差、计算复杂度和跟踪性能等指标的基础上，该文提出一种类箕舌线函数的变步长归一化自适应滤波算法，用类箕舌线函数代替Sigmoid函数作为步长迭代公式，引入基于相关误差的变步长调整原则，在大大增强算法稳定性的同时大幅度提升了算法的收敛速度、跟踪性能，减小了算法的计算复杂度。在Matlab平台上分析了改进的步长函数中参数
\begin{document}$\alpha $\end{document}
,
以及
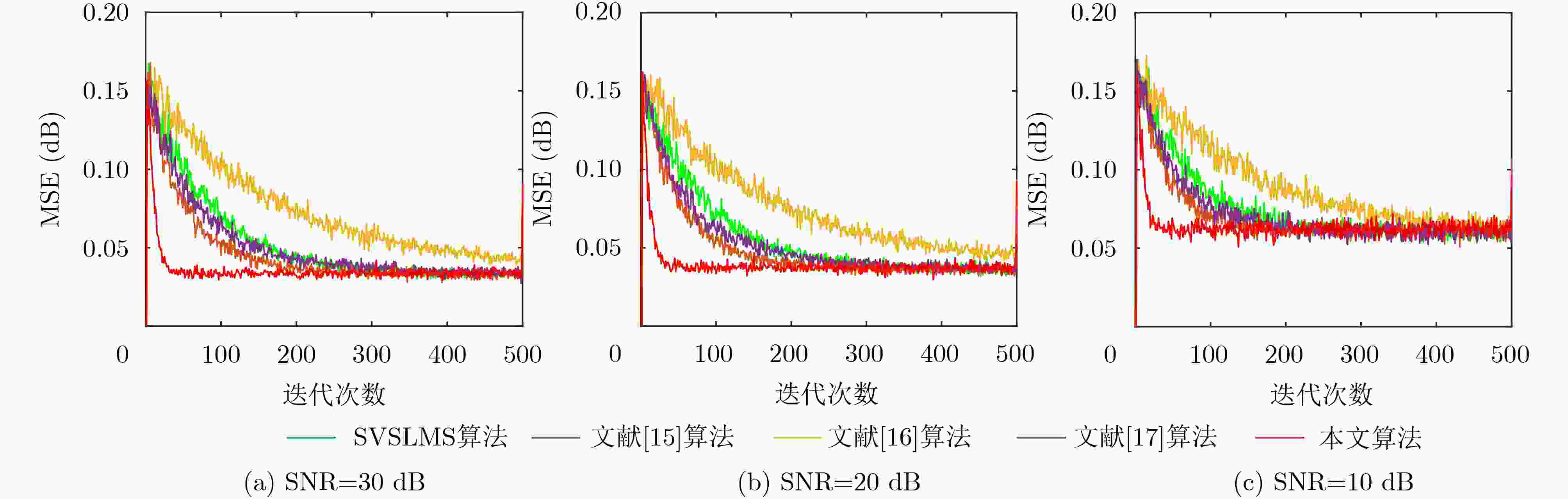
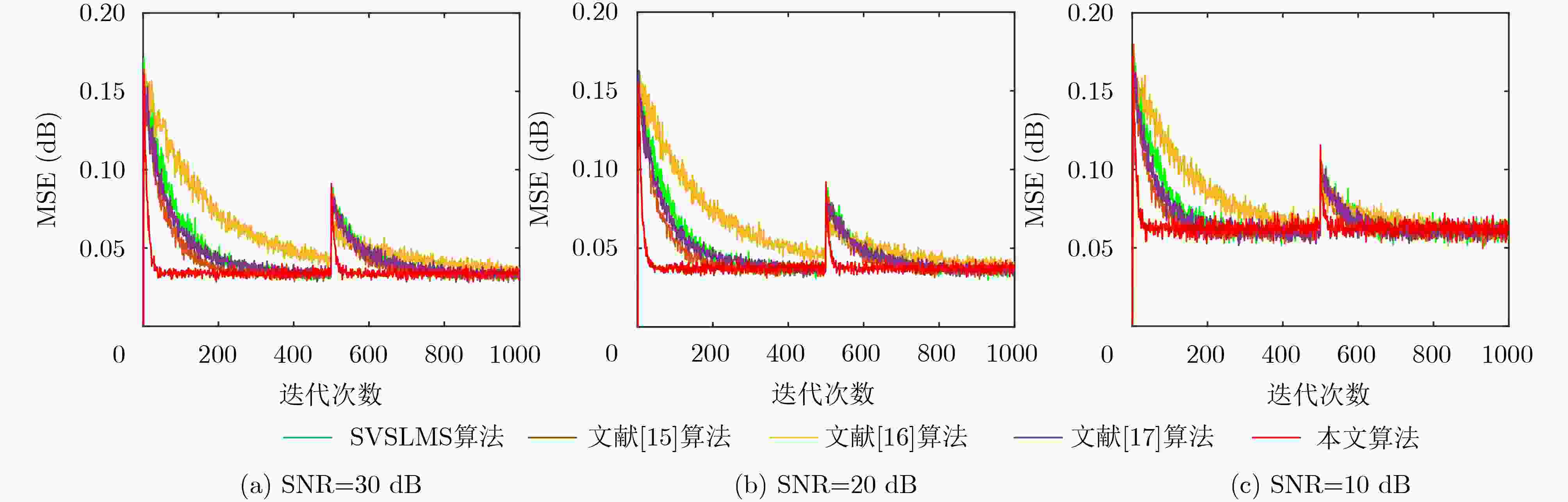
的不同取值对算法的影响，并将该文算法与已有的基于Sigmoid函数和基于箕舌线函数的变步长LMS算法进行了比较，仿真结果表明，该文算法有更快的收敛速度、更好的跟踪能力以及较小的稳态误差和较强的鲁棒性。
- 自适应滤波 /
- 变步长自适应算法 /
- 最小均方算法 /
- 箕舌线函数
Abstract:
On the basis of the comprehensive consideration in the design of the indexs of the adaptive filter algorithm convergence speed, steady-state error, computational complexity and tracking performance, a kind of Versoria function normalized adaptive filtering algorithm is proposed in this paper. The class Versoria function is used instead of Sigmoid function as step iterative formula, introducing variable step size based on the relevant error adjustment principle, the stability of the algorithm is enhanced greatly. At the same time, the convergence speed and tracking performance of the algorithm is promoted and the computational complexity of the algorithm is reduced. The influence of the parameter
\begin{document}$\alpha $\end{document}
,
and
different value of the step function of algorithm is analyzed on Matlab platform. Compared with the Sigmoid function variable step size LMS algorithm and variable step size LMS algorithm based on Versoria function, and the simulation results show that this algorithm has faster convergence speed, better tracking ability, smaller steady-state error and strong robustness.
- Adaptive filtering /
- Variable step size adaptive algorithm /
- LMS algorithm /
- Versoria function

HTML全文

图 1 类箕舌线函数曲线调整过程曲线图

下载: 全尺寸图片幻灯片

图 2 步长因子$\mu \left( n \right)$与误差${{e}}\left( n \right)$调整曲线图

下载: 全尺寸图片幻灯片

图 3 $\gamma $不同时算法收敛速度图

下载: 全尺寸图片幻灯片

图 4 5种算法步长调整曲线图

下载: 全尺寸图片幻灯片

图 5 不同信噪比背景下5种算法MSE对比图

下载: 全尺寸图片幻灯片

图 6 不同信噪比背景下5种算法面对时变系统时的跟踪性能对比图

下载: 全尺寸图片幻灯片

表 1 不同信噪比下算法收敛次数比较

信噪比(dB) SVSLMS算法文献[15]算法文献[16]算法文献[17]算法本文算法

10 250 200 450 210 25
20 300 250 900 320 30
30 400 270 1000 400 35

下载: 导出CSV

参考文献(22)

孙慧贤, 刘建成, 崔佩璋, 等. 基于多通道最小均方算法的多发单收同车电台射频干扰对消[J]. 电子与信息学报, 2019, 41(3): 556–562. doi: 10.11999/JEIT180356

SUN Huixian, LIU Jiancheng, CUI Peizhang, et al. RF interference cancellation based on multi-channel least mean square for multi-transmits and single-receive co-vehicle radios[J]. Journal of Electronics &Information Technology, 2019, 41(3): 556–562. doi: 10.11999/JEIT180356

赵杨, 尚朝轩, 韩壮志, 等. 分数阶傅里叶和压缩感知自适应抗频谱弥散干扰[J]. 电子与信息学报, 2019, 41(5): 1047–1054. doi: 10.11999/JEIT180569

ZHAO Yang, SHANG Chaoxuan, HAN Zhuangzhi, et al. Fractional Fourier transform and compressed sensing adaptive countering smeared spectrum jamming[J]. Journal of Electronics &Information Technology, 2019, 41(5): 1047–1054. doi: 10.11999/JEIT180569

唐敏, 齐栋, 刘成城, 等. 基于多级阻塞的稳健相干自适应波束形成[J]. 电子与信息学报, 2019, 41(7): 1705–1711. doi: 10.11999/JEIT180332

TANG Min, QI Dong, LIU Chengcheng, et al. New adaptive beamformer for coherent interference based on multistage blockin[J]. Journal of Electronics &Information Technology, 2019, 41(7): 1705–1711. doi: 10.11999/JEIT180332

王世元, 史春芬, 蒋云翔, 等. 基于q梯度的仿射投影算法及其稳态均方收敛分析[J]. 电子与信息学报, 2018, 40(10): 2402–2407. doi: 10.11999/JEIT171125

WANG Shiyuan, SHI Chunfen, JIANG Yunxiang, et al. q-affine projection algorithm and its steady-state mean square convergence analysis[J]. Journal of Electronics &Information Technology, 2018, 40(10): 2402–2407. doi: 10.11999/JEIT171125

毕晓君, 王朝. 一种基于角度惩罚距离的高维多目标进化算法[J]. 电子与信息学报, 2018, 40(2): 314–322. doi: 10.11999/JEIT170454

BI Xiaojun and WANG Chao. A many-objective evolutionary algorithm based on angle penalized distance[J]. Journal of Electronics &Information Technology, 2018, 40(2): 314–322. doi: 10.11999/JEIT170454

ZHENG Weixing. Adaptive filter design subject to output envelope constraints and bounded input noise[J]. IEEE Transactions on circuits and systems II: Analog and Digital Signal Processing, 2003, 50(12): 1023–1027. doi: 10.1109/tcsii.2003.820237

FENG Dazheng and ZHENG Weixing. Fast RLS-type algorithm for unbiased equation-error adaptive IIR filtering based on approximate inverse-power iteration[J]. IEEE Transactions on Signal Processing, 2005, 53(11): 4169–4185. doi: 10.1109/tsp.2005.857036

FENG D Z and ZHENG W X. Fast approximate inverse power iteration algorithm for adaptive total least-squares FIR filtering[J]. IEEE Transactions on Signal Processing, 2006, 54(10): 4032–4039. doi: 10.1109/tsp.2006.880245

SONG Xinmin and PARK J H. Linear minimum mean-square estimation for discrete-time measurement-delay systems with multiplicative noise and Markov jump[J]. IET Control Theory & Applications, 2016, 10(10): 1161–1169. doi: 10.1049/iet-cta.2015.1197

于霞, 刘建昌, 李鸿儒. 基于箕舌线函数的快速凸组合最小均方算法[J]. 系统仿真学报, 2010, 22(5): 1097–1100, 1105.

YU Xia, LIU Jianchang, and LI Hongru. Fast convex combination of least-mean-square algorithm based on versoria function[J]. Journal of System Simulation, 2010, 22(5): 1097–1100, 1105.

ABBAS H and HAI Huang. Weight size determined variable step size LMS method for identifying under damped systems[J]. Applied Mechanics and Materials, 2014, 511–512: 238–241. doi: 10.4028/www.scientific.net/AMM.511-512.238

高鹰, 谢胜利. 一种变步长LMS自适应滤波算法及分析[J]. 电子学报, 2001, 29(8): 1094–1097. doi: 10.3321/j.issn:0372-2112.2001.08.023

GAO Ying and XIE Shengli. A variable step size LMS adaptive filtering algorithm and its analysis[J]. Acta Electronica Sinica, 2001, 29(8): 1094–1097. doi: 10.3321/j.issn:0372-2112.2001.08.023

仝喜峰, 陈卫松, 钱隆彦, 等. 一种非线性变步长LMS自适应滤波算法[J]. 无线电通信技术, 2019, 45(4): 391–396. doi: 10.3969/j.issn.1003-3114.2019.04.011

TONG Xifeng, CHEN Weisong, QIAN Longyan, et al. A nonlinear variable step size LMS adaptive filtering algorithm[J]. Radio Communications Technology, 2019, 45(4): 391–396. doi: 10.3969/j.issn.1003-3114.2019.04.011

覃景繁, 欧阳景正. 一种新的变步长LMS自适应滤波算法[J]. 数据采集与处理, 1997, 12(3): 171–174.

QIN Jingfan and OUYANG Jingzheng. A novel variable step size LMS adaptive filtering algorithm based on sigmoid function[J]. Journal of Data Acquisition &Processing, 1997, 12(3): 171–174.

刘宪爽, 吴华明, 肖文波, 等. 改进的双Sigmoid函数变步长自适应算法及在OCT中的应用[J]. 电子学报, 2019, 47(1): 234–240. doi: 10.3969/j.issn.0372-2112.2019.01.031

LIU Xianshuang, WU Huaming, XIAO Wenbo, et al. Improved double sigmoid function variable step size adaptive algorithm and its application in OCT[J]. Acta Electronica Sinica, 2019, 47(1): 234–240. doi: 10.3969/j.issn.0372-2112.2019.01.031

邓江波, 侯新国, 吴正国. 基于箕舌线的变步长LMS自适应算法[J]. 数据采集与处理, 2004, 19(3): 282–285. doi: 10.3969/j.issn.1004-9037.2004.03.010

DENG Jiangbo, HOU Xinguo, and WU Zhengguo. Variable step adaptive filtering LMS algorithm based on tongue-like curve[J]. Journal of Data Acquisition &Processing, 2004, 19(3): 282–285. doi: 10.3969/j.issn.1004-9037.2004.03.010

韩允, 解传军, 刘宝华, 等. 基于箕舌线函数的变步长归一化最小均方算法[J]. 现代电子技术, 2008, 31(19): 29–32. doi: 10.3969/j.issn.1004-373X.2008.19.010

HAN Yun, XIE Chuanjun, LIU Baohua, et al. Modified variable step-size normalized least-mean-square algorithm based on versoria function[J]. Modern Electronics Technique, 2008, 31(19): 29–32. doi: 10.3969/j.issn.1004-373X.2008.19.010

陈泳, 田金鹏, 刘燕平. 一种新的变步长LMS自适应滤波算法[J]. 电子测量技术, 2015, 38(4): 27–31. doi: 10.3969/j.issn.1002-7300.2015.04.007

CHEN Yong, TIAN Jinpeng, and LIU Yanping. New variable step size LMS adaptive filtering algorithm[J]. Electronic Measurement Technology, 2015, 38(4): 27–31. doi: 10.3969/j.issn.1002-7300.2015.04.007

YASUKAWA H, SHIMADA S, and FURUKAWA I. Acoustic echo canceller with high speech quality[C]. IEEE International Conference on Acoustics, Speech, and Signal Processing, Dallas, USA, 1987: 2125–2128. doi: 10.1109/ICASSP.1987.1169314.

叶华, 吴伯修. 变步长自适应滤波算法的研究[J]. 电子学报, 1990, 18(4): 63–70. doi: 10.3321/j.issn:0372-2112.1990.04.012

YE Hua and WU Boxiu. The study on a variable step size adaptive filtering algorithm[J]. Acta Electronica Sinica, 1990, 18(4): 63–70. doi: 10.3321/j.issn:0372-2112.1990.04.012

GITLIN R, MAZO J, and TAYLOR M. On the design of gradient algorithms for digitally implemented adaptive filters[J]. IEEE Transactions on Circuit Theory, 1973, 20(2): 125–136. doi: 10.1109/tct.1973.1083627

耿艳香, 徐文超, 张铮, 等. 基于箕舌线函数的变步长自适应均衡的研究[J]. 实验室科学, 2010, 13(4): 111–113. doi: 10.3969/j.issn.1672-4305.2010.04.037

GENG Yanxiang, XU Wenchao, ZHANG Zheng, et al. Research on the variable step adaptive equalizer based on Versoria function[J]. Laboratory Science, 2010, 13(4): 111–113. doi: 10.3969/j.issn.1672-4305.2010.04.037

施引文献

资源附件(0)

访问统计