Single Base Station Localization Algorithm Based on B-LM Ring of Scattering Model Using NLOS Information
-
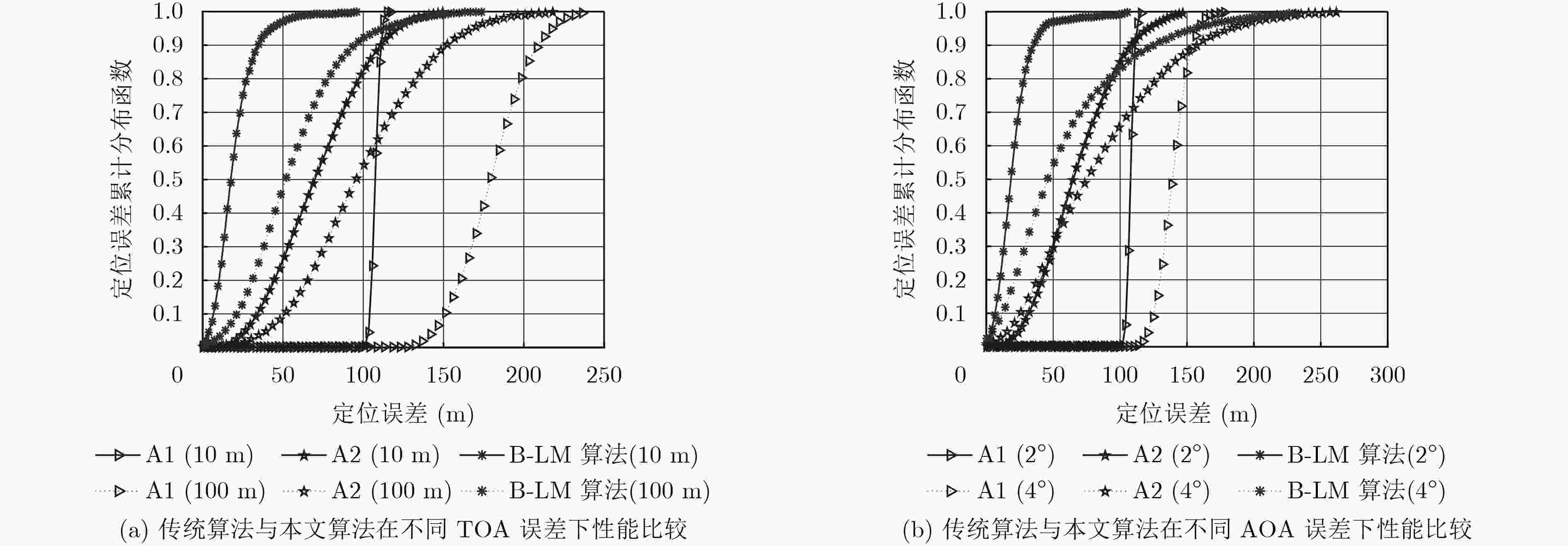
摘要: 针对当前室外蜂窝网多基站定位需要基站之间时间同步、数据同步的要求,以及NLOS环境造成的非服务区基站的信号可测性问题,该文提出基于B-LM圆环模型的NLOS信息约束单基站定位算法。首先根据散射体、目标和基站间的几何位置关系以及NLOS多路径信息构建定位方程,然后将定位方程转化为最小二乘优化问题,之后基于LM算法海森矩阵修正思想和拟牛顿2阶偏导构造思想提出B-LM算法,保证算法收敛于最优解,以得到目标位置。仿真结果表明,所提单基站定位算法能在宏蜂窝NLOS环境实现较高的定位精度。Abstract: Considering the requirements of time and data synchronization in multi-BS (Base Station) positioning in the current outdoor cellular network and the problem of signals’ detectability in area without service BS due to NLOS (Non-Line-Of-Sight) environment, a single base station localization algorithm based on B-LM (Broyden Fletcher Goldfarb Shanno-Levenberg Marquard) ring of scattering model using NLOS information is proposed. Firstly, the localization objective equation is constructed according to the geometric positions of the scatterers, the target, the base station and the NLOS multipath information. Then, the localization equation is transformed into the least square optimization problem. Finally, the B-LM algorithm based on Hessian matrix modification methodology in LM algorithm and the construction of second order partial derivative in quasi-Newton algorithm is proposed, which ensures the localization algorithm converges to the optimal solutions to obtain the target’s location. The simulation results show that the proposed single base station localization algorithm can achieve a high positioning accuracy in the NLOS environment for macrocell.
-
表 1 B-LM算法伪代码
输入: 每条路径到达时间 ${\tau _i}$和到达角 ${\alpha _i}$; 输出: 目标位置 $\left( {x,y} \right)$; (1) 选取可行域内目标初始位置 ${{{X}}} \in \operatorname{int} {{{g}}}\left( {{{X}}} \right)$,设置算法参数
$\varepsilon = 0.01$,尺度因子 $\sigma = 10$, ${{B}} = {{I}}$,最大容忍误差 $\xi = {10^{ - 3}}$
和最大迭代次数 $T = 50$;(2) for $k = 1:T\;$ (3) 计算 ${ψ} \left( {{{{{X}}}_k}} \right)$, $\nabla {ψ} \left( {{{{{X}}}_k}} \right)$, ${{{{J}}}_k}$; (4) 根据式(14)更新迭代方向 ${{δ} _k}$; (5) 根据Armijo准则[17]确定搜索步长 ${\lambda _k}$,根据式(11)更新 ${{{{X}}}_{k + 1}}$; (6) 计算下一时刻目标函数1阶偏导 $\nabla {ψ} \left( {{{{{X}}}_{k + 1}}} \right)$,计算 ${{{{q}}}_k}$和 ${{{{p}}}_k}$; (7) 根据式(15)更新 ${{{B}}_{k + 1}}$; (8) 计算 ${ψ} \left( {{{{{X}}}_{k + 1}}} \right)$; (9) if ${ψ} \left( {{{{{X}}}_{k + 1}}} \right) < {ψ} \left( {{{{{X}}}_k}} \right)$ then (10) if ${\left\| {\Delta {{{{X}}}_k}} \right\|_2} \le \xi $ then (11) ${{{X}}} = {{{{X}}}_{k + 1}}$ and break; (12) else (13) $\mu : = \mu /\sigma $, $k: = k + 1$并且返回第3行; (14) end if (15) else (16) if ${\left\| {\Delta {{{{X}}}_k}} \right\|_2} \le \xi $ then (17) ${{{X}}} = {{{{X}}}_k}$ and break; (18) else (19) $\mu : = \mu \sigma $, $k: = k + 1$,并且返回第3行; (20) end if (21) end if (22) end for -
王建辉, 陈乐然, 胡捍英. 一种新的蜂窝网NLOS误差抑制算 法[J]. 电子与信息学报, 2008, 30(6): 1424–1427 doi: 10.3724/SP.J.1146.2006.01471WANG Jianhui, CHEN Leran, and HU Hanying. A new algorithm to mitigate NLOS errors in cellular networks[J]. Journal of Electronics&Information Technology, 2008, 30(6): 1424–1427 doi: 10.3724/SP.J.1146.2006.01471 GARCIA N, WYMEERSCH H, LARSSON E G, et al. Direct localization for massive MIMO[J]. IEEE Transactions on Signal Processing, 2017, 65(10): 2475–2487 doi: 10.1109/TSP.2017.2666779 ABU-SHABAN Z, ZHOU Xiangyun, and ABHAYAPALA T D. A novel TOA-based mobile localization technique under mixed LOS/NLOS conditions for cellular networks[J]. IEEE Transactions on Vehicular Technology, 2016, 65(11): 8841–8853 doi: 10.1109/TVT.2016.2517151 COMPAGNONI M, NOTARI R, ANTONACCI F, et al. On the statistical model of source localization based on range difference measurements[J]. Journal of the Franklin Institute, 2017, 354(15): 7183–7214 doi: 10.1016/j.jfranklin.2017.07.034 WANG L and ZAWODNIOK M J. Bias and CRB analysis of LoS-based and RSS-based ranging methods[J]. IEEE Transactions on Vehicular Technology, 2016, 65(11): 9085–9097 doi: 10.1109/TVT.2016.2518166 SHI Weiguang, QI Xiaoli, LI Jianxiong, et al. Simple solution to the optimal deployment of cooperative nodes in two-dimensional TOA-based and AOA-based localization system[J]. Eurasip Journal on Wireless Communications&Networking, 2017, 2017(1): 1–16 doi: 10.1186/s13638-017-0859-6 钱志鸿, 王雪. 面向5G通信网的D2D技术综述[J]. 通信学报, 2016, 37(7): 1–14 doi: 10.11959/j.issn.1000-436x.2016129QIAN Zhihong and WANG Xue. Reviews of D2D technology for 5G communication networks[J]. Journal on Communications, 2016, 37(7): 1–14 doi: 10.11959/j.issn.1000-436x.2016129 刘申建, 万群, 彭应宁. 非直达波条件下的TDD高精度移动定位算法[J]. 电子学报, 2002, 30(9): 1288–1291 doi: 10.3321/j.issn:0372-2112.2002.09.009LIU Shenjian, WAN Qun, and PENG Yingning. A non-line-of-sight high-resolution location algorithm based on TDD for mobile station[J]. Acta Electronica Sinica, 2002, 30(9): 1288–1291 doi: 10.3321/j.issn:0372-2112.2002.09.009 WAN Qun, YANG Wanlin, and PENG Yingnig. Closed-form solution to mobile location using linear constraint on scatterer[J]. Electronics Letters, 2004, 40(14): 883–884 doi: 10.1049/el:20040581 ZHAOUNIA M, LANDOLSI M A, and BOUALLEGUE R. Mobile localization under non-line-of-sight conditions using scattering information[J]. International Journal of Wireless Information Networks, 2010, 17(1/2): 1–10 doi: 10.1007/s10776-010-0117-x SHIU D S, FOSCHINI G J, GANS M J, et al. Fading correlation and its effect on the capacity of multielement antenna systems[J]. IEEE Transactions on Communications, 2000, 48(3): 502–513 doi: 10.1109/26.837052 周杰, 朱慧娟, 袁梅. 基于二维空间域移动通信统计信道的空时特性[J]. 电子技术应用, 2016, 42(8): 116–120 doi: 10.16157/j.issn.0258-7998.2016.08.029ZHOU Jie, ZHU Huijuan, and YUAN Mei. Analysis of mobile communication in a two-dimensional sacttering channel model[J]. Application of Electronic Technique, 2016, 42(8): 116–120 doi: 10.16157/j.issn.0258-7998.2016.08.029 BORHANI A and PATZOLD M. A unified disk scattering model and its angle-of-departure and time-of-arrival statistics[J]. IEEE Transactions on Vehicular Technology, 2013, 62(2): 473–485 doi: 10.1109/TVT.2012.2227859 AL-JAZZAR S, CAFFERY J, and YOU H R. Scattering-model-based methods for TOA location in NLOS environments[J]. IEEE Transactions on Vehicular Technology, 2007, 56(2): 583–593 doi: 10.1109/TVT.2007.891491 ABDI A, BARGER J, and KAVEH M. A parametric model for the distribution of the angle of arrival and the associated correlation function and power spectrum at the mobile station[J]. IEEE Transactions on Vehicular Technology, 2002, 51(3): 425–434 doi: 10.1109/TVT.2002.1002493 BORHANI A and PATZOLD M. A non-stationary one-ring scattering model[C]. IEEE Wireless Communications and Networking Conference (WCNC), Shanghai, China, 2013: 2620–2625. CHEN Liang, DU Cuizhen, and MA Yanfang. The higher-order Levenberg–Marquardt method with Armijo type line search for nonlinear equations[J]. Optimization Methods and Software, 2017, 32(3): 516–533 doi: 10.1080/10556788.2016.1225214 YUAN Gonglin, SHENG Zhou, WANG Bopeng, et al. The global convergence of a modified BFGS method for nonconvex functions[J]. Journal of Computational&Applied Mathematics, 2017: 274–294 doi: 10.1016/j.cam.2017.05.030 -





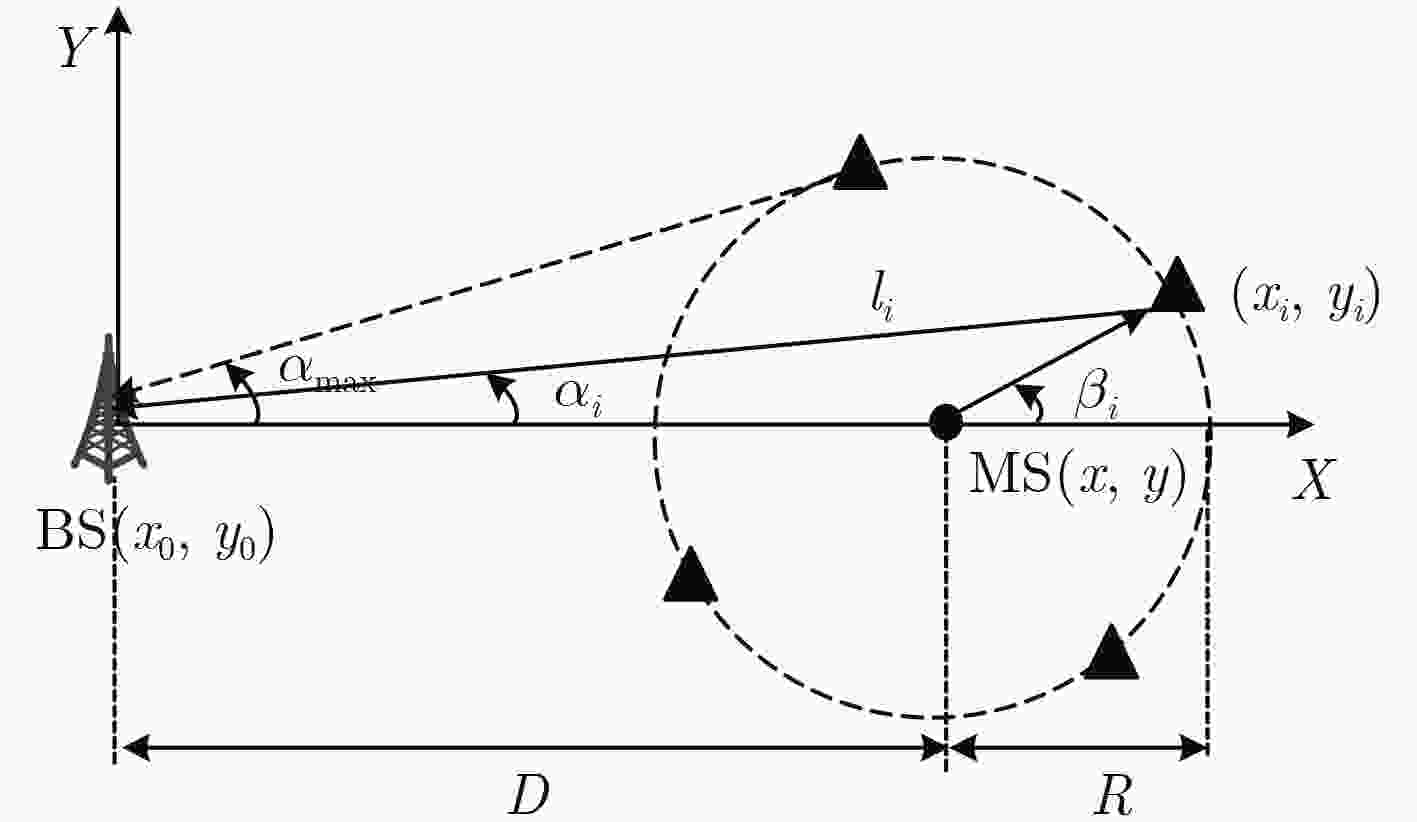
 下载:
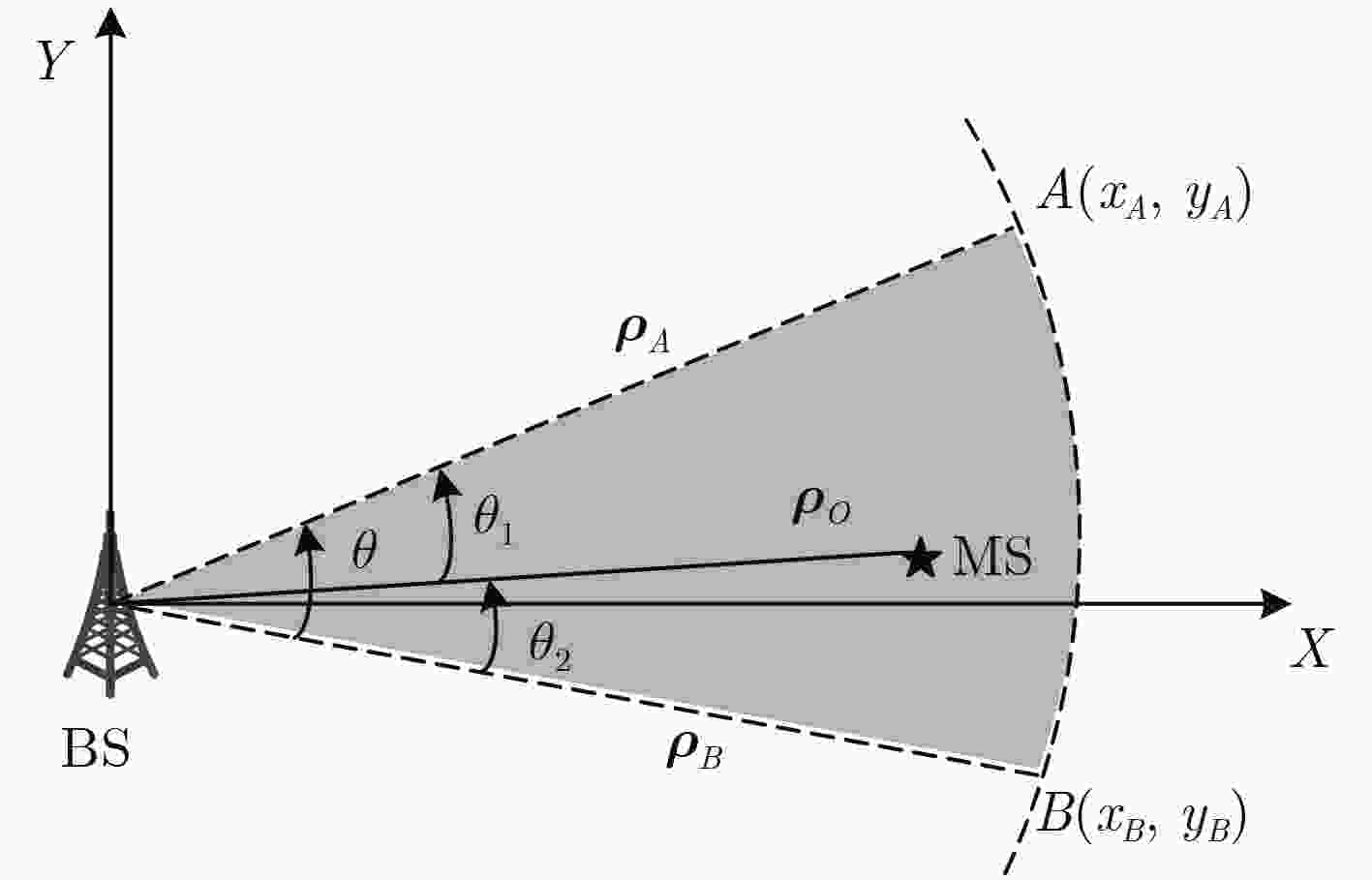
下载:

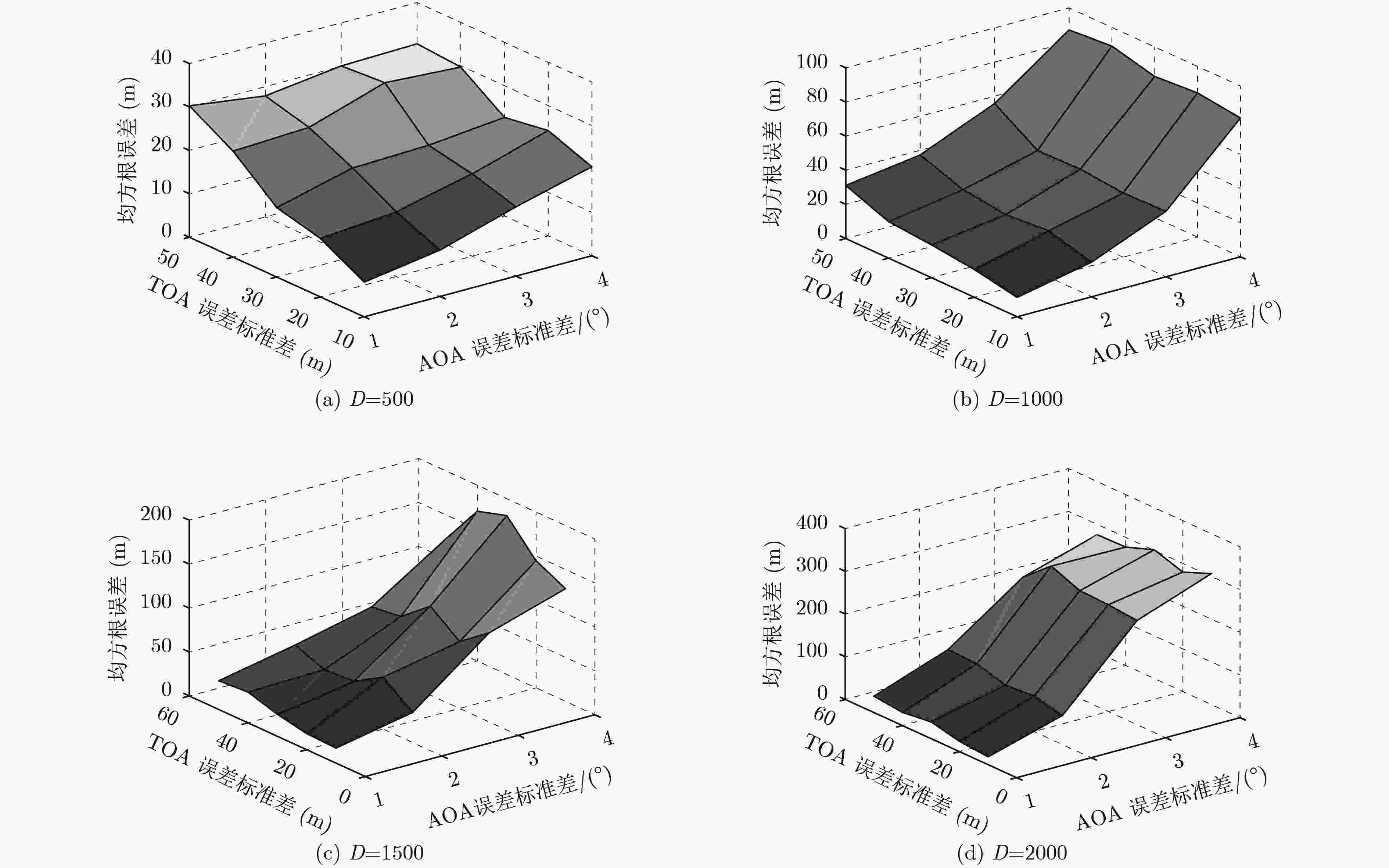



 下载:
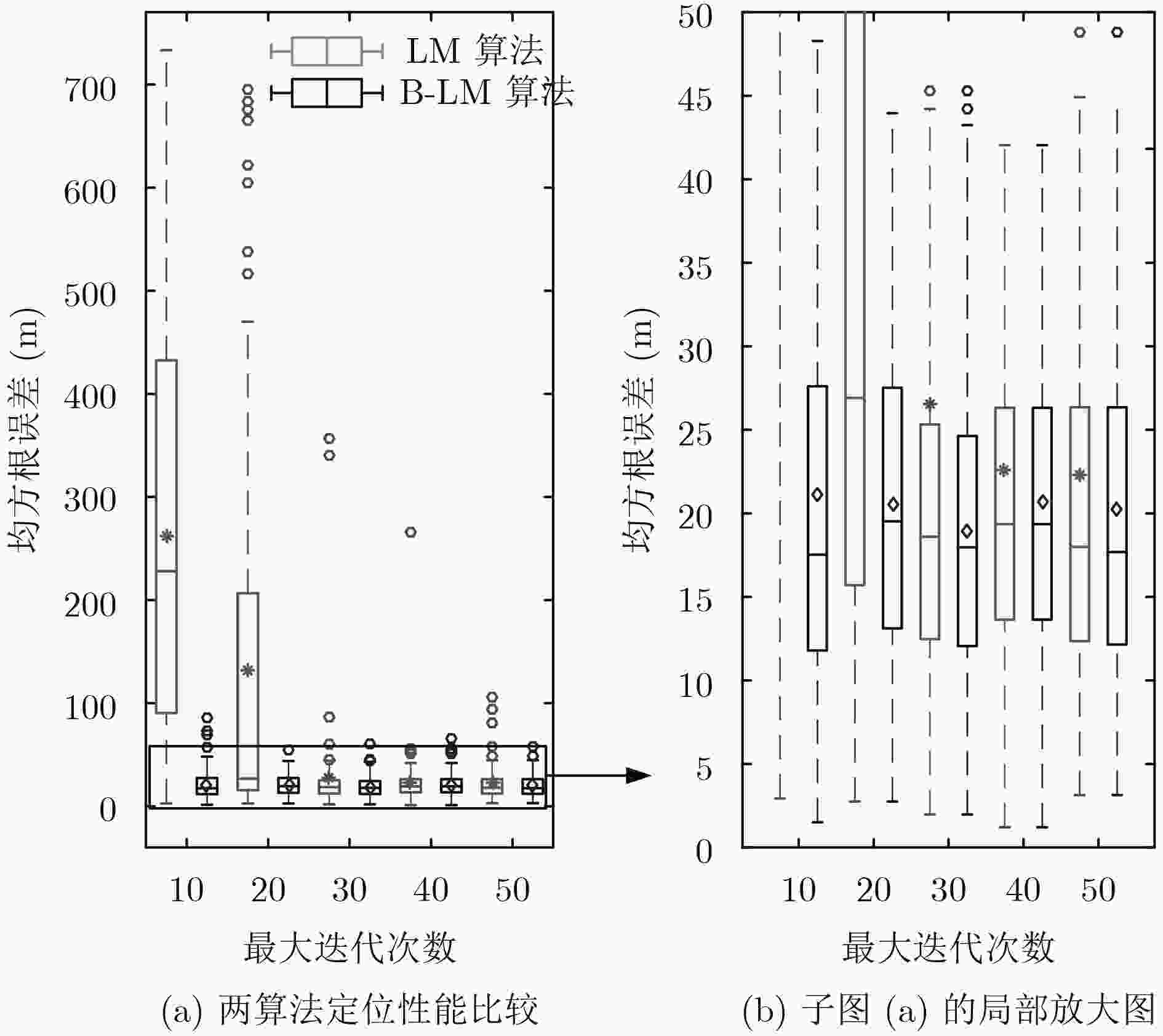
下载:
