基于全局最优的快速一致性点漂移算法
doi: 10.3724/SP.J.1146.2011.00916
Fast Coherent Point Drift Algorithm Based on Global Optimization
-
摘要: 目前受到广泛关注和研究的一致性点漂移(CPD)算法是一种基于高斯混合模型的点模式匹配算法,虽然该算法具有较强的鲁棒性,但其存在局部最优性和收敛速度随点集大小增加而下降等问题。针对上述问题,该文提出了一种新的基于全局最优的快速一致性点漂移算法。该算法首先将点集进行正交标准形约简,利用约简后点集的重要性质,推导出不完全观测数据的对数似然函数在全局最优解附近凸函数区域的边界值,再以该边界值为基础,采用多重初始化策略来实现全局最优。最后,提出了基于置信域的全局收敛二次平方迭代期望最大化算法,实现了全局优化算法的超线性收敛。模拟仿真与真实数据实验验证了该文算法是有效的、快速的以及鲁棒性较强的。Abstract: Currently, the Coherent Point Drift (CPD) which based on Gaussian Mixture Model (GMM) is one of popular point pattern matching algorithms because of its robustness. However, The CPD is local optimization and its convergent rate is slower along with the size of point-set become larger. For resolving these problems, this paper presents a Global Optimal and Fast algorithm which based on CPD (GOF-CPD). The orthogonal normalization first reduce the general affine case to the orthogonal case, and the convex region boundary of the unoberserved datas log likelihood nearby the global optimal solutions are deduced by the properties of normalized point-sets. Then, the multi-start strategy based on the convex region boundary is introduced to achieve the global optimization. Finally, a new iterative scheme, called the Trust Region based global convergent SQUARed iterative EM (TR-gSQUAREM), is proposed to achieve the superlinear convergence. Experiments on both synthetic point-sets and real world data show that the proposed algorithm is efficient, speedy and robust.
-
1. 引言
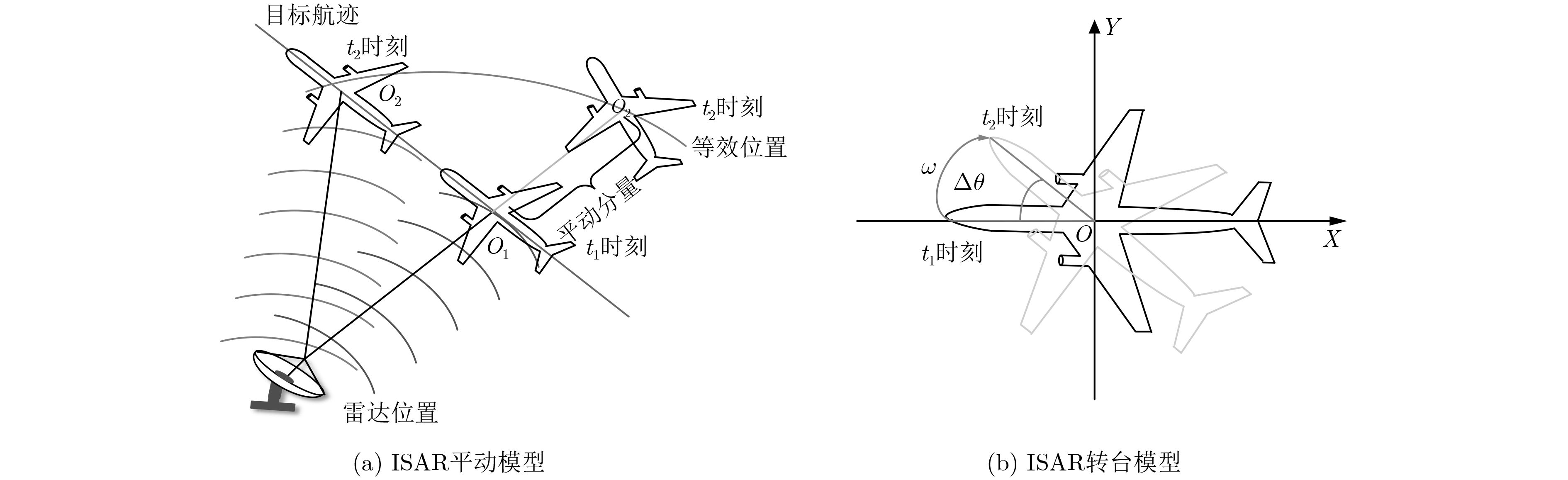
逆合成孔径雷达(Inverse Synthetic Aperture Radar, ISAR)是通过大带宽信号和雷达与目标之间的相对运动实现非合作目标2维成像的雷达,在目标成像和识别中有重要的应用。它的距离成像分辨率由信号带宽决定,方位成像分辨率由雷达波长和脉冲积累时间决定[1]。微波光子雷达结合了光子学方法和电子学方法[2–5],解决了大带宽信号的产生问题,提高了雷达的信号的距离向分辨力,配合较短的波长,能够实现分辨率更高的ISAR图像。文献[6]中所提微波光子雷达系统基于光子波形产生方法发射了具有4 GHz带宽以及10 GHz载频的线性调频信号。文献[7]中微波光子ISAR系统发射调频连续波,并且采用解线频调模式接收信号,在降低计算量的同时实现了8 GHz带宽信号的高分辨成像。但在针对运动目标的2维成像过程中,大带宽和较小波长的信号也使目标转动分量引起的2次项距离徙动不可忽略。在距离维中,该项形成空变的距离弯曲项,具体表现为离目标旋转中心距离越远的散射点的弯曲越大;在方位维中,该项形成空变的2次项相位误差,较大的2次项相位误差将导致图像严重散焦。
ISAR成像可分为包络对齐和相位补偿两个步骤。传统ISAR包络对齐方法有互相关法等[8,9]。传统方法通过计算相邻脉冲数据的相关系数确定回波包络的偏移量。此类方法没有考虑目标的转动分量对包络的影响。而在距离向高分辨的情况下,目标的转动分量会引起包络的偏移。在相位补偿方面,传统ISAR成像通常采用的自聚焦方法[10,11](PGA,特显点,最小熵等)认为相位误差只和方位慢时间有关,忽略了相位误差的距离空变性。而微波光子雷达信号的信号波长较小,转动引起的2次相位误差不可忽略。
综上所述,在带宽较大、波长较小的微波光子雷达ISAR成像处理中,包络和相位的校正都需要更高精度的补偿。针对微波光子雷达中的成像难点,本文提出了一种基于转速估计的包络相位高精度联合运动补偿方法。该方法首先利用包络相关值为准则迭代求解目标转速,其次在距离时域进行时间重采样校正转动分量引起的包络弯曲,最后在方位时域构造距离空变的补偿函数校正转动分量引起的2次相位,从而实现2维高分辨成像。
2. ISAR成像模型
2.1 信号模型
图1(a)为目标运动和雷达录取回波示意图。微波光子雷达发射线性调频信号,采用Dechirp模式接收。雷达录取回波为
Secho(tr,tm)=q∑i=1Airect(tr−2Ri(tm)/cTp)⋅exp[−j(4πcΔRi(tm)γtr+4πcfcΔRi(tm))]⋅exp[j(8πγRsc2ΔRi(tm)+4πγc2ΔRi(tm)2)](1) ΔRi(tm)=Ri(tm)−Rs (2) 其中,
tr 为快时间,tm 为慢时间,rect(⋅) 为门函数,Tp 为脉冲时间宽度,γ 为信号调频率,c 为光速,q 为散射点总数,Ai 为目标散射点i 的后向散射系数,Ri(tm) 为目标散射点i 在慢时间时刻tm 与雷达的距离,Rs 为系统参考斜距。在式(2)中,令fr=γtr ,忽略RVP项、回波包络斜置项和相位常数项,并将目标的斜距历程Ri(tm) 展开为转动分量和平动分量,得Secho(fr,tm)=q∑i=1AiP(fr)exp[−j4πc(fr+fc)⋅(Xisinωtm+Yicosωtm+ΔRTR(tm))] (3) P(fr)=rect(fr/γ−2Ri(tm)/cTp) (4) 其中,
ω 为目标转速,转角Δθ=ωtm ,Xi 和Yi 分别为散射点i 的横、纵坐标,ΔRTR(tm) 为目标平动分量。2.2 微波光子雷达成像分析
假设平动补偿后,旋转中心
O 的坐标为(Xo,Yo) ,信号在tm=0 处进行泰勒展开得Srot(fr,tm)=q∑i=1AiP(fr)exp{−j4πc(fr+fc)⋅[(Xi−Xo)ωtm+(Yi−Yo)(1−12ω2t2m)]} (5) 微波光子雷达带宽大,距离分辨率高,因此目标转动分量产生的2次项距离徙动是微波光子雷达信号处理中必须考虑的问题。另一方面微波光子雷达频段高,中心频率
fc 较大,转动分量产生的距离空变的2次相位误差会造成严重散焦[12]。设目标散射点坐标为(Xi,Yi) ,聚焦位置为Ri(tm) ,合成孔径时间为tM ,散射点i 的2次距离徙动ΔR 与相位误差ΔP 为ΔR=Ri(tM/2)−RM(0)=14(Yi−Yo)ω2t2M (6) ΔP(tM/2)=πfc2c(Yi−Yo)ω2t2M (7) 假设目标纵向长度为60 m,转速
ω 为0.2 rad/s,合成孔径时间tM 为1 s,选取目标最远点作为参考,不同频段传统雷达与微波光子雷达在观测相同目标时的距离徙动与相位误差如表1所示。表 1 不同频段雷达成像误差分析信号频段 发射带宽(GHz) 中心频率(GHz) 距离分辨率(m) ΔR(m) ΔP(rad) L波段 0.3 1.5 0.500 0.6 3 C波段 0.5 6.0 0.300 0.6 12 X波段 1.0 10.0 0.150 0.6 20 微波光子 10.0 35.0 0.015 0.6 70 图2(a)为不同频段雷达的距离徙动量分析,纵坐标为目标最远点距离徙动
ΔR 与距离分辨率ρr 的比值(ΔR/ρr ),横坐标为慢时间tm 。图2(b)为不同频段雷达的相位误差分析,纵坐标为目标最远点相位误差,横坐标为慢时间tm 。由表1和图2可知:相较于传统雷达,在微波光子雷达ISAR成像处理中,由于系统2维分辨率高,目标转动分量对包络的影响远远大于传统雷达,使得利用传统的相关法进行包络对齐处理的效果差。另外由于雷达波长较小,中心频率高,信号相位中的转动分量引起的2次相位误差对成像的影响也不可忽略,而传统的自聚焦方法无法解决此类空变的相位误差问题。3. 基于转速估计的包络相位高精度联合运动补偿方法
针对微波光子雷达ISAR成像处理中出现的问题,本文提出了一种基于转速估计的包络相位高精度联合运动补偿方法。首先去除包络中的走动分量,对式(5)作Keystone变换,即令
tm=fc/(fr+fc)τm ,并对fr 进行泰勒展开,忽略相位常数项后为Skrot(fr,τm)=q∑i=1AiP(fr)exp{−j4πfcc⋅[(Xi−Xo)ωτm−12(Yi−Yo)ω2τ2m]}⋅exp[−j4πfrc(Yi−Yo)(1+12ω2τ2m)] (8) 式(7)中存在随距离变化的2次项
12(Yi−Yo)ω2τ2m ,经本文2.2节分析可得此项对包络有明显影响,具体体现为与旋转中心相对距离越远的包络线有严重的弯曲现象。将式(8)变换到距离时域为skrot(t′,τm)=q∑i=1AiTpsinc{Tp[t′+2γc(1+12ω2τ2m)⋅(Yi−Yo)]}exp(−j4πRΔict′)⋅exp{−j4πfcc[(Xi−Xo)ωτm−12(Yi−Yo)ω2τ2m]} (9) 补偿距离弯曲项目的是令散射点包络重新聚焦在位置
(Yi−Yo) 处。在距离频域补偿关于fr 的距离弯曲项时,由于距离弯曲项是根据散射点距离向位置发生变化的,补偿函数应与散射点位置相关。而在距离频域时,不同距离向位置的散射点频域信号叠加在一起,因此使用同一补偿函数无法在频域对不同位置散射点的距离弯曲项进行统一补偿。所以本文从式(9)出发,使用时间重采样完成弯曲项校正。重采样尺度由转速ω 确定,即令t′=(1+12ω2τ2m)tn ,a(τm)=(1+12ω2τ2m) ,式(9)写为skrot(tn,τm)=q∑i=1AiTpsinc{a(τm)Tp[tn+2γc(Yi−Yo)]}⋅exp(−j4πRΔica(τm)tn)⋅exp{−j4πfcc[(Xi−Xo)ωτm−12(Yi−Yo)ω2τ2m]} (10) 在进行该操作之前需估计转速
ω 的值,常用的ISAR目标转角估计方法主要分为2类:一类是基于信号的转速估计[12–16],此类方法需要包络完全对齐后提取信号调频率估计转速;另一类为基于图像匹配的转速估计方法[17–21],此类方法需要完成成像后对图像进行匹配提取转速。而在微波光子成像处理中,由于包络和相位存在转动分量误差,传统转速估计方法的估计精度无法保证。利用微波光子雷达距离分辨率高的特点,本文提出了一种以包络相关值为目标函数的转速迭代求解方法。因时间重采样不改变中心时刻
τm=0 时的包络,取中心时刻包络与其余时刻包络相关值之和作为判断整体包络对齐的准则。设参数ω 第d 步迭代的转速估计值为ω[d] ,并用ω[d] 对式(10)进行时间重采样,令t′=[1+12(ω[d])2τ2m]tn ,Ki,ω=2γc ⋅(1+12ω2τ2m)(Yi−Yo) ,重采样并取实包络后写为|skrot(tn,τm,ω[d])|=q∑i=1AiTp ⋅sinc{Tp[(1+12(ω[d])2τ2m)tn+Ki,ω]} (11) 令中心时刻的实包络为
L0=∑qi=1AiTpsinc{Tp[tn+ 2γ/c(Yi−Yo)]} ,则目标函数为J(ω[d])=M∑m=1,m≠na/2N∑n=1[|skrot(tn,τm,ω[d])|L0] (12) 当
ω 的估计值准确时,J(ω[d]) 的值达到最大,即˜ω=argmaxJ(ω[d]) (13) 由于
J(ω[d]) 是单峰函数,使用线搜索的方法可求ω 的估计值,并利用˜ω 进行运动补偿。算法步骤如下:(1) 选取转速初始值
ω[0] ,一般为0值,选取初始步长Δω0 ,计算J(ω[d]) 的最大值:J(ω[d])max= ∑Mm=1,m≠na/2∑Nn=1(L02) ;(2) 第
d 步迭代:令第d 步步长为Δωd=Δω0 ,ω[d]=ω[d−1]+Δωd ,计算若J(ω[d])max>J(ω[d])> J(ω[d−1]) ,继续执行第(2)步,进行下一次迭代。若J(ω[d])<J(ω[d−1])<J(ω[d])max ,转第3步操作。若J(ω[d])=J(ω[d])max ,迭代停止,输出˜ω=ω[d] ;(3) 当
J(ω[d])<J(ω[d−1]) 时,说明ω[d]>˜ω ,此时进行反向搜索,并减小步长,令Δωd= αΔω0,α<1 ,ω[d]=ω[d−1]−Δωd ,若J(ω[d])< J(ω[d−1]) ,迭代停止,输出J(ω[d]),d=0,1,2··· ,˜ω=argmaxJ(ω[d]) 。若J(ω[d])=J(ω[d])max ,迭代停止,输出˜ωω[d] 。在利用线搜索得到
ω 的估计值后,沿着方位向构造距离空变的相位补偿项phase|xo,yi=exp[j2πfcc(Yi−Yo)ω2τ2m] (14) 将式(10)与式(14)补偿相位共轭相乘得
s′krot(tn,τm)=skrot(tn,τm)⋅conj(phase|xo,yi)=q∑i=1AiTpsinc{a(τm)Tp[tn+2γc(Yi−Yo)]}⋅exp(−j4πRΔica(τm)tn)⋅exp{−j4πfcc(Xi−Xo)ωτm} (15) 忽略残余相位的影响,将式(14)变换到方位频域即可完成ISAR成像。
s′krot(tn,fa)=q∑i=1AiTpsinc{a(τm)⋅Tp[tn+2γc(Yi−Yo)]}⋅sinc{Ta[fa−2ωfcc(Xi−Xo)]} (16) 式(16)为本文算法的成像表达式,目标散射点方位向聚焦位置为
(Xi−Xo) ,距离向聚焦位置为(Yi−Yo) 。本文算法成像流程如图3所示。4. 仿真实验与实测验证
4.1 仿真实验分析
为验证本文算法的有效性,本文首先进行成像仿真实验。仿真参数如表2所示。
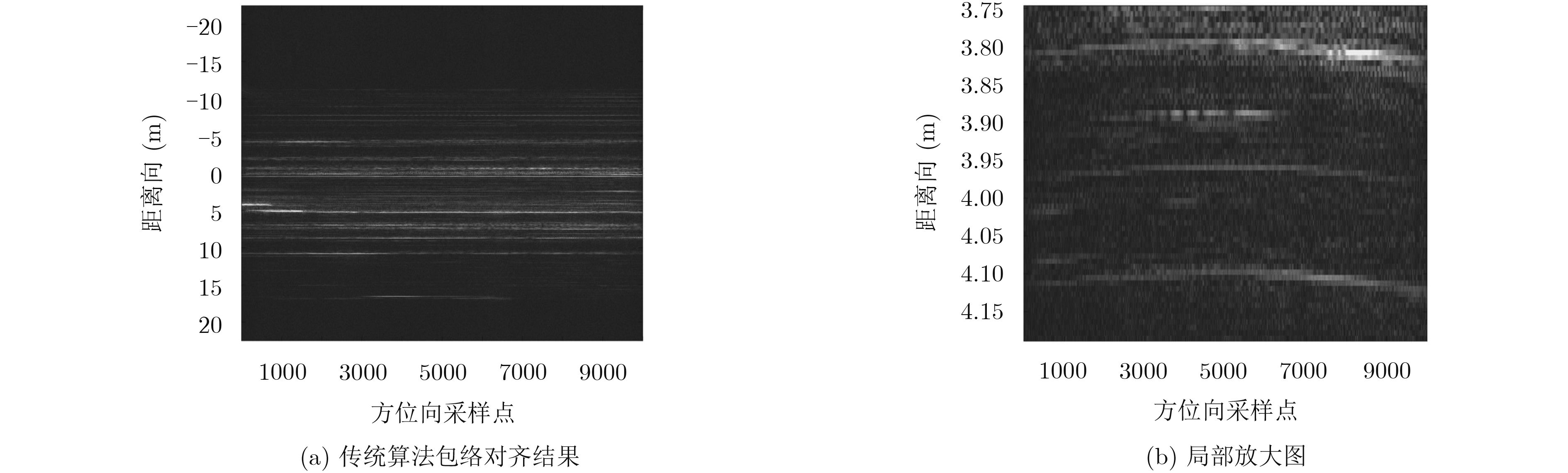
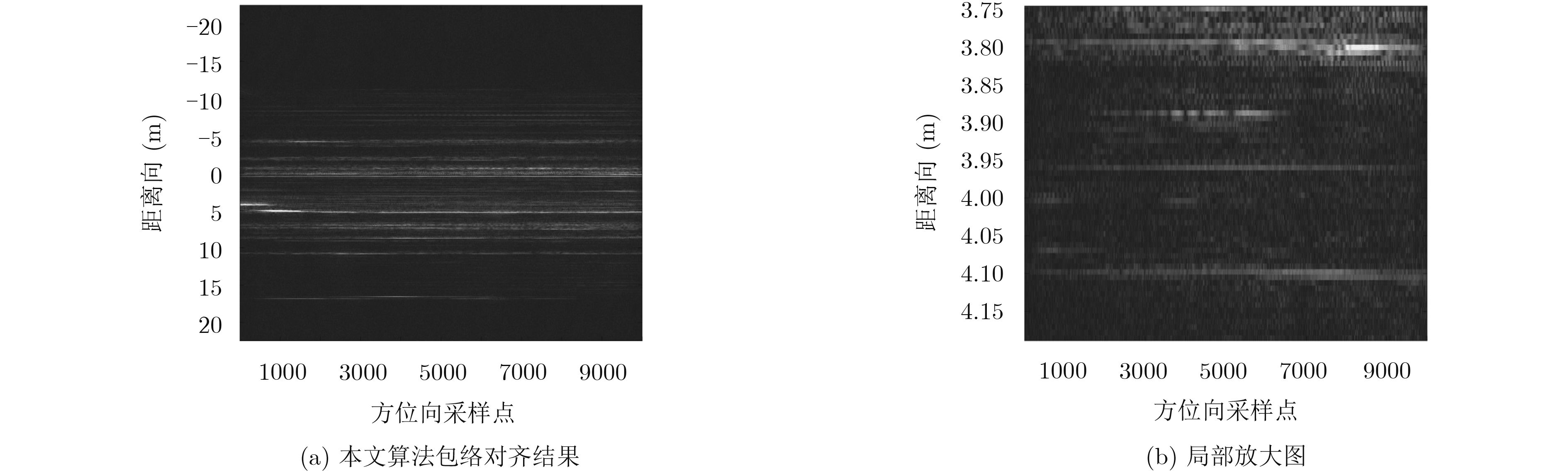
表 2 仿真数据参数信号带宽 载频 脉冲宽度 脉冲重复频率 采样率 参考斜距 目标速度 观测时间 10 GHz 35 GHz 150 μs 6000 Hz 500 MHz 750 m 83 m/s 1.33 s 图4(a)为传统方法处理的包络线,在包络相关对齐之后使用Keystone变换校正包络。图4(b)和图4(c)为其局部放大图,可以看到信号中仍然存在由转动分量引起的距离弯曲项,传统包络对齐法往往不考虑这一影响,导致包络对齐效果不理想。图5(a)为本文算法处理的包络线,在Keystone变换操作完成后,本文所讲转速估计方法确定重采样尺度,算法经过7次迭代计算后,得到转速估计值为0.115 rad/s。然后对数据进行时间重采样后对齐目标包络线。图5(b)和图5(c)为其局部放大图,数据段与图5(b)和图5(c)相对应,可以看到距离空变的弯曲已经消除。
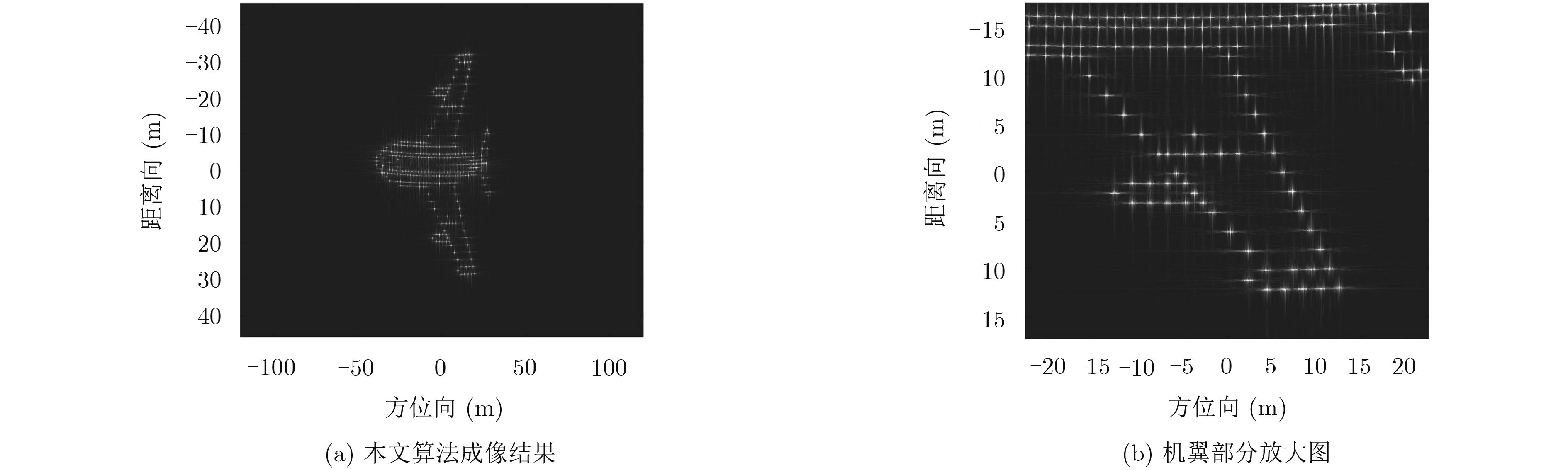
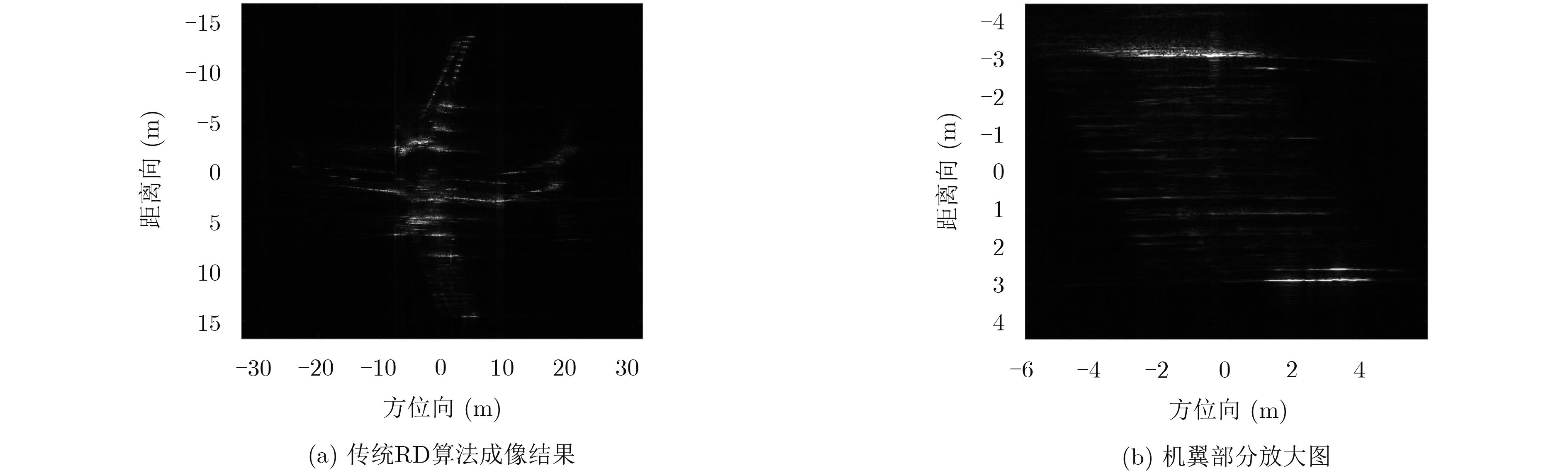
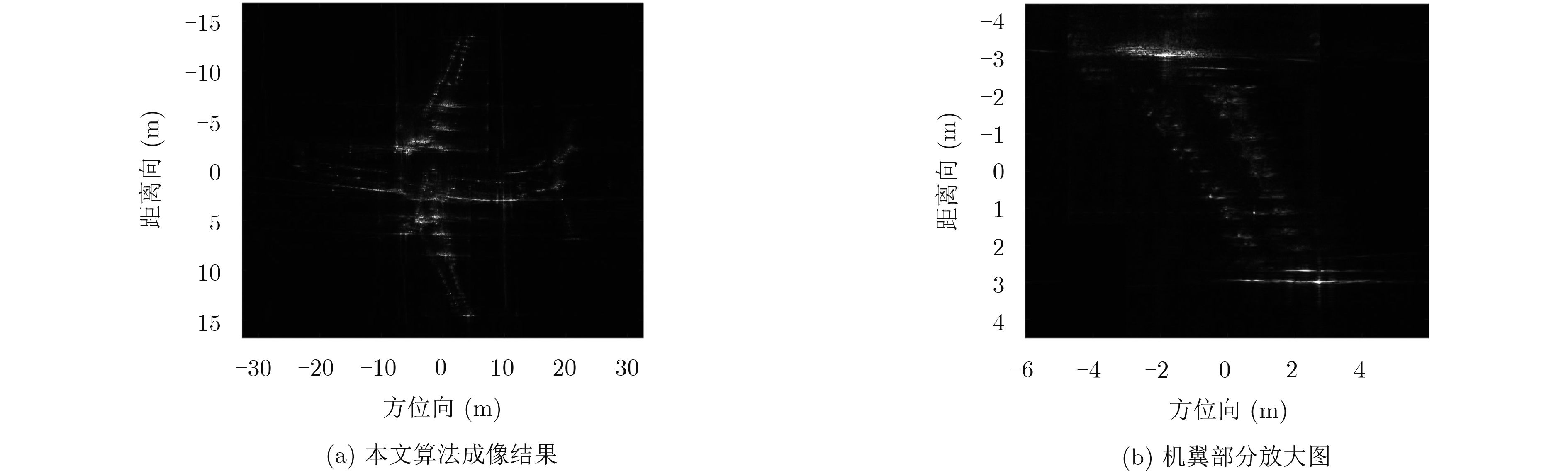
图6(a)和图6(b)分别为传统RD算法成像结果和机翼部分放大结果,由于传统算法不考虑距离空变的2次相位对成像结果的影响,导致聚焦效果差。图7(a)和图7(b)为本文算法成像结果和机翼部分放大结果,在估计得到目标转速后根据相对距离位置构造2次补偿函数对数据进行补偿,使得聚焦效果明显提升。
4.2 实测数据验证
实验参数如表3所示,目标为民航飞机。
表 3 实测数据参数信号带宽 载频 脉冲宽度 数据采样率 脉冲重复频率 观测时间 10 GHz Ka波段 150 μs 500 MHz 6670 Hz 1.2 s 图8(a)、图9(a)分别为传统算法和本文算法包络对齐结果,图8(b)中的局部放大图中包络有明显的弯曲现象,而经过本文算法处理后,得到转速估计值为0.124 rad/s,经过时间重采样后相同区域的包络对齐结果如图9(b)所示,可以看到距离空变的包络弯曲现象得到了校正。
图10(a)和图10(b)分别为传统RD算法成像结果和机翼部分放大结果,图11(a)和图11(b)分别为本文算法成像结果和机翼部分放大结果。本文算法成像聚焦性能更好,在飞机目标的机翼部分成像效果对比中可以看到:传统RD算法因为忽略了转动相位对包络和相位的影响,散射点严重展宽;而本文算法有效地去除了目标转动对包络和相位的影响,获得了更好的聚焦效果。
5. 结束语
微波光子雷达具有超高2维分辨率,有着广阔的应用前景。而在微波光子雷达信号处理中,由于信号带宽大、波长小,转动分量的影响不可忽略。传统ISAR成像算法一般不考虑目标运动中的转动分量对回波包络和相位的影响,不适用于微波光子雷达成像。本文提出了更有效的ISAR成像算法,并且在ISAR目标转速估计方法上有所创新。该方法利用微波光子雷达距离分辨率高的特点,以包络相关值为目标函数迭代估计目标转速。然后利用得到的转速估计值有效校正了回波包络的弯曲现象,并对转动分量引起的方位2次相位作了有效补偿,获得了更好的聚焦效果。
期刊类型引用(10)
1. 高永胜,谭佳俊,王瑞琼. 基于光子学的微波移频方法研究. 电子与信息学报. 2023(06): 2123-2133 .  本站查看
本站查看2. 陈学斌,叶春茂,张彦,胡庆荣. 含旋转部件的ISAR目标分离式成像. 信号处理. 2021(02): 209-221 .  百度学术
百度学术3. 杨磊,夏亚波,毛欣瑶,廖仙华,方澄,高洁. 基于分层贝叶斯Lasso的稀疏ISAR成像算法. 电子与信息学报. 2021(03): 623-631 .  本站查看
本站查看4. 谢意远,高悦欣,邢孟道,郭亮,孙光才. 跨谱段SAR散射中心多维参数解耦和估计方法. 电子与信息学报. 2021(03): 632-639 .  本站查看
本站查看5. 范北辰,杨悦,马丛,王祥传,张方正,潘时龙. 微波光子雷达组网技术. 雷达科学与技术. 2021(02): 195-207+216 .  百度学术
百度学术6. 王安乐,王党卫,余岚. 微波光子成像雷达技术发展综述. 雷达科学与技术. 2021(02): 217-224+232 .  百度学术
百度学术7. 侯颖妮,谢洁. 稀疏采样数据大转角高分辨ISAR成像技术研究. 雷达科学与技术. 2021(05): 604-609+624 .  百度学术
百度学术8. 赵忠凯,陈通. ISAR基带干扰系统设计与实现. 应用科技. 2020(03): 24-29 .  百度学术
百度学术9. 俞传龙. 逆合成孔径雷达成像算法的研究与仿真. 电子设计工程. 2020(23): 183-187 .  百度学术
百度学术10. 熊强强,陈黎艳,姚卫国,曾美琳. 可调光子滤波器微波信号接收码元速率估计. 激光杂志. 2020(12): 181-184 .  百度学术
百度学术其他类型引用(9)
-

计量
- 文章访问数: 3820
- HTML全文浏览量: 198
- PDF下载量: 1733
- 被引次数: 19






 下载:
下载:




















































































































 百度学术
百度学术
 下载:
下载: