Beamforming Design for IRS-assisted D2D Communication System Under Urban Street Scenarios
-
摘要: 针对城市街道场景下蜂窝用户和D2D通信用户共享频谱以及城市街道下无线信道特性,该文提出一种IRS辅助的联合波束成形设计方法。在D2D链路信号与干扰加噪声比的约束下,以最大化蜂窝用户容量为目标,设计了最优的波束形成向量、相移矩阵和D2D链路发射功率。引入松弛变量将非凸且变量耦合的优化问题转换为解耦后的凸优化问题和二分法搜索功率分配,采用黎曼共轭梯度算法对反射相移矩阵进行优化。仿真结果表明,所提算法收敛性较好,且与基准方案相比能有效地提升用户信道容量。Abstract: Considering the spectrum sharing between cellular users and D2D users, and the wireless channel characteristic of urban streets, an Intelligent Reflecting Surface (IRS)-assisted joint beamforming method is proposed. Under the constraints of signal to interference plus noise ratio for D2D link, the parameter including optimal beamforming vectors and phase-shift matrices and D2D transmitting powers are designed with the objective of maximizing cellular user capacity. The nonconvex coupling variable optimization problem is transformed into the convex decoupling variable optimization problem and binary search power allocation by introducing slack variables, and the reflection phase-shift matrices are also optimized with Riemann conjugate gradient algorithms. Simulation results show that the proposed algorithm has perfect convergence and higher user channel capacity comparing with the baseline schemes.
-
Key words:
- Intelligent Reflecting Surface (IRS) /
- D2D /
- Beamforming /
- Alternating optimization
-
1 二分法搜索功率算法
给定基站波束形成向量${\boldsymbol{w}}$和反射相移矩阵$ {\boldsymbol{\varTheta }} $,初始化功率$ {P_{\text{t}}}^{\left( 0 \right)} $,右边界${r^{\left( 0 \right)}} = {P_{{\text{max}}}}$,左边界${l^{\left( 0 \right)}} = 0$,$i = 0$和迭代更新精度${\varepsilon _1} > 0$; (1) 循环 (2) 计算${P_{{\text{mid}}}}^{\left( i \right)} = \dfrac{{{l^{\left( i \right)}} + {r^i}}}{2}$,其中${P_{{\text{mid}}}}^{\left( i \right)}$是搜索范围的中间值; (3) 计算目标函数的值${\gamma _{\text{c}}}\left( {{P_{{\text{mid}}}}^{\left( i \right)}} \right)$,如果$\left| {{\gamma _{\text{c}}}\left( {{P_{{\text{mid}}}}^{\left( i \right)}} \right) - {\gamma _{\text{c}}}\left( {{P_{{\text{mid}}}}^{\left( {i - 1} \right)}} \right)} \right| < {\varepsilon _1}$,则${P_{{\text{mid}}}}^{\left( i \right)}$是最优解的一部分,搜索结束; (4) 判断目标函数的值${\gamma _{\text{c}}}\left( {{P_{{\text{mid}}}}^{\left( i \right)}} \right)$分母是否为 0。如果分母为0,说明${P_{\text{t}}} = 0$是最优解,直接返回目标值为0; (5) 如果${\gamma _{\text{c}}}\left( {{P_{{\text{mid}}}}^{\left( i \right)}} \right)$大于目标值,则${P_{{\text{mid}}}}^{\left( i \right)}$说明可能是最优解的一部分,将${P_{{\text{mid}}}}^{\left( i \right)}$作为新的右边界,返回步骤 2。如果${\gamma _{\text{c}}}\left( {{P_{{\text{mid}}}}^{\left( i \right)}} \right)$小于
目标值,则说明${P_{{\text{mid}}}}^{\left( i \right)}$不可能是最优解的一部分,将${P_{{\text{mid}}}}^{\left( i \right)}$作为新的左边界,返回步骤2。2 基于迭代的黎曼共轭梯度算法
给定基站波束形成向量${\boldsymbol{w}}$,DS发射功率${P_{\text{t}}}$。初始化${{\boldsymbol{\theta }}_0}$,$ {{\boldsymbol{\eta }}_0} = - {{\mathrm{grad}}} {\gamma _{\text{c}}}({{\boldsymbol{\theta }}_0}) $,$i = 0$和迭代更新精度${\varepsilon _2} > 0$; (1) 循环 (2) 选择Armijo回溯线搜索步长$\alpha $; (3) 根据(30)更新${\boldsymbol{\theta }}$; (4) 计算新的${\gamma _{\text{d}}}$,如果${\gamma _{\text{d}}} \ge {\gamma ^{{\text{req}}}}$,则保持当前的优化变量不变;否则,将优化变量投影到约束空间${C}$中,即${{\boldsymbol{\theta }}_i} = {\text{Pro}}{{\text{j}}_{C}}({{\boldsymbol{\theta }}_i})$; (5) 根据(24)更新黎曼梯度; (6) 根据(28)更新搜索方向; (7) $i \leftarrow i + 1$; (8) until $ \Vert \mathrm{grad}{\gamma }_{\text{c}}({{\boldsymbol{\theta}} }_{i}){\Vert }_{2}\le {\varepsilon}_{2} $。 3 问题P1的交替优化算法
初始化${{\boldsymbol{w}}^{(0)}}$, $P_t^{(0)}$和${{\boldsymbol{\varTheta }}^{(0)}}$和迭代更新精度${\varepsilon _3} > 0$; (1) 循环 (2) 给定${{\boldsymbol{\varTheta }}^{(k)}}$,$P_t^{(k)}$,使用CVX工具求解子问题P1.3,更新${{\boldsymbol{w}}^{(k + 1)}}$; (3) 给定${{\boldsymbol{\varTheta }}^{(k)}}$,${{\boldsymbol{w}}^{(k + 1)}}$,基于算法1,更新$P_t^{(k + 1)}$; (4) 给定${{\boldsymbol{w}}^{(k + 1)}}$,$P_t^{(k + 1)}$,基于算法2,更新${{\boldsymbol{\theta }}^{k + 1}}$,${{\boldsymbol{\varTheta }}^{(k + 1)}} = {{\mathrm{diag}}} \left( {{{\boldsymbol{\theta}}^{k + 1}}} \right)$; (5) 根据迭代结果${{\boldsymbol{w}}^{(k + 1)}}$,$P_t^{(k + 1)}$和${{\boldsymbol{\varTheta }}^{(k + 1)}}$,计算CU用户容量$ {\gamma _{\text{c}}}^{\left( {k + 1} \right)} $; (6) until $ \left| {{{\log }_2}\left(\dfrac{{1 + {\gamma _{\text{c}}}^{\left( {k + 1} \right)}}}{{1 + {\gamma _{\text{c}}}^{\left( k \right)}}}\right)} \right| < {\varepsilon _3} $。 表 1 仿真参数设置
仿真参数设置 取值 仿真参数设置 取值 拐角损耗$ {L_{{\text{corner}}}} $(dB) 20 IRS单元间隔与电磁波波长比值 0.5 街道宽度${w_1}$, ${w_2}$(m) 20 与IRS相关的路径损耗指数(dB) 2 IRS反射单元数 100 BS到D2D路径损耗指数(dB) 3.5 基站天线数 4 D2D用户之间路径损耗指数(dB) 3 D2D用户和蜂窝用户天线数 1 莱斯衰落因子${\varepsilon _x}$(dB) 3 D2D链路最小SINR需求$ {\gamma ^{{\text{req}}}} $(dBm) 1 单位路径损耗(dB) 30 基站最大发射功率$P$(dBm) 20 噪声功率谱密度(dBm·Hz–1) –170 D2D链路最大发射功率${P_{\max }}$(dBm) 5 误差精度 0.001 -
[1] AGIWAL M, ROY A, and SAXENA N. Next generation 5G wireless networks: A comprehensive survey[J]. IEEE Communications Surveys & Tutorials, 2016, 18(3): 1617–1655. doi: 10.1109/COMST.2016.2532458. [2] ZHANG Lin, XIAO Ming, WU Gang, et al. Efficient scheduling and power allocation for D2D-assisted wireless caching networks[J]. IEEE Transactions on Communications, 2016, 64(6): 2438–2452. doi: 10.1109/TCOMM.2016.2552164. [3] LIANG Le, LI G Y, and XU Wei. Resource allocation for D2D-enabled vehicular communications[J]. IEEE Transactions on Communications, 2017, 65(7): 3186–3197. doi: 10.1109/TCOMM.2017.2699194. [4] 申滨, 孙万平, 张楠, 等. 基于加权二部图及贪婪策略的蜂窝网络D2D通信资源分配[J]. 电子与信息学报, 2023, 45(3): 1055–1064. doi: 10.11999/JEIT220029.SHEN Bin, SUN Wanping, ZHANG Nan, et al. Resource allocation based on weighted bipartite graph and greedy strategy for D2D communication in cellular networks[J]. Journal of Electronics & Information Technology, 2023, 45(3): 1055–1064. doi: 10.11999/JEIT220029. [5] STRINATI E C, ALEXANDROPOULOS G C, WYMEERSCH H, et al. Reconfigurable, intelligent, and sustainable wireless environments for 6G smart connectivity[J]. IEEE Communications Magazine, 2021, 59(10): 99–105. doi: 10.1109/MCOM.001.2100070. [6] NING Boyu, TIAN Zhongbao, MEI Weidong, et al. Beamforming technologies for ultra-massive MIMO in terahertz communications[J]. IEEE Open Journal of the Communications Society, 2023, 4: 614–658. doi: 10.1109/OJCOMS.2023.3245669. [7] GAO Ying, WU Qingqing, ZHANG Guangchi, et al. Beamforming optimization for active intelligent reflecting surface-aided SWIPT[J]. IEEE Transactions on Wireless Communications, 2023, 22(1): 362–378. doi: 10.1109/TWC.2022.3193845. [8] WANG Zhaorui, LIU Liang, ZHANG Shuowen, et al. Massive MIMO communication with intelligent reflecting surface[J]. IEEE Transactions on Wireless Communications, 2023, 22(4): 2566–2582. doi: 10.1109/TWC.2022.3212537. [9] JIA Shuaiqi, YUAN Xiaojun, and LIANG Yingchang. Reconfigurable intelligent surfaces for energy efficiency in D2D communication network[J]. IEEE Wireless Communications Letters, 2021, 10(3): 683–687. doi: 10.1109/LWC.2020.3046358. [10] NI Yiyang, LIU Yaxuan, WANG Jue, et al. Performance analysis for RIS-assisted D2D communication under Nakagami-m fading[J]. IEEE Transactions on Vehicular Technology, 2021, 70(6): 5865–5879. doi: 10.1109/TVT.2021.3077805. [11] WANG Wenhao, YANG Lei, MENG Anqi, et al. Resource allocation for IRS-aided JP-CoMP downlink cellular networks with underlaying D2D communications[J]. IEEE Transactions on Wireless Communications, 2022, 21(6): 4295–4309. doi: 10.1109/TWC.2021.3128711. [12] CHEN Yuanbin, WANG Ying, ZHANG Jiayi, et al. Resource allocation for Intelligent reflecting surface aided vehicular communications[J]. IEEE Transactions on Vehicular Technology, 2020, 69(10): 12321–12326. doi: 10.1109/TVT.2020.3010252. [13] 张雷, 王玉, 田建杰, 等. 基于IRS辅助的MIMO车联网系统联合波束成形设计[J]. 通信学报, 2023, 44(2): 59–69. doi: 10.11959/j.issn.1000-436x.2023035.ZHANG Lei, WANG Yu, TIAN Jianjie, et al. Joint beam forming design for IRS-aided MIMO Internet of vehicles system[J]. Journal on Communications, 2023, 44(2): 59–69. doi: 10.11959/j.issn.1000-436x.2023035. [14] ERCEG V, SCHILLING D L, GHASSEMZADEH S, et al. Propagation modeling and measurements in an urban and suburban environment using broadband direct sequence spread spectrum[C]. [1992 Proceedings] Vehicular Technology Society 42nd VTS Conference-Frontiers of Technology, Denver, USA, 1992: 317–320. doi: 10.1109/VETEC.1992.245414. [15] ERCEG V, RUSTAKO A J, and ROMAN R S. Diffraction around corners and its effects on the microcell coverage area in urban and suburban environments at 900 MHz, 2 GHz, and 4 GHz[J]. IEEE Transactions on Vehicular Technology, 1994, 43(3): 762–766. doi: 10.1109/25.312770. [16] MASUI H, ISHII M, SAKAWA K, et al. Microwave path-loss characteristics in urban LOS and NLOS environments[C]. IEEE VTS 53rd Vehicular Technology Conference, Spring 2001. Proceedings (Cat. No. 01CH37202), Rhodes, Greece, 2001: 395–398. doi: 10.1109/VETECS.2001.944872. [17] LU J S, BERTONI H L, REMLEY K A, et al. Site-specific models of the received power for radio communication in urban street canyons[J]. IEEE Transactions on Antennas and Propagation, 2014, 62(4): 2192–2200. doi: 10.1109/TAP.2013.2297164. [18] MATOLAK D W, ZHANG Qian, and WU Qiong. Path loss in an urban peer-to-peer channel for six public-safety frequency bands[J]. IEEE Wireless Communications Letters, 2013, 2(3): 263–266. doi: 10.1109/WCL.2013.020513.120919. [19] MATOLAK D W, REMLEY K A, GENTILE C, et al. Peer-to-peer urban channel characteristics for two public-safety frequency bands[J]. IEEE Antennas and Propagation Magazine, 2014, 56(5): 101–115. doi: 10.1109/MAP.2014.6971921. [20] ZHAO Xiongwen, COULIBALY B M, LIANG Xiaolin, et al. Comparisons of channel parameters and models for urban microcells at 2 GHz and 5 GHz [wireless corner][J]. IEEE Antennas and Propagation Magazine, 2014, 56(6): 260–276. doi: 10.1109/MAP.2014.7011070. [21] KIM K W and OH S J. Geometric optics-based propagation prediction model in urban street canyon environments[J]. IEEE Antennas and Wireless Propagation Letters, 2016, 15: 1128–1131. doi: 10.1109/LAWP.2015.2496182. [22] WU Qingqing and ZHANG Rui. Towards smart and reconfigurable environment: Intelligent reflecting surface aided wireless network[J]. IEEE Communications Magazine, 2020, 58(1): 106–112. doi: 10.1109/MCOM.001.1900107. [23] ITU. Propagation data and prediction methods for the planning of short-range outdoor radiocommunication systems and radio local area networks in the frequency range 300 MHz to 100 GHz[R]. Recommendation P. 1411-7, 2013. [24] ZHANG Shuowen and ZHANG Rui. Capacity characterization for intelligent reflecting surface aided MIMO communication[J]. IEEE Journal on Selected Areas in Communications, 2020, 38(8): 1823–1838. doi: 10.1109/JSAC.2020.3000814. [25] LI Renweng, SUN Shu, and TAO Meixia. Ergodic achievable rate maximization of RIS-assisted millimeter-wave MIMO-OFDM communication systems[J]. IEEE Transactions on Wireless Communications, 2023, 22(3): 2171–2184. doi: 10.1109/TWC.2022.3210227. [26] ABSIL P A, MAHONY R, and SEPULCHRE R. Optimization Algorithms on Matrix Manifolds[M]. Princeton: Princeton University Press, 2008: 231–232. doi: 10.1515/9781400830244. [27] GUTIÉRREZ-RUIZ D, GONZALEZ D, CHÁVEZ-CARLOS J, et al. Quantum geometric tensor and quantum phase transitions in the Lipkin-Meshkov-Glick model[J]. Physical Review B, 2021, 103(17): 174104. doi: 10.1103/PhysRevB.103.174104. [28] MUNKRES J. Algorithms for the assignment and transportation problems[J]. Journal of the Society for Industrial and Applied Mathematics, 1957, 5(1): 32–38. doi: 10.1137/0105003. [29] YU Xianghao, XU Dongfang, and SCHOBER R. MISO wireless communication systems via intelligent reflecting surfaces: (Invited paper)[C]. 2019 IEEE/CIC International Conference on Communications in China (ICCC), Changchun, China, 2019: 735–740. doi: 10.1109/ICCChina.2019.8855810. [30] SUN Ruijin, CHENG Nan, ZHANG Ran, et al. Sum-rate maximization in IRS-assisted wireless-powered multiuser MIMO networks with practical phase shift[J]. IEEE Internet of Things Journal, 2023, 10(5): 4292–4306. doi: 10.1109/JIOT.2022.3216449. [31] KUDATHANTHIRIGE D, GUNASINGHE D, and AMARASURIYA G. Performance analysis of intelligent reflective surfaces for wireless communication[C]. ICC 2020–2020 IEEE International Conference on Communications (ICC), Dublin, Ireland, 2020: 1–6. doi: 10.1109/ICC40277.2020.9148760. -






 下载:
下载:



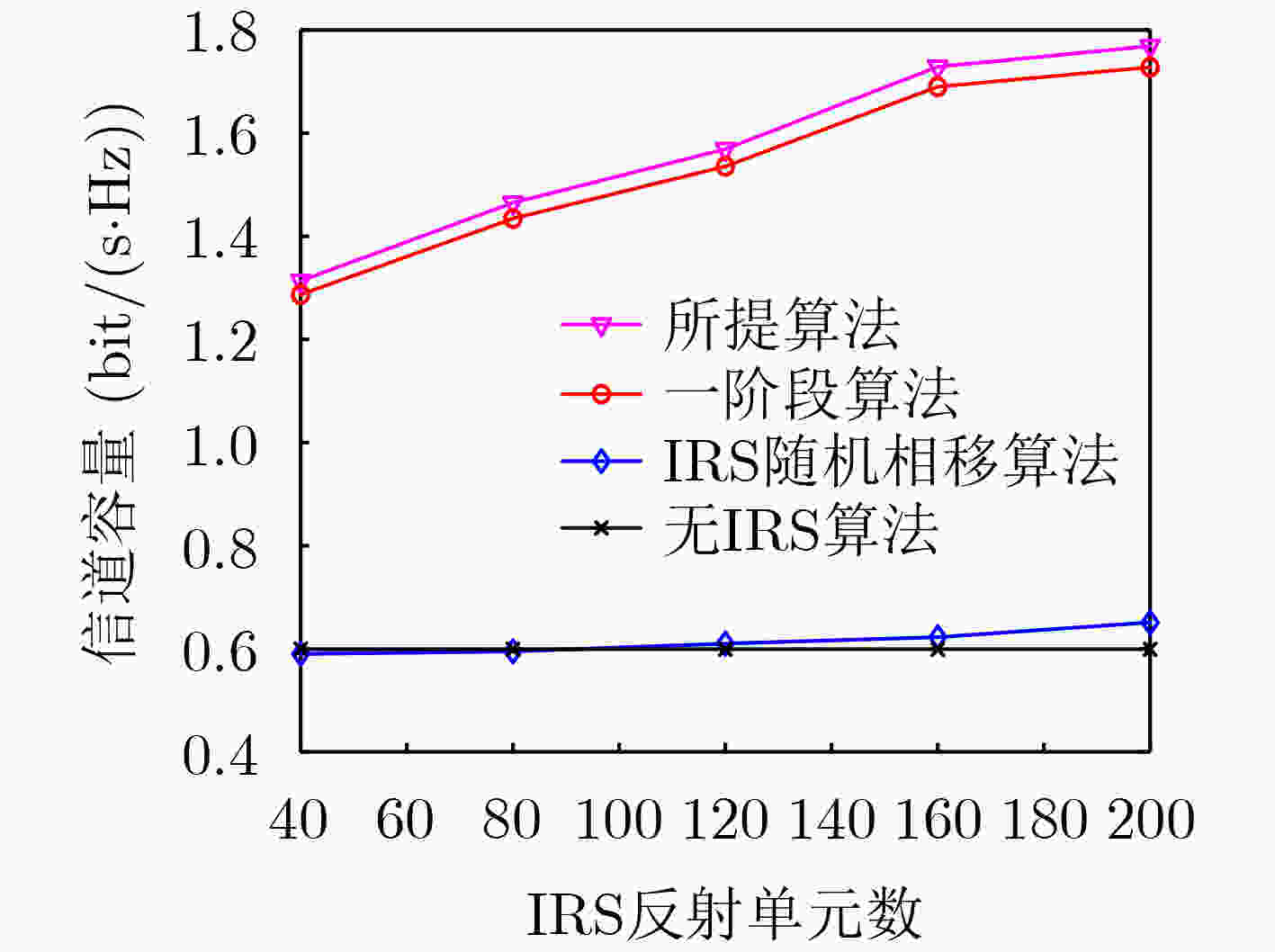


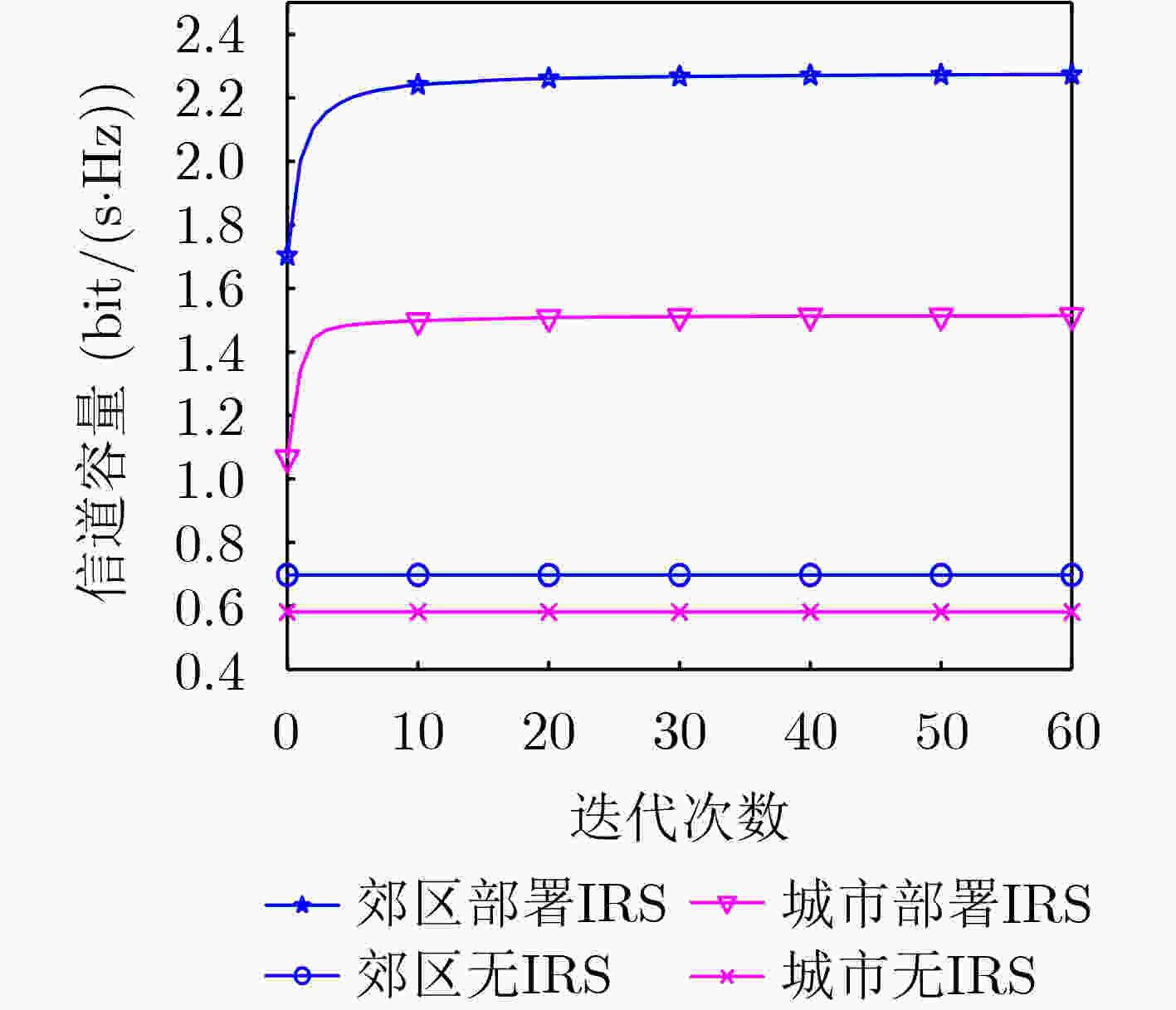



 下载:
下载:
