A Target Tracking Method Based on Box-particle Filter Under Measurement Uncertainty
-
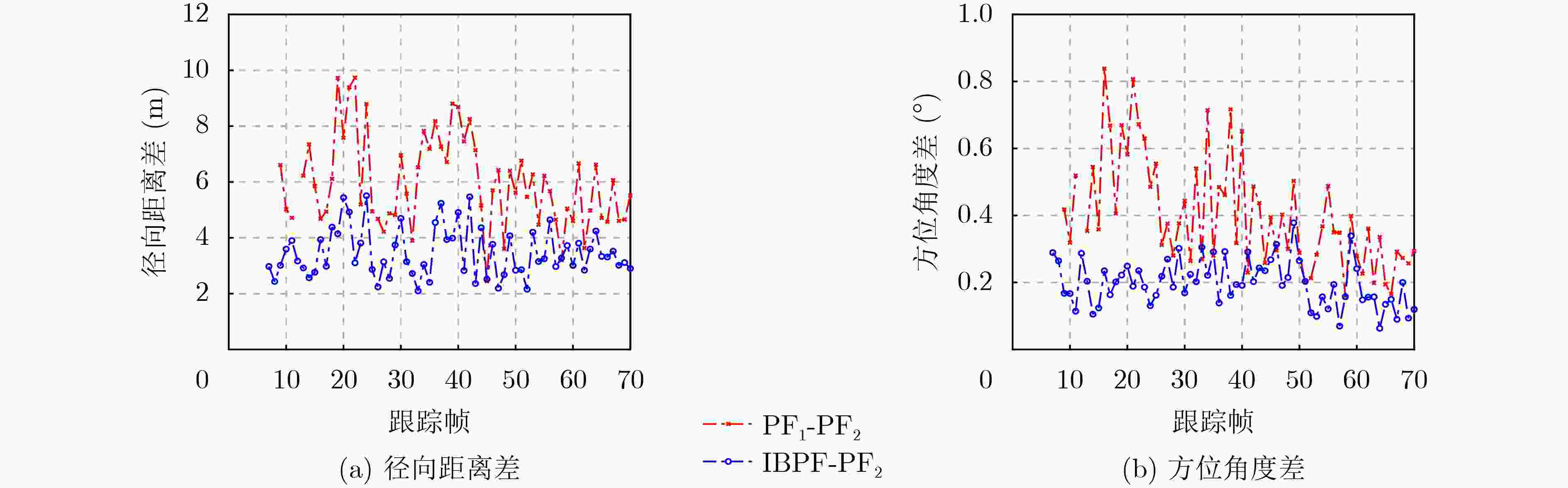
摘要: 在复杂水下环境中,主动声呐的量测值在距离和方位分辨率上存在较大的不确定性,即一个目标回波的能量可能覆盖声呐距离-方位能量谱的多个相邻位置网格。并且,当环境中混响较强时,上述量测不确定性将引起多个区域性的杂波干扰。为了减小状态空间估计的偏差,基于粒子滤波(PF)的跟踪方法需要大量粒子来近似后验概率密度,跟踪的实时性急剧降低。针对上述问题,该文提出一种基于区间量测的箱粒子滤波跟踪方法(IBPF),对主动声呐量测值进行区间表示,即用一个表示距离和方位区间的箱粒子代替点值量测,用区间表示这种量测不确定性,在提高状态估计稳定性的同时,极大程度地减少了后验概率密度估计所需的粒子数,从而进一步提高计算效率。实验结果表明,所提IBPF与PF相比,能以更高的计算效率获得更优的跟踪性能,对目标的跟踪时间缩短了18.06%,跟踪成功帧数增加了4.29%。Abstract: There is significant uncertainty in the measurements of active sonar in terms of range-bearing resolution because of the complex underwater environment. In this case, the energy of the target echo may occupy multiple adjacent coordinate grids in the sonar range-bearing energy spectrum. Moreover, the measurement uncertainty mentioned above will cause multiple regional clutter interferences when there exists strong reverberation in the environment. To reduce the bias of state space estimation, Particle Filtering (PF) based tracking methods require a large number of particles to approximate the posterior probability density, resulting in a rapid decrease in real-time tracking performance. A Box-Particle Filtering tracking method based on Interval measurement (IBPF) is proposed to address the above problem. IBPF utilizes a box particle with range-bearing intervals instead of point measurements of active sonar, which greatly reduces the number of particles required for posterior probability density estimation while improving the stability of state estimation, and can further improve computational efficiency. The experiment result shows that the proposed IBPF achieves better tracking performance with higher computational efficiency, which reduces computation time by 18.06% and increases the number of successful tracking frames by 4.29%.
-
Key words:
- Underwater target tracking /
- Active sonar /
- Box-particle filter /
- Measurement uncertainty
-
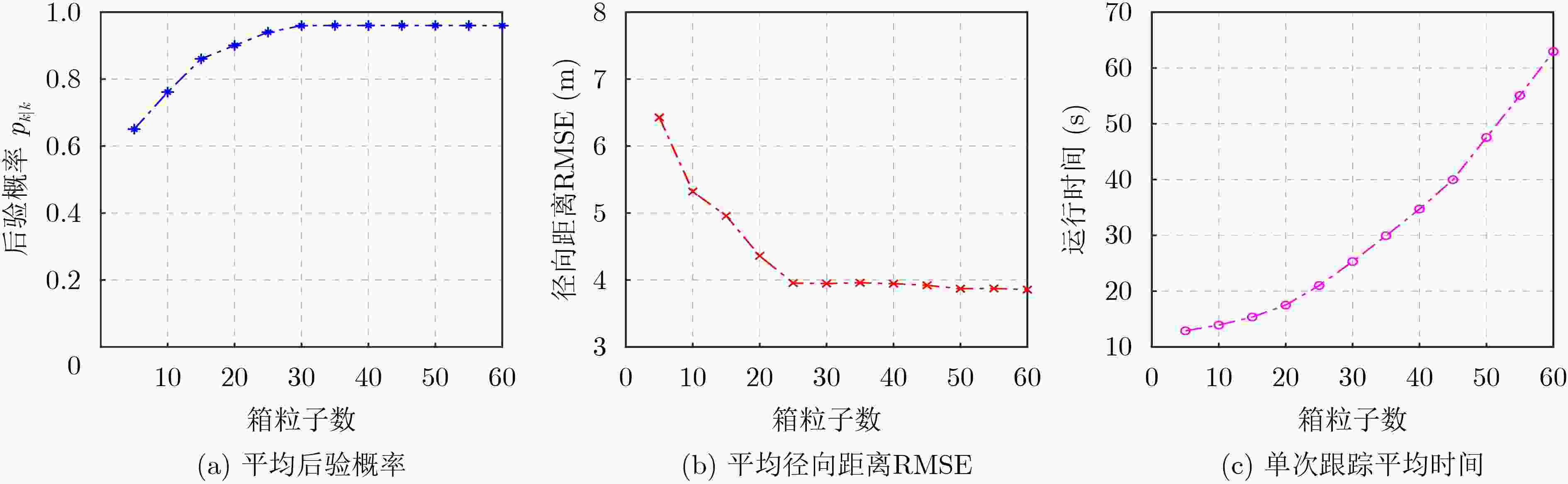
表 1 仿真实验平均计算时间(s)
粒子数/箱粒子数 PF IBPF 1000 13.27 – 2000 16.25 – 5000 31.06 – 10000 43.82 – 5 – 16.32 10 – 18.29 25 – 22.80 50 – 29.87 1 IBPF算法
参数初始化:$ {p_{k\mid k}} $,$ {p_{\text{b}}} $,$ {p_{\text{s}}} $,$ {{{Z}}_k} $,$ \{ \begin{array}{*{20}{c}} {\omega _k^i,}&{[{\boldsymbol{x}}_k^i]} \end{array}\} _{i = 1}^N $ 1. 通过式(14)进行箱粒子状态更新,得到$ [{\boldsymbol{x}}_{p,k}^i] $; 2. 基于$ \mathop Z\nolimits_k $新生箱粒子$ \{ {\omega _{{\mathrm{b}},k}^i,}\;\; {[{\boldsymbol{x}}_{{\mathrm{b}},k}^i]} \} _{i = 1}^{{N_{\mathrm{b}}}} $,其中
$ \omega _{{\mathrm{b}},k}^i = 1/{N_{\mathrm{b}}} $;3. 通过式(14)进行新生箱粒子状态更新,得到$ [{\boldsymbol{x}}_{{\mathrm{b}},k + 1}^i] $; 4. 由式(7)更新目标后验概率$ {p_{k + 1\mid k}} $; 5. 由式(15)预测$k{\text{ + 1}}$时刻持续存在的箱粒子权重$ \omega _{{\mathrm{p}},k + 1}^i $; 6. 由式(16)预测$k{\text{ + 1}}$时刻的新生箱粒子权重$ \omega _{{\mathrm{b}},k + 1}^i $; 7. 进行箱粒子权重联合,得到$ \{ \begin{array}{*{20}{c}} {\omega _{k + 1|k}^i,}&{[{\boldsymbol{x}}_{k + 1|k}^i]} \end{array}\} _{i = 1}^{{N_{\mathrm{u}}}} $; 8. 通过式(11)计算每个箱粒子与量测$ \mathop {{Z}}\nolimits_{k + 1} $的似然函数值; 9. 由式(9)和式(10)更新得到$k{\text{ + 1}}$时刻的后验概率$ {p_{k + 1\mid k + 1}} $; 10. 由式(18)进行箱粒子权重更新,归一化处理后进行重采样,
得到$ \{ \begin{array}{*{20}{c}} {\omega _{k + 1}^i = 1/N,}&{[{\boldsymbol{x}}_{k + 1}^i]} \end{array}\} _{i = 1}^N $;11. 进行循环步骤1–步骤10,到$K$时刻结束; 12. 结束循环,输出目标后验概率和状态估计。 -
[1] STONE L D, STREIT R L, CORWIN T L, et al. Bayesian Multiple Target Tracking[M]. 2nd ed. Boston: Artech House, 2014. [2] ORTON M and FITZGERALD W. A Bayesian approach to tracking multiple targets using sensor arrays and particle filters[J]. IEEE Transactions on Signal Processing, 2002, 50(2): 216–223. doi: 10.1109/78.978377. [3] XU Cong, HE Zishu, LIU Haicheng, et al. Bayesian track-before-detect algorithm for nonstationary sea clutter[J]. Journal of Systems Engineering and Electronics, 2021, 32(6): 1338–1344. doi: 10.23919/JSEE.2021.000113. [4] EDELSON G S. Two-stage active sonar network track-before-detect processing in a high clutter harbor environment[J]. The Journal of the Acoustical Society of America, 2016, 140(S4): 3349. doi: 10.1121/1.4970702. [5] DIAMANT R, KIPNIS D, BIGAL E, et al. An active acoustic track-before-detect approach for finding underwater mobile targets[J]. IEEE Journal of Selected Topics in Signal Processing, 2019, 13(1): 104–119. doi: 10.1109/JSTSP.2019.2899237. [6] HOU Xianghao, YANG Long, YANG Yixin, et al. Bearing-only underwater uncooperative target tracking for non-Gaussian environment using fast particle filter[J]. IET Radar, Sonar & Navigation, 2022, 16(3): 501–514. doi: 10.1049/rsn2.12198. [7] SALMOND D J and BIRCH H. A particle filter for track-before-detect[C]. The 2001 American Control Conference, Arlington, USA, 2001: 3755–3760. doi: 10.1109/ACC.2001.946220. [8] RISTIC B, VO B T, VO B N, et al. A tutorial on Bernoulli filters: Theory, implementation and applications[J]. IEEE Transactions on Signal Processing, 2013, 61(13): 3406–3430. doi: 10.1109/TSP.2013.2257765. [9] DUAN Rui, YANG Kunde, WU Feiyun, et al. Particle filter for multipath time delay tracking from correlation functions in deep water[J]. The Journal of the Acoustical Society of America, 2018, 144(1): 397–411. doi: 10.1121/1.5047671. [10] ZHANG Dianlun, GAO Linsen, SUN Dajun, et al. Soft-decision detection of weak tonals for passive sonar using track-before-detect method[J]. Applied Acoustics, 2022, 188: 108549. doi: 10.1016/j.apacoust.2021.108549. [11] MACAULAY J, KINGSTON A, CORAM A, et al. Passive acoustic tracking of the three-dimensional movements and acoustic behaviour of toothed whales in close proximity to static nets[J]. Methods in Ecology and Evolution, 2022, 13(6): 1250–1264. doi: 10.1111/2041-210X.13828. [12] JANG J, MEYER F, SNYDER E R, et al. Bayesian detection and tracking of odontocetes in 3-D from their echolocation clicks[J]. The Journal of the Acoustical Society of America, 2023, 153(5): 2690–2705. doi: 10.1121/10.0017888. [13] TIAN Mengchu, CHEN Zhimin, WANG Huifen, et al. An intelligent particle filter for infrared dim small target detection and tracking[J]. IEEE Transactions on Aerospace and Electronic Systems, 2022, 58(6): 5318–5333. doi: 10.1109/TAES.2022.3169447. [14] DAUM F and HUANG J. Curse of dimensionality and particle filters[C]. IEEE Aerospace Conference Proceedings, Big Sky, USA, 2003: 1979-1993. doi: 10.1109/AERO.2003.1235126. [15] JAULIN L, KIEFFER M, DIDRIT O, et al. Applied Interval Analysis[M]. London: Springer, 2001: 11-42. doi: 10.1007/978-1-4471-0249-6. [16] MAHLER R P S. Statistical Multisource-Multitarget Information Fusion[M]. Boston: Artech House, 2007: CH5. [17] ABDALLAH F, GNING A, and BONNIFAIT P. Box particle filtering for nonlinear state estimation using interval analysis[J]. Automatica, 2008, 44(3): 807–815. doi: 10.1016/j.automatica.2007.07.024. [18] GNING A, RISTIC B, and MIHAYLOVA L. Bernoulli particle/box-particle filters for detection and tracking in the presence of triple measurement uncertainty[J]. IEEE Transactions on Signal Processing, 2012, 60(5): 2138–2151. doi: 10.1109/TSP.2012.2184538. -






 下载:
下载:






 下载:
下载:
