A Robust Multi-Channel Moving Target Detection Algorithm for Complex Scenes
-
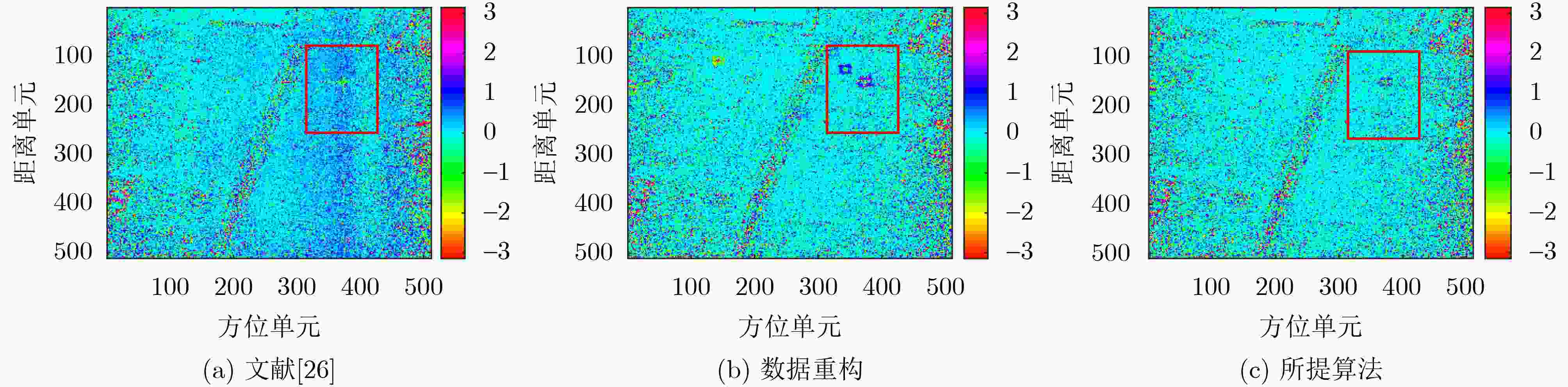
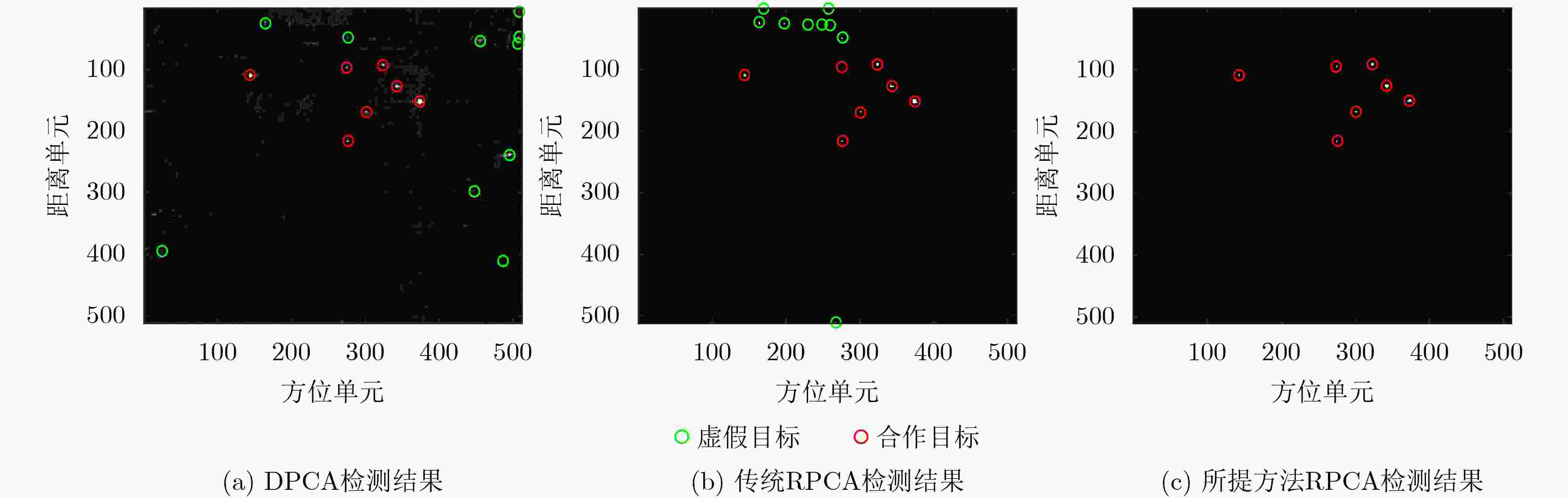
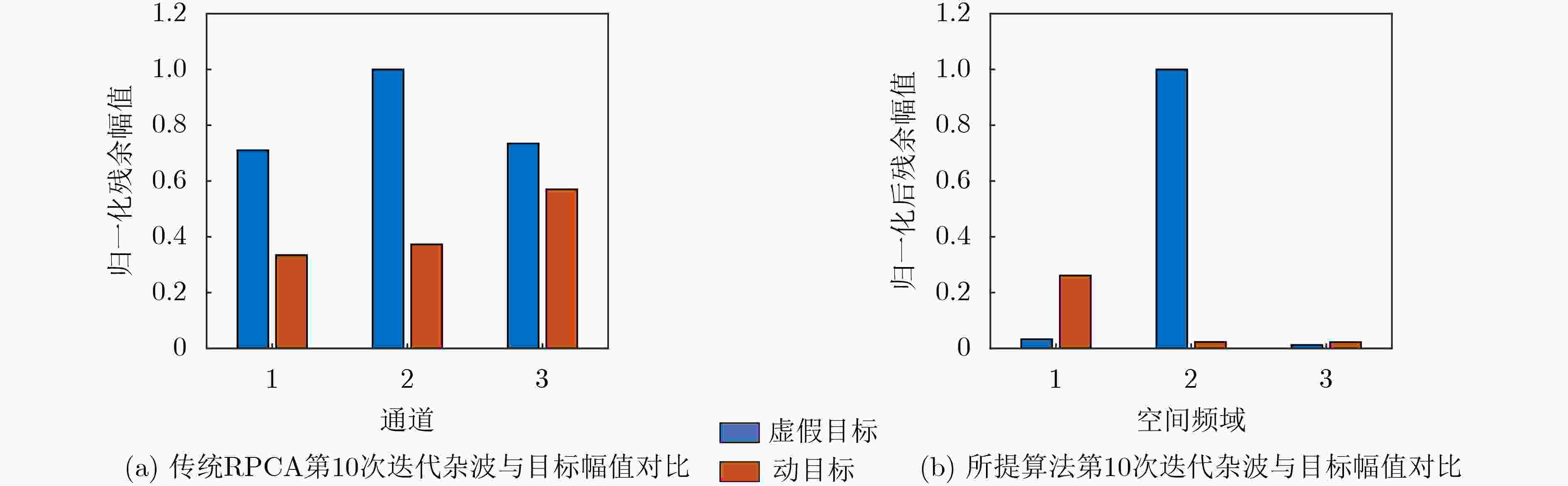
摘要: 针对鲁棒主成分分析(RPCA)算法在多通道慢速地面动目标指示(GMTI)中存在的高虚警以及对通道误差敏感问题,该文提出一种数据重构与速度合成孔径雷达(VSAR)-RPCA联合处理的方法。首先,通过样本挑选与联合像素法完成通道间数据精确重构;然后结合VSAR检测模式提出一种新的RPCA优化模型,通过采用交替投影乘子法对其进行求解得到空间频域的稀疏矩阵,进一步利用动目标与强杂波残余在空间频域通道的分布特性差异实现强杂波残余剔除与动目标检测;最后采用沿航迹干涉算法估计目标径向速度完成动目标重定位。相较于传统RPCA算法,所提算法在非理想强杂波背景下的虚警率显著降低。理论分析与实测实验验证了所提算法的有效性。Abstract: Aiming at the problems of high false alarm and sensitivity to channel error of Robust Principal Component Analysis (RPCA) algorithm in multi-channel Ground Moving Target Indication (GMTI), this paper proposes a data reconstruction and Velocity Synthetic Aperture Radar (VSAR)-RPCA joint processing method. Firstly, the sample selection and joint pixel method are used to complete the accurate reconstruction of inter-channel data; then a new RPCA optimization model is proposed by combining the VSAR detection mode, and the sparse matrix in the spatial frequency domain is obtained by solving the new RPCA optimization model with the alternating projection multiplier method, and then the differences in the distribution characteristics of the moving target and the strong clutter residuals in the spatial frequency domain channel are used to realize the strong clutter residuals rejection and the detection of the moving target; finally, the radial velocity of the target is estimated by the Along-Track Interferometry algorithm to complete the moving target relocation. Compared with the traditional RPCA algorithm, the proposed algorithm significantly reduces the false alarm rate under the background of non-ideal strong clutter. Theoretical analyses and experiments verify the effectiveness of the proposed algorithm.
-
1 传统RPCA模型求解算法
输入:观测矩阵$ {\boldsymbol{D}} $,参数$ \kappa $; 输出:低秩矩阵$ {{\boldsymbol{A}}_{k + 1}} $,稀疏矩阵$ {{\boldsymbol{E}}_{k + 1}} $; 初始化:$ {{\boldsymbol{Y}}_0} $=0,$ {\mu _0} \gt 0 $, $k = 0$, $\delta = 1{\rm{e}} - 6$, ${\mathrm{iter}} = 1000$; While ${\left\| {{\boldsymbol{D}} - {{\boldsymbol{A}}_{k + 1}} - {{\boldsymbol{E}}_{k + 1}}} \right\|_{\rm F}} > \delta $ or $k < {\text{iter}}$ do $ \left( {{\boldsymbol{U,\varXi ,V}}} \right) = {\rm{svd}}\left( {{\boldsymbol{D}} - {{\boldsymbol{E}}_k} + {{{{\boldsymbol{Y}}_k}} \mathord{\left/ {\vphantom {{{{\boldsymbol{Y}}_k}} \mu }} \right. } \mu }} \right) $; $ {{\boldsymbol{A}}_{k + 1}} = {\boldsymbol{U}}{{S}_{{1 \mathord{\left/ {\vphantom {1 \mu }} \right. } \mu }}}\left[ {\boldsymbol{\varXi }} \right]{{\boldsymbol{V}}^{\mathrm{H}}} $; $ {{\boldsymbol{E}}_{k + 1}} = {{S}_{{\kappa \mathord{\left/ {\vphantom {\kappa \mu }} \right. } \mu }}}\left( {{\boldsymbol{D}} - {{\boldsymbol{A}}_{k + 1}} + {{{{\boldsymbol{Y}}_k}} \mathord{\left/ {\vphantom {{{{\boldsymbol{Y}}_k}} \mu }} \right. } \mu }} \right) $; $ {{\boldsymbol{Y}}_{k + 1}} = {{\boldsymbol{Y}}_k} + \mu \left( {{\boldsymbol{D - }}{{\boldsymbol{A}}_{k + 1}}{\boldsymbol{ - }}{{\boldsymbol{E}}_{k + 1}}} \right) $; $k = k + 1$; End while 2 所提RPCA优化模型求解流程
输入:观测矩阵$ {\boldsymbol{D}} $,参数$ \lambda $; 输出:低秩矩阵$ {{\boldsymbol{A}}_{k + 1}} $,稀疏矩阵$ {F}{\left( {\boldsymbol{E}} \right)_{k + 1}} $; 初始化:$ {{\boldsymbol{Y}}_0} $=0; $ {\mu _0} \gt 0 $, $k = 0$, $\delta = 1{\rm{e}} - 6$, ${\mathrm{iter}} = 1000$; While ${\left\| {{\boldsymbol{D}} - {{\boldsymbol{A}}_{k + 1}} - {{\boldsymbol{E}}_{k + 1}}} \right\|_{\rm F}} > \delta $ or $k < {\mathrm{iter}}$ do $ \left( {{\boldsymbol{U,\varSigma ,V}}} \right) = {\rm{svd}}\left( {{\boldsymbol{D}} - {{\boldsymbol{E}}_k} + {{{{\boldsymbol{Y}}_k}} \mathord{\left/ {\vphantom {{{{\boldsymbol{Y}}_k}} \mu }} \right. } \mu }} \right) $; $ {{\boldsymbol{A}}_{k + 1}} = {\boldsymbol{U}}{S_{{1 \mathord{\left/ {\vphantom {1 \mu }} \right. } \mu }}}\left[ {\boldsymbol{\varXi }} \right]{{\boldsymbol{V}}^{\mathrm{H}}} $; $ {F}{\left( {\boldsymbol{E}} \right)_{k + 1}} = {S_{{\kappa \mathord{\left/ {\vphantom {\kappa \mu }} \right. } \mu }}}\left( {{F}\left( {{\boldsymbol{D}} - {{\boldsymbol{A}}_{k + 1}} + {{{{\boldsymbol{Y}}_k}} \mathord{\left/ {\vphantom {{{{\boldsymbol{Y}}_k}} \mu }} \right. } \mu }} \right)} \right) $; ${{\boldsymbol{E}}_{k + 1}} = {{F}^{ - 1}}\left( {{F}{{\left( {\boldsymbol{E}} \right)}_{k + 1}}} \right)$; $ {{\boldsymbol{Y}}_{k + 1}} = {{\boldsymbol{Y}}_k} + \mu \left( {{\boldsymbol{D}} - {{\boldsymbol{A}}_{k + 1}} - {{\boldsymbol{E}}_{k + 1}}} \right) $; $k = k + 1$; End while 表 1 多通道SAR系统参数
参数 值 载频(GHz) 8.85 带宽(MHz) 40 采样频率(MHz) 60 脉冲重复频率(Hz) 1000 通道间距(m) 0.559 平台速度(m/s) 115 通道数目(个) 3 表 2 不同处理方法通道相关性
方法 相关性 文献[31]算法 0.80 数据重构 0.90 本文算法 0.94 表 3 不同目标检测算法耗时对比
方法 耗时(s) DPCA 0.007 RPCA 4.970 VSAR-RPCA 5.300 -
[1] HUANG Penghui, XIA Xianggen, WANG Lingyu, et al. Imaging and relocation for extended ground moving targets in multichannel SAR-GMTI systems[J]. IEEE Transactions on Geoscience and Remote Sensing, 2022, 60: 5214024. doi: 10.1109/TGRS.2021.3106906. [2] HE Xiongpeng, LIAO Guisheng, ZHU Shengqi, et al. Range-ambiguous clutter suppression for the SAR-GMTI system based on extended azimuth phase coding[J]. IEEE Transactions on Geoscience and Remote Sensing, 2020, 58(11): 8147–8162. doi: 10.1109/TGRS.2020.2987630. [3] HE Xiongpeng, YU Yue, GUO Yifan, et al. Ground moving target detection with nonuniform subpulse coding in SAR system[J]. IEEE Transactions on Geoscience and Remote Sensing, 2023, 61: 5200718. doi: 10.1109/TGRS.2022.3231914. [4] YANG Jungang, HUANG Xiaotao, JIN Tian, et al. New approach for SAR imaging of ground moving targets based on a keystone transform[J]. IEEE Geoscience and Remote Sensing Letters, 2011, 8(4): 829–833. doi: 10.1109/LGRS.2011.2118739. [5] XU Ruipeng, QIU Xiaolan, HU Donghui, et al. A novel single-channel SAR-GMTI method based on defocusing shifted difference[C]. The 2nd International Conference on Signal Processing Systems, Dalian, China, 2010: V3-83–V3-86. doi: 10.1109/ICSPS.2010.5555697. [6] LÜ Gaohuan, WANG Junfeng, and LIU Xingzhao. Ground moving target indication in SAR images by symmetric defocusing[J]. IEEE Geoscience and Remote Sensing Letters, 2013, 10(2): 241–245. doi: 10.1109/LGRS.2012.2200232. [7] HUANG Yan, LIAO Guisheng, XU Jingwei, et al. GMTI and parameter estimation via time-Doppler chirp-varying approach for single-channel airborne SAR system[J]. IEEE Transactions on Geoscience and Remote Sensing, 2017, 55(8): 4367–4383. doi: 10.1109/TGRS.2017.2691742. [8] WU Jiaye, LIU Peng, ZHAO Bingji, et al. Single channel SAR ground moving target detection algorithm based on subband interferometry[J]. IEEE Geoscience and Remote Sensing Letters, 2022, 19: 4503805. doi: 10.1109/LGRS.2022.3143874. [9] CHAPIN E and CHEN C W. Airborne along-track interferometry for GMTI[J]. IEEE Aerospace and Electronic Systems Magazine, 2009, 24(5): 13–18. doi: 10.1109/MAES.2009.5109948. [10] LIGHTSTONE L, FAUBERT D, and REMPEL G. Multiple phase centre DPCA for airborne radar[C]. The 1991 IEEE National Radar Conference, Los Angeles, USA, 1991: 36–40. doi: 10.1109/NRC.1991.114720. [11] 郑明洁, 杨汝良. 一种改进的DPCA运动目标检测方法[J]. 电子学报, 2004, 32(9): 1429–1432. doi: 10.3321/j.issn:0372-2112.2004.09.005.ZHENG Mingjie and YANG Ruliang. An improved DPCA moving targets detecting algorithm[J]. Acta Electronica Sinica, 2004, 32(9): 1429–1432. doi: 10.3321/j.issn:0372-2112.2004.09.005. [12] ZHANG Sheng, ZHOU Fang, SUN Guangcai, et al. A new SAR–GMTI high-accuracy focusing and relocation method using instantaneous interferometry[J]. IEEE Transactions on Geoscience and Remote Sensing, 2016, 54(9): 5564–5577. doi: 10.1109/TGRS.2016.2569259. [13] SUWA K, YAMAMOTO K, TSUCHIDA M, et al. Image-based target detection and radial velocity estimation methods for multichannel SAR-GMTI[J]. IEEE Transactions on Geoscience and Remote Sensing, 2017, 55(3): 1325–1338. doi: 10.1109/TGRS.2016.2622712. [14] LIU Qinghua, WANG Junfeng, and LIU Xingzhao. SAR-GMTI based on ATI with normalized amplitude weighted phase difference[C]. 2021 IEEE International Geoscience and Remote Sensing Symposium, Brussels, Belgium, 2021: 3364–3367. doi: 10.1109/IGARSS47720.2021.9553369. [15] WANG Zhihao, WANG Yongliang, XING Mengdao, et al. A novel two-step scheme based on joint GO-DPCA and local STAP in image domain for multichannel SAR-GMTI[J]. IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2021, 14: 8259–8272. doi: 10.1109/JSTARS.2021.3104595. [16] ENDER J H G. Space-time adaptive processing for synthetic aperture radar[C]. IEE Colloquium on Space-Time Adaptive Processing, London, UK, 1998. doi: 10.1049/ic:19980244. [17] CERUTTI-MAORI D and SIKANETA I. Optimum GMTI processing for space-based SAR/GMTI systems-simulation results[C]. The 8th European Conference on Synthetic Aperture Radar, Aachen, Germany, 2010: 1–4. [18] CERUTTI-MAORI D and SIKANETA I. A generalization of DPCA processing for multichannel SAR/GMTI radars[J]. IEEE Transactions on Geoscience and Remote Sensing, 2013, 51(1): 560–572. doi: 10.1109/tgrs.2012.2201260. [19] SUO Zhiyong, LI Zhenfang, and BAO Zheng. Multi-channel SAR-GMTI method robust to coregistration error of SAR images[J]. IEEE Transactions on Aerospace and Electronic Systems, 2010, 46(4): 2035–2043. doi: 10.1109/TAES.2010.5595612. [20] CHEN Zhanye, ZHOU Shuwei, WANG Xing, et al. Single range data-based clutter suppression method for multichannel SAR[J]. IEEE Geoscience and Remote Sensing Letters, 2022, 19: 4012905. doi: 10.1109/LGRS.2021.3072411. [21] YAN He, WANG R, LI Fei, et al. Ground moving target extraction in a multichannel wide-area surveillance SAR/GMTI system via the relaxed PCP[J]. IEEE Geoscience and Remote Sensing Letters, 2013, 10(3): 617–621. doi: 10.1109/LGRS.2012.2216248. [22] YANG Dong, YANG Xi, LIAO Guisheng, et al. Strong clutter suppression via RPCA in multichannel SAR/GMTI system[J]. IEEE Geoscience and Remote Sensing Letters, 2015, 12(11): 2237–2241. doi: 10.1109/LGRS.2015.2461654. [23] 傅东宁, 廖桂生, 黄岩, 等. 基于多通道SAR系统的ATI-RPCA地面动目标指示方法[J]. 系统工程与电子技术, 2021, 43(1): 48–54. doi: 10.3969/j.issn.1001-506X.2021.01.07.FU Dongning, LIAO Guisheng, HUANG Yan, et al. ATI-RPCA GMTI method based on multi-channel SAR system[J]. Systems Engineering and Electronics, 2021, 43(1): 48–54. doi: 10.3969/j.issn.1001-506X.2021.01.07. [24] HUANG Yan, LIAO Guisheng, XU Jingwei, et al. GMTI and parameter estimation for MIMO SAR system via fast interferometry RPCA method[J]. IEEE Transactions on Geoscience and Remote Sensing, 2018, 56(3): 1774–1787. doi: 10.1109/TGRS.2017.2768243. [25] GUO Yifan, LIAO Guisheng, LI Jun, et al. A novel moving target detection method based on RPCA for SAR systems[J]. IEEE Transactions on Geoscience and Remote Sensing, 2020, 58(9): 6677–6690. doi: 10.1109/TGRS.2020.2978496. [26] SHU Yuxiang, LIAO Guisheng, and YANG Zhiwei. Robust radial velocity estimation of moving targets based on adaptive data reconstruction and subspace projection algorithm[J]. IEEE Geoscience and Remote Sensing Letters, 2014, 11(6): 1101–1105. doi: 10.1109/LGRS.2013.2287285. [27] HU Yuanlin, ZHANG Xiaoling, and ZHAN Xu. Multiple-overlaid-targets separation and high precision velocity estimation based on Bayesian criterion in VSAR system[C]. IEEE International Geoscience and Remote Sensing Symposium, Brussels, Belgium, 2021: 3145–3148. doi: 10.1109/IGARSS47720.2021.9553402. [28] YANG Yongpeng, YANG Zhenzhen, and LI Jianlin. Novel RPCA with nonconvex logarithm and truncated fraction norms for moving object detection[J]. Digital Signal Processing, 2023, 133: 103892. doi: 10.1016/j.dsp.2022.103892. [29] 张学攀, 廖桂生, 朱圣棋, 等. 距离频率ML方法无模糊估计动目标径向速度[J]. 西安电子科技大学学报: 自然科学版, 2014, 41(5): 42–47,104. doi: 10.3969/j.issn.1001-2400.2014.05.09.ZHANG Xuepan, LIAO Guisheng, ZHU Shengqi, et al. Unambiguous estimation of the radial velocity of a moving target by the range frequency maximum likelihood method[J]. Journal of Xidian University, 2014, 41(5): 42–47,104. doi: 10.3969/j.issn.1001-2400.2014.05.09. [30] CHEN Zhaoyan and WANG Tong. Unambiguous across-track velocity estimation of moving targets for multichannel synthetic aperture radar-ground moving target indication systems[J]. IET Signal Processing, 2014, 8(9): 950–957. doi: 10.1049/iet-spr.2013.0423. [31] WANG Chenghao, LIAO Guisheng, and ZHANG Qingjun. First spaceborne SAR-GMTI experimental results for the Chinese Gaofen-3 dual-channel SAR sensor[J]. Sensors, 2017, 17(11): 2683. doi: 10.3390/s17112683. -





 下载:
下载:



 下载:
下载:
