Analysis and Optimization of Signal Reconstruction Modeling Based on Mixed Analog-digital Subband Division
-
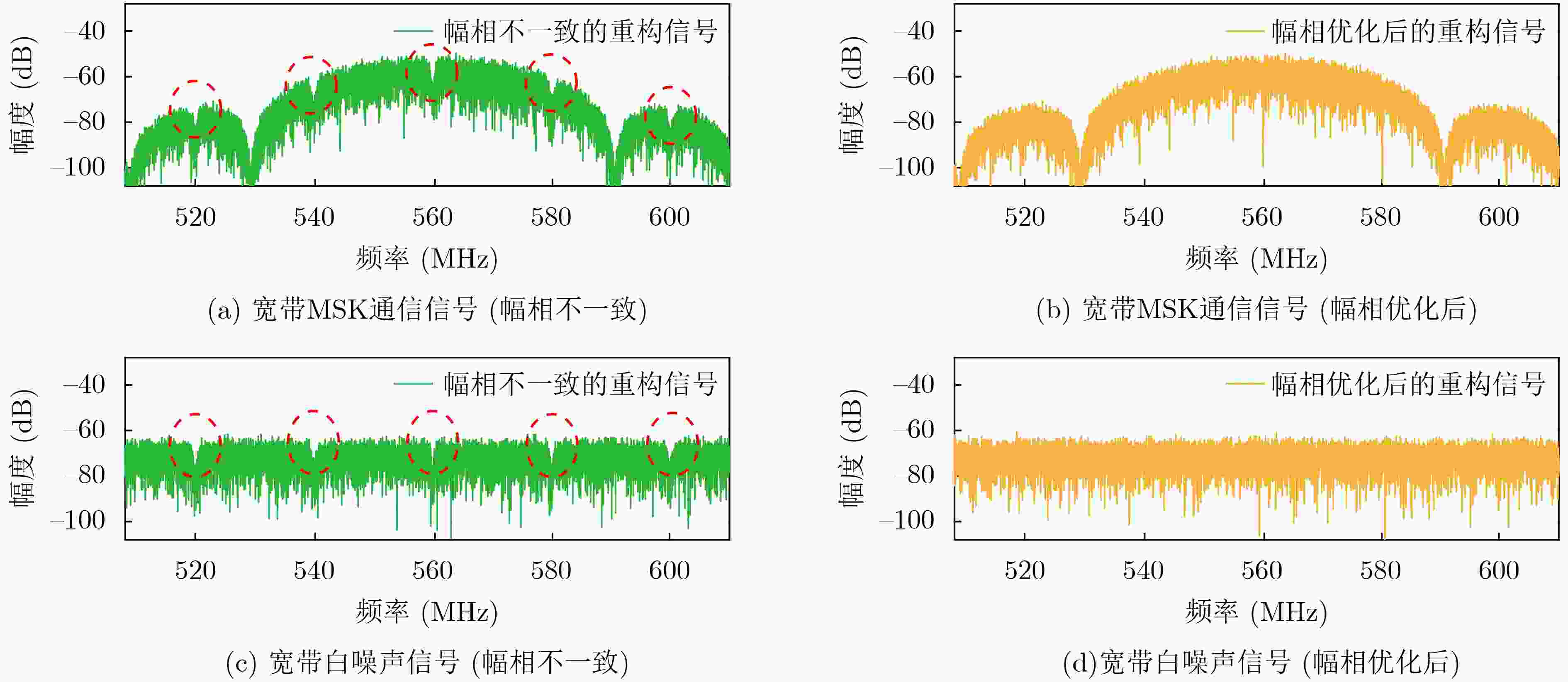
摘要: 为了应对宽带阻塞式干扰,通信干扰对消系统通常应用基于子带划分的干扰对消技术来提升宽带干扰抑制能力,同时为了兼顾通信性能,将子带信号重构恢复为通信信号,保障通信质量。该文采用模拟电路与数字处理相结合的方式,搭建了子带划分与信号重构框架,通过模拟滤波器实现第1级宽滤波,减小信号处理带宽,利用数字滤波器完成第2级窄滤波,进一步提高信号信噪比。针对通信信号在子带划分过程中,存在跨子带重构失真的问题,建立了子带划分与信号重构系统时频域模型,分析子带间幅相不一致对信号重构的影响。为了解决重构信号的幅相不一致问题,提出了相位校准方法与滤波器幅频优化方法,从幅度和相位两方面同时保证跨子带信号的近似无失真重构。仿真与实验结果表明,该文所设计的滤波器幅频响应具有良好的重构精度,并且解决了跨子带信号重构的相位失真问题,有效降低了重构通信信号的误码率。Abstract: In order to cope with broadband blocking interference, the integrated communication and interference cancellation system usually applies the interference cancellation technology based on subband division to improve the broadband interference suppression capability. To guarantee the communication performance meanwhile, it is required to reconstruct the subband signal to communication signal. In this paper, the combination of analog circuits and digital processing is used to build a subband division and signal reconstruction framework. The wide filtering in the first-stage is realized through analog filters to reduce the signal processing bandwidth. The narrow filtering in the second-stage is completed by digital filters to improve further the signal-to-noise ratio. This paper focusses on the problem of cross-subband reconstruction distortion in the communication signals’ subband division process. The time-frequency domain model of the subband division and signal reconstruction system is established to analyze the influence of the amplitude and phase inconsistency between subbands. To solve the inconsistency problem, the phase calibration method and the filter amplitude-frequency optimization method are proposed. The methods are to ensure the approximately distortion-free reconstruction of the cross-subband signal. Simulation and experiment results show that the amplitude-frequency response of the filter designed in the paper has good reconstruction accuracy. The phase calibration method solves the phase distortion problem of cross-subband signal reconstruction, and reduces effectively the bit error rate of reconstructed communication signals.
-
算法1 相位校准方法 目的:求解相位补偿量,配平相邻子带的相位 输入:校准信号 输出:相位补偿项 步骤: (1) 令 $ i = 1 $; (2) 子带 $ i $的中心频点为 $ {f_i} $,相邻子带 $ i + 1 $的中心频点为 $ {f_{i + 1}} $,产生一个频率为 $ {f_0} = ({f_i} + {f_{i + 1}})/2 $的校准信号,即校准信号既可以划分
到子带 $ i $,又可以划分到子带 $ i + 1 $;(3) 将校准信号注入到射频调理电路前端,进入子带划分与信号重构链路; (4) 在信号合成前,通过快速傅里叶变换得到子带 $ i $与 $ i + 1 $的校准信号频谱; (5) 抽取频谱中频率为 $ {f_0} $的复数分量,计算其相位 $ {\varphi _i} $, $ {\varphi _{i + 1}} $,作差后得到相邻子带的相位差 $ \Delta \varphi = {\varphi _{i + 1}} - {\varphi _i} $; (6) 计算出相位补偿量 $ {{\text{e}}^{{\text{j}}\Delta \varphi }} $配平 $ i + 1 $子带的相移; (7) 令 $ i = i + 1 $; (8) 重复步骤(2)~(7),直到 $ i = K \times M - 1 $(其中 $ K $为模拟子带数, $ M $为每个模拟子带中包含的数字子带数)。 表 1 预选滤波器组通带范围与中心频率(MHz)
预选滤波器组 预选滤波器① 预选滤波器② 预选滤波器③ 预选滤波器④ 起始频率 458 498 538 578 截止频率 502 542 582 622 中心频率 480 520 560 600 -
[1] CHENG C H and JAMES T S. An Introduction to Electronic Warfare; from the First Jamming to Machine Learning Techniques[M]. New York, USA: River Publishers, 2021: 97–101. [2] TEGLER J. Electronic warfare[J]. National Defense, 2022, 17(4): 107–109. [3] ZHANG Yongshun and JIA Xin. Adaptive interference suppression for DSSS communications based on compressive sensing[J]. International Journal of Communication Systems, 2018, 31(11): e3699. doi: 10.1002/dac.3699 [4] ZHANG Xiaolu, QUAN Houde, CUI Peizhang, et al. Simulation and analysis of frequency hopping communication jamming[J]. Journal of Physics:Conference Series, 2020, 1550: 052025. doi: 10.1088/1742-6596/1550/5/052025 [5] CHEN Xin, LI Xin, WU Weiyi, et al. Simulation and analysis of anti-jamming performance of frequency hopping communication system[J]. SPIE, 2020, 1606: 1160619. [6] GIUSTINIANO D, SCHALCH M, LIECHTI M, et al. Interference suppression in bandwidth hopping spread spectrum communications[C]. The 11th ACM Conference on Security & Privacy in Wireless and Mobile Networks, Stockholm, Sweden, 2018: 134–143. [7] 孟进, 何方敏, 李亚星, 等. L波段高速跳频数据链非合作干扰对消装置及方法[P]. 中国专利, 114513228B, 2022.MENG Jin, HE Fangmin, LI Yaxing, et al. L-band high-speed frequency hopping data link non cooperative interference cancellation device and method[P]. China Patent, 114513228B, 2022. [8] WU Renbiao, HUANG Jianyu, ZHANG Chuntian, et al. An adaptive receiver for constant modulus signal interference suppression in civil aviation air- ground communication[C]. 2007 Asia-Pacific Conference on Communications, Bangkok, Thailand, 2022: 487–489. [9] 孟进, 王青, 何方敏, 等. Ku和Ka双频段卫通地面站的多频点干扰对消装置及方法[P]. 中国专利, 113922889B, 2022.MENG Jin, WANG Qing, HE Fangmin, et al. Multi frequency interference cancellation device and method for Ku and Ka dual band SATCOM ground stations[P]. China Patent, 113922889B, 2022. [10] 孟进, 李亚星, 葛松虎, 等. 超短波电台干扰防护装置[P]. 中国专利, 113438035B, 2021.MENG Jin, LI Yaxing, GE Songhu, et al. Interference protection device for ultrashort wave radio[P]. China Patent, 113438035B, 2021. [11] ZHANG Yunshuo, HE Fangmin, LU Qiaran, et al. Wideband adaptive interference cancellation in spread-spectrum communication with subband bandwidth design[C]. 2022 IEEE 9th International Symposium on Microwave, Antenna, Propagation and EMC Technologies for Wireless Communications, Chengdu, China, 2022: 347–351. [12] YANG Xiaopeng, LI Shuai, LIU Quanhua, et al. Robust wideband adaptive beamforming based on focusing transformation and steering vector compensation[J]. IEEE Antennas and Wireless Propagation Letters, 2020, 19(12): 2280–2284. doi: 10.1109/LAWP.2020.3029950 [13] CHEN Xinzhu, SHU Ting, YU K B, et al. Implementation of an adaptive wideband digital array radar processor using subbanding for enhanced jamming cancellation[J]. IEEE Transactions on Aerospace and Electronic Systems, 2021, 57(2): 762–775. doi: 10.1109/TAES.2020.3042764 [14] ZHANG Wenxu, YAO Yushuang, ZHAO Zhongkai, et al. Design and FPGA implementation of a novel efficient FRM-based channelized receiver structure[J]. IEEE Access, 2019, 7: 114778–114787. doi: 10.1109/ACCESS.2019.2935562 [15] MURTHY C S and SRIDEVI K. Optimized DA-reconfigurable FIR filters for software defined radio channelizer applications[J]. Circuit World, 2021, 47(3): 252–261. doi: 10.1108/CW-11-2020-0332 [16] SU Yu. Design of a narrow transition band dynamic digital channelized receiver without merging adjacent sub-channels[C]. The 4th International Seminar on Computer Technology, Mechanical and Electrical Engineering, Chengdu, China, 2020: 042044. [17] TIAN Rujun. Investigation of radio signal reconnaissance based on intermediate frequency channel receiver[C]. The 4th International Conference on Computer, Civil Engineering and Mechatronics, Sanya, China, 2021: 378–386. [18] ZHANG Wenxu, ZHANG Chunguang, ZHAO Zhongkai, et al. Low-complexity channelizer based on FRM for passive radar multi-channel wideband receiver[J]. Circuits,Systems,and Signal Processing, 2020, 39(1): 420–438. doi: 10.1007/s00034-019-01192-0 [19] HRISTOVA V and CHERNEVA G. Coherent formation and receiving of frequency hopping spread spectrum signals[J]. SPIE, 2020: 1524: 115241K. [20] NGUYEN T Q and VAIDYANATHAN P P. Two-channel perfect-reconstruction FIR QMF structures which yield linear-phase analysis and synthesis filters[J]. IEEE Transactions on Acoustics,Speech,and Signal Processing, 1989, 37(5): 676–690. doi: 10.1109/29.17560 [21] 赵亮, 金梁, 刘双平, 等. 基于Dechirp和多相滤波结构的超宽带通信系统[J]. 电子与信息学报, 2011, 33(11): 2582–2587. doi: 10.3724/SP.J.1146.2011.00309ZHAO Liang, JIN Liang, LIU Shuangping, et al. Ultra wideband communication system based on Dechirp and polyphase filter structure[J]. Journal of Electronics&Information Technology, 2011, 33(11): 2582–2587. doi: 10.3724/SP.J.1146.2011.00309 [22] 张超, 马宏, 焦义文. 具有重构特性的原型滤波器的设计[J]. 雷达科学与技术, 2019, 17(3): 335–338,344. doi: 10.3969/j.issn.1672-2337.2019.03.016ZHANG Chao, MA Hong, and JIAO Yiwen. Design of a prototype filter with reconstruction characteristics[J]. Radar Science and Technology, 2019, 17(3): 335–338,344. doi: 10.3969/j.issn.1672-2337.2019.03.016 [23] LIU Hongying, YI Caixia, and YANG Zhiming. Design perfect reconstruction cosine-modulated filter banks via quadratically constrained quadratic programming and least squares optimization[J]. Signal Processing, 2017, 141: 199–203. doi: 10.1016/j.sigpro.2017.06.009 [24] SHAEEN K and ELIAS E. Prototype filter design approaches for near perfect reconstruction cosine modulated filter banks - a review[J]. Journal of Signal Processing Systems, 2015, 81(2): 183–195. doi: 10.1007/s11265-014-0929-5 [25] 蒋俊正, 江庆, 欧阳缮. 一种设计近似完全重构非均匀余弦调制滤波器组的新算法[J]. 电子与信息学报, 2016, 38(9): 2385–2390. doi: 10.11999/JEIT151260JIANG Junzheng, JIANG Qing, and OUYANG Shan. Novel method for designing near-perfect-reconstruction nonuniform cosine modulated filter banks[J]. Journal of Electronics&Information Technology, 2016, 38(9): 2385–2390. doi: 10.11999/JEIT151260 [26] CRUZ-ROLDAN F, MARTIN-MARTIN P, SAEZ-LANDETE J, et al. A fast windowing-based technique exploiting spline functions for designing modulated filter banks[J]. IEEE Transactions on Circuits and Systems I:Regular Papers, 2009, 56(1): 168–178. doi: 10.1109/TCSI.2008.925350 [27] 周芳, 水鹏朗. 基于相位调制的非均匀DFT调制滤波器组的构造算法[J]. 电子与信息学报, 2017, 39(9): 2169–2174. doi: 10.11999/JEIT170040ZHOU Fang and SHUI Penglang. Construction of nonuniform DFT modulated filter banks via phase modulation[J]. Journal of Electronics&Information Technology, 2017, 39(9): 2169–2174. doi: 10.11999/JEIT170040 [28] KHA H H, TUAN H D, and NGUYEN T Q. Efficient design of cosine-modulated filter banks via convex optimization[J]. IEEE Transactions on Signal Processing, 2009, 57(3): 966–976. doi: 10.1109/TSP.2008.2009268 [29] KANG A S and VIG R. Performance analysis of near perfect reconstruction filter bank in cognitive radio environment[J]. International Journal of Advanced Networking and Applications, 2016, 8(3): 3070–3083. [30] 焦义文, 马宏, 刘燕都, 等. 天线组阵频域合成方法最佳子带划分数分析[J]. 系统工程与电子技术, 2020, 42(10): 2156–2163. doi: 10.3969/j.issn.1001-506X.2020.10.02JIAO Yiwen, MA Hong, LIU Yandu, et al. Analysis on the optimal sub-band partition number in frequency domain combining for antenna arraying[J]. Systems Engineering and Electronics, 2020, 42(10): 2156–2163. doi: 10.3969/j.issn.1001-506X.2020.10.02 [31] 张文旭, 崔鑫磊, 陆满君. 一种基于MMF-FRM的低复杂度信道化接收机结构[J]. 电子学报, 2023, 51(3): 720–727. doi: 10.12263/DZXB.20210763ZHANG Wenxu, CUI Xinlei, and LU Manjun. A low complexity channelized receiver structure based on MMF-FRM[J]. Acta Electronica Sinica, 2023, 51(3): 720–727. doi: 10.12263/DZXB.20210763 [32] 赵廷刚, 王杰, 莘济豪, 等. 基于信道化架构的宽带I/Q不平衡校准技术[J]. 雷达科学与技术, 2023, 21(2): 199– 207, 214. doi: 10.3969/j.issn.1672-2337.2023.02.011ZHAO Tinggang, WANG Jie, SHEN Jihao, et al. Wideband I/Q imbalance calibration technique based on channelized architecture[J]. Radar Science and Technology, 2023, 21(2): 199– 207, 214. doi: 10.3969/j.issn.1672-2337.2023.02.011 [33] HENTHORN S, O’FARRELL T, ANBIYAEI M R, et al. Concurrent multiband direct rf sampling receivers[J]. IEEE Transactions on Wireless Communications, 2023, 22(1): 550–562. doi: 10.1109/TWC.2022.3196279 -





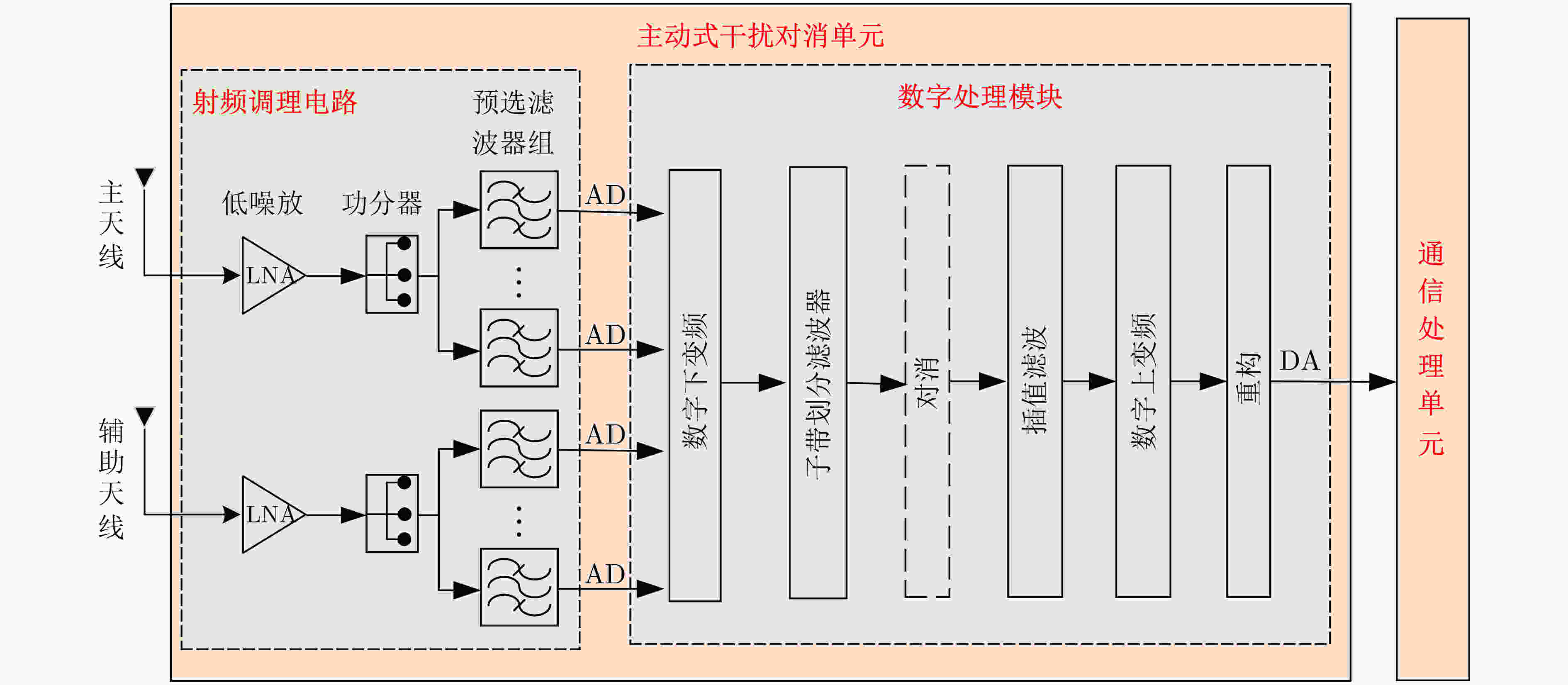
 下载:
下载:
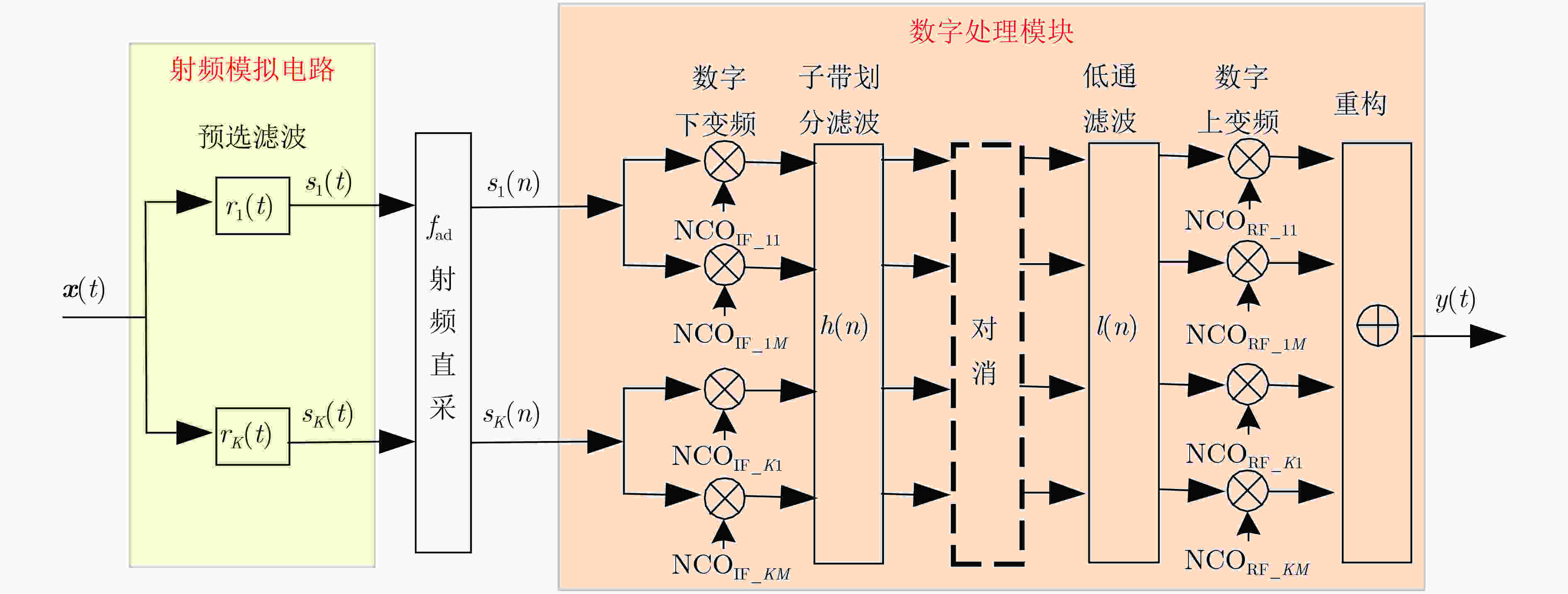

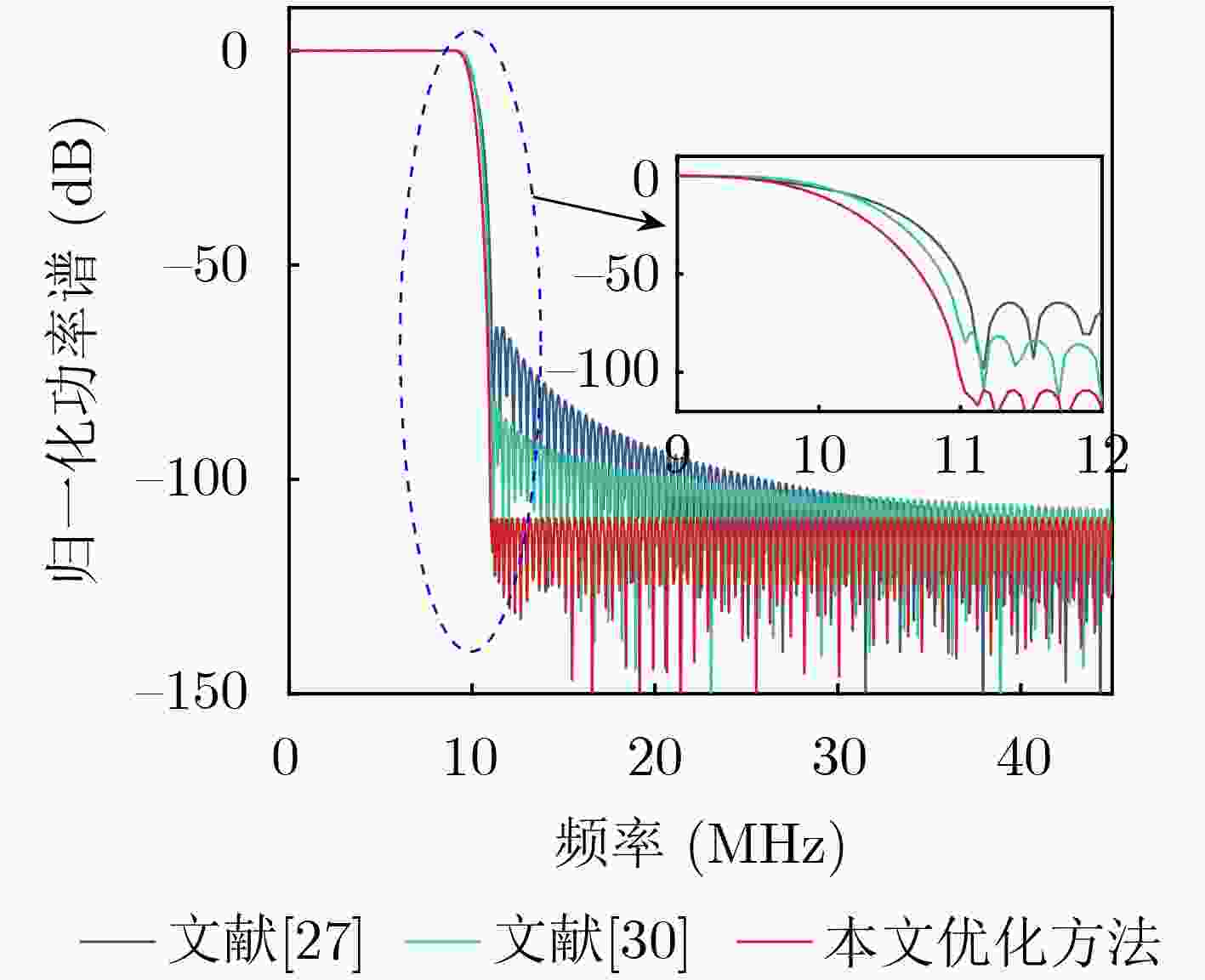
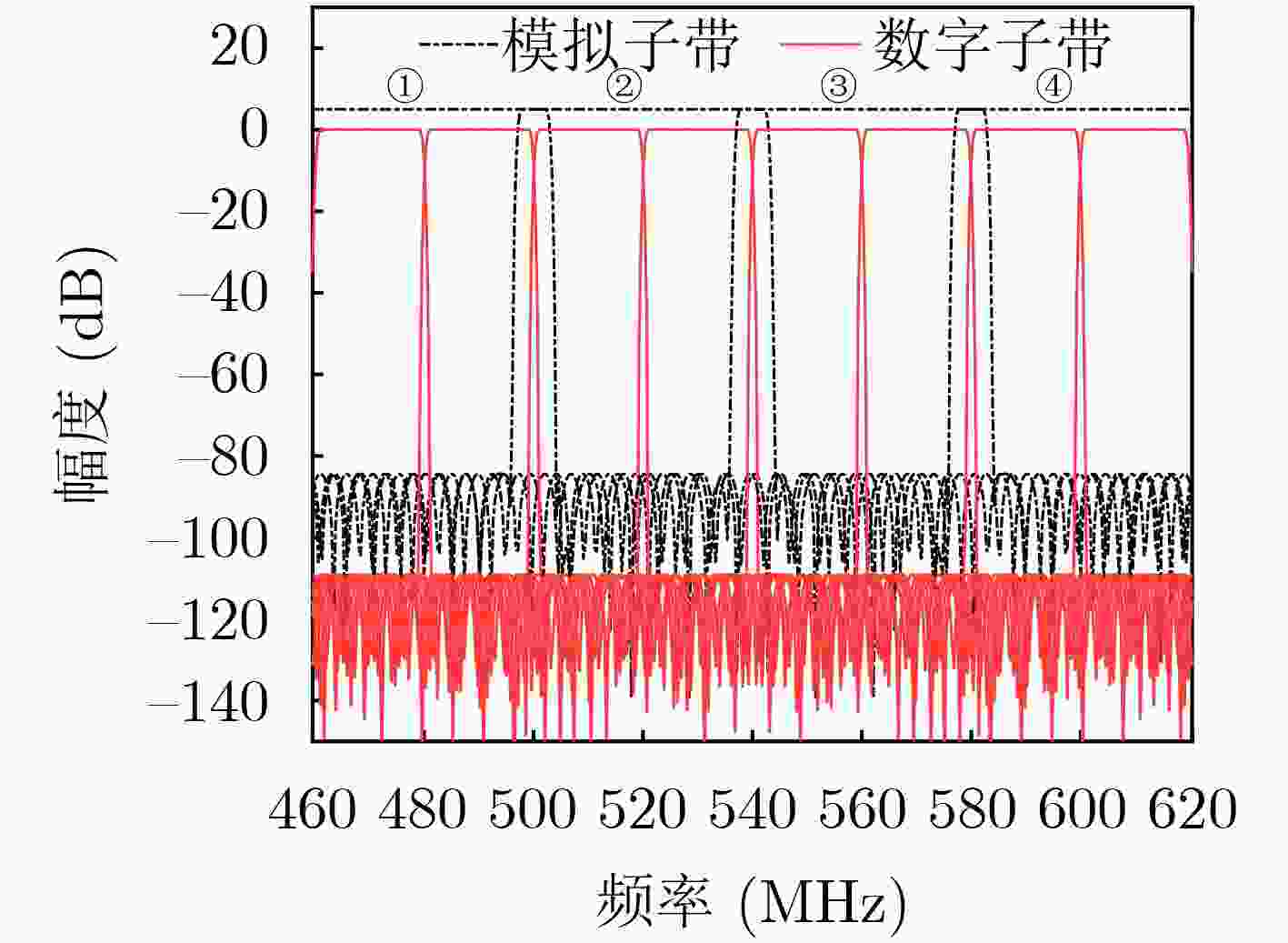

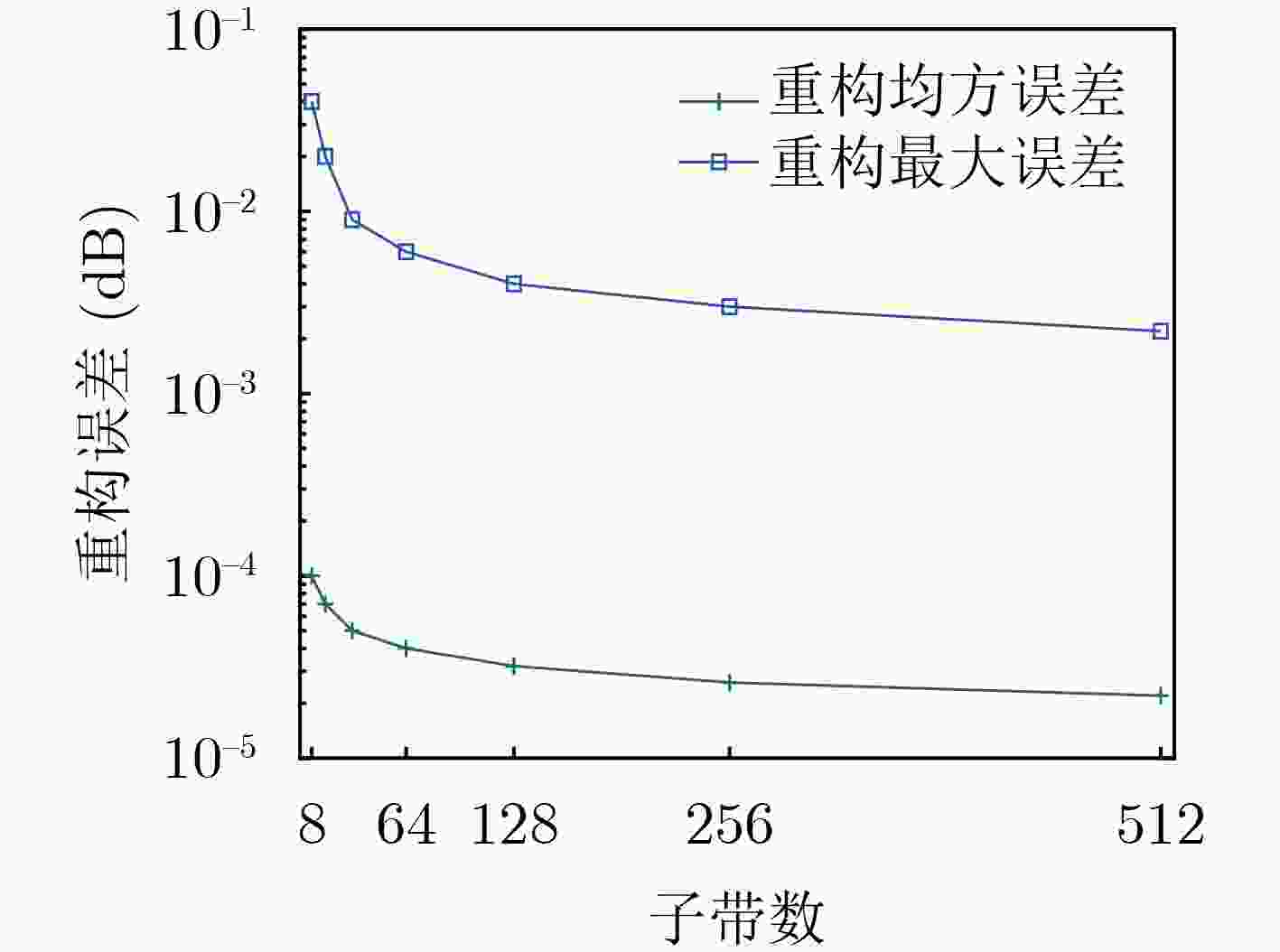
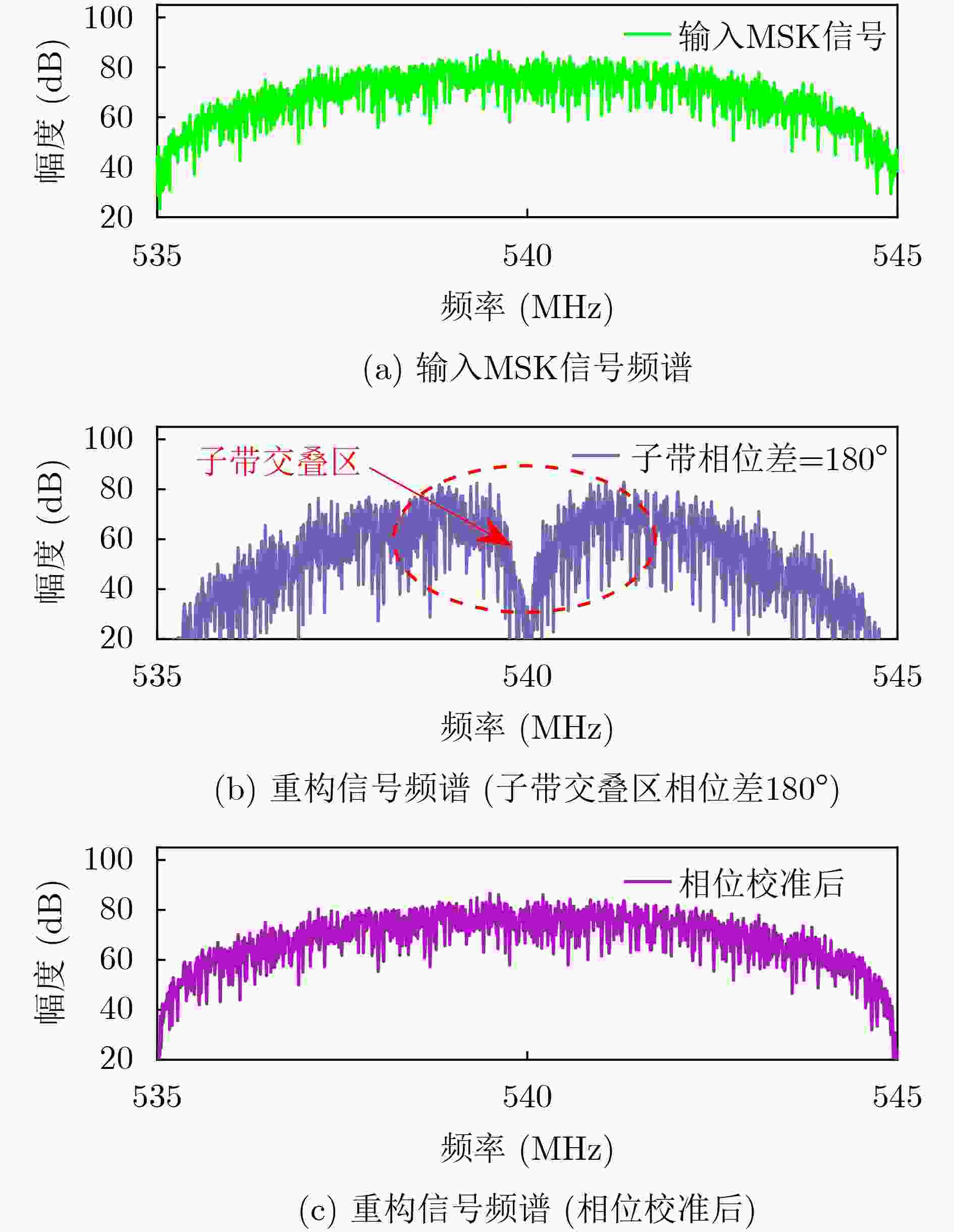
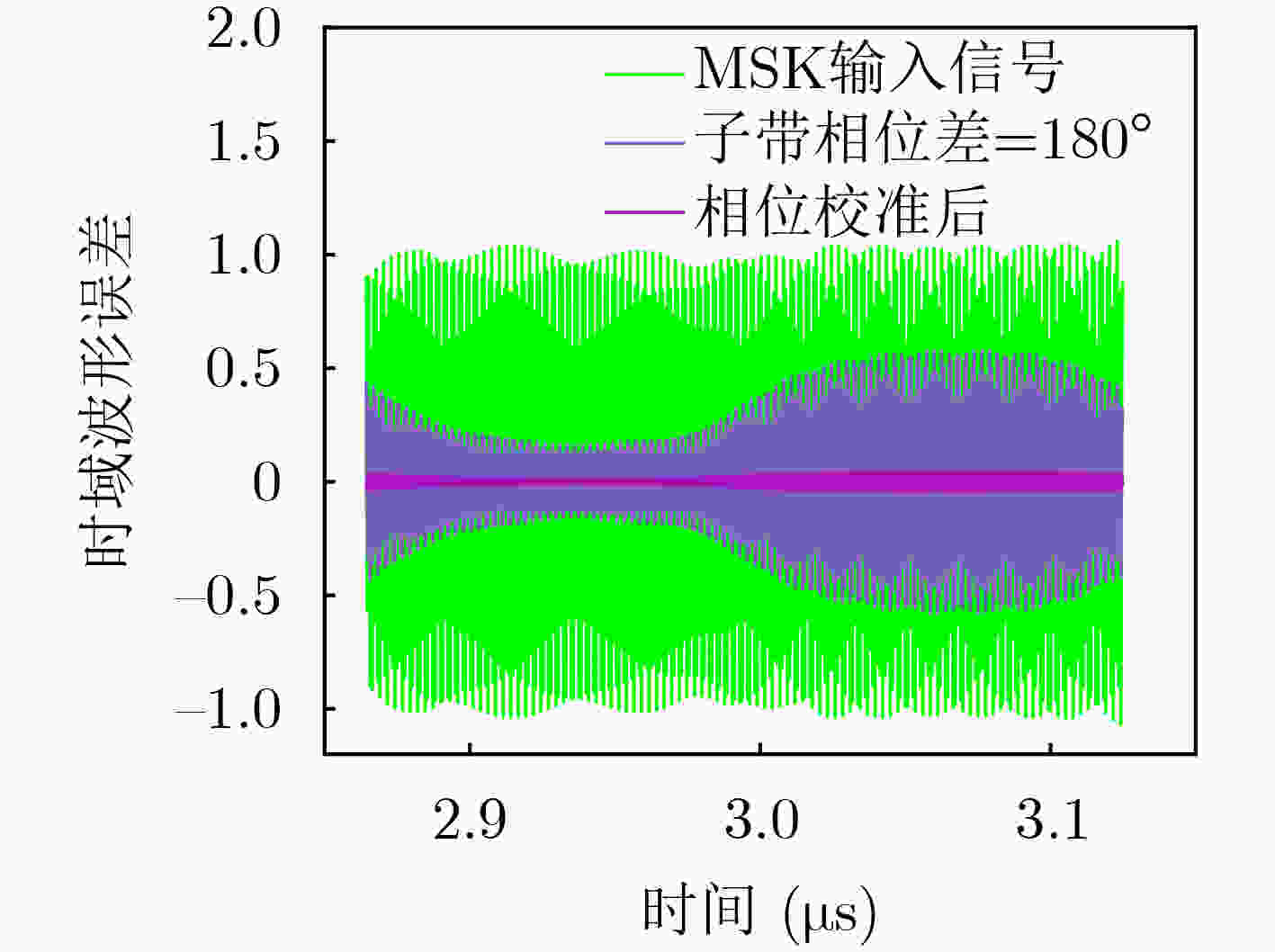
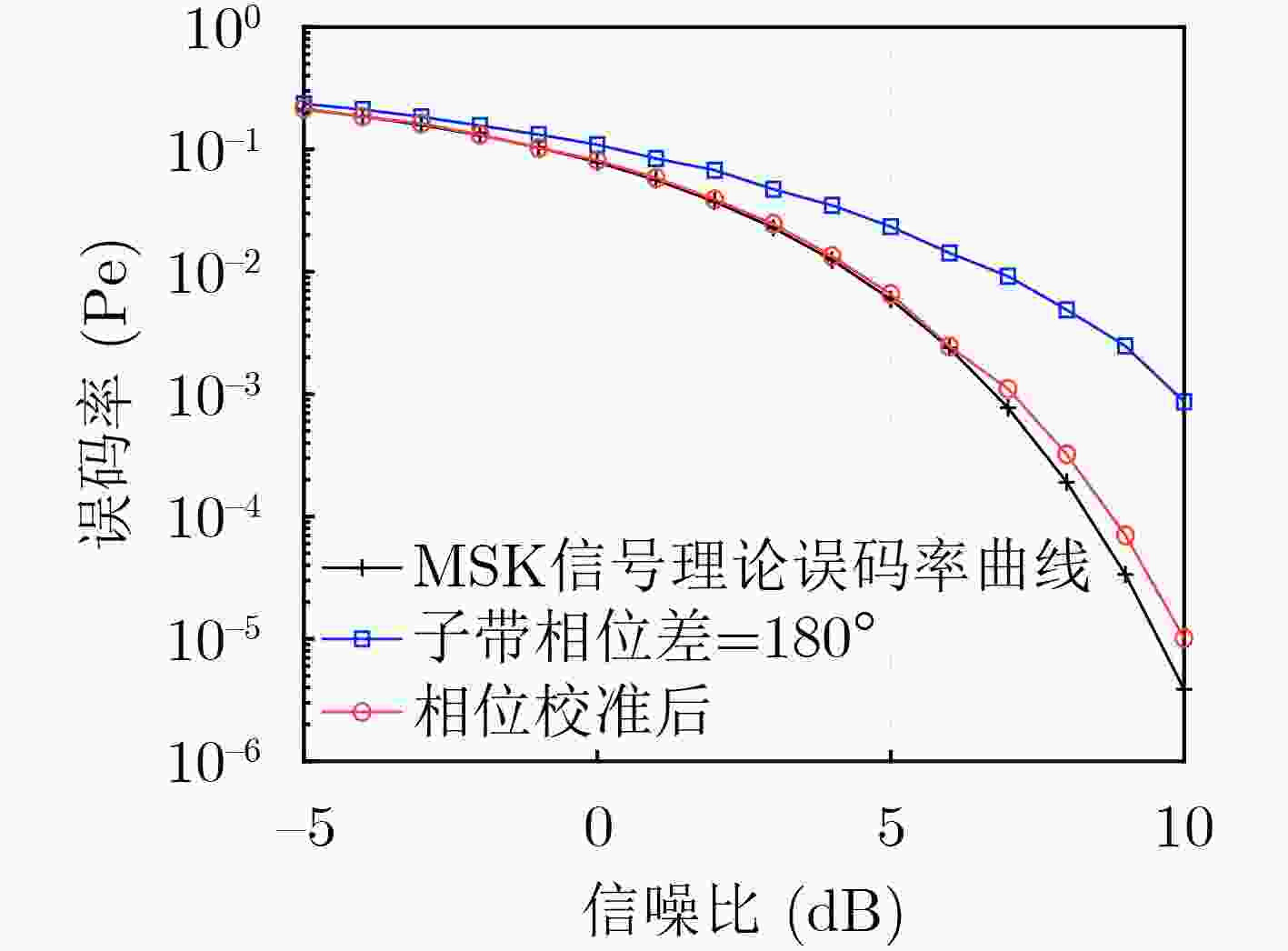



 下载:
下载:
