Survey of Differential Chaotic Communications: Signal Design and Performance Optimization
-
摘要: 作为一种低复杂度的非相干信息传输方案,差分混沌通信系统以其良好的抗多径衰落性能而受到广泛关注。近年来,研究者围绕着以差分混沌移位键控(DCSK)为代表的差分混沌通信开展了一系列富有成效的研究,逐渐发展了差分混沌通信的信号设计与性能优化方法。为此,该文从信号帧结构设计、正交多级信号设计、信号星座图设计和多载波信号设计4个层面详细综述了差分混沌通信信号设计的主要研究进展。此外,该文重点总结了面向差分混沌通信的噪声抑制辅助性能优化、索引调制辅助性能优化和混沌成形滤波辅助性能优化等方面的研究工作。Abstract: As a kind of low-complexity and non-coherent information transmission schemes, the differential chaotic communication system has been widely studied because of its good performance against multipath fading. Recently, a series of fruitful researches on Differential Chaos Shift Keying (DCSK) have been carried out, and the signal design and performance optimization for differential chaotic communications have also been developed. Therefore, the main research progresses in signal design of differential chaotic communications are surveyed in detail in the paper from the following four perspectives: design of signal frames, design of orthogonal multilevel signals, design of constellation diagrams and design of multicarrier signals. In addition, the research works on noise suppression aided performance optimization, index modulation aided performance optimization and chaotic shape forming aided performance optimization for differential chaotic communications are summarized in the paper.
-
表 1 差分混沌通信不同信号设计的对比
信号设计方法 优势 劣势 信号帧结构设计 实现简单,硬件成本较低 多径时延较大时将导致信号间干扰增大 正交多级信号设计 参考信号和信息承载信号在相同时隙中传输,提高系统的频谱效率 接收端需要Walsh码同步,误码率性能与同步质量密切相关 信号星座图设计 一个多元传输符号可传输多个信息比特,提高系统的数据传输速率 误码率性能随数据传输速率增大而恶化 多载波信号设计 多个信息承载信号子载波共享一个参考信号,提高系统的能量效率 峰均功率比较大,影响系统总体性能 表 2 不同噪声抑制方案的对比
噪声抑制方案 基本原理 优势 劣势 NR-DCSK [59]
SA-MC-DCSK [60]利用冗余信息进行平滑滤波降噪 系统实现相对简单,硬件成本较低 引入冗余信息,降低系统的能量效率和频谱效率 ND-MDCSK-DHT [62] 利用信息比特的再调制和迭代算法降噪 能够估计出噪声强度且降噪性能良好 系统复杂度高,高阶调制下的噪声抑制效果差 MC-DCSK-IR [63]
NS-MC-MDCSK [64]利用传输信号的似然信息实现迭代降噪 少量迭代就能获得良好的误码率性能 载波同步要求精度高,其质量直接影响系统性能 LRAM-MC-DCSK [65]
I-OFDM-DCSK [66]利用传输信号矩阵的低秩特性实现降噪 能够大幅度地提高系统的误码率性能 接收端需要额外的信号处理电路,系统复杂度高 -
[1] The Editors of Encyclopaedia Britannica. Chaos theory[EB/OL]. https://www.britannica.com/science/chaos-theory, 2021. [2] MATSUMOTO T. A chaotic attractor from Chua's circuit[J]. IEEE Transactions on Circuits and Systems, 1984, 31(12): 1055–1058. doi: 10.1109/TCS.1984.1085459 [3] PECORA L M and CARROLL T L. Synchronization in chaotic systems[J]. Physical Review Letters, 1990, 64(8): 821–824. doi: 10.1103/PhysRevLett.64.821 [4] CUOMO K M and OPPENHEIM A V. Circuit implementation of synchronized chaos with applications to communications[J]. Physical Review Letters, 1993, 71(1): 65–68. doi: 10.1103/PhysRevLett.71.65 [5] OPPENHEIM A V, WORNELL G W, ISABELLE S H, et al. Signal processing in the context of chaotic signals[C]. [Proceedings] ICASSP-92: 1992 IEEE International Conference on Acoustics, Speech, and Signal Processing, San Francisco, USA, 1992: 117–120. [6] SCHUSTER H G and JUST W. Deterministic Chaos: An Introduction[M]. Weinheim: John Wiley & Sons, 2005. [7] HALLE K S, WU C W, ITOH M, et al. Spread spectrum communication through modulation of chaos[J]. International Journal of Bifurcation and Chaos, 1993, 3(2): 469–477. doi: 10.1142/S0218127493000374 [8] DEDIEU H, KENNEDY M P, and HASLER M. Chaos shift keying: Modulation and demodulation of a chaotic carrier using self-synchronizing Chua's circuits[J]. IEEE Transactions on Circuits and Systems II:Analog and Digital Signal Processing, 1993, 40(10): 634–642. doi: 10.1109/82.246164 [9] KOCAREV L and PARLITZ U. General approach for chaotic synchronization with applications to communication[J]. Physical Review Letters, 1995, 74(25): 5028–5031. doi: 10.1103/PhysRevLett.74.5028 [10] KOLUMBÁN G, VIZVÁRI B, SCHWARZ W, et al. Differential chaos shift keying: A robust coding for chaos communication[C]. The 4th International Workshop on Nonlinear Dynamics of Electronic Systems, Seville, Spain, 1996: 87–92. [11] ABEL A and SCHWARZ W. Chaos communications-principles, schemes, and system analysis[J]. Proceedings of the IEEE, 2002, 90(5): 691–710. doi: 10.1109/JPROC.2002.1015002 [12] XIA Yongxiang, TSE C K, and LAU F C M. Performance of differential chaos-shift-keying digital communication systems over a multipath fading channel with delay spread[J]. IEEE Transactions on Circuits and Systems II:Express Briefs, 2004, 51(12): 680–684. doi: 10.1109/TCSII.2004.838329 [13] FANG Yi, HAN Guojun, CHEN Pingping, et al. A survey on DCSK-based communication systems and their application to UWB scenarios[J]. IEEE Communications Surveys & Tutorials, 2016, 18(3): 1804–1837. doi: 10.1109/COMST.2016.2547458 [14] KADDOUM G. Wireless chaos-based communication systems: A comprehensive survey[J]. IEEE Access, 2016, 4: 2621–2648. doi: 10.1109/ACCESS.2016.2572730 [15] KOLUMBÁN G, JÁKÓ Z, and KENNEDY M P. Enhanced versions of DCSK and FM-DCSK data transmission systems[C]. 1999 IEEE International Symposium on Circuits and Systems, Orlando, USA, 1999: 475–478. [16] LAU F C M, CHEONG K Y, and TSE C K. Permutation-based DCSK and multiple-access DCSK systems[J]. IEEE Transactions on Circuits and Systems I:Fundamental Theory and Applications, 2003, 50(6): 733–742. doi: 10.1109/TCSI.2003.812616 [17] YANG Hua and JIANG Guoping. High-efficiency differential-chaos-shift-keying scheme for chaos-based noncoherent communication[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2012, 59(5): 312–316. doi: 10.1109/TCSII.2012.2190859 [18] YANG Hua and JIANG Guoping. Reference-modulated DCSK: A novel chaotic communication scheme[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2013, 60(4): 232–236. doi: 10.1109/TCSII.2013.2251949 [19] YANG Hua, JIANG Guoping, and DUAN Junyi. Phase-separated DCSK: A simple delay-component-free solution for chaotic communications[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2014, 61(12): 967–971. doi: 10.1109/TCSII.2014.2356914 [20] KADDOUM G, SOUJERI E, ARCILA C, et al. I-DCSK: An improved noncoherent communication system architecture[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2015, 62(9): 901–905. doi: 10.1109/TCSII.2015.2435831 [21] KADDOUM G, SOUJERI E, and NIJSURE Y. Design of a short reference noncoherent chaos-based communication systems[J]. IEEE Transactions on Communications, 2016, 64(2): 680–689. doi: 10.1109/TCOMM.2015.2514089 [22] KIS G. Performance analysis of chaotic communications systems[D]. [Ph. D. dissertation], Budapest University of Technology and Economics, 2005. [23] XU Weikai, WANG Lin, and KOLUMBÁN G. A novel differential chaos shift keying modulation scheme[J]. International Journal of Bifurcation and Chaos, 2011, 21(3): 799–814. doi: 10.1142/S0218127411028829 [24] XU Weikai, WANG Lin, and KOLUMBÁN G. A new data rate adaption communications scheme for code-shifted differential chaos shift keying modulation[J]. International Journal of Bifurcation and Chaos, 2012, 22(8): 1250201. doi: 10.1142/S021812741250201X [25] KADDOUM G and GAGNON F. Design of a high-data-rate differential chaos-shift keying system[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2012, 59(7): 448–452. doi: 10.1109/TCSII.2012.2198982 [26] HUANG Tingting, WANG Lin, XU Weikai, et al. Multilevel code-shifted differential-chaos-shift-keying system[J]. IET Communications, 2016, 10(10): 1189–1195. doi: 10.1049/iet-com.2015.1109 [27] NGUYEN B. V, NGUYEN M. T, JUNG H, et al. Designing anti-jamming receivers for NR-DCSK systems utilizing ICA, WPD, and VMD methods[J]. IEEE Transactions on Circuits and Systems II: Express Briefs, 2019, 66(9): 1522–1526. doi: 10.1109/TCSII.2019.2891254 [28] YANG Hua, TANG W K S, CHEN Guanrong, et al. System design and performance analysis of orthogonal multi-level differential chaos shift keying modulation scheme[J]. IEEE Transactions on Circuits and Systems I:Regular Papers, 2016, 63(1): 146–156. doi: 10.1109/TCSI.2015.2510622 [29] GALIAS Z and MAGGIO G M. Quadrature chaos-shift keying: Theory and performance analysis[J]. IEEE Transactions on Circuits and Systems I:Fundamental Theory and Applications, 2001, 48(12): 1510–1519. doi: 10.1109/TCSI.2001.972858 [30] WANG Lin, CAI Guofa, and CHEN G R. Design and performance analysis of a new multiresolution M-ary differential chaos shift keying communication system[J]. IEEE Transactions on Wireless Communications, 2015, 14(9): 5197–5208. doi: 10.1109/TWC.2015.2434820 [31] CAI Guofa and SONG Yang. Closed-form BER expressions of M-ary DCSK systems over multipath Rayleigh fading channels[J]. IEEE Communications Letters, 2020, 24(6): 1192–1196. doi: 10.1109/LCOMM.2020.2981060 [32] CAI Guofa, FANG Yi, and HAN Guojun. Design of an adaptive multiresolution M-ary DCSK system[J]. IEEE Communications Letters, 2017, 21(1): 60–63. doi: 10.1109/LCOMM.2016.2614682 [33] CAI Guofa, FANG Yi, HAN Guojun, et al. A square-constellation-based M-ary DCSK communication system[J]. IEEE Access, 2016, 4: 6295–6303. doi: 10.1109/ACCESS.2016.2612224 [34] CAI Guofa, FANG Yi, HAN Guojun, et al. A new hierarchical M-ary DCSK communication system: Design and analysis[J]. IEEE Access, 2017, 5: 17414–17424. doi: 10.1109/ACCESS.2017.2740973 [35] CAI Xiangming, XU Weikai, ZHANG Rongfang, et al. A multilevel code shifted differential chaos shift keying system with M-ary modulation[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2019, 66(8): 1451–1455. doi: 10.1109/TCSII.2018.2886377 [36] ZHANG Haotian, ZHANG Lin, CHENG Julian, et al. An intelligent detection based on deep learning for multilevel code shifted differential chaos shift keying system with M-ary modulation[J]. IEEE Transactions on Cognitive Communications and Networking, 2022, 8(1): 155–169. doi: 10.1109/TCCN.2021.3111981 [37] CHEN Zuwei, ZHANG Lin, and WU Zhiqiang. High data rate discrete-cosine-spreading aided M-ary differential chaos shift keying scheme with low PAPR[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2020, 67(11): 2492–2496. doi: 10.1109/TCSII.2020.2980738 [38] MIAO Meiyuan, WANG Lin, CHEN Guanrong, et al. Design and analysis of replica piecewise M-ary DCSK scheme for power line communications with asynchronous impulsive noise[J]. IEEE Transactions on Circuits and Systems I:Regular Papers, 2020, 67(12): 5443–5453. doi: 10.1109/TCSI.2020.3023749 [39] KADDOUM G, RICHARDSON F D, and GAGNON F. Design and analysis of a multi-carrier differential chaos shift keying communication system[J]. IEEE Transactions on Communications, 2013, 61(8): 3281–3291. doi: 10.1109/TCOMM.2013.071013.130225 [40] YANG Hua, TANG W K S, CHEN Guanrong, et al. Multi-carrier chaos shift keying: System design and performance analysis[J]. IEEE Transactions on Circuits and Systems I:Regular Papers, 2017, 64(8): 2182–2194. doi: 10.1109/TCSI.2017.2685344 [41] LI Shuying, ZHAO Yaqin, and WU Zhilu. Design and analysis of an OFDM-based differential chaos shift keying communication system[J]. Journal of Communications, 2015, 10(3): 199–205. doi: 10.12720/jcm.10.3.199-205 [42] HUANG Tingting, WANG Lin, XU Weikai, et al. A multi-carrier M-ary differential chaos shift keying system with low PAPR[J]. IEEE Access, 2017, 5: 18793–18803. doi: 10.1109/ACCESS.2017.2752238 [43] LIU Zhaofeng, ZHANG Lin, and CHEN Zuwei. Low PAPR OFDM-based DCSK design with carrier interferometry spreading codes[J]. IEEE Communications Letters, 2018, 22(8): 1588–1591. doi: 10.1109/LCOMM.2018.2842196 [44] ZHANG Lin, ZHANG Haotian, JIANG Yuan, et al. Intelligent and reliable deep learning LSTM neural networks-based OFDM-DCSK demodulation design[J]. IEEE Transactions on Vehicular Technology, 2020, 69(12): 16163–16167. doi: 10.1109/TVT.2020.3022043 [45] CHEN Menglei, XU Weikai, WANG Deqing, et al. Design of a multi-carrier different chaos shift keying communication system in doubly selective fading channels[C]. The 23rd Asia-Pacific Conference on Communications, Perth, Australia, 2017: 1–6. [46] CHEN Menglei, XU Weikai, WANG Deqing, et al. Multi-carrier chaotic communication scheme for underwater acoustic communications[J]. IET Communications, 2019, 13(14): 2097–2105. doi: 10.1049/iet-com.2018.5524 [47] KADDOUM G and SHOKRANEH F. Analog network coding for multi-user multi-carrier differential chaos shift keying communication system[J]. IEEE Transactions on Wireless Communications, 2015, 14(3): 1492–1505. doi: 10.1109/TWC.2014.2367508 [48] KADDOUM G. Design and performance analysis of a multiuser OFDM based differential chaos shift keying communication system[J]. IEEE Transactions on Communications, 2016, 64(1): 249–260. doi: 10.1109/TCOMM.2015.2502259 [49] CHEN Zuwei, ZHANG Lin, WU Zhiqiang, et al. Reliable and efficient sparse code spreading aided MC-DCSK transceiver design for multiuser transmissions[J]. IEEE Transactions on Communications, 2021, 69(3): 1480–1495. doi: 10.1109/TCOMM.2020.3040422 [50] ZHANG Lin, CHEN Zuwei, RAO Weiwei, et al. Efficient and secure non-coherent OFDM-based overlapped chaotic chip position shift keying system: Design and performance analysis[J]. IEEE Transactions on Circuits and Systems I:Regular Papers, 2020, 67(1): 309–321. doi: 10.1109/TCSI.2019.2948789 [51] LIU Zhaofeng, ZHANG Lin, WU Zhiqiang, et al. A secure and robust frequency and time diversity aided OFDM-DCSK modulation system not requiring channel state information[J]. IEEE Transactions on Communications, 2020, 68(3): 1684–1697. doi: 10.1109/TCOMM.2019.2951512 [52] LIU Zhaofeng, ZHANG Lin, and WU Zhiqiang. Reliable and secure pre-coding OFDM-DCSK design for practical cognitive radio systems with the carrier frequency offset[J]. IEEE Transactions on Cognitive Communications and Networking, 2020, 6(1): 189–200. doi: 10.1109/TCCN.2019.2959332 [53] CAI Xiangming, HU Luyao, XU Weikai, et al. Design of an OFDM-based differential cyclic-shifted DCSK system for underwater acoustic communications[C]. The 26th IEEE Asia-Pacific Conference on Communications, Kuala Lumpur, Malaysia, 2021: 304–309. [54] KISEL A, DEDIEU H, and OGORZALEK M. Noise reduction methods for chaotic communication schemes[C]. 1999 IEEE International Symposium on Circuits and Systems, Orlando, USA, 1999: 446–449. [55] JAKO Z and KIS G. Application of noise reduction to chaotic communications: A case study[J]. IEEE Transactions on Circuits and Systems I:Fundamental Theory and Applications, 2000, 47(12): 1720–1725. doi: 10.1109/81.899924 [56] JAKO Z and KIS G. On the effectiveness of noise reduction methods in DCSK systems[C]. 2000 IEEE International Symposium on Circuits and Systems, Geneva, Switzerland, 2000: 437–440. [57] SCHWEIZER J and SCHIMMING T. Symbolic dynamics for processing chaotic signals. I. Noise reduction of chaotic sequences[J]. IEEE Transactions on Circuits and Systems I:Fundamental Theory and Applications, 2001, 48(11): 1269–1282. doi: 10.1109/81.964416 [58] WANG Zhenchao and ZHANG Shibing. An improved scheme for noise reduction to DCSK based on its retransmission characteristic[C]. The 4th International Conference on Computer Science & Education, Nanning, China, 2009: 366–370. [59] KADDOUM G and SOUJERI E. NR-DCSK: A noise reduction differential chaos shift keying system[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2016, 63(7): 648–652. doi: 10.1109/TCSII.2016.2532041 [60] YANG Hua, JIANG Guoping, TANG W K S, et al. Multi-carrier differential chaos shift keying system with subcarriers allocation for noise reduction[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2018, 65(11): 1733–1737. doi: 10.1109/TCSII.2017.2752754 [61] RAO Weiwei, ZHANG Lin, ZHANG Zhiping, et al. Noise-suppressing chaos generator to improve BER for DCSK systems[C]. 2017 IEEE International Conference on Communications, Paris, France, 2017: 1–6. [62] CAI Xiangming, XU Weikai, and WANG Lin. Design of divide-and-conquer noise decontamination strategy for M-ary DCSK: From remodulation to denoising[J]. IEEE Communications Letters, 2022, 26(7): 1673–1677. doi: 10.1109/LCOMM.2022.3173644 [63] CHEN Bingjun, ZHANG Lin, and WU Zhiqiang. General iterative receiver design for enhanced reliability in multi-carrier differential chaos shift keying systems[J]. IEEE Transactions on Communications, 2019, 67(11): 7824–7839. doi: 10.1109/TCOMM.2019.2939799 [64] CAI Xiangming, XU Weikai, WANG Lin, et al. Design and performance analysis of a robust multi-carrier M-ary DCSK system: A noise suppression perspective[J]. IEEE Transactions on Communications, 2022, 70(3): 1623–1637. doi: 10.1109/TCOMM.2022.3144276 [65] ZHANG Lin, ZHENG Jieheng, CHEN Bingjun, et al. Reliable low-rank approximation of matrices detection aided multicarrier DCSK receiver design[J]. IEEE Systems Journal, 2021, 15(4): 5277–5288. doi: 10.1109/JSYST.2020.3043420 [66] LIU Zhaofeng, SO H C, ZHANG Lin, et al. Robust receiver for OFDM-DCSK modulation via rank-1 modeling and ℓ p-minimization[J]. Signal Processing, 2021, 188: 108219. doi: 10.1016/j.sigpro.2021.108219 [67] BASAR E, WEN Miaowen, MESLEH R, et al. Index modulation techniques for next-generation wireless networks[J]. IEEE Access, 2017, 5: 16693–16746. doi: 10.1109/ACCESS.2017.2737528 [68] KADDOUM G, AHMED M F A, and NIJSURE Y. Code index modulation: A high data rate and energy efficient communication system[J]. IEEE Communications Letters, 2015, 19(2): 175–178. doi: 10.1109/LCOMM.2014.2385054 [69] XU Weikai, HUANG Tingting, and WANG Lin. Code-shifted differential chaos shift keying with code index modulation for high data rate transmission[J]. IEEE Transactions on Communications, 2017, 65(10): 4285–4294. doi: 10.1109/TCOMM.2017.2725261 [70] CAI Xiangming, XU Weikai, WANG Deqing, et al. An M-ary orthogonal multilevel differential chaos shift keying system with code index modulation[J]. IEEE Transactions on Communications, 2019, 67(7): 4835–4847. doi: 10.1109/TCOMM.2019.2908367 [71] CAI Guofa, FANG Yi, WEN Jinming, et al. Multi-carrier M-ary DCSK system with code index modulation: An efficient solution for chaotic communications[J]. IEEE Journal of Selected Topics in Signal Processing, 2019, 13(6): 1375–1386. doi: 10.1109/JSTSP.2019.2913944 [72] CAI Guofa, FANG Yi, CHEN Pingping, et al. Design of an MISO-SWIPT-aided code-index modulated multi-carrier M-DCSK system for e-health IoT[J]. IEEE Journal on Selected Areas in Communications, 2021, 39(2): 311–324. doi: 10.1109/JSAC.2020.3020603 [73] HERCEG M, KADDOUM G, VRANJEŠ D, et al. Permutation index DCSK modulation technique for secure multiuser high-data-rate communication systems[J]. IEEE Transactions on Vehicular Technology, 2018, 67(4): 2997–3011. doi: 10.1109/TVT.2017.2774108 [74] LIU Sujie, CHEN Pingping, and CHEN Guanrong. Differential permutation index DCSK modulation for chaotic communication system[J]. IEEE Communications Letters, 2021, 25(6): 2029–2033. doi: 10.1109/LCOMM.2021.3061675 [75] CAI Xiangming, XU Weikai, HONG Shaohua, et al. A trinal-code shifted differential chaos shift keying system[J]. IEEE Communications Letters, 2021, 25(3): 1000–1004. doi: 10.1109/LCOMM.2020.3041460 [76] CAI Xiangming, XU Weikai, HONG Shaohua, et al. Discrete W transform based index-keying M-ary DCSK for non-coherent chaotic communications[J]. IEEE Communications Letters, 2021, 25(9): 3104–3108. doi: 10.1109/LCOMM.2021.3095075 [77] CHENG Guixian, WANG Lin, XU Weikai, et al. Carrier index differential chaos shift keying modulation[J]. IEEE Transactions on Circuits and Systems Ⅱ:Express Briefs, 2017, 64(8): 907–911. doi: 10.1109/TCSII.2016.2613093 [78] CHENG Guixian, WANG Lin, CHEN Qiwang, et al. Design and performance analysis of generalised carrier index M-ary differential chaos shift keying modulation[J]. IET Communications, 2018, 12(11): 1324–1331. doi: 10.1049/iet-com.2017.0800 [79] YANG Hua, XU Siyuan, and JIANG Guoping. A high data rate solution for differential chaos shift keying based on carrier index modulation[J]. IEEE Transactions on Circuits and Systems II:Express Briefs, 2021, 68(4): 1487–1491. doi: 10.1109/TCSII.2020.3038163 [80] CAI Xiangming, XU Weikai, HONG Shaohua, et al. General carrier index aided dual-mode differential chaos shift keying with full mapping: Design and optimization[J]. IEEE Transactions on Vehicular Technology, 2021, 70(11): 11665–11677. doi: 10.1109/TVT.2021.3113315 [81] TAO Yiwei, FANG Yi, MA Huan, et al. Multi-carrier DCSK with hybrid index modulation: A new perspective on frequency-index-aided chaotic communication[J]. IEEE Transactions on Communications, 2022, 70(6): 3760–3773. doi: 10.1109/TCOMM.2022.3169214 [82] CAI Xiangming, XU Weikai, WANG Lin, et al. Multicarrier M-ary orthogonal chaotic vector shift keying with index modulation for high data rate transmission[J]. IEEE Transactions on Communications, 2020, 68(2): 974–986. doi: 10.1109/TCOMM.2019.2957431 [83] LIU Zhaofeng, ZHANG Lin, WU Zhiqiang, et al. Energy efficient parallel concatenated index modulation and M-ary PSK aided OFDM-DCSK communications with QoS consideration[J]. IEEE Transactions on Vehicular Technology, 2020, 69(9): 9469–9482. doi: 10.1109/TVT.2020.3002067 [84] MA Huan, FANG Yi, TAO Yiwei, et al. A novel differential chaos shift keying scheme with transmit diversity[J]. IEEE Communications Letters, 2022, 26(7): 1668–1672. doi: 10.1109/LCOMM.2022.3168151 [85] MIAO Meiyuan, WANG Lin, KATZ M, et al. Hybrid modulation scheme combining PPM with differential chaos shift keying modulation[J]. IEEE Wireless Communications Letters, 2019, 8(2): 340–343. doi: 10.1109/LWC.2018.2871137 [86] CAI Xiangming, XU Weikai, MIAO Meiyuan, et al. Design and performance analysis of a new M-ary differential chaos shift keying with index modulation[J]. IEEE Transactions on Wireless Communications, 2020, 19(2): 846–858. doi: 10.1109/TWC.2019.2949315 [87] CAI Xiangming, XU Weikai, HONG Shaohua, et al. Dual-mode differential chaos shift keying with index modulation[J]. IEEE Transactions on Communications, 2019, 67(9): 6099–6111. doi: 10.1109/TCOMM.2019.2918518 [88] CAI Xiangming, XU Weikai, LAU F C M, et al. Joint carrier-code index modulation aided M-ary differential chaos shift keying system[J]. IEEE Transactions on Vehicular Technology, 2020, 69(12): 15486–15499. doi: 10.1109/TVT.2020.3041927 [89] CAI Xiangming, XU Weikai, WANG Lin, et al. Towards high-data-rate noncoherent chaotic communication: A multiple-mode differential chaos shift keying system[J]. IEEE Transactions on Wireless Communications, 2021, 20(8): 4888–4901. doi: 10.1109/TWC.2021.3062836 [90] CAI Xiangming, XU Weikai, WANG Lin, et al. Joint energy and correlation detection assisted non-coherent OFDM-DCSK system for underwater acoustic communications[J]. IEEE Transactions on Communications, 2022, 70(6): 3742–3759. doi: 10.1109/TCOMM.2022.3169227 [91] CORRON N J, BLAKELY J N, and STAHL M T. A matched filter for chaos[J]. Chaos:An Interdisciplinary Journal of Nonlinear Science, 2010, 20(2): 023123. doi: 10.1063/1.3432557 [92] YAO Junliang, LI Chen, REN Haipeng, et al. Chaos-based wireless communication resisting multipath effects[J]. Physical Review E, 2017, 96(3): 032226. doi: 10.1103/PhysRevE.96.032226 [93] YAO Junliang, SUN Yuzhe, REN Haipeng, et al. Experimental wireless communication using chaotic baseband waveform[J]. IEEE Transactions on Vehicular Technology, 2019, 68(1): 578–591. doi: 10.1109/TVT.2018.2882422 [94] BAI Chao, REN Haipeng, ZHENG Wuyun, et al. Radio-wave communication with chaos[J]. IEEE Access, 2020, 8: 167019–167026. doi: 10.1109/ACCESS.2020.3022632 [95] REN Haipeng, YIN Huiping, BAI Chao, et al. Performance improvement of chaotic baseband wireless communication using echo state network[J]. IEEE Transactions on Communications, 2020, 68(10): 6525–6536. doi: 10.1109/TCOMM.2020.3007757 [96] REN Haipeng, YIN Huiping, ZHAO Honger, et al. Artificial intelligence enhances the performance of chaotic baseband wireless communication[J]. IET Communications, 2021, 15(11): 1467–1479. doi: 10.1049/cmu2.12162 [97] BAI Chao, REN Haipeng, and GREBOGI C. Experimental phase separation differential chaos shift keying wireless communication based on matched filter[J]. IEEE Access, 2019, 7: 25274–25287. doi: 10.1109/ACCESS.2019.2900729 [98] BAI Chao, REN Haipeng, and KOLUMBÁN G. Double-sub-stream M-ary differential chaos shift keying wireless communication system using chaotic shape-forming filter[J]. IEEE Transactions on Circuits and Systems I:Regular Papers, 2020, 67(10): 3574–3587. doi: 10.1109/TCSI.2020.2993674 [99] REN Haipeng, GUO Silong, BAI Chao, et al. Cross correction and chaotic shape-forming filter based quadrature multi-carrier differential chaos shift keying communication[J]. IEEE Transactions on Vehicular Technology, 2021, 70(12): 12675–12690. doi: 10.1109/TVT.2021.3119176 [100] BAI Chao, ZHAO Xiaohui, REN Haipeng, et al. Double-stream differential chaos shift keying communications exploiting chaotic shape forming filter and sequence mapping[J]. IEEE Transactions on Wireless Communications, 2022, 21(7): 4954–4972. doi: 10.1109/TWC.2021.3135043 -






 下载:
下载:
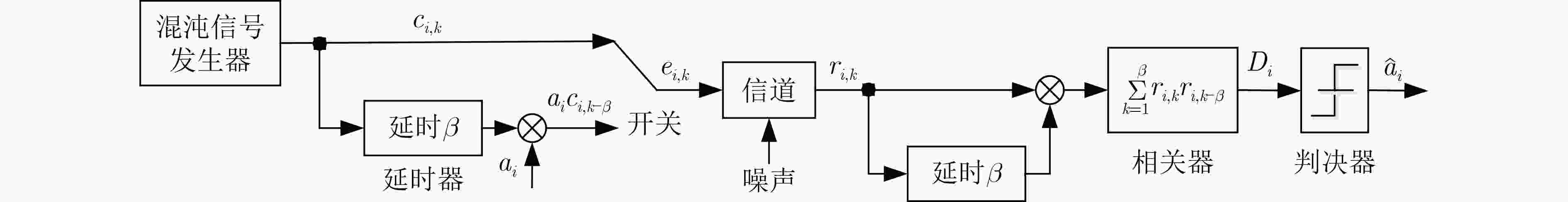

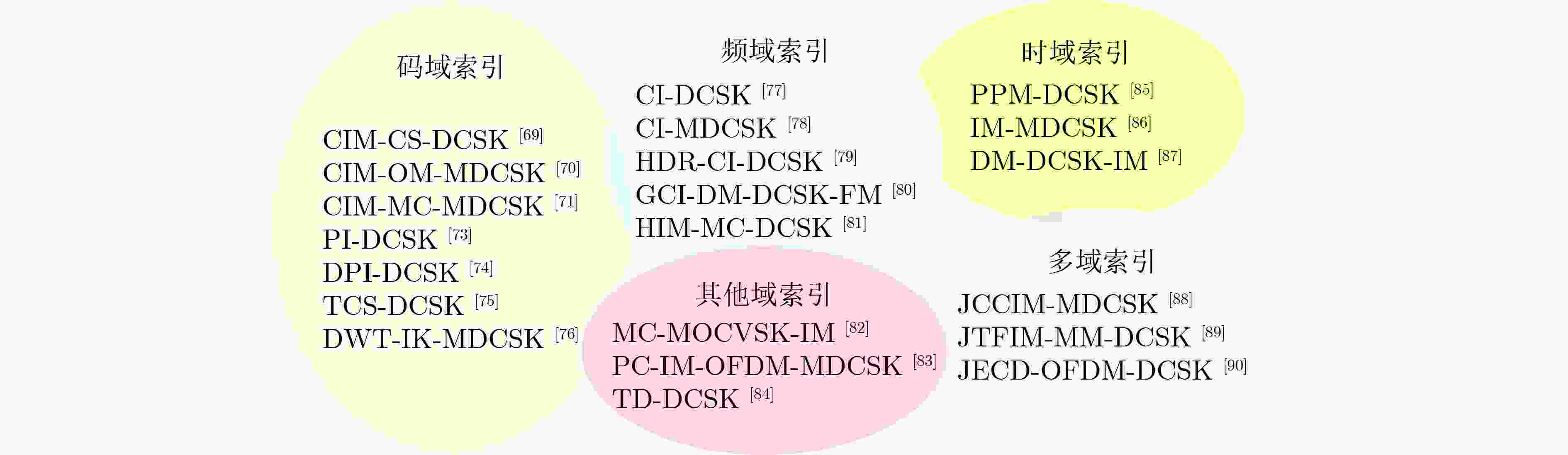


 下载:
下载:
