Research on Optimal Spectral Efficiency of Orthogonal Frequency Division Multiplexing Visible Light Communication-Radio FrequencyAggregation System Based on Finite Alphabet Inputs
-
摘要: 迄今为止，基于有限字母输入的正交频分复用(OFDM)可见光(VLC)和射频(RF)聚合系统的信息理论界仍然是未知的。基于这种情况，该文推导出OFDM VLC-RF聚合系统的无闭式形式的可达速率及其下界闭式表达式，并且研究了满足平均光功率和总电功率约束的基于可达速率及其下界的频谱效率(SE)最大化问题。该文利用互信息与最小均方误差(MMSE)之间的关系对速率偏导数进行了处理，提出双重注水算法解决谱效最大化问题。由于谱效的非闭式形式导致双重注水方案计算复杂度高，该文进一步研究具有闭式形式的谱效最大化问题，并使用内点法解决。仿真结果表明聚合系统相比于单个链路在通信性能上的优越性，以及基于可达速率下界的频谱效率可以作为基于可达速率的频谱效率的一个很好的低复杂度近似。Abstract: So far, the information theory of Orthogonal Frequency Division Multiplexing (OFDM) Visible Light Communication (VLC) and Radio Frequency (RF) aggregation systems based on finite alphabet inputs is still unknown. Based on this situation, the achievable rate of unclosed expression and the lower bound with closed expression of OFDM VLC-RF aggregation system are derived, and the maximization of Spectral Efficiency (SE) based on achievable rate and its lower bound satisfying the constraints of average optical power and total electric power is studied. In this paper, the relationship between mutual information and Minimum Mean Square Error (MMSE) is used to deal with the rate partial derivative, and the double Water-filling algorithm is proposed to solve the maximization problem of spectral efficiency. Because the non-closed form of spectral efficiency leads to high computational complexity of the double Water-filling algorithm, this paper further studies the problem of spectral efficiency maximization with closed form and uses the interior point method to solve it. The simulation results show that the aggregation system has the advantages in communication performance compared with a single link, and the spectral efficiency based on the lower bound of achievable rate can be used as a good low complexity approximation of the spectral efficiency based on achievable rate.
-
图 2 谱效
$ {\text{S}}{{\text{E}}_{\text{F}}} $ 和$ {\text{S}}{{\text{E}}_{\text{L}}} $ 随着总电功率约束$ {P_{{\text{total}}}} $ 的变化情况
图 4 谱效
$ {\text{S}}{{\text{E}}_{\text{F}}} $ 和$ {\text{S}}{{\text{E}}_{\text{L}}} $ 随着光功率约束$ {P_o} $ 的变化情况算法1 双重注水算法 输入：给定$ \beta \in \left[ {0,\hat \beta } \right] $，$ {\delta _\beta } $表示终止参数，$ {\delta _\beta } $是控制算法准确性的一个小的正常数。 (1)进入循环； (2) 令$ \beta = \left( {{1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}} \right)\left( {{\beta _{\min }} + {\beta _{\max }}} \right) $； (3) 基于式(32)，可以得到$ {p_{{\text{r}},k}} $；
(4) 找出最小$ \alpha $，$ \alpha \ge 0 $使其满足$\displaystyle\sum\nolimits_{i = 1}^{ {N_{\text{v} } }/2} { {p_{ {\text{v} },2i - 1} } } \le {\text{ } }{ { {4P_0^2}/\mathbb{E} }^2}\left\{ {\left| { {X_{ {\text{v} },2i - 1} } } \right|} \right\}$；(5) 基于式(31)，可以得到$ {p_{{\text{v}},2i - 1}} $；
(6) 如果$ \displaystyle\sum\nolimits_{i = 1}^{{N_{\text{v}}}/2} {p_{{\text{v}},2i - 1}^*} + \displaystyle\sum\nolimits_{k = 0}^{{N_{\text{r}}} - 1} {p_{{\text{r}},k}^*} \le {P_{{\text{total}}}} $，令$ {\beta _{\max }} \leftarrow \beta $；否则$ {\beta _{\min }} \leftarrow \beta $；(7)直到满足$ {\beta _{\max }} - {\beta _{\min }} \le {\delta _\beta } $，结束循环。 
下载: 导出CSV
表 1 仿真参数表
VLC链路 RF链路 仿真参数及符号 参数值 仿真参数及符号 参数值 子载波数$ {N_{\text{v}}} $ $ 64 $ 子载波数$ {N_{\text{r}}} $ $ 32 $ 接收器视场角$ \psi $ $ {90^ \circ } $ 断点距离$ {d_{\text{B}}} $ ${\text{10 m}}$ 各子载波的带宽$ {B_{\text{v}}} $ $ 1{\text{ MHz}} $ RF链路距离$ {d_{2,k}} $ ${\text{5 m}}$ 半功率角$ {\theta _{{1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}}} $ ${60^\circ }$ 各子载波的带宽$ {B_{\text{r}}} $ ${\text{1}}{\text{.25 MHz}}$ 房间反射率$ \rho $ $ 0.8 $ 中心载波频率$ {f_{\text{c}}} $ ${\text{2}}{\text{.4 GHz}}$ 噪声功率谱密度$ \sigma _{\text{v}}^2 $ $1 \times {10^{ - 19}}{\text{ }}{{\text{A}}^{\text{2}}}{\text{/Hz}}$ 到达/离开角度$ {\psi _k} $ $ {45^ \circ } $ 调制方式 $ 4{\text{ - QAM}} $ 噪声功率谱密度$ \sigma _{\text{r}}^2 $ $- 57{\text{ } }{ { {\text{dBm} } } \mathord{\left/ {\vphantom { { {\text{dbm} } } { {\text{MHz} } } }} \right. } { {\text{MHz} } } }$
下载: 导出CSV
表 2
$ {\text{S}}{{\text{E}}_{\text{F}}} $ 和$ {\text{S}}{{\text{E}}_{\text{L}}} $ 计算时间的比较(s)$ {\text{S}}{{\text{E}}_{\text{F}}} $ $ {\text{S}}{{\text{E}}_{\text{L}}} $ 计算时间 123.263 44.64
下载: 导出CSV
-
[1] CHEN Shanzhi, REN Bin, GAO Qiubin, et al. Pattern division multiple access—a novel nonorthogonal multiple access for fifth-generation radio networks[J]. IEEE Transactions on Vehicular Technology, 2017, 66(4): 3185–3196. doi: 10.1109/TVT.2016.2596438 [2] SHAFI M, MOLISCH A F, SMITH P J, et al. 5G: A tutorial overview of standards, trials, challenges, deployment, and practice[J]. IEEE Journal on Selected Areas in Communications, 2017, 35(6): 1201–1221. doi: 10.1109/JSAC.2017.2692307 [3] MAEDER A, ALI A, BEDEKAR A, et al. A scalable and flexible radio access network architecture for fifth generation mobile networks[J]. IEEE Communications Magazine, 2016, 54(11): 16–23. doi: 10.1109/MCOM.2016.1600140CM [4] CHEN Jie, ZHANG Lin, LIANG Yingchang, et al. Resource allocation for wireless-powered IoT networks with short packet communication[J]. IEEE Transactions on Wireless Communications, 2019, 18(2): 1447–1461. doi: 10.1109/TWC.2019.2893335 [5] 王玉皞, 曹凡, 邓震宇, 等. 可见光通信中LED非线性补偿和带宽拓展技术[J]. 光电工程, 2020, 47(3): 190671. doi: 10.12086/oee.2020.190671WANG Yuhao, CAO Fan, DENG Zhenyu, et al. LED nonlinearity compensation and bandwidth expansion techniques in visible light communication[J]. Opto-Electronic Engineering, 2020, 47(3): 190671. doi: 10.12086/oee.2020.190671 [6] AL-ANSI M, ALJUNID S A, SOUROUR E, et al. Multi-RF and generalized single-RF combination models for spatial modulation and NOMA technologies[J]. IEEE Transactions on Vehicular Technology, 2021, 20(10): 6329–6342. doi: 10.1109/TVT.2022.3165026 [7] TANG Qi, LI Kunying, LIU Xingming, et al. Performance analysis of spectral efficiently adaptive modulation DFT-spread polar coordinate-based OFDM in hybrid fiber-visible laser light communication system[C]. The 2020 IEEE 20th International Conference on Communication Technology (ICCT), Nanning, China, 2020: 594–598. [8] MULYAWAN R, AVERLY R, SYAFALNI I, et al. Dynamic pilot allocation for channel estimation in high-mobility OFDM receiver[C]. 2021 International Conference on Electronics, Information, and Communication (ICEIC), Jeju, Korea (South), 2021: 1–4. [9] 程国兵, 肖丽霞, 肖悦, 等. 一种改进的OFDM/OQAM系统信道估计算法[J]. 电子与信息学报, 2012, 34(2): 427–432. doi: 10.3724/SP.J.1146.2011.00530CHENG Guobing, XIAO Lixia, XIAO Yue, et al. An improved channel estimation algorithm for OFDM/OQAM systems[J]. Journal of Electronics &Information Technology, 2012, 34(2): 427–432. doi: 10.3724/SP.J.1146.2011.00530 [10] MARDANIKORANI S, DENG Xiong, and LINNARTZ J P M G. Sub-carrier loading strategies for DCO-OFDM LED communication[J]. IEEE Transactions on Communications, 2020, 68(2): 1101–1117. doi: 10.1109/TCOMM.2019.2953612 [11] OPPENHEIM A V, WILLSKY A S, and NAWAB W S H. Signals and Systems[M]. 2nd ed. Upper Saddle River: Prentice Hall, 1996. [12] BAI Bo, XU Zhengyuan, and FAN Yangyu. Joint LED dimming and high capacity visible light communication by overlapping PPM[C]. The 19th Annual Wireless and Optical Communications Conference (WOCC 2010), Shanghai, China, 2010: 1–5. [13] WU Liang, ZHANG Zaichen, DANG Jian, et al. Adaptive modulation schemes for visible light communications[J]. Journal of Lightwave Technology, 2015, 33(1): 117–125. doi: 10.1109/JLT.2014.2374171 [14] KAHN J M and BARRY J R. Wireless infrared communications[J]. Proceedings of the IEEE, 1997, 85(2): 265–298. doi: 10.1109/5.554222 [15] JUNGNICKEL V, POHL V, NONNIG S, et al. A physical model of the wireless infrared communication channel[J]. IEEE Journal on Selected Areas in Communications, 2002, 20(3): 631–640. doi: 10.1109/49.995522 [16] KHALID A M, COSSU G, CORSINI R, et al. 1-Gb/s transmission over a phosphorescent white LED by using rate-adaptive discrete multitone modulation[J]. IEEE Photonics Journal, 2012, 4(5): 1465–1473. doi: 10.1109/JPHOT.2012.2210397 [17] WANG Yunlu, WU Xiping, and HAAS H. Load balancing game with shadowing effect for indoor hybrid LiFi/RF networks[J]. IEEE Transactions on Wireless Communications, 2017, 16(4): 2366–2378. doi: 10.1109/TWC.2017.2664821 [18] PERAHIA E and STACEY R. Next Generation Wireless LANs: 802.11n and 802.11ac[M]. 2nd ed. Cambridge: Cambridge University Press, 2013. [19] CHRYSTAL G. Algebra: An Elementary Text-book for the Higher Classes of Secondary Schools and for Colleges[M]. 7th ed. New York: Chelsea Pub Co, 1999. [20] XIAO Chengshan, ZHENG Y R, and DING Zhi. Globally optimal linear precoders for finite alphabet signals over complex vector Gaussian channels[J]. IEEE Transactions on Signal Processing, 2011, 59(7): 3301–3314. doi: 10.1109/TSP.2011.2140112 [21] GUO Dongning, SHAMAI S, and VERDU S. Mutual information and minimum mean-square error in Gaussian channels[J]. IEEE Transactions on Information Theory, 2005, 51(4): 1261–1282. doi: 10.1109/TIT.2005.844072 [22] CAO Wei, DYTSO A, FAUß M, et al. Robust power allocation for parallel Gaussian channels with approximately Gaussian input distributions[J]. IEEE Transactions on Wireless Communications, 2020, 19(6): 3685–3699. doi: 10.1109/TWC.2020.2975784 [23] LOZANO A, TULINO A M, and VERDU S. Optimum power allocation for parallel Gaussian channels with arbitrary input distributions[J]. IEEE Transactions on Information Theory, 2006, 52(7): 3033–3051. doi: 10.1109/TIT.2006.876220 [24] ZENG Weiliang, XIAO Chengshan, and LU Jianhua. A low-complexity design of linear precoding for MIMO channels with finite-alphabet inputs[J]. IEEE Wireless Communications Letters, 2012, 1(1): 38–41. doi: 10.1109/WCL.2012.121411.110025 -
图(5) / 表(3)
计量
- 文章访问数: 617
- HTML全文浏览量: 119
- PDF下载量: 53
- 被引次数: 0
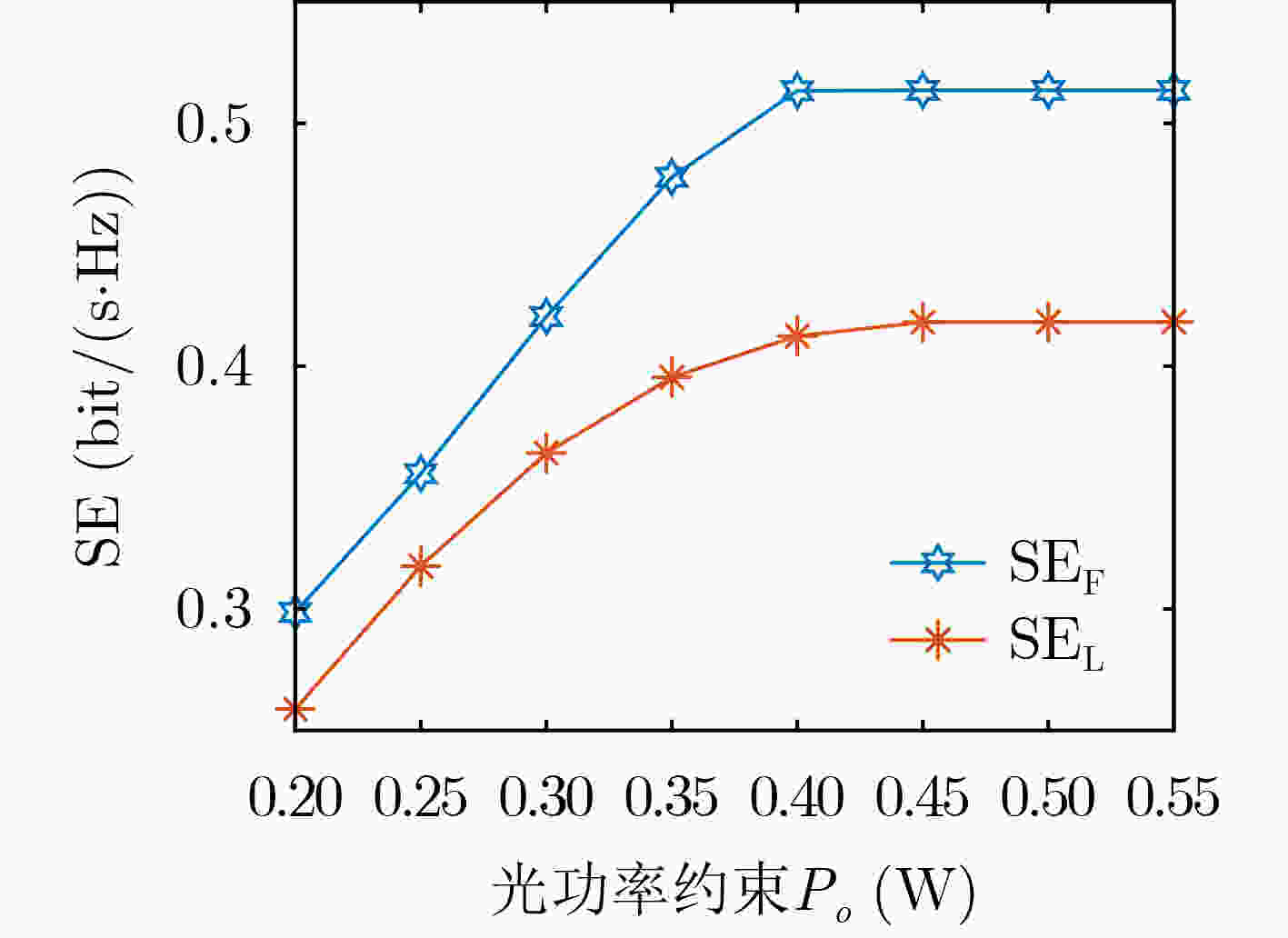
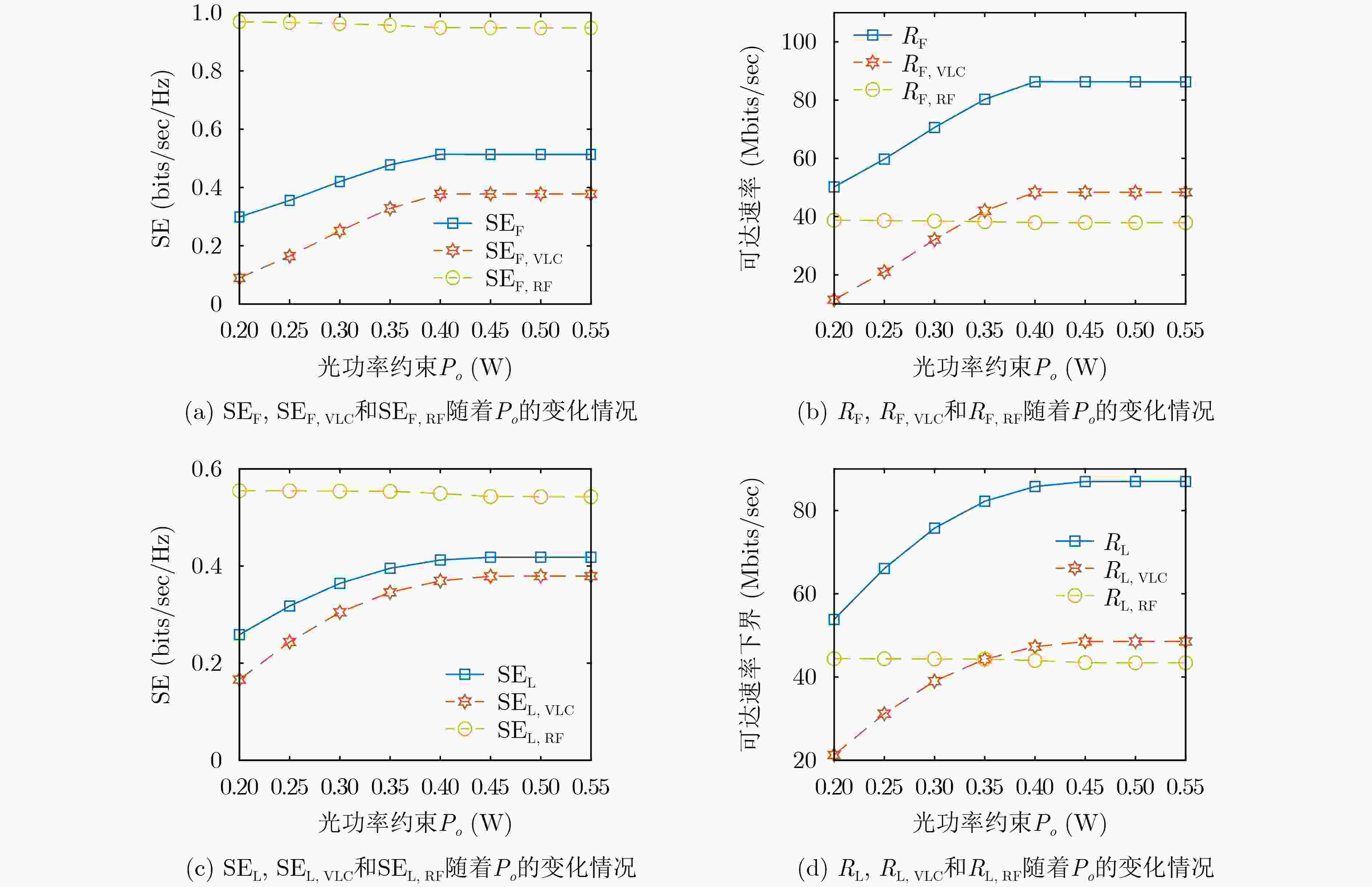
下载:
