Hardware Optimization of S-box of Camellia Algorithm Based on Polynomial Basis
-
摘要: 该文提出一种基于不可约多项式的Camellia算法S盒的代数表达式,并给出了该表达式8种不同的同构形式。然后,结合Camellia算法S盒的特点,基于理论证明给出一种基于多项式基的S盒优化方案,此方法省去了表达式中的部分线性操作。相对于同一种限定门的方案,在中芯国际(SMIC)130 nm工艺库中,该文方案减少了9.12%的电路面积;在SMIC 65 nm工艺库中,该文方案减少了8.31%的电路面积。最后,根据Camellia算法S盒设计中的计算冗余,给出了2类完全等价的有限域的表述形式,此等价形式将对Camellia算法S盒的优化产生积极影响。
-
关键词:
- 有限域 /
- 多项式基 /
- 正规基 /
- Camellia算法 /
- S盒
Abstract: An algebraic expression for the S-box of Camellia’s algorithm based on irreducible polynomials is proposed in this paper, and eight different isomorphic expressions are also given. Then combined with the characteristics of S-box, an optimization scheme based on polynomial basis is given by theoretical proof, in which some redundant linear operations are reduced. Compared with the same gate-limited scheme the circuit area is saved by 9.12% in the Semiconductor Manufacturing International Corporation (SMIC) 130 nm process library and by 8.31% in the SMIC 65 nm process library. Finally, according to the computational redundancy in the design of the S-box of Camellia algorithm, two completely equivalent representations on the finite field are given, which will have a positive impact on the optimization of the S-box of Camellia algorithm.-
Key words:
- Finite field /
- Polynomial basis /
- Normal basis /
- Camellia algorithm /
- S-box
-
表 1 正规基构造细节
不可约多项式 $ \alpha $ $\beta $ $p1(x) = {x^8} + {x^6} + {x^5} + {x^3} + 1$ 108 2 108 3 18 4 18 5 $p2(x) = {x^4} + x + 1$ 109 16 109 17 19 104 19 105 表 2 有限域构造参数
复合域 复合域 不可约多项式 ${\rm GF}({2^{(n/2)}})$ ${\rm GF}(2)[x]/{\rm{p}}2(x)$ ${\rm{p}}2(x)$ ${\rm GF}({({2^{(n/2)}})^2})$ ${\rm GF}({2^{(n/2)} })[x]/{\rm{p}}3(x)$ ${\rm{p}}3(x)={x}^{2}+Ax+B;A,B\in {\rm GF}({2}^{(n/2)})$ 表 3 GF((24)2)构造参数
复合域 复合域 不可约多项式 ${\rm GF}({2^4})$ ${\rm GF}(2)[x]/{\rm{p}}2(x)$ ${\rm{p}}2(x) = {x^4} + x + 1$ ${\rm GF}({({2^4})^2})$ ${\rm GF}({2^{(n/2)} })[x]/{\rm{p}}3(x)$ ${\rm{p}}3(x) = {x^2} + 0001x + 1001$ 表 4 两种域的参数
不可约多项式 $i(\alpha=\beta^i)$ $\alpha $ ${\beta }$ A B $ {\alpha ^4} + {\alpha ^3} = 1 $ 17, 34, 68, 136 106, 6, 20, 121 104, 105 1 2, 14, 9, 4 121, 106, 6, 20 16, 17 20, 121, 106, 6 4, 5 6, 20, 121, 106 2, 3 $ {\alpha ^4} + \alpha = 1 $ 119, 187, 221, 238 109, 18, 108, 19 104, 105 1 13, 14, 11, 9 18, 108, 19, 109 16, 17 108, 19, 109, 18 4, 5 19, 109, 18, 108 2, 3 $ {\alpha ^4} + {\alpha ^3} + {\alpha ^2} + \alpha = 1 $ 51, 102, 153, 204 21, 120, 7, 107 104, 105 1 14, 5, 9, 3 7, 21, 107, 120 16, 17 107, 7, 120, 21 4, 5 120, 107, 21, 7 2, 3 表 5 3种算法S盒代数安全性对比
算法 差分均匀度 分线性度 不动点个数 代数次数 代数项数 AES 4 112 0 7 (110,112,114,131,136,145,133,132) SM4 4 112 1 7 (124,139,124,126,123,128,130,134) Camellia 4 112 0 7 (127,132,126,135,129,133,129,126) 表 6 逻辑门参数(nm)
NOT AND NAND OR XOR XOR MUX NANDN SMIC130 0.67 1.33 1.00 1.33 2.33 2.33 2.67 1.33 SMIC65 0.75 1.50 1.00 1.50 2.25 2.25 2.25 1.50 表 7 逻辑门参数
-
[1] BOGDANOV A, KNUDSEN L R, LEANDER G, et al. PRESENT: An ultra-lightweight block cipher[C]. Cryptographic Hardware and Embedded Systems - CHES 2007, 9th International Workshop, Vienna, Austria, September 10–13, 2007, Proceedings, Berlin, Heidelberg, Germany, 2007. [2] WU Wenling and ZHANG Lei. LBlock: A lightweight block cipher[C]. The 9th International Conference on Applied Cryptography and Network Security, Berlin, Heidelberg, Germany, 2011. [3] CHEN Shiyao, FAN Yanhong, SUN Ling, et al. SAND: An AND-RX Feistel lightweight block cipher supporting S-box-based security evaluations[J]. Designs, Codes and Cryptography, 2021, 90(1): 155–198. doi: 10.1007/s10623-021-00970-9 [4] FENG Jingya and LI Lang. SCENERY: A lightweight block cipher based on Feistel structure[J]. Frontiers of Computer Science, 2022, 16(3): 163813. doi: 10.1007/S11704-020-0115-9 [5] GUO Ying, LI Lang, and LIU Botao. Shadow: A lightweight block cipher for IoT nodes[J]. IEEE Internet of Things Journal, 2021, 8(16): 13014–13023. doi: 10.1109/JIOT.2021.3064203 [6] AOKI K, ICHIKAWA T, KANDA M, et al. Camellia: A 128-bit block cipher suitable for multiple platforms-design and analysis[C]. 7th International Workshop on Selected Areas in Cryptography, Berlin Heidelberg, Germany, 2000. [7] SATOH A and MORIOKA S. Hardware-focused performance comparison for the standard block ciphers AES, camellia, and triple-DES[C]. 6th International Conference on Information Security, Berlin, Heidelberg, Germany, 2003: 252–266. [8] ZOU Jian, WEI Zihao, SUN Siwei, et al. Some efficient quantum circuit implementations of Camellia[J]. Quantum Information Processing, 2022, 21(4): 131. doi: 10.1007/S11128-022-03477-X [9] WEI Z, SUN S, HU L, et al. Scrutinizing the tower field implementation of the GF(2^8) inverter--with applications to AES, Camellia, and SM4[J]. Cryptology ePrint Archive, 2019. [10] CI C W, NAZIRI S Z M, ISMAIL R C, et al. Crypto-core design using camellia cipher[J]. Journal of Physics:Conference Series, 2021, 1755(1): 012019. doi: 10.1088/1742-6596/1755/1/012019 [11] RASHIDI B. Compact and efficient structure of 8-bit S-box for lightweight cryptography[J]. Integration, 2021, 76: 172–182. doi: 10.1016/j.vlsi.2020.10.009 [12] DAEMEN J and RIJMEN V. The Rijndael block cipher: AES proposal[C]. First Candidate Conference (AeS1), 1999: 343–348. [13] LIU Fen, JI Wen, HU Lei, et al. Analysis of the SMS4 block cipher[C]. Information Security and Privacy, 12th Australasian Conference, ACISP 2007, Townsville, Australia, July 2–4, 2007, Proceedings, Berlin, Heidelberg, Germany, 2007. [14] SATOH A, MORIOKA S, TAKANO K, et al. A compact Rijndael hardware architecture with S-box optimization[C]. 7th International Conference on the Theory and Application of Cryptology and Information Security, Berlin, Heidelberg, Germany, 2001: 239–254. [15] MAXIMOV A and EKDAHL P. New circuit minimization techniques for smaller and faster AES SBoxes[J]. IACR Transactions on Cryptographic Hardware and Embedded Systems, 2019: 91–125. doi: 10.46586/tches.v2019.i4.91-125 [16] MARTÍNEZ-HERRERA A F, MEX-PERERA J C, and NOLAZCO-FLORES J A. Some representations of the S-Box of Camellia in GF (((22)2)2)[C]. 11th International Conference on Cryptology and Network Security, Berlin, Heidelberg, Germany, 2012: 296–309. [17] SATOH A and MORIOKA S. Unified hardware architecture for 128-bit block ciphers AES and Camellia[C]. 5th International Workshop on Cryptographic Hardware and Embedded Systems, Berlin, Heidelberg, Germany, 2003: 304–318. [18] 魏子豪, 张英杰, 胡磊, 等. Camellia算法S盒的紧凑硬件实现[J]. 密码学报, 2021, 8(5): 844–855. doi: 10.13868/j.cnki.jcr.000481WEI Zihao, ZHANG Yingjie, HU Lei, et al. A compact hardware implementation of S-Box for Camellia[J]. Journal of Cryptologic Research, 2021, 8(5): 844–855. doi: 10.13868/j.cnki.jcr.000481 [19] BOYAR J, FIND M G, and PERALTA R. Small low-depth circuits for cryptographic applications[J]. Cryptography and Communications, 2019, 11(1): 109–127. doi: 10.1007/s12095-018-0296-3 [20] 刘建. 两类密码组件的实现优化方法研究[D]. [硕士论文], 战略支援部队信息工程大学, 2019.LIU Jian. Optimization on the implementation of two types of cryptographic components [D]. [Master dissertation], PLA Strategic Support Force Information Engineering University, 2019. -





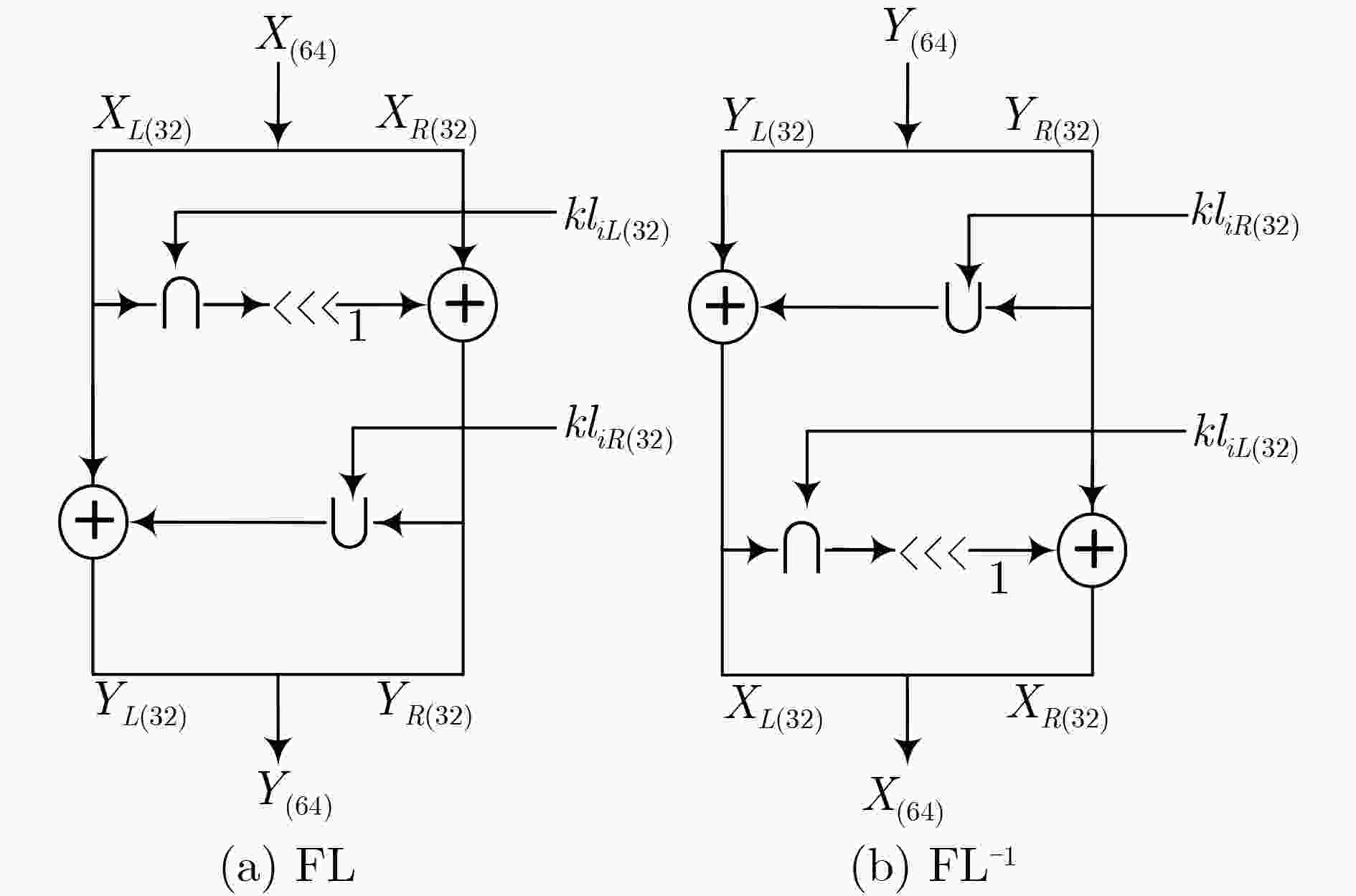


 下载:
下载:
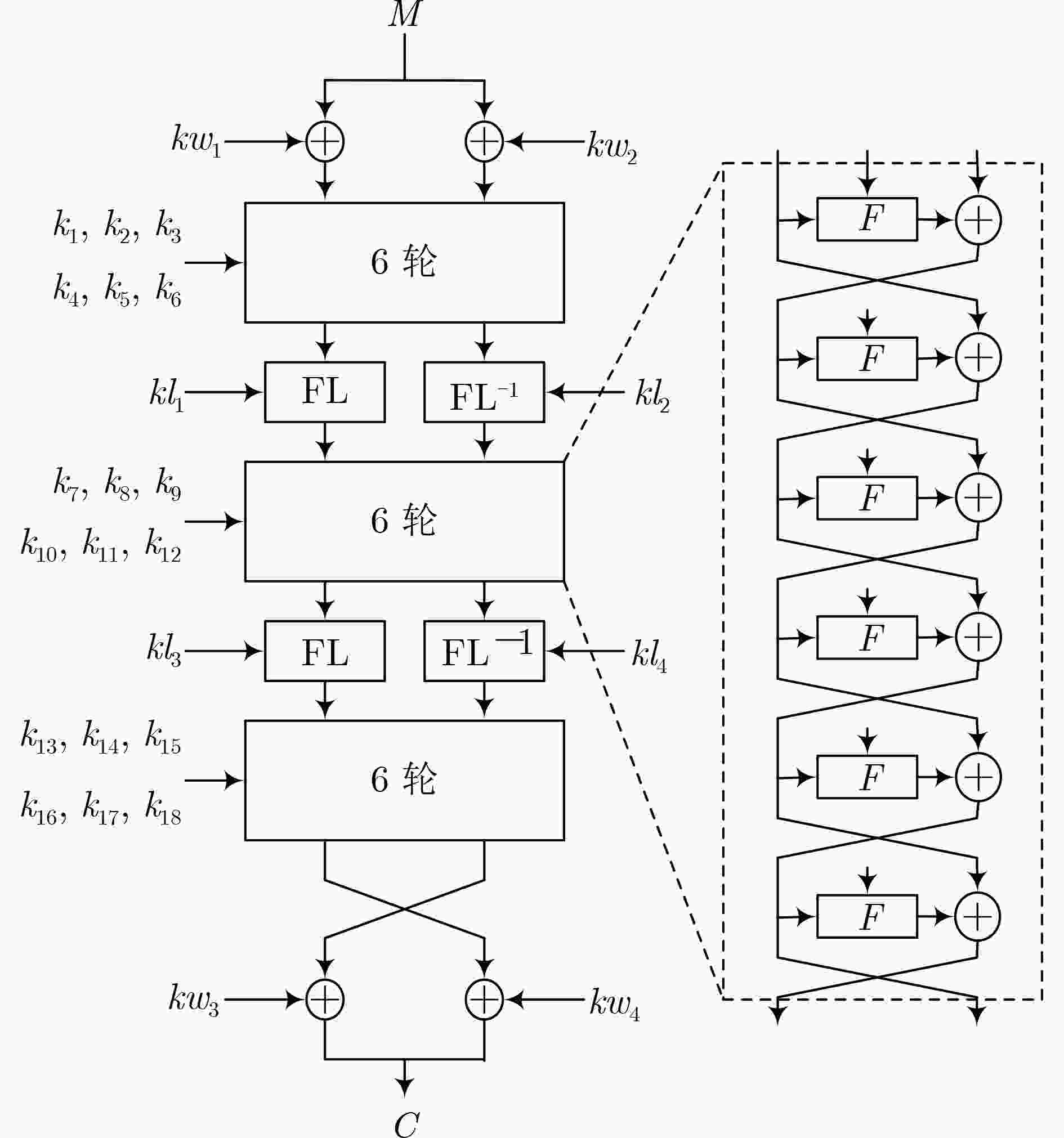
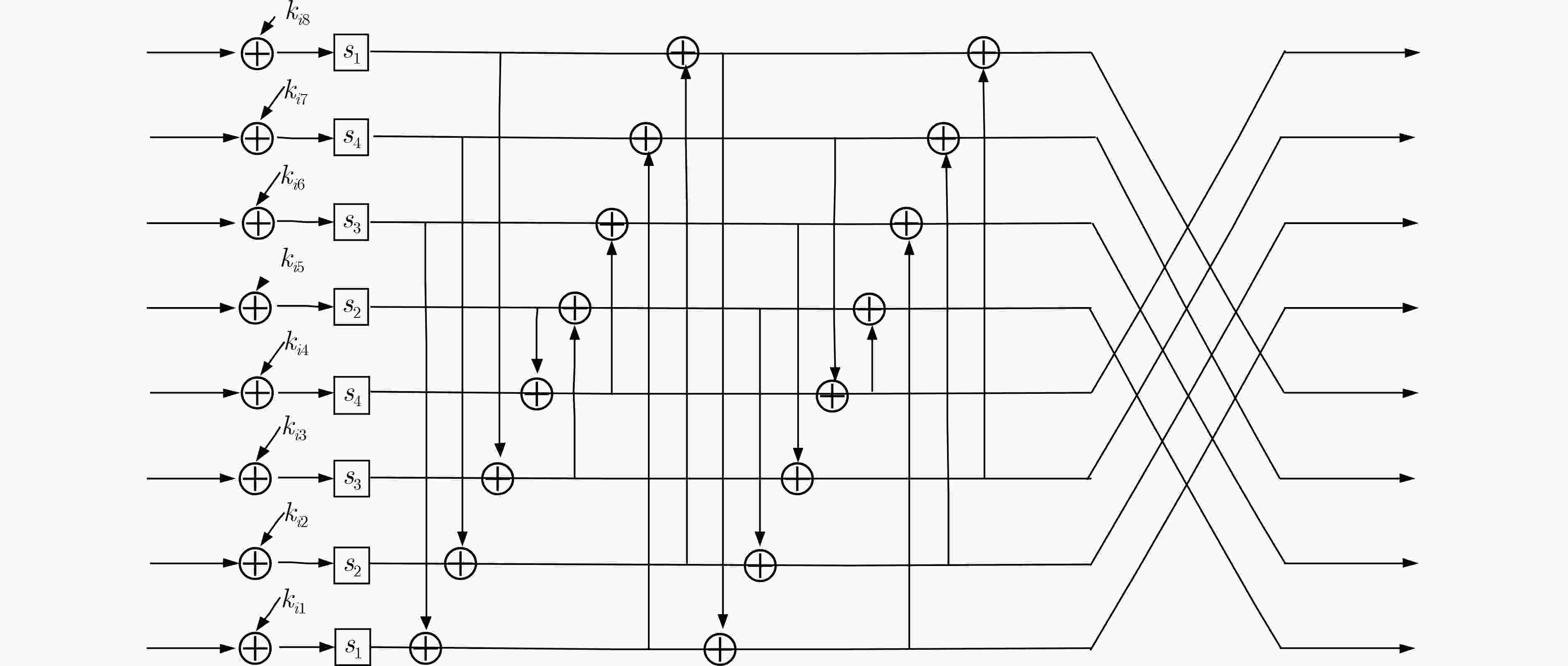
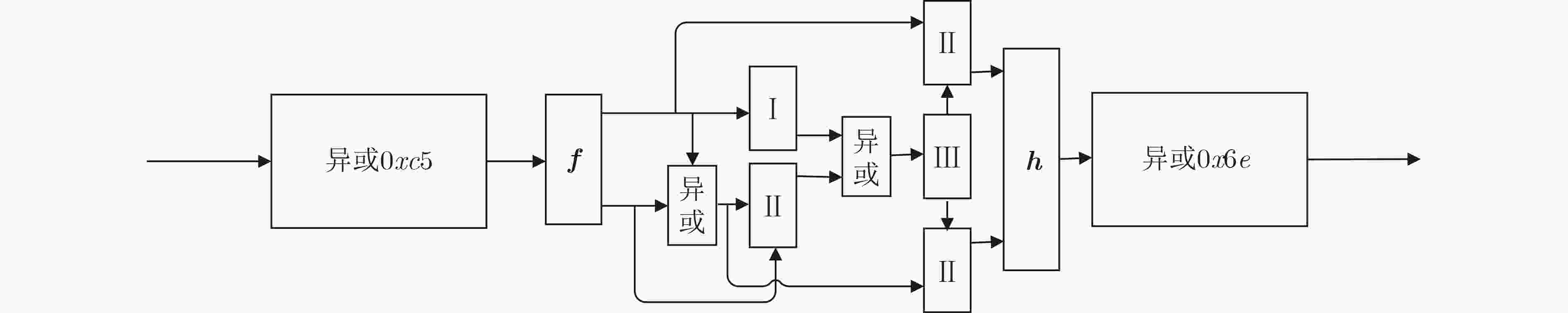


 下载:
下载:
