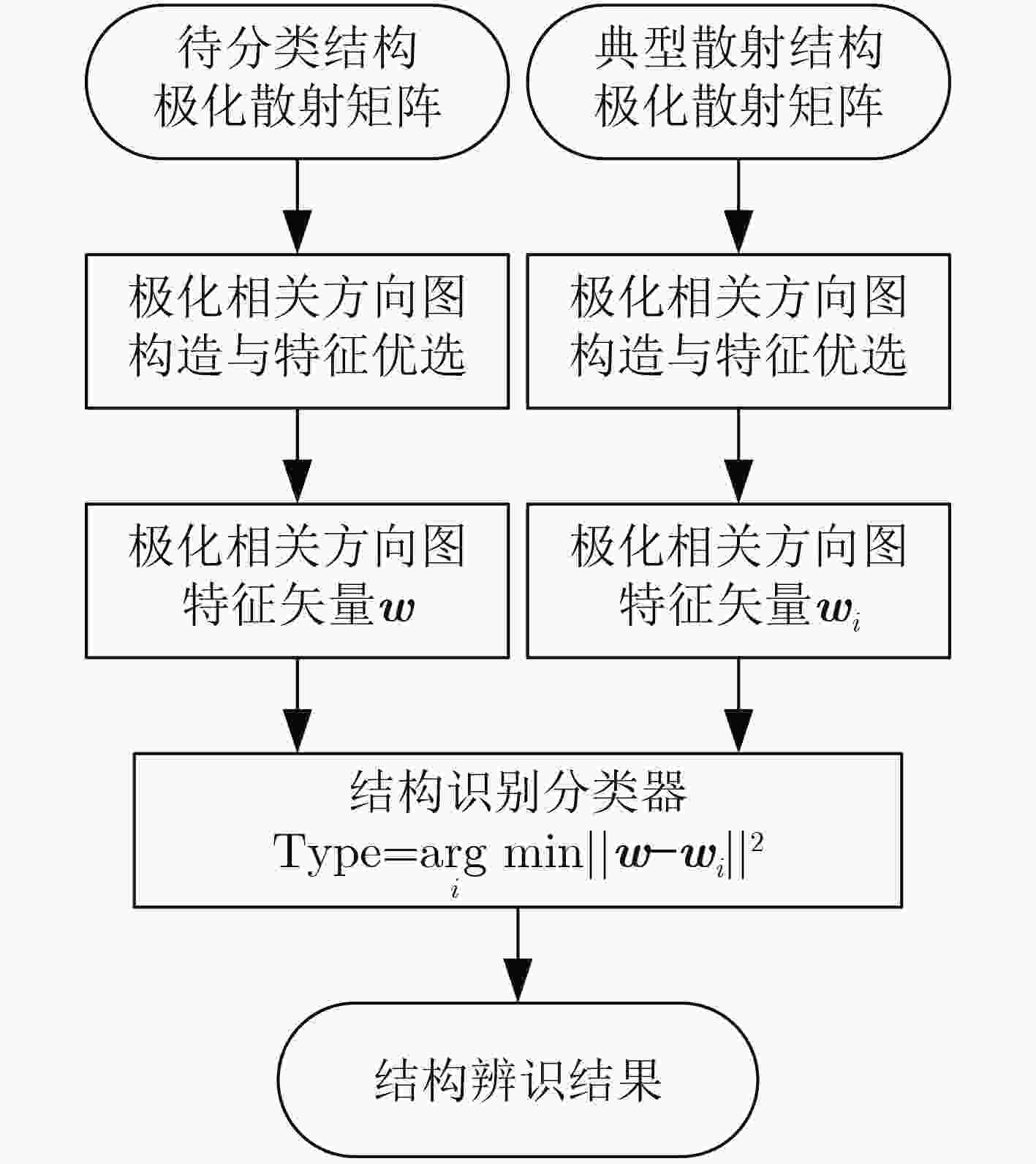
Scattering Structure Recognition of Space Target in Polarimetric Rotation Domain
-
摘要: 极化逆合成孔径雷达(ISAR)具备全极化测量和高分辨成像能力,已成为空间态势感知的重要传感器。作为典型的人造目标,空间目标散射特性敏感于目标姿态和雷达视线方向的相对夹角。这种散射多样性给极化ISAR数据解译带来困难,也蕴藏着丰富的极化散射信息。为提高空间目标散射结构辨识性能,该文深入挖掘绕雷达视线的极化旋转域信息,提出一种空间目标散射结构极化旋转域辨识方法,共包含3个步骤。首先,对极化ISAR数据开展绕雷达视线的极化旋转域分析,导出极化相关方向图特征。其次,分析基本散射体的极化相关方向图特性,构造极化特征编码矢量。最后,基于极化特征编码矢量距离度量实现散射结构极化辨识。围绕太阳能帆板、反射面天线等空间目标典型部件开展仿真实验研究,所提方法相较于传统的Cameron分解方法性能更优,鲁棒性更高。Abstract: Polarimetric Inverse Synthetic Aperture Radar (ISAR), which has the ability of full polarization measurement and high-resolution imaging, has become an important sensor for space awareness. As a typical man-made target, space target has various scattering characteristic, which is sensitive to the relative angle between the target orientation and the radar’s line of sight. This scattering diversity makes it difficult for polarimetric ISAR data interpretation. Besides, enrich polarimetric scattering information is hidden within it. In order to promote the interpretation performance of space target, a scattering structure recognition method in polarimetric rotation domain is proposed by mining the polarimetric rotation domain information along the radar’s line of sight, which mainly contains three steps. Firstly, polarimetric rotation domain analysis along the radar’s line of sight is conducted on the polarimetric ISAR data and a set of polarimetric correlation pattern features are derived. Secondly, the polarimetric correlation pattern characteristics of canonical structures are analyzed and the polarimetric feature coding vectors are given. Finally, the target scattering structure can be recognized by the distance of polarimetric feature coding vectors. Simulation experimental studies are carried out with the typical space target components of solar panel and reflector antenna. Compared with the traditional Cameron decomposition, the proposed method has superior and robust performance.
-
表 1 极化相关方向图特征表达式及物理意义
极化相关方向图特征 表达式 物理意义 原始极化相关特征值 ${\tilde \gamma _{ \text{-}{\text{org} } } } = \left| {\tilde \gamma \left( 0 \right)} \right|$ 原始成像几何下的去相关效应 极化相关度 ${\tilde \gamma _{ \text{-}{\text{mean} } } } = {\text{mean} }\left\{ {\left| {\tilde \gamma \left( \theta \right)} \right|} \right\}$ 极化旋转域的平均去相关效应 极化相关起伏度 ${\tilde \gamma _{ \text{-}{\text{std} } } } = {\text{std} }\left\{ {\left| {\tilde \gamma \left( \theta \right)} \right|} \right\}$ 极化相关值在极化旋转域中的起伏程度 极化相关特征最大值 ${\tilde \gamma _{ \text{-} \max } } = \max \left\{ {\left| {\tilde \gamma \left( \theta \right)} \right|} \right\}$ 极化相关值上界 极化相关特征最小值 ${\tilde \gamma _{ \text{-} \min } } = \min \left\{ {\left| {\tilde \gamma \left( \theta \right)} \right|} \right\}$ 极化相关值下界 极化相关对比度 ${\tilde \gamma _{\text{-}{\text{contrast} } } } = {\tilde \gamma _{ \text{-} \max } } - {\tilde \gamma _{ \text{-} \min } }$ 极化相关值极差 极化相关特征反熵 ${\tilde \gamma _{ \text{-}{\text{A} } } } = \left( { { {\tilde \gamma }_{ \text{-} \max } } - { {\tilde \gamma }_{ \text{-} \min } } } \right)/\left( { { {\tilde \gamma }_{\text{-} \max } } + { {\tilde \gamma }_{\text{-} \min } } } \right)$ 极化相关值相对极差 最小化旋转角 ${\theta _{\tilde \gamma \text{-} \min } } = \arg {\text{ } }\min \left\{ {\left| {\tilde \gamma \left( \theta \right)} \right|} \right\}$ 极化相关值最小时对应旋转角 最大化旋转角 ${\theta _{\tilde \gamma \text{-} \min } } = \arg {\text{ } }\max \left\{ {\left| {\tilde \gamma \left( \theta \right)} \right|} \right\}$ 极化相关值最大时对应旋转角 极化相关宽度 $\left\{ \begin{aligned}& { { {\tilde \gamma }_{ \text{-}{\text{bw0} }{\text{.95} } } } = {\theta _1} - {\theta _2} } \\ & {\tilde \gamma \left( { {\theta _1} } \right) = \tilde \gamma \left( { {\theta _2} } \right) = 0.95 \cdot { {\tilde \gamma }_{ {\text{-max} } } } } \end{aligned} \right.$ 目标对取向依赖性效应的敏感程度 表 2 7种典型散射体极化散射矩阵及极化相关方向图
平板 二面角 偶极子 圆柱 窄二面角 1/4波器件 螺旋散射体 极化散射矩阵 $\left[ {\begin{array}{*{20}{c}} 1&0 \\ 0&1 \end{array}} \right]$ $\left[ {\begin{array}{*{20}{c}} 1&0 \\ 0&{ - 1} \end{array}} \right]$ $\left[ {\begin{array}{*{20}{c}} 1&0 \\ 0&0 \end{array}} \right]$ $\left[ {\begin{array}{*{20}{c} } 1&0 \\ 0&{\dfrac{1}{2} } \end{array} } \right]$ $\left[ {\begin{array}{*{20}{c} } 1&0 \\ 0&{ - \dfrac{1}{2} } \end{array} } \right]$ $\left[ {\begin{array}{*{20}{c}} 1&0 \\ 0&{\text{j}} \end{array}} \right]$ $\dfrac{1}{2}\left[ {\begin{array}{*{20}{c} } 1&{\text{j} } \\ {\text{j} }&{ - 1} \end{array} } \right]$ $\left| { { {\tilde \gamma }_{ {\text{HH-HV} } } }\left( \theta \right)} \right|$ 






$\left| { { {\tilde \gamma }_{ {\text{HH-VV} } } }\left( \theta \right)} \right|$ 






$\left| { { {\tilde \gamma }_{({\text{HH+VV} }){\text{-(HH-VV)} } } }\left( \theta \right)} \right|$ 






$\left| { { {\tilde \gamma }_{({\text{HH-VV} }){\text{-(HV)} } } }\left( \theta \right)} \right|$ 






表 3 电磁仿真参数
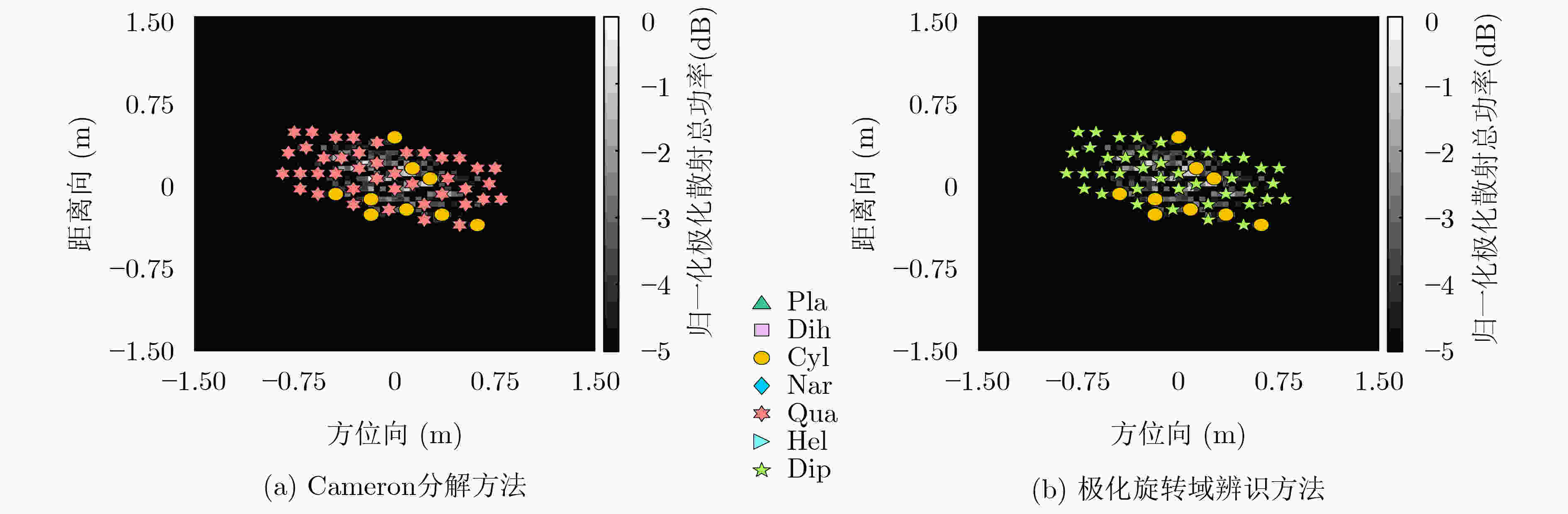
波段 载频(GHz) 距离向分辨率(m) 成像孔径(°) 方位向分辨率(m) 方位角(°) 俯仰角(°) X 9.5~10.5 0.150 5.73 0.150 [0:15:90] [30 40] Ku 15.5~17.5 0.075 6.94 0.075 [0:15:90] [30 40] 表 4 X波段太阳能帆板结构辨识结果中各散射结构占比(%)
观测视角 Cameron分解 极化旋转域辨识 俯仰角 方位角 平板 圆柱 1/4波器件 其它 平板 圆柱 1/4波器件 其它 30° 0° 73.33 26.67 0 0 73.33 26.67 0 0 15° 0 86.67 13.33 0 0 100.00 0 0 30° 23.33 76.67 0 0 76.67 13.33 10.00 0 45° 100.00 0 0 0 96.67 0 0 3.33 60° 100.00 0 0 0 100.00 0 0 0 75° 23.33 73.33 3.33 0 73.33 3.33 23.33 0 90° 0 30.00 70.00 0 0 83.33 6.67 10.00 40° 0° 90.00 10.00 0 0 90.00 10.00 0 0 15° 100.00 0 0 0 100.00 0 0 0 30° 0 100.00 0 0 0 100.00 0 0 45° 73.33 26.67 0 0 46.67 53.33 0 0 60° 0 100.00 0 0 0 100.00 0 0 75° 0 100.00 0 0 0 100.00 0 0 90° 63.33 20.00 13.33 3.33 63.33 10.00 23.33 3.33 表 5 Ku波段太阳能帆板结构辨识结果中各散射结构占比(%)
观测视角 Cameron分解 极化旋转域辨识 俯仰角 方位角 平板 圆柱 1/4波器件 其它 平板 圆柱 偶极子 其它 30° 0° 0 28.00 72.00 0 0 30.00 70.00 0 15° 0 18.00 82.00 0 0 18.00 82.00 0 30° 0 94.00 6.00 0 0 100.00 0 0 45° 0 14.00 62.00 24.00 0 24.00 22.00 54.00 60° 2.00 50.00 46.00 2.00 2.00 66.00 18.00 14.00 75° 0 28.00 72.00 0 0 50.00 50.00 0 90° 40.00 60.00 0 0 98.00 0 0 2.00 40° 0° 54.00 40.00 6.00 0 56.00 42.00 0 2.00 15° 0 48.00 52.00 0 0 66.00 34.00 0 30° 8.00 92.00 0 0 62.00 38.00 0 0 45° 2.00 12.00 64.00 22.00 2.00 8.00 34.00 56.00 60° 26.00 52.00 22.00 0 20.00 24.00 2.00 54.00 75° 0 100.00 0 0 0 100.00 0 0 90° 58.00 42.00 0 0 58.00 42.00 0 0 表 6 X波段反射面天线结构辨识结果中各散射结构占比(%)
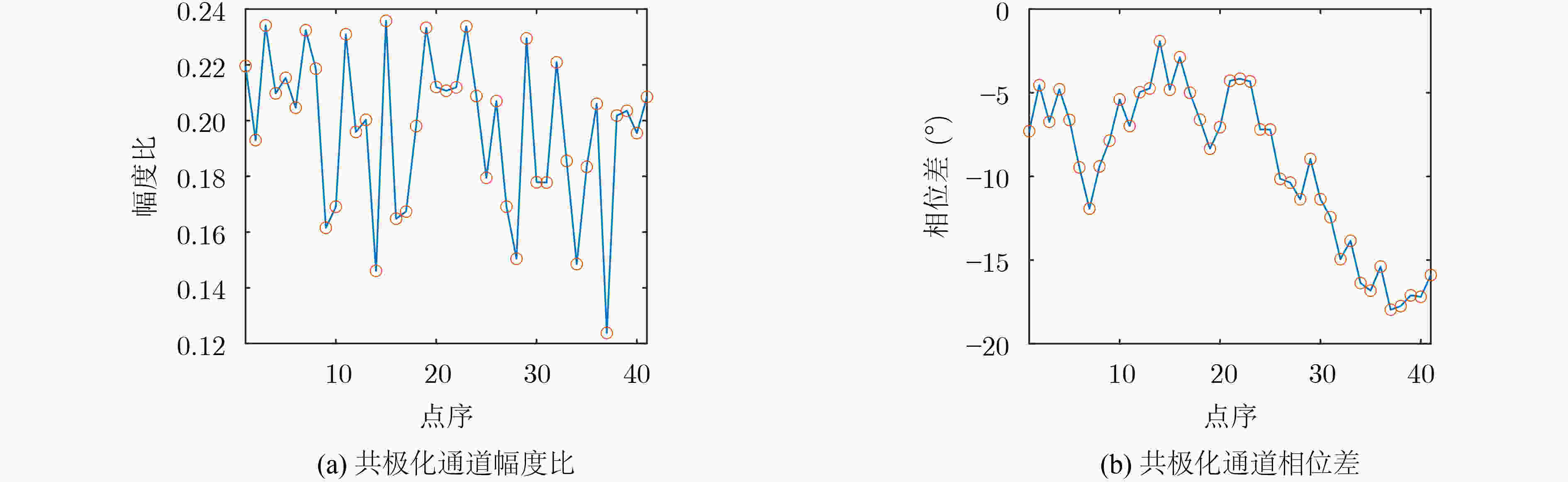
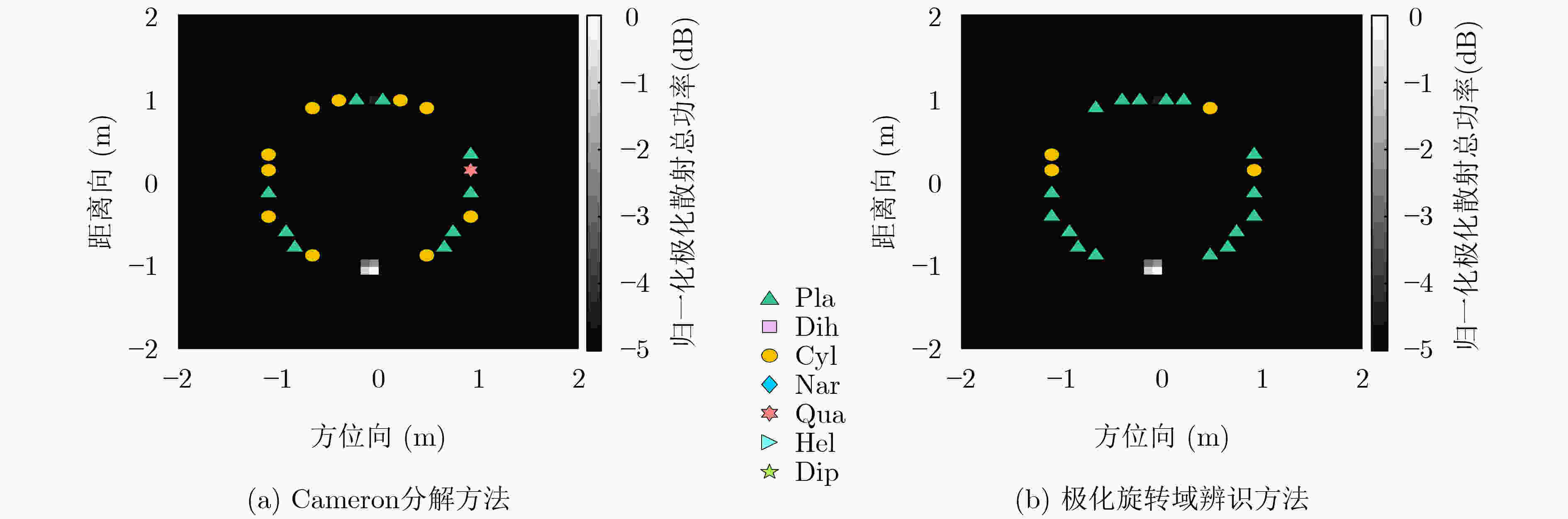
观测视角 Cameron分解 极化旋转域辨识 俯仰角 方位角 平板 圆柱 1/4波器件 其它 平板 圆柱 1/4波器件 其它 30° 0° 85.00 10.00 0 5.00 85.00 10.00 5.00 0 15° 80.00 20.00 0 0 80.00 20.00 0 0 30° 95.00 5.00 0 0 95.00 5.00 0 0 45° 100.00 0 0 0 100.00 0 0 0 60° 80.00 20.00 0 0 85.00 15.00 0 0 75° 90.00 10.00 0 0 90.00 10.00 0 0 90° 85.00 10.00 0 5.00 85.00 10.00 5.00 0 40° 0° 35.00 40.00 20.00 5.00 50.00 15.00 30.00 5.00 15° 75.00 25.00 0 0 90.00 10.00 0 0 30° 65.00 25.00 5.00 5.00 80.00 0 15.00 5.00 45° 45.00 50.00 5.00 0 80.00 20.00 0 0 60° 80.00 15.00 5.00 0 90.00 0 5.00 5.00 75° 75.00 25.00 0 0 90.00 10.00 0 0 90° 35.00 40.00 15.00 10.00 50.00 15.00 30.00 5.00 表 7 Ku波段反射面天线结构辨识结果中各散射结构占比(%)
观测视角 Cameron分解 极化旋转域辨识 俯仰角 方位角 平板 圆柱 1/4波器件 其它 平板 圆柱 1/4波器件 其它 30° 0° 56.67 36.67 6.67 0 66.67 20.00 13.33 0 15° 56.67 36.67 6.67 0 66.67 26.67 6.67 0 30° 70.00 23.33 3.33 3.33 73.33 16.67 6.67 3.33 45° 56.67 36.67 3.33 3.33 70.00 20.00 3.33 6.67 60° 66.67 30.00 0 3.33 70.00 26.67 0 3.33 75° 63.33 33.33 3.33 0 73.33 23.33 3.33 0 90° 56.67 33.33 10.00 0 66.67 20.00 13.33 0 40° 0° 46.67 40.00 13.33 0 40.00 56.67 0 3.33 15° 56.67 40.00 3.33 0 60.00 36.67 3.33 0 30° 60.00 40.00 0 0 66.67 33.33 0 0 45° 56.67 40.00 3.33 0 56.67 36.67 3.33 3.33 60° 46.67 50.00 3.33 0 63.33 33.33 3.33 0 75° 60.00 36.67 3.33 0 60.00 40.00 0 0 90° 46.67 40.00 13.33 0 40.00 56.67 0 3.33 -
[1] CHEN V C, MARTORELLA M, 胡明春, 孙俊, 译. 逆合成孔径雷达成像[M]. 北京: 国防工业出版社, 2020.CHEN V C, MARTORELLA M, HU Mingchun, SUN Jun. translation. Inverse Synthetic Aperture Radar Imaging[M]. Beijing: National Defense Industry Press, 2020. [2] FHR. Long-term analysis of the attitude motion of the defunct ENVISAT[EB/OL]. https://www.fhr.fraunhofer.de/en/businessunits/space/long-term-analysis-of-the-attitude-motion-of-the-defunct-satellite-ENVISAT.html, 2022. [3] FHR. Monitoring the re-entry of the chinese space station Tiangong-1 with TIRA[EB/OL]. https://www.fhr.fraunhofer.de/en/businessunits/space/monitoring-the-re-entry-of-the-chinese-space-station-tiangong-1-with-tira.html, 2022. [4] LIU Yang, LI Gang, TIAN Biao, et al. ISAR imaging at low SNR level based on polarimetric whitening filter[J]. SPIE 8917, 2013, 891703-1–891703-8. [5] 吴佳妮. 人造目标几何结构反演与极化雷达识别研究[D]. [博士论文], 国防科学技术大学, 2017.WU Jiani. Study on geometrical structure retrieval and polarization radar recognition of man-made targets[D]. [Ph. D. dissertation], National University of Defense Technology, 2017. [6] 田彪, 刘洋, 呼鹏江, 等. 宽带逆合成孔径雷达高分辨成像技术综述[J]. 雷达学报, 2020, 9(5): 765–802. doi: 10.12000/JR20060TIAN Biao, LIU Yang, HU Pengjiang, et al. Review of high-resolution imaging techniques of wideband inverse synthetic aperture radar[J]. Journal of Radars, 2020, 9(5): 765–802. doi: 10.12000/JR20060 [7] HURST M and MITTRA R. Scattering center analysis via Prony's method[J]. IEEE Transactions on Antennas and Propagation, 1987, 35(8): 986–988. doi: 10.1109/TAP.1987.1144210 [8] POTTER L C, CHIANG Daming, CARRIERE R, et al. A GTD-based parametric model for radar scattering[J]. IEEE Transactions on Antennas and Propagation, 1995, 43(10): 1058–1067. doi: 10.1109/8.467641 [9] POTTER L C and MOSES R L. Attributed scattering centers for SAR ATR[J]. IEEE Transactions on Image Processing, 1997, 6(1): 79–91. doi: 10.1109/83.552098 [10] JACKSON J A, RIGLING B D, and MOSES R L. Canonical scattering feature models for 3D and bistatic SAR[J]. IEEE Transactions on Aerospace and Electronic Systems, 2010, 46(2): 525–541. doi: 10.1109/TAES.2010.5461639 [11] 张腊梅, 段宝龙, 邹斌. 极化SAR图像目标分解方法的研究进展[J]. 电子与信息学报, 2016, 38(12): 3289–3297. doi: 10.11999/JEIT160992ZHANG Lamei, DUAN Baolong, and ZOU Bin. Research development on target decomposition method of polarimetric SAR image[J]. Journal of Electronics &Information Technology, 2016, 38(12): 3289–3297. doi: 10.11999/JEIT160992 [12] CLOUDE S R and POTTIER E. A review of target decomposition theorems in radar polarimetry[J]. IEEE Transactions on Geoscience and Remote Sensing, 1996, 34(2): 498–518. doi: 10.1109/36.485127 [13] KROGAGER E. New decomposition of the radar target scattering matrix[J]. Electronics Letters, 1990, 26(18): 1525–1527. doi: 10.1049/el:19900979 [14] CAMERON W L, YOUSSEF N N, and LEUNG L K. Simulated polarimetric signatures of primitive geometrical shapes[J]. IEEE Transactions on Geoscience and Remote Sensing, 1996, 34(3): 793–803. doi: 10.1109/36.499784 [15] TOUZI R and CHARBONNEAU F. Characterization of target symmetric scattering using polarimetric SARs[J]. IEEE Transactions on Geoscience and Remote Sensing, 2002, 40(11): 2507–2516. doi: 10.1109/TGRS.2002.805070 [16] 吴国庆, 陈思伟, 李永祯, 等. 人造目标散射结构的零极化辨识与应用[J]. 电波科学学报, 2022, 37(5): 733–742, 760.WU Guoqing, CHEN Siwei, LI Yongzhen, et al. Manmade targets scattering structure null-polarization recognition and application[J]. Chinese Journal of Radio Science, 2022, 37(5): 733–742, 760. [17] 段佳, 曹兰英, 吴亿锋. 基于属性散射中心的SAR成像方法[J]. 系统工程与电子技术, 2021, 43(10): 2782–2788. doi: 10.12305/j.issn.1001-506X.2021.10.10DUAN Jia, CAO Lanying, and WU Yifeng. Imaging algorithm for SAR based on attributed scattering center models[J]. Systems Engineering and Electronics, 2021, 43(10): 2782–2788. doi: 10.12305/j.issn.1001-506X.2021.10.10 [18] DUAN Jia, ZHANG Lei, XING Mengdao, et al. Polarimetric target decomposition based on attributed scattering center model for synthetic aperture radar targets[J]. IEEE Geoscience and Remote Sensing Letters, 2014, 11(12): 2095–2099. doi: 10.1109/LGRS.2014.2320053 [19] ARII M. Ship detection from full polarimetric SAR data at different incidence angles[C]. 2011 3rd International Asia-Pacific Conference on Synthetic Aperture Radar, Seoul, Korea (South), 2011: 1–4. [20] SAVILLE M A, JACKSON J A, and FULLER D F. Rethinking vehicle classification with wide-angle polarimetric SAR[J]. IEEE Aerospace and Electronic Systems Magazine, 2014, 29(1): 41–49. doi: 10.1109/MAES.2014.130057 [21] CHEN Siwei. Polarimetric coherence pattern: A visualization and characterization tool for PolSAR data investigation[J]. IEEE Transactions on Geoscience and Remote Sensing, 2018, 56(1): 286–297. doi: 10.1109/TGRS.2017.2746662 [22] 陈思伟, 李永祯, 王雪松, 等. 极化SAR目标散射旋转域解译理论与应用[J]. 雷达学报, 2017, 6(5): 442–455. doi: 10.12000/jr17033CHEN Siwei, LI Yongzhen, WANG Xuesong, et al. Polarimetric SAR target scattering interpretation in rotation domain: Theory and application[J]. Journal of Radars, 2017, 6(5): 442–455. doi: 10.12000/jr17033 [23] CUI Xingchao, TAO Chensong, SU Yi, et al. PolSAR ship detection based on polarimetric correlation pattern[J]. IEEE Geoscience and Remote Sensing Letters, 2021, 18(3): 471–475. doi: 10.1109/LGRS.2020.2976477 [24] 陶臣嵩, 陈思伟, 李永祯, 等. 结合旋转域极化特征的极化SAR地物分类[J]. 雷达学报, 2017, 6(5): 524–532. doi: 10.12000/jr16131TAO Chensong, CHEN Siwei, LI Yongzhen, et al. Polarimetric SAR terrain classification using polarimetric features derived from rotation domain[J]. Journal of Radars, 2017, 6(5): 524–532. doi: 10.12000/jr16131 [25] CHEN Siwei and TAO Chensong. PolSAR image classification using polarimetric-feature-driven deep convolutional neural network[J]. IEEE Geoscience and Remote Sensing Letters, 2018, 15(4): 627–631. doi: 10.1109/LGRS.2018.2799877 [26] LI Haoliang, LI Mingdian, CUI Xingchao, et al. Man-made target structure recognition with polarimetric correlation pattern and roll-invariant feature coding[J]. IEEE Geoscience and Remote Sensing Letters, 2022, 19: 8024105. doi: 10.1109/LGRS.2021.3121100 [27] WU Guoqing, CHEN Siwei, LI Yongzhen, et al. Null-pol response pattern in polarimetric rotation domain: Characterization and application[J]. IEEE Geoscience and Remote Sensing Letters, 2022, 19: 4503105. doi: 10.1109/LGRS.2021.3139889 [28] VAN ZYL J J. Calibration of polarimetric radar images using only image parameters and trihedral corner reflector responses[J]. IEEE Transactions on Geoscience and Remote Sensing, 1990, 28(3): 337–348. doi: 10.1109/36.54360 -





 下载:
下载:

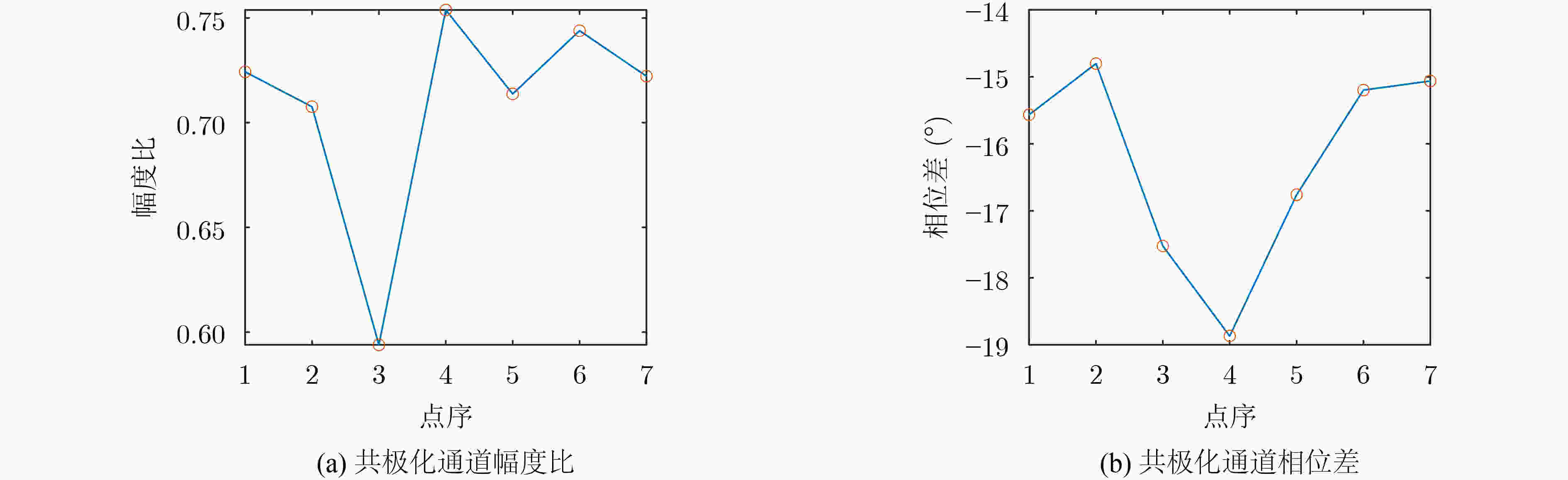


 下载:
下载:
