Spaceborne Interrupted Frequency Modulate Continuous Wave SAR Imaging Based on Low-Rank Hankel Matrix Reconstruction Technique
-
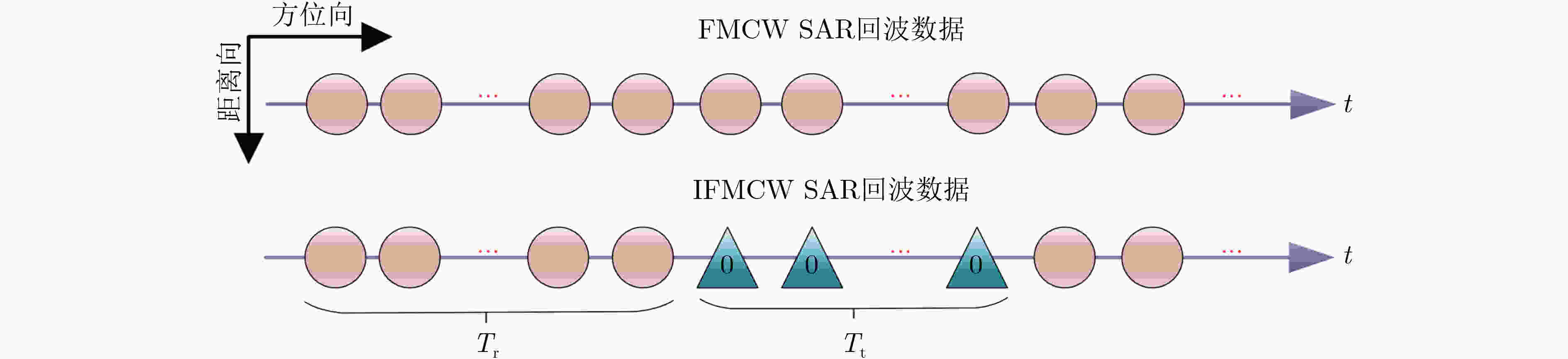
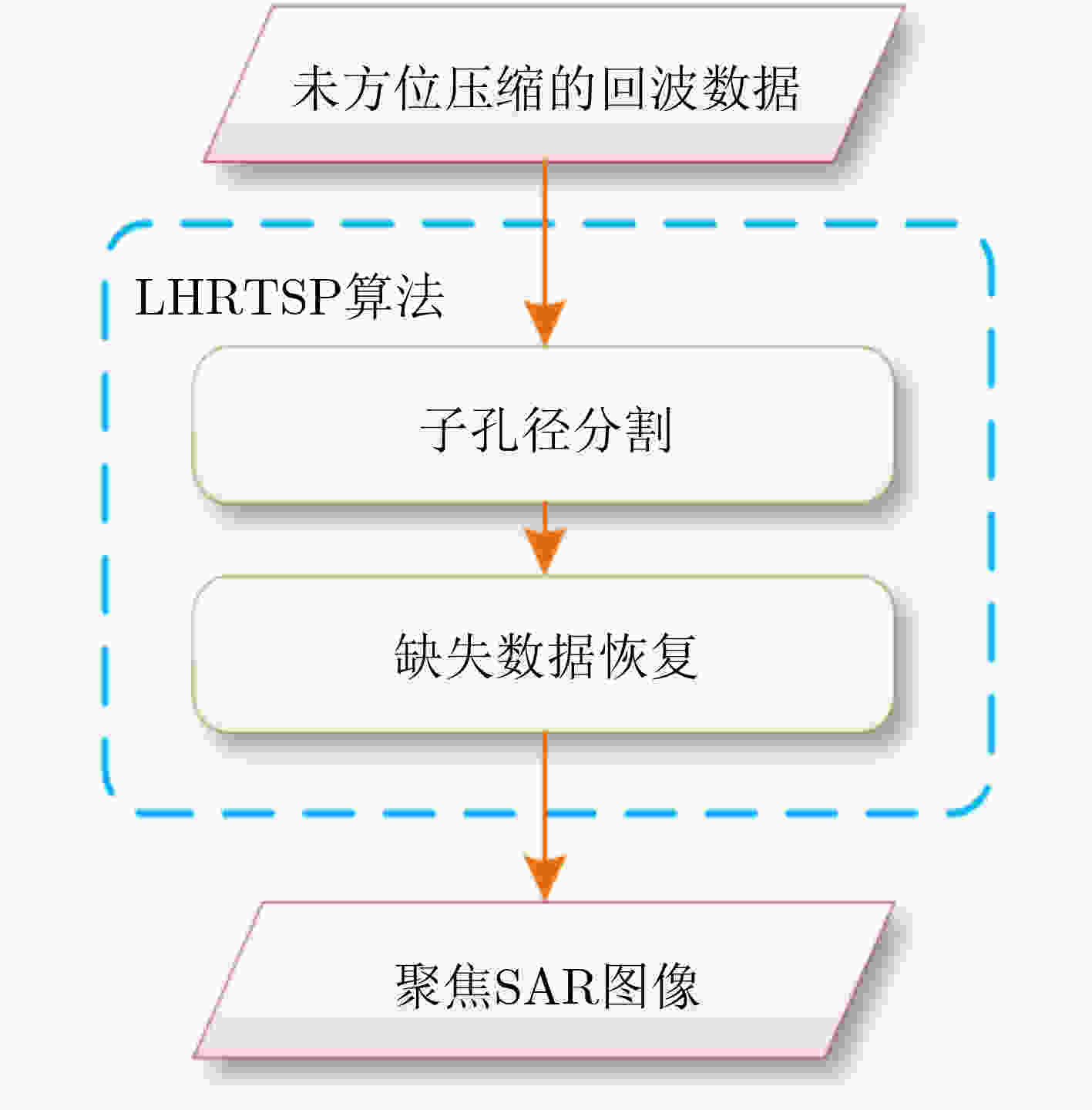
摘要: 间断调频连续波(IFMCW)合成孔径雷达(SAR)是一种新型的集轻量化、低成本和低功耗于一体的新型SAR系统。该系统采用单根天线发射和接收信号,颠覆了传统的调频连续波(FMCW) SAR系统设计理念。在该模式下,由于发射机工作时接收机关闭,导致合成孔径中出现周期性的间隔,采用传统成像算法进行成像,在聚焦SAR图像中将会出现周期性的虚假目标。为了有效地抑制虚假目标,该文基于子孔径回波数据,提出一种新的成像算法,即基于子孔径投影的低秩汉克尔矩阵重构技术(LHRTSP)。实验结果表明与现有方法相比,所提方法对虚假目标的抑制效果更佳,验证了所提方法的有效性。Abstract: The Interrupted Frequency Modulated Continuous Wave (IFMCW) Synthetic Aperture Radar (SAR) is a novel type of SAR system,which has the advantages of light weight, low cost, and low power consumption. The system subverts the design concept of the traditional Frequency Modulated Continuous Wave (FMCW) SAR system, which use a single antenna to transmit and receive signals.In this system, the transmitter and receiver operate at different time intervals, resulting in periodic gaps in the synthetic aperture. When the received echo data is imaged using traditional imaging algorithms, the artifacts will appear in the focused SAR image. In order to suppress effectively the appearance of artifacts, this paper proposes a new imaging algorithm for subaperture echo data processing, which is called Low-rank Hankel matrix Reconstruction Technique based on Subaperture Projection (LHRTSP). The experimental results show that the proposed method has better suppression effect on artifacts compared with the existing methods, which verifies the effectiveness and superiority of the proposed method.
-
算法1 GIRAF算法 初始化: $ {{X}^{{\text{(0)}}}} $和$\varepsilon 0$ for n=1:I max 第1步: 利用公式(11),构建格拉姆矩阵${{\boldsymbol{G}}}$ 对${{\boldsymbol{G}}}$进行特征值分解 利用式(14)计算湮灭滤波器${{\boldsymbol{h}}}$ 利用式(18),将滤波器${{\boldsymbol{h}}}$转换为权重 第2步: 求解最小二乘问题: 通过ADMM迭代式(15)-式(17),计算$ {{X}^{{\text{(}}n{\text{)}}}} $ if $0 < \varepsilon_n < \varepsilon_n - 1$ end end 表 1 星载IFMCW SAR模式仿真参数
参数 数值 参数 数值 雷达工作载频(Hz) 16.70 脉冲发射频率(Hz) 3479.00 雷达有效速度(m/s) 7613.00 天线长度(m) 4.48 带宽(MHz) 180.00 接收脉冲(个) 13 景中心斜距(km) 534.00 缺失脉冲(个) 12 发射脉冲时宽(μs) 266.71 斜视角(°) 0 表 2 研究区域A和B中不同方法的定量分析
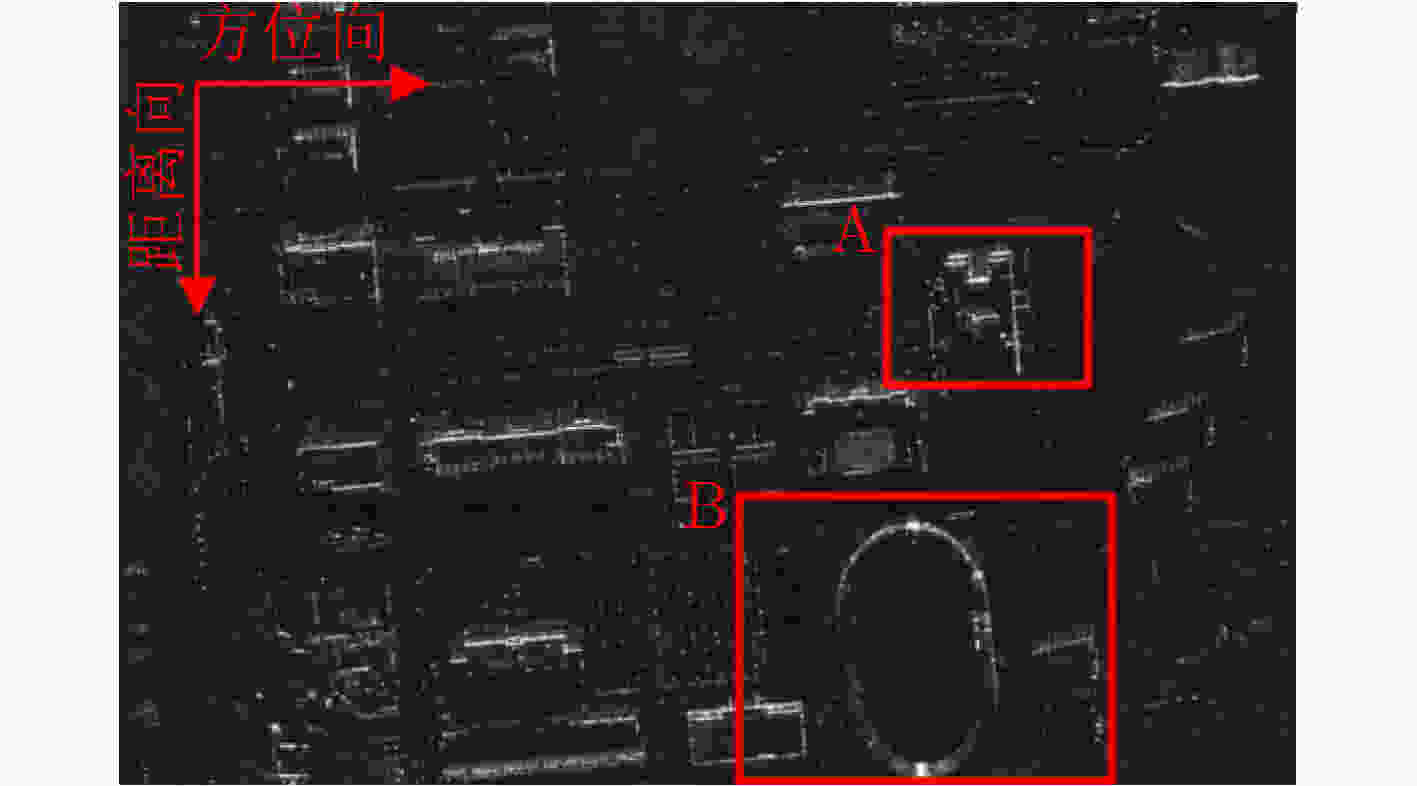
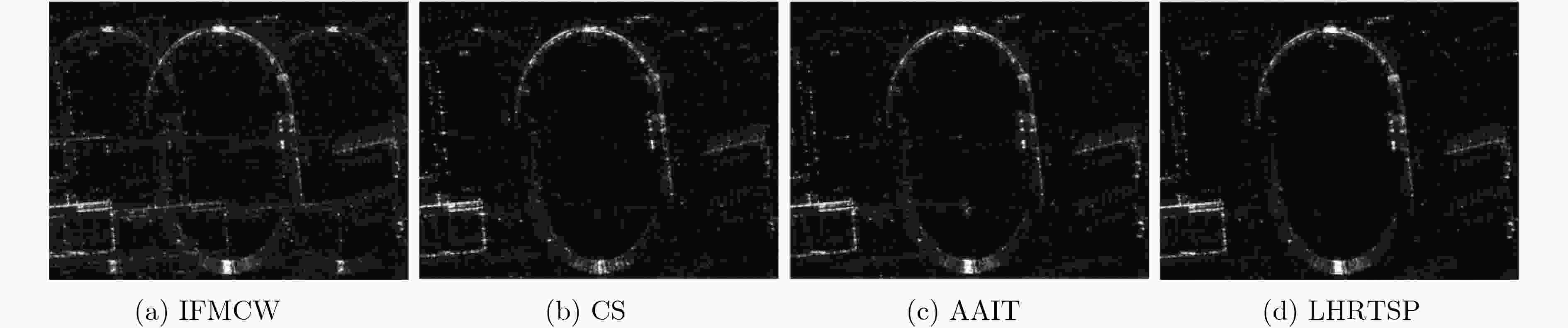
指标 IFMCW AAIT CS LHRTSP RMSE(A) 303.04 178.26 134.93 67.78 SSIM(A) 0.39 0.48 0.51 0.63 IE(A) 11.59 11.38 11.33 11.21 RMSE(B) 241.94 98.75 82.79 57.57 SSIM(B) 0.39 0.50 0.46 0.61 IE(B) 9.82 9.75 9.96 9.22 -
[1] 李春升, 王伟杰, 王鹏波, 等. 星载SAR技术的现状与发展趋势[J]. 电子与信息学报, 2016, 38(1): 229–240. doi: 10.11999/JEIT151116LI Chunsheng, WANG Weijie, WANG Pengbo, et al. Current situation and development trends of spaceborne SAR technology[J]. Journal of Electronics &Information Technology, 2016, 38(1): 229–240. doi: 10.11999/JEIT151116 [2] 邓云凯, 禹卫东, 张衡, 等. 未来星载SAR技术发展趋势[J]. 雷达学报, 2020, 9(1): 1–33. doi: 10.12000/JR20008DENG Yunkai, YU Weidong, ZHANG Heng, et al. Forthcoming spaceborne SAR development[J]. Journal of Radars, 2020, 9(1): 1–33. doi: 10.12000/JR20008 [3] SALZMAN J, AKAMINE D, LEFEVRE R, et al. Interrupted synthetic aperture radar (SAR)[C]. Proceedings of the 2001 IEEE Radar Conference (Cat. No. 01CH37200), Atlanta, USA, 2001: 117–122. [4] AHMED N and UNDERWOOD C. Monostatic CW SAR concept for microsatellites[C]. 8th European Conference on Synthetic Aperture Radar, Aachen, Germany, 2010: 736–739. [5] 王宝平. 线性调频连续波雷达信号泄漏对消技术的研究[D]. [硕士论文], 电子科技大学, 2013.WANG Baoping. Research of FMCW radar leakage signal cancellation techonology[D]. [Master dissertation], University of Electronic Science and Technology of China, 2013. [6] LI Ning, WANG R, DENG Yunkai, et al. Improved full-aperture ScanSAR imaging algorithm based on aperture interpolation[J]. IEEE Geoscience and Remote Sensing Letters, 2015, 12(5): 1101–1105. doi: 10.1109/LGRS.2014.2384594 [7] LI Ning, NIU Shilin, GUO Zhengwei, et al. Processing spaceborne interrupted FMCW SAR data with modified aperture interpolation technique[C]. 2018 IEEE International Geoscience and Remote Sensing Symposium, Valencia, Spain, 2018: 6695–6698. [8] LIU Kang, YU Weidong, and LV Jiyu. Azimuth interrupted FMCW SAR for high-resolution imaging[J]. IEEE Geoscience and Remote Sensing Letters, 2022, 19: 4001105. doi: 10.1109/LGRS.2020.3019047 [9] LIU Kang, YU Weidong, and LV Jiyu. Parameter design and imaging method of spaceborne azimuth interrupted FMCW SAR[J]. IEEE Geoscience and Remote Sensing Letters, 2022, 19: 4015505. doi: 10.1109/LGRS.2021.3095173 [10] 闵林, 刘向前, 李宁. 基于积累孔径插值技术的星载间断调频连续波SAR成像方法[J]. 电子与信息学报, 2022, 44(7): 2461–2468. doi: 10.11999/JEIT210140MIN Lin, LIU Xiangqian, and LI Ning. Spaceborne interrupted frequency modulation continuous wave SAR imaging based on accumulated aperture interpolation technique[J]. Journal of Electronics &Information Technology, 2022, 44(7): 2461–2468. doi: 10.11999/JEIT210140 [11] HU Yue, LIU Xiaohan, and JACOB M. A generalized structured low-rank matrix completion algorithm for MR image recovery[J]. IEEE Transactions on Medical Imaging, 2019, 38(8): 1841–1851. doi: 10.1109/TMI.2018.2886290 [12] QU Xiaobo, MAYZEL M, CAI Jianfeng, et al. Accelerated NMR spectroscopy with low-rank reconstruction[J]. Angewandte Chemie International Edition, 2015, 54(3): 852–854. doi: 10.1002/anie.201409291 [13] PRAMANIK A, AGGARWAL H K, and JACOB M. Deep generalization of structured low-rank algorithms (Deep-SLR)[J]. IEEE Transactions on Medical Imaging, 2020, 39(12): 4186–4197. doi: 10.1109/tmi.2020.3014581 [14] FORNASIER M, RAUHUT H, and WARD R. Low-rank matrix recovery via iteratively reweighted least squares minimization[J]. SIAM Journal on Optimization, 2011, 21(4): 1614–1640. doi: 10.1137/100811404 [15] ONGIE G and JACOB M. A fast algorithm for structured low-rank matrix recovery with applications to undersampled MRI reconstruction[C]. 2016 IEEE 13th International Symposium on Biomedical Imaging (ISBI), Prague, Czech Republic, 2016: 522–525. [16] ONGIE G and JACOB M. A fast algorithm for convolutional structured low-rank matrix recovery[J]. IEEE Transactions on Computational Imaging, 2017, 3(4): 535–550. doi: 10.1109/TCI.2017.2721819 [17] QIU Tianyu, WANG Zi, LIU Huiting, et al. Review and prospect: NMR spectroscopy denoising and reconstruction with low-rank Hankel matrices and tensors[J]. Magnetic Resonance in Chemistry, 2021, 59(3): 324–345. doi: 10.1002/mrc.5082 [18] CHEN Jinchi, GAO Weiguo, and WEI Ke. Exact matrix completion based on low rank Hankel structure in the Fourier domain[J]. Applied and Computational Harmonic Analysis, 2021, 55: 149–184. doi: 10.1016/j.acha.2021.05.002 [19] LI Xi, LIU Guosui, and NI Jinlin. Autofocusing of ISAR images based on entropy minimization[J]. IEEE Transactions on Aerospace and Electronic Systems, 1999, 35(4): 1240–1252. doi: 10.1109/7.805442 [20] 李春升, 于泽, 陈杰. 高分辨率星载SAR成像与图像质量提升方法综述[J]. 雷达学报, 2019, 8(6): 717–731. doi: 10.12000/JR19085LI Chunsheng, YU Ze, and CHEN Jie. Overview of techniques for improving high-resolution spaceborne SAR imaging and image quality[J]. Journal of Radars, 2019, 8(6): 717–731. doi: 10.12000/JR19085 -






 下载:
下载:






 下载:
下载:
