Parameter Sstimation Methods of Uni-Direction-ElectroMagnetic-Vector-Sensor
-
摘要: 传统的单电磁矢量传感器(UEMVS)由3个电偶极子和3个磁环构成且方向图是全向的。但是当多个单电磁矢量传感器依附在共形载体上构成共形电磁矢量传感器阵列时，为了降低共形电磁矢量传感器阵列的副瓣，通常每个传感器的方向图是有向的。基于有向方向图的单电磁矢量传感器也称为单有向电磁矢量传感器(UDEMVS)。该文针对UDEMVS的参数估计问题，提出两种参数估计方法，分别是基于免搜索的旋转不变信号参数估计和矢量叉积(ESPRIT-VCP)方法以及基于网格搜索的多重信号分类和最小瑞利商(MUSIC-MRQ)方法。ESPRIT-VCP方法是根据旋转不变性和矢量叉积，获得4维参数的闭式解，MUSIC-MRQ方法根据信号和噪声子空间正交性与最小瑞利商，利用网格搜索得到2维角度估计值，进而结合信号回波模型得到2维极化的估计值。所提两种方法只利用了UDEMVS的6通道数据就能有效得到目标的参数估计结果，有较低的计算复杂度。仿真结果从角度和极化的估计性能出发验证了所提方法的有效性。
-
关键词:
- 单有向电磁矢量传感器 /
- 参数估计 /
- 矢量叉积 /
- 最小瑞利商
Abstract: The traditional Uni-ElectroMagnetic-Vector-Sensor (UEMVS) is composed of three electric dipoles and three magnetic loops, and the pattern is omnidirectional. However, when multiple UEMVS are attached to the conformal platform to form a conformal UEMVS array, the pattern of each sensor is usually directional to reduce the sidelobe of the conformal UEMVS array. The UEMVS based on directional pattern is also called Uni-Direction-ElectroMagnetic-Vector-Sensor(UDEMVS). Considering the parameter estimation problem of UDEMVS, two parameter estimation methods, namely the Estimation of Signal Parameters via Rotational Invariant Technique and Vector Cross Product(ESPRIT-VCP) based on gridless search, and MUltiple SIgnal Classification and Minimum Rayleigh Quotient(MUSIC-MRQ) based on grid search, are proposed. The closed-form solutions of ESPRIT-VCP method can be obtained by the rotation invariance and vector cross product. The angle estimation results of MUSIC-MRQ method can be obtained by grid search and the minimum Rayleigh quotient model, and then the two-dimensional polarization parameters can be obtained by combining with the signal echo model. Using only six channel data of UDEMVS, the proposed two methods can effectively obtain the parameter estimation results, and have low computational complexity. Simulation results verify the effectiveness of the proposed method from the estimation performance of angle and polarization. -
图 6 分辨概率随SNR变化的结果，其中分辨门限
${\varDelta _\phi } = {\varDelta _\theta } = {\varDelta _\gamma } = {\varDelta _\eta } = 1^\circ$ -
[1] LI Weilin, LIAO Wenjing, and FANNJIANG A. Super-resolution limit of the ESPRIT algorithm[J]. IEEE Transactions on Information Theory, 2020, 66(7): 4593–4608. doi: 10.1109/TIT.2020.2974174 [2] XU Baoqing, ZHAO Yongbo, CHENG Zengfei, et al. A novel unitary PARAFAC method for DOD and DOA estimation in bistatic MIMO radar[J]. Signal Processing, 2017, 138: 273–279. doi: 10.1016/j.sigpro.2017.03.016 [3] XU Baoqing and ZHAO Yongbo. Transmit beamspace-based DOD and DOA estimation method for bistatic MIMO radar[J]. Signal Processing, 2019, 157: 88–96. doi: 10.1016/j.sigpro.2018.11.016 [4] HU Bin, SONG Zuxun, and ZHANG Linxi. Fast and efficient time-reversal imaging using space-frequency propagator method[J]. IEEE Transactions on Signal Processing, 2020, 68: 2077–2086. doi: 10.1109/TSP.2020.2981672 [5] AHMED T, ZHANG Xiaofei, and HASSAN W U. A higher-order propagator method for 2D-DOA estimation in massive MIMO systems[J]. IEEE Communications Letters, 2020, 24(3): 543–547. doi: 10.1109/LCOMM.2019.2960341 [6] FORSTER P, GINOLHAC G, and BOIZARD M. Derivation of the theoretical performance of a tensor MUSIC algorithm[J]. Signal Processing, 2016, 129: 97–105. doi: 10.1016/j.sigpro.2016.05.033 [7] HALAY N and TODROS K. MSE based optimization of the measure-transformed MUSIC algorithm[J]. Signal Processing, 2019, 160: 150–163. doi: 10.1016/j.sigpro.2019.01.025 [8] WONG K T and ZOLTOWSKI M D. Uni-vector-sensor ESPRIT for multisource azimuth, elevation, and polarization estimation[J]. IEEE Transactions on Antennas and Propagation, 1997, 45(10): 1467–1474. doi: 10.1109/8.633852 [9] KHAN S and WONG K T. A six-component vector sensor comprising electrically Long dipoles and Large loops—To simultaneously estimate incident sources’ directions-of-arrival and polarizations[J]. IEEE Transactions on Antennas and Propagation, 2020, 68(8): 6355–6363. doi: 10.1109/TAP.2020.2988980 [10] CHINTAGUNTA S and PONNUSAMY P. 2D-DOD and 2D-DOA estimation using the electromagnetic vector sensors[J]. Signal Processing, 2018, 147: 163–172. doi: 10.1016/j.sigpro.2018.01.025 [11] 郑桂妹, 陈伯孝, 吴渤. 三正交分离式极化敏感阵列的波达方向估计[J]. 电子与信息学报, 2014, 36(5): 1088–1093. doi: 10.3724/SP.J.1146.2013.00967ZHENG Guimei, CHEN Baixiao, and WU Bo. DOA estimation with three orthogonally oriented and spatially spread polarization sensitive array[J]. Journal of Electronics &Information Technology, 2014, 36(5): 1088–1093. doi: 10.3724/SP.J.1146.2013.00967 [12] YUAN Xin, WONG K T, XU Zixin, et al. Various compositions to form a triad of collocated dipoles/loops, for direction finding and polarization estimation[J]. IEEE Sensors Journal, 2012, 12(6): 1763–1771. doi: 10.1109/JSEN.2011.2179532 [13] COSTA M, RICHTER A, and KOIVUNEN V. DOA and polarization estimation for arbitrary array configurations[J]. IEEE Transactions on Signal Processing, 2012, 60(5): 2330–2343. doi: 10.1109/TSP.2012.2187519 [14] SHI Shuli, XU Yougen, ZHUANG Junpeng, et al. Tri-polarized sparse array design for mutual coupling reduction in direction finding and polarization estimation[J]. Electronics, 2019, 8(12): 1557. doi: 10.3390/electronics8121557 [15] KHAN S and WONG K T. Electrically long dipoles in a crossed pair for closed-form estimation of an incident source’s polarization[J]. IEEE Transactions on Antennas and Propagation, 2019, 67(8): 5569–5581. doi: 10.1109/TAP.2019.2916581 [16] EL KORSO M N, BOYER R, RENAUX A, et al. Statistical resolution limit of the uniform linear cocentered orthogonal loop and dipole array[J]. IEEE Transactions on Signal Processing, 2011, 59(1): 425–431. doi: 10.1109/TSP.2010.2083657 [17] 张贤达. 矩阵分析与应用[M]. 2版. 北京: 清华大学出版社, 2013.ZHANG Xianda. Matrix Analysis and Applications[M]. 2nd ed. Beijing: Tsinghua University Press, 2013. [18] 张涛, 宋婷, 李晓明, 等. 弯曲阵面机载共形阵列雷达方向图与杂波相关性研究[J]. 电子与信息学报, 2021, 43(3): 572–579. doi: 10.11999/JEIT200572ZHANG Tao, SONG Ting, LI Xiaoming, et al. Research on the relation between the beampattern and clutter distribution of curved surface array for airborne conformal array radar[J]. Journal of Electronics &Information Technology, 2021, 43(3): 572–579. doi: 10.11999/JEIT200572 [19] LIU Chao, LI Chuan, and YANG Bo. Improved alternating projection algorithm for pattern synthesis with dual polarised conformal arrays[J]. IET Microwaves, Antennas & Propagation, 2020, 14(9): 891–896. doi: 10.1049/iet-map.2019.0941 [20] 王布宏, 郭英, 王永良, 等. 共形天线阵列流形的建模方法[J]. 电子学报, 2009, 37(3): 481–484. doi: 10.3321/j.issn:0372-2112.2009.03.010WANG Buhong, GUO Ying, WANG Yongliang, et al. Array manifold modeling for conformal array antenna[J]. Acta Electronica Sinica, 2009, 37(3): 481–484. doi: 10.3321/j.issn:0372-2112.2009.03.010 [21] 徐友根, 刘志文, 龚晓峰. 极化敏感阵列信号处理[M]. 北京: 北京理工大学出版社, 2013.XU Yougen, LIU Zhiwen, and GONG Xiaofeng. Polarization Sensitive Array Signal Processing[M]. Beijing: Beijing Institute of Technology Press, 2013. [22] SHANG Fang, KISHI N, and HIROSE A. Degree of polarization-based data filter for fully polarimetric synthetic aperture radar[J]. IEEE Transactions on Geoscience and Remote Sensing, 2019, 57(6): 3767–3777. doi: 10.1109/TGRS.2018.2887102 [23] JIN K H and YE J C. Sparse and low-rank decomposition of a hankel structured matrix for impulse noise removal[J]. IEEE Transactions on Image Processing, 2018, 27(3): 1448–1461. doi: 10.1109/TIP.2017.2771471 [24] TAN Jun and NIE Zaiping. Polarisation smoothing generalised MUSIC algorithm with PSA monostatic MIMO radar for low angle estimation[J]. Electronics Letters, 2018, 54(8): 527–529. doi: 10.1049/el.2017.4378 -
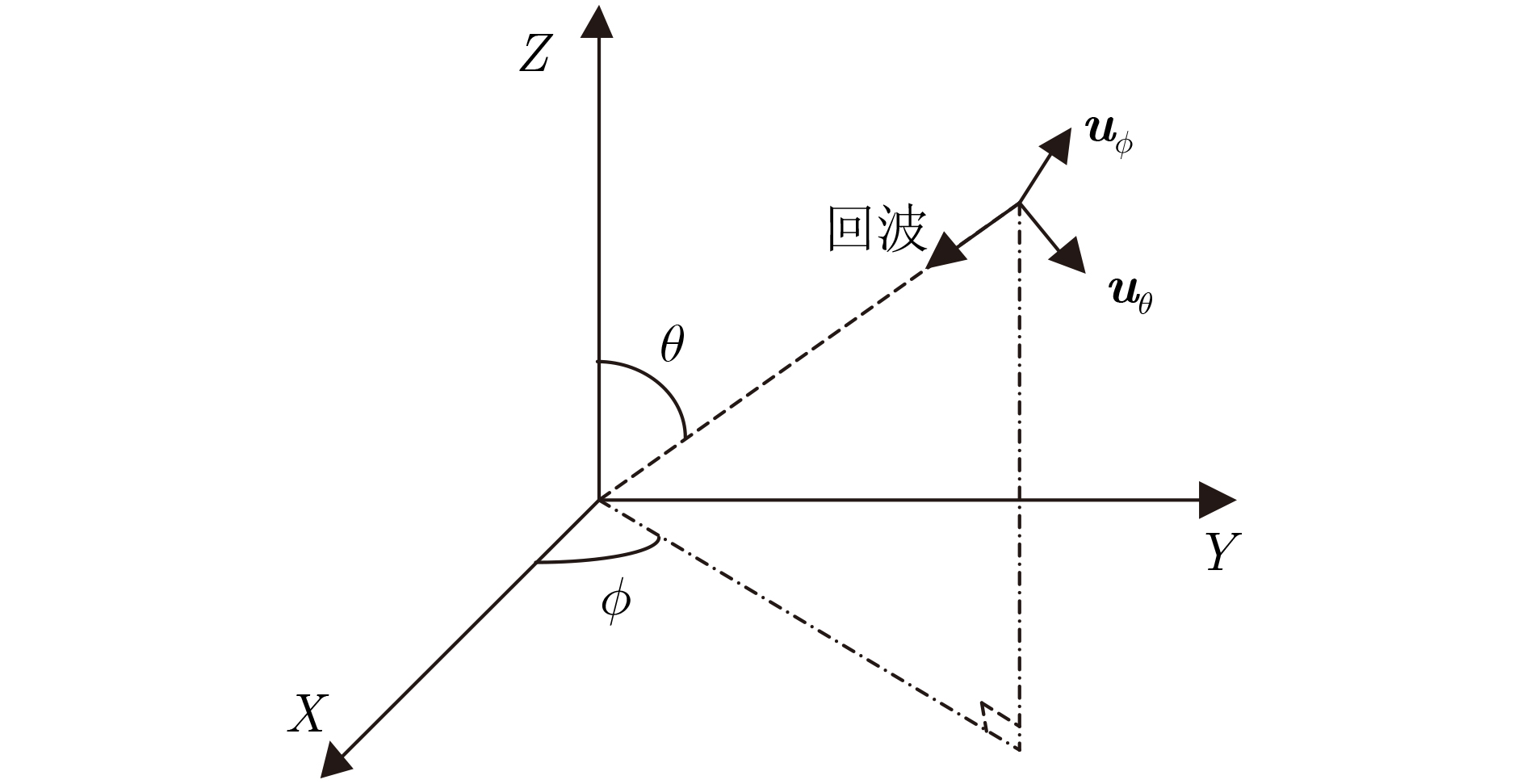
下载:
图(8)
计量
- 文章访问数: 541
- HTML全文浏览量: 207
- PDF下载量: 71
- 被引次数: 0
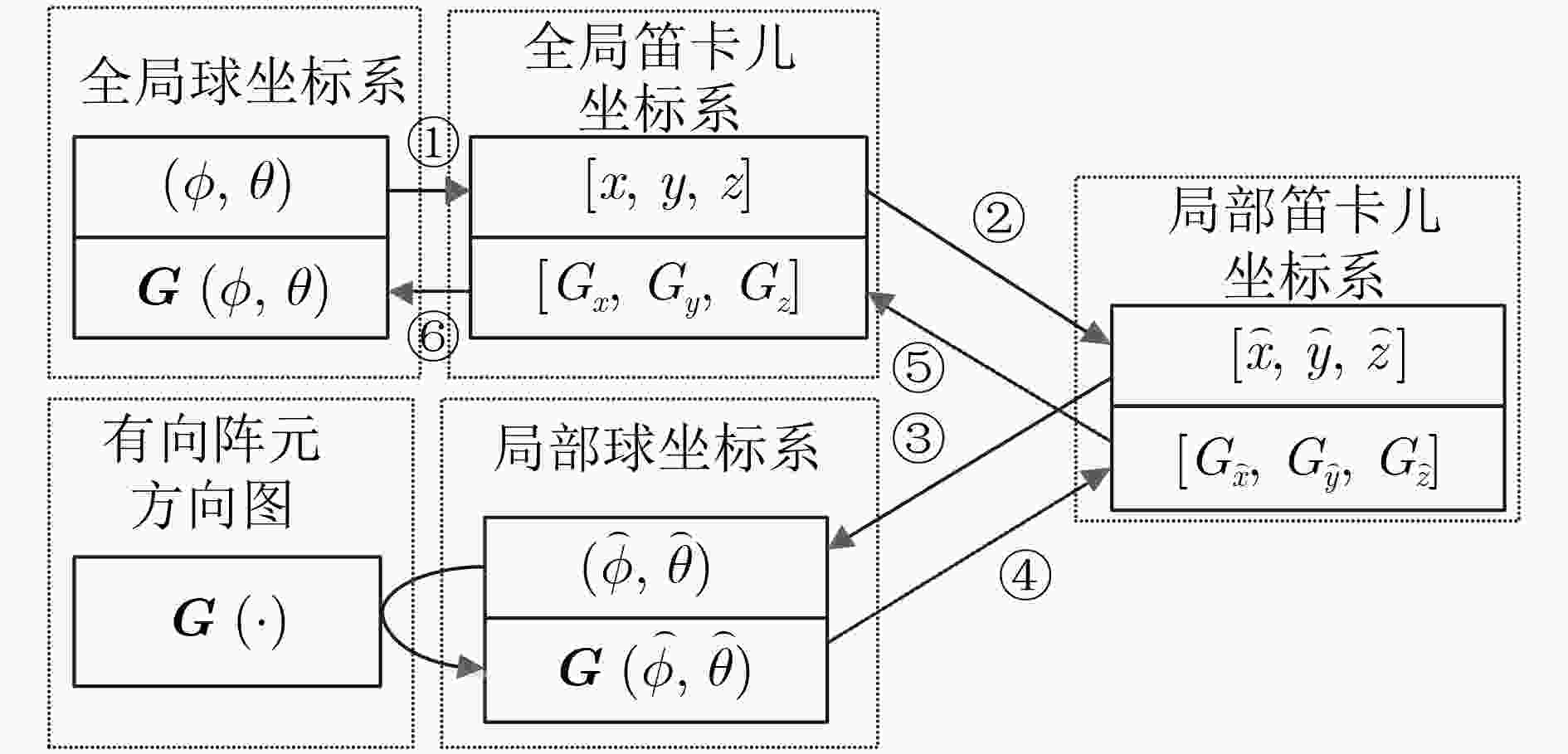
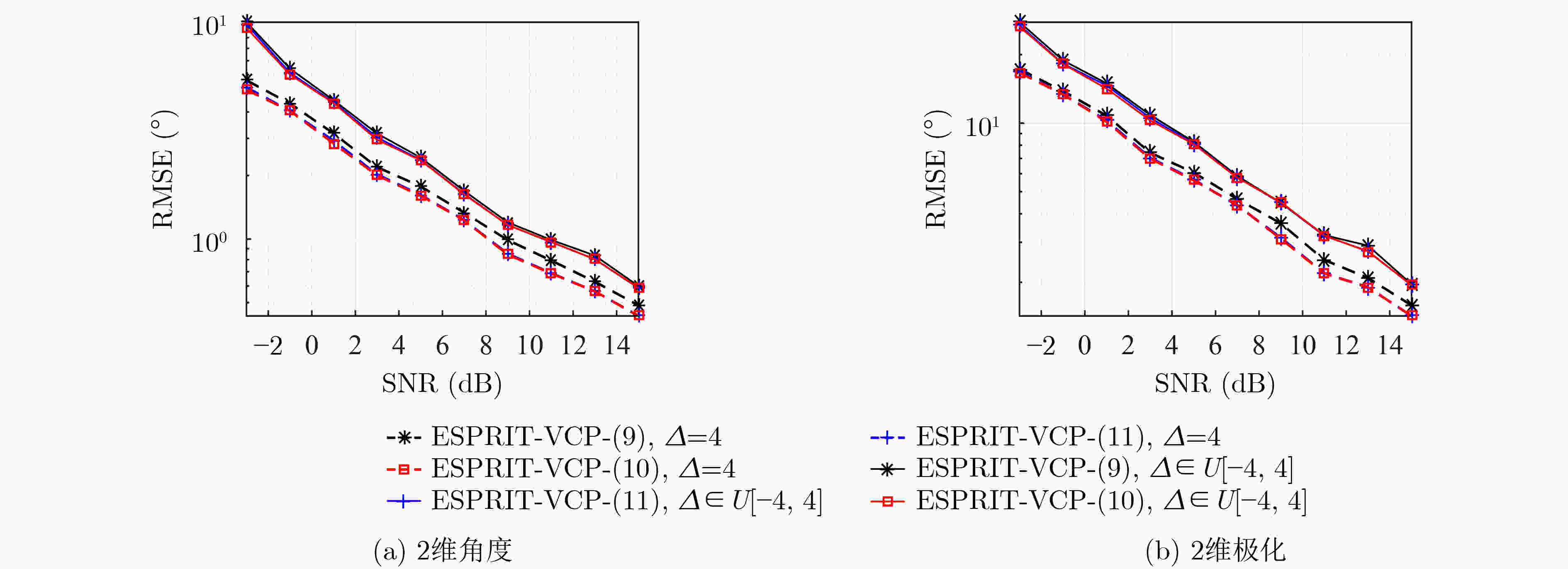
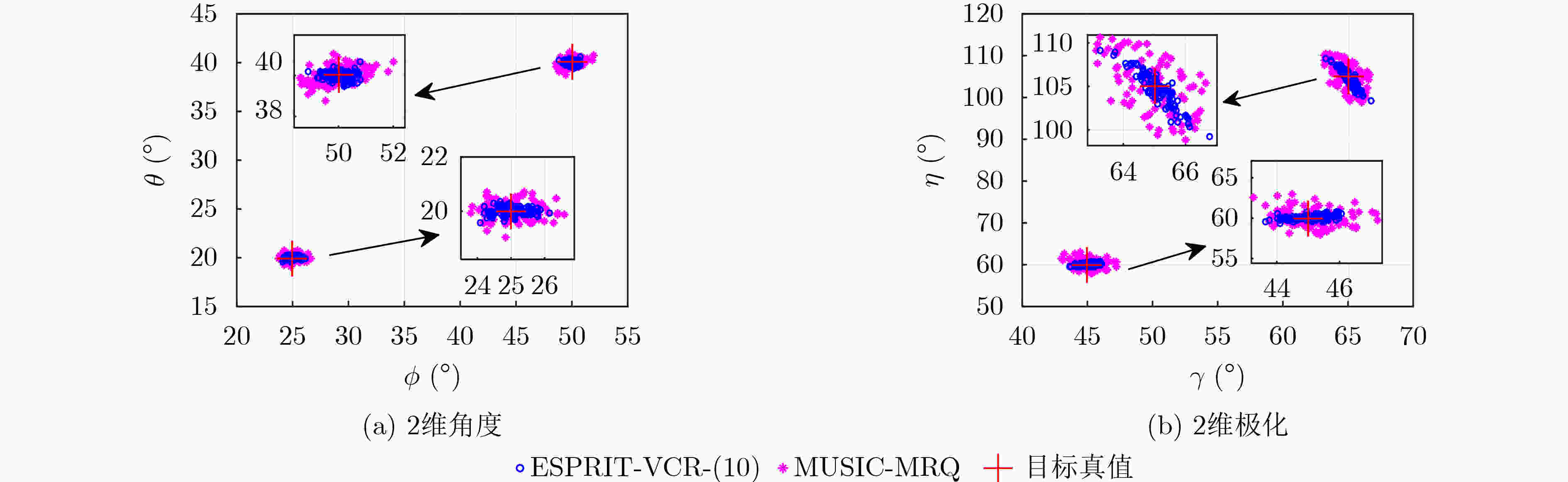
下载:
