三维数据关联情况下外辐射源雷达多目标跟踪研究

李晓花; 李亚安; 金海燕; 鲁晓锋

doi:10.11999/JEIT210620

三维数据关联情况下外辐射源雷达多目标跟踪研究

doi: 10.11999/JEIT210620

李晓花^{1, 2, ,},
李亚安³,
金海燕^{1, 2},
鲁晓锋^{1, 2}

1.
西安理工大学计算机科学与工程学院西安 710048
2.
陕西省网络计算与安全技术重点实验室西安 710048
3.
西北工业大学航海学院西安 710072

基金项目: 国家自然科学基金(61703333, U1934222)，陕西省自然科学基础研究计划(2019JQ-746, 18JK0557)，陕西省重点实验室项目(20JS088)，西安市碑林区科技计划项目 (GX2017)

详细信息

作者简介:
李晓花：女，1986年生，博士，讲师，研究方向为多目标跟踪，多传感器信息融合

李亚安：男，1961年生，博士，教授，研究方向为目标定位与跟踪，特征提取与分类

金海燕：女，1976年生，博士，教授，博士生导师，研究方向为机器学习，目标优化，智能信息处理

鲁晓锋：男，1976年生，博士，副教授，硕士生导师，研究方向为视觉目标检测与跟踪，深度学习

通讯作者:
李晓花　lixiaohua@xaut.edu.cn

中图分类号: TN957
计量
- 文章访问数: 742
- HTML全文浏览量: 274
- PDF下载量: 75
- 被引次数: 0
出版历程
- 收稿日期: 2021-06-22
- 修回日期: 2021-08-16
- 网络出版日期: 2021-08-30
- 刊出日期: 2021-10-18

Multistatic Passive Radar Multi-target Tracking Under Target-measurement-illuminator Data Association Uncertainty

Xiaohua LI^{1, 2
, ,},
Ya’an LI³,
Haiyan JIN^{1, 2},
Xiaofeng LU^{1, 2}

1.
School of Computer and Engineering, Xi’an University of Technology, Xi’an 710048, China
2.
Shaanxi Key Laboratory for Network Computing and Security Technology, Xi’an 710048, China
3.
School of Marine Science and Technology, Northwestern Polytechnical University, Xi’an, 710072, China

Funds: The National Natural Science Foundation of China (61703333, U1934222), The Natural Science Basic Research Program of Shaanxi Province (2019JQ-746 and 18JK0557), The Kay Laboratory of Shaanxi Provincial Department of Education (20JS088), The Science and Technology Project of Beilin District (GX2017)

摘要

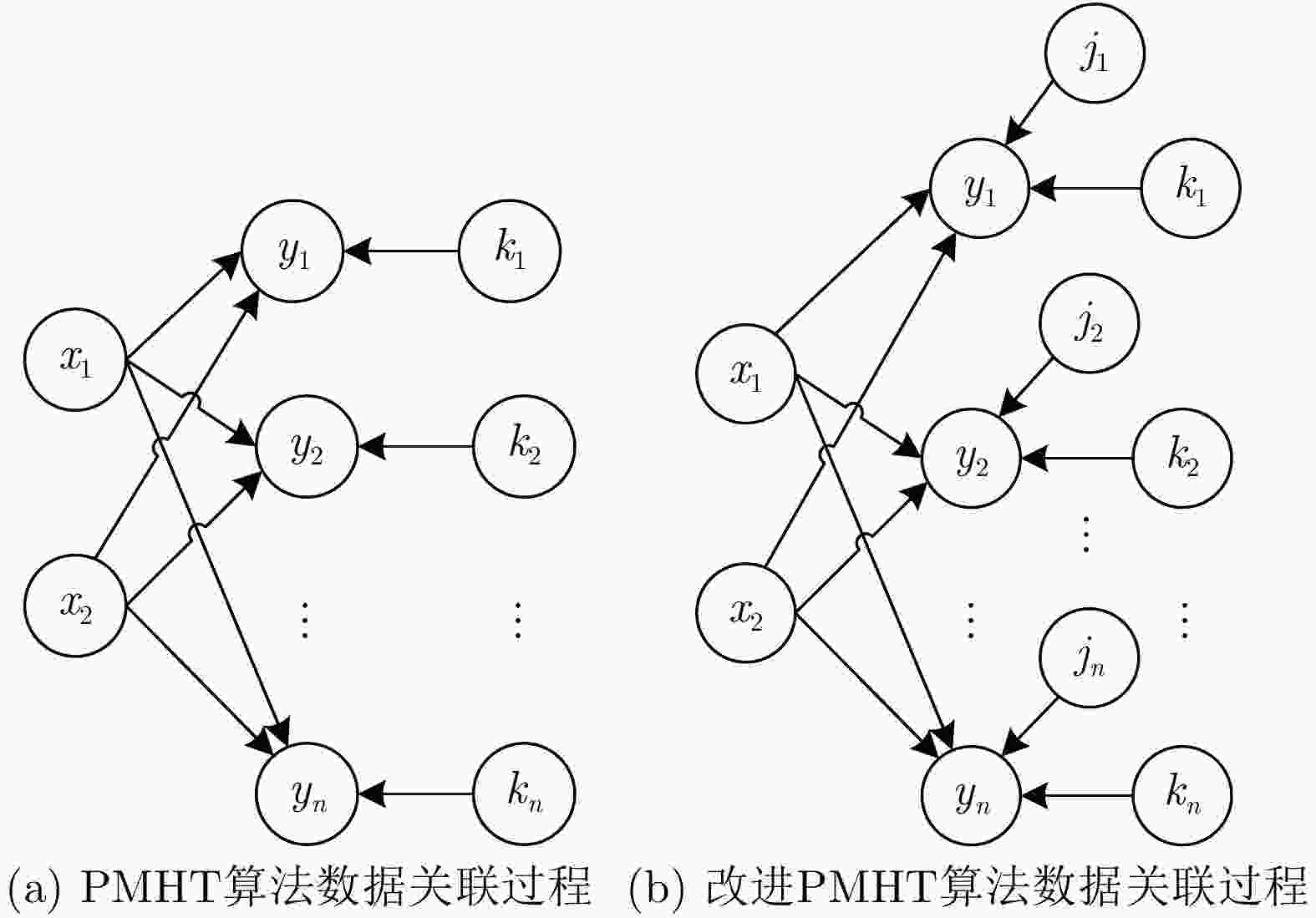
摘要: 不同于传统多目标跟踪，除了量测-目标数据关联模糊问题外，外辐射源雷达跟踪系统新增了量测-发射机数据关联模糊问题。针对此问题，该文通过引入一个新的关联变量来表示量测和发射机之间的数据关联关系，提出了目标-量测-发射机3维数据关联改进概率多假设跟踪(PMHT)算法。该算法利用期望极大化(EM)算法的独立性假设条件得到最大后验概率意义下的最优跟踪。为了增加目标-量测-发射机之间数据关联的准确性，提高多目标与量测后验关联概率的精确度，将量测信息设定为均值相同协方差不同的混合高斯分布。针对距离-多普勒量测的非线性性，利用无味卡尔曼平滑(UKS)算法进行多目标状态估计。仿真结果表明，对于FKIE外辐射源雷达数据集(杂波密度很高)，所提算法的目标与航迹关联成功率高，抗杂波性能强，证明了算法的有效性。
- 外辐射源雷达 /
- 多目标跟踪 /
- 概率多假设跟踪 /
- 无味卡尔曼平滑算法 /
- 数据关联
Abstract: Different from the traditional multi-target tracking problem which has the measurements to targets data association uncertainty problem, the multistatic passive radar multi-target tacking system has the additional measurements to illuminators data association uncertainty problem, which means the data association relationship is three dimensional. A novel target-measurement-illuminator Probabilistic Multiple Hypothesis Tracking (PMHT) algorithm is proposed, which introduces a new data association variable to represent the data association relationship. The proposed algorithm is based on the Expectation-Maximization (EM). To handle the nonlinear problem of range-Doppler measurements, the Unscented Kalman Smoother (UKS) is used to get the multi-targets’ estimated states. To increase the data association accuracy, the measurements are set to mixture Gaussian distribution. Simulation results show that for the FKIE passive radar data set, the proposed algorithm can track multi-targets effectively in dense clutter environment.
- Multistatic passive radar /
- Multi-target tracking /
- Probabilistic Multiple Hypothesis Tracking (PMHT) /
- Unscented Kalman Smoother (UKS) /
- Data association

HTML全文

图 1 PMHT算法和改进PMHT算法数据关联过程示意图

下载: 全尺寸图片幻灯片

图 2 基于距离-多普勒的多目标跟踪真实轨迹和本文算法的估计轨迹

下载: 全尺寸图片幻灯片

图 3 密集杂波情况下距离-多普勒量测和所提算法的合成量测，以及无杂波情况下的多目标量测值

下载: 全尺寸图片幻灯片

图 4 本文算法对10个目标的位置均方根误差，3维数据关联关系未知

下载: 全尺寸图片幻灯片

图 5 PMHT算法对10个目标的位置均方根误差，3维数据关联关系未知

下载: 全尺寸图片幻灯片

图 6 10个目标的平均归一化估计误差平方ANEES

下载: 全尺寸图片幻灯片

表 1 目标初始位置和初始速度

目标	初始位置(m)	初始速度(m/s)	目标	初始位置(m)	初始速度(s/m)
1	(–55000, –3498, 890)	(200, 10, 0)	6	(5000, –25000, 1890)	(–20, –160, 0)
2	(–52500, 12562, 890)	(200, –90, 0)	7	(5000, –25000, 1890)	(120, –160, 0)
3	(–50000, 33000, 1890)	(–200, –100, 0)	8	(43500, 25000, 1890)	(–100, –200, 0)
4	(30500, 53000, 890)	(–100, –200, 0)	9	(51000, 23000, 1890)	(–200, –100, 0)
5	(–30000, –110000, 1890)	(160, 100, 0)	10	(10000, –20000, 1890)	(80, –190, 0)

下载: 导出CSV

表 2 本文算法和PMHT算法速度平均均方根误差(m/s)

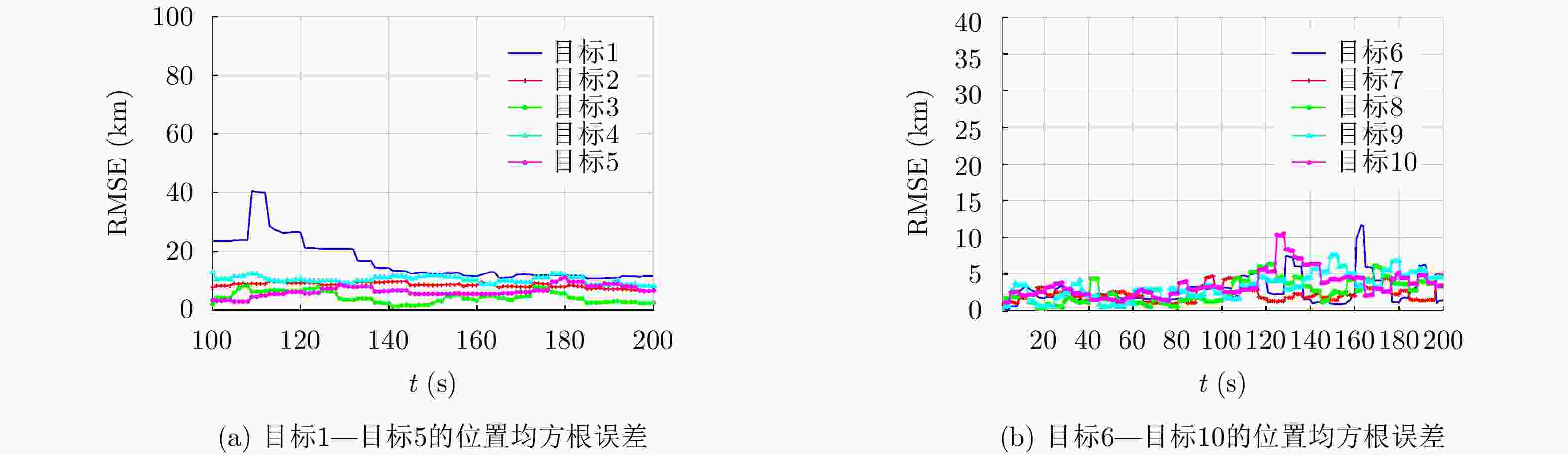
目标	1	2	3	4	5	6	7	8	9	10
本文算法 PMHT算法	0.973 4.273	0.897 7.993	1.616 4.042	1.045 16.33	1.602 7.518	3.452 2.784	5.683 2.237	1.915 2.614	1.955 3.205	4.101 3.478

下载: 导出CSV

表 3 采样200步的跟踪运行时间对比(s)

EM迭代次数	4	5	6	7	8
本文算法 PMHT	58 56	72 73	87 85	101 100	114 113

下载: 导出CSV

表 4 10个目标的平均归一化估计误差平方(ANEES)

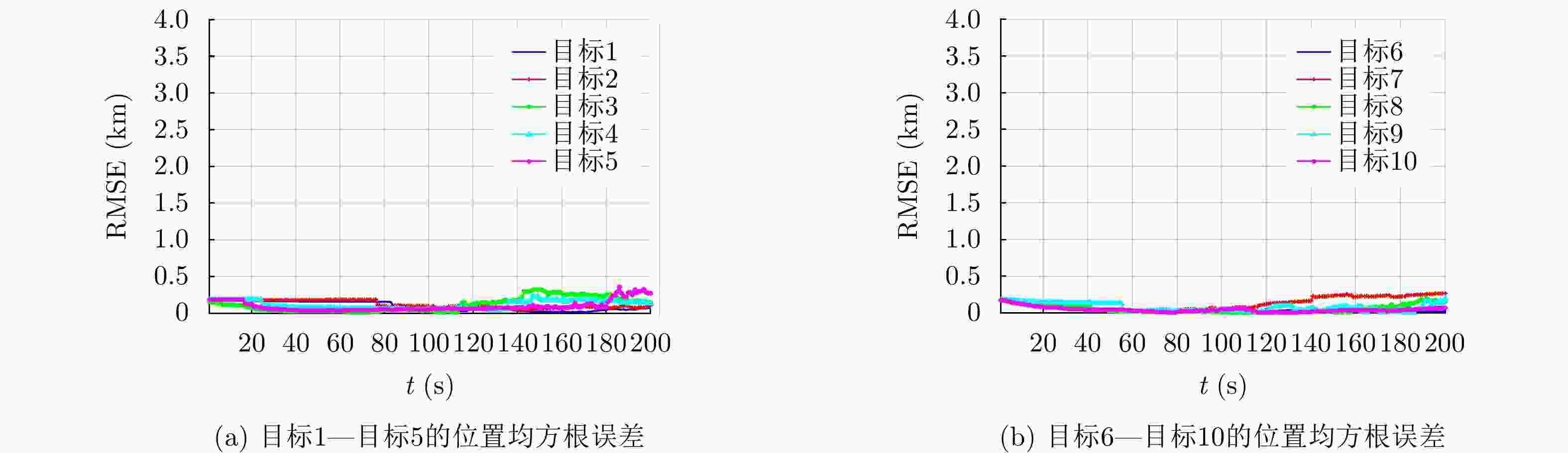
目标	1	2	3	4	5	6	7	8	9	10
ANEES	4.658	4.261	3.951	3.890	4.953	4.389	4.538	3.964	3.480	4.365

下载: 导出CSV

参考文献(29)

[1]	RASHID M and NARAGHI-POUR M. Multi-target delay and Doppler estimation in bistatic passive radar systems[C]. 2021 IEEE Radar Conference, Atlanta, USA, 2021: 1–6.
[2]	JING Tong, TIAN Wei, HUANG Gaoming, et al. Observability and estimability of passive radar with unknown illuminator states using different observations[J]. Journal of Systems Engineering and Electronics, 2020, 31(6): 1193–1205. doi: 10.23919/JSEE.2020.000092
[3]	左燕, 蒋陶然, 陈志猛, 等. 误差校正下单站多外辐射源BR/BRR多目标定位算法[J]. 电子与信息学报, 2021, 43(4): 1162–1169. doi: 10.11999/JEIT200042 ZUO Yan, JIANG Taoran, CHEN Zhimeng, et al. BR/BRR passive localization and registration for multiple moving targets in single-observer multi-illuminator radar systems[J]. Journal of Electronics &Information Technology, 2021, 43(4): 1162–1169. doi: 10.11999/JEIT200042
[4]	黄东华, 赵勇胜, 赵拥军, 等. 基于DOA-TDOA-FDOA的单站无源相干定位代数解[J]. 电子与信息学报, 2021, 43(3): 735–744. doi: 10.11999/JEIT200470 HUANG Donghua, ZHAO Yongsheng, ZHAO Yongjun, et al. An algebraic solution for single-observer passive coherent location using DOA-TDOA-FDOA measurements[J]. Journal of Electronics &Information Technology, 2021, 43(3): 735–744. doi: 10.11999/JEIT200470
[5]	KONOVALOV A A. Target tracking algorithm for passive coherent location[J]. IET Radar, Sonar & Navigation, 2016, 10(7): 1228–1233.
[6]	AKHTAR A, YONEL B, and YAZICI B. Passive multistatic radar imaging with prior information[C]. 2021 IEEE Radar Conference, Atlanta, USA, 2021: 1–6.
[7]	GUO Yunfei, THARMARASA R, KIRUBARAJAN T, et al. Passive coherent location with unknown transmitter states[J]. IEEE Transactions on Aerospace and Electronic Systems, 2017, 53(1): 148–168. doi: 10.1109/TAES.2017.2649739
[8]	万显荣, 刘玉琪, 程丰, 等. 基于信道分段平滑的外辐射源雷达非平稳杂波抑制方法[J]. 电子与信息学报, 2020, 42(1): 132–139. doi: 10.11999/JEIT190754 WAN Xianrong, LIU Yuqi, CHENG Feng, et al. Nonstationary clutter suppression method for passive radar based on channel segmentation and smoothing[J]. Journal of Electronics &Information Technology, 2020, 42(1): 132–139. doi: 10.11999/JEIT190754
[9]	YI Jianxin, WAN Xianrong, CHENG Feng, et al. Deghosting for target tracking in single frequency network based passive radar[J]. IEEE Transactions on Aerospace and Electronic Systems, 2015, 51(4): 2655–2668. doi: 10.1109/TAES.2015.130424
[10]	CHOI S, CROUSE D F, WILLETT P, et al. Approaches to Cartesian data association passive radar tracking in a DAB/DVB network[J]. IEEE Transactions on Aerospace and Electronic Systems, 2014, 50(1): 649–663. doi: 10.1109/TAES.2013.120431
[11]	BOZDOGAN A O, SOYSAL G, and EFE M. Multistatic tracking using bistatic range-range rate measurements[C]. The 12th International Conference on Information Fusion, Seattle, USA, 2009: 2107–2113.
[12]	DEMING R, SCHINDLER J, and PERLOVSKY L. Multi-target/multi-sensor tracking using only range and Doppler measurements[J]. IEEE Transactions on Aerospace and Electronic Systems, 2009, 45(2): 593–611. doi: 10.1109/TAES.2009.5089543
[13]	吴伟, 王东进, 陈卫东. 基于动态多维分配的多基地雷达多目标跟踪算法[J]. 中国科学技术大学学报, 2006, 36(11): 1143–1147, 1152. doi: 10.3969/j.issn.0253-2778.2006.11.003 WU Wei, WANG Dongjin, and CHEN Weidong. Multi-target tracking algorithm for multi-static radar based on dynamic multi-dimension assignment[J]. Journal of University of Science and Technology of China, 2006, 36(11): 1143–1147, 1152. doi: 10.3969/j.issn.0253-2778.2006.11.003
[14]	SANTHI L R, GOPI S, and JOHN A. Adaptive switching between JPDA and set JPDA algorithms based on validation gates[C]. 2015 International Conference on Control Communication & Computing, Trivandrum, India, 2015: 42–47.
[15]	ZHENG Le and WANG Xiaodong. Improved multiple hypothesis tracker for joint multiple target tracking and feature extraction[J]. IEEE Transactions on Aerospace and Electronic Systems, 2019, 55(6): 3080–3089. doi: 10.1109/TAES.2019.2897035
[16]	DAUN M and KOCH W. Multistatic target tracking for non-cooperative illumination by DAB/DVB-T[C]. 2008 IEEE Radar Conference, Rome, Italy, 2008.
[17]	DAUN M, NICKEL U, and KOCH W. Tracking in multistatic passive radar systems using DAB/DVB-T illumination[J]. Signal Processing, 2012, 92(6): 1365–1386. doi: 10.1016/j.sigpro.2011.09.005
[18]	TOBIAS M and LANTERMAN A D. Probability hypothesis density-based multitarget tracking with bistatic range and Doppler observations[J]. IEE Proceedings-Radar, Sonar and Navigation, 2005, 152(3): 195–205. doi: 10.1049/ip-rsn:20045031
[19]	PIKORA K and EHLERS F. Analysis of the FKIE passive radar data set with GMPHD and GMCPHD[C]. The 16th International Conference on Information Fusion, Istanbul, Turkey, 2013: 272–279.
[20]	杨威, 付耀文, 龙建乾, 等. 基于有限集统计学理论的目标跟踪技术研究综述[J]. 电子学报, 2012, 40(7): 1440–1448. YANG Wei, FU Yaowen, LONG Jianqian, et al. The FISST-based target tracking techniques: A survey[J]. Acta Electronica Sinica, 2012, 40(7): 1440–1448.
[21]	GAETJENS H X, DAVEY S J, and LUGINBUHL T E. A tale of three priors[J]. IEEE Transactions on Aerospace and Electronic Systems, 2021, 57(3): 1633–1645. doi: 10.1109/TAES.2021.3054057
[22]	杨威, 付耀文, 潘晓刚, 等. 弱目标检测前跟踪技术研究综述[J]. 电子学报, 2014, 42(9): 1786–1793. doi: 10.3969/j.issn.0372-2112.2014.09.019 YANG Wei, FU Yaowen, PAN Xiaogang, et al. Track-before-detect technique for dim targets: An overview[J]. Acta Electronica Sinica, 2014, 42(9): 1786–1793. doi: 10.3969/j.issn.0372-2112.2014.09.019
[23]	DAVEY S J. Efficient histogram PMHT via single target Chip processing[J]. IEEE Signal Processing Letters, 2015, 22(5): 569–572. doi: 10.1109/LSP.2014.2364300
[24]	LI Xiaohua, WILLETT P, BAUM M, et al. PMHT approach for underwater bearing-only multisensor-multitarget tracking in clutter[J]. IEEE Journal of Oceanic Engineering, 2016, 41(4): 831–839. doi: 10.1109/JOE.2015.2506220
[25]	LEXA M, CORALUPPI S, CARTHEL C, et al. Distributed MHT and ML-PMHT approaches to multi-sensor passive sonar tracking[C]. 2020 IEEE Aerospace Conference, Big Sky, USA, 2020: 1–12.
[26]	王磊, 程向红, 李双喜. 高斯和高阶无迹卡尔曼滤波算法[J]. 电子学报, 2017, 45(2): 424–430. doi: 10.3969/j.issn.0372-2112.2017.02.022 WANG Lei, CHENG Xianghong, and LI Shuangxi. Gaussian sum high order unscented Kalman filtering algorithm[J]. Acta Electronica Sinica, 2017, 45(2): 424–430. doi: 10.3969/j.issn.0372-2112.2017.02.022
[27]	SINGH A K. Major development under Gaussian filtering since unscented Kalman filter[J]. IEEE/CAA Journal of Automatica Sinica, 2020, 7(5): 1308–1325.
[28]	LI X R and ZHAO Zhanlue. Evaluation of estimation algorithms part I: Incomprehensive measures of performance[J]. IEEE Transactions on Aerospace and Electronic Systems, 2006, 42(4): 1340–1358. doi: 10.1109/TAES.2006.314576
[29]	LI X R and ZHAO Zhanlue. Measures of performance for evaluation of estimators and filters[C]. SPIE 4473, Signal and Data Processing of Small Targets 2001, San Diego, USA, 2001: 530–541.