A Novel Image Encryption Algorithm Based on Exponent-cosine Chaotic Mapping
-
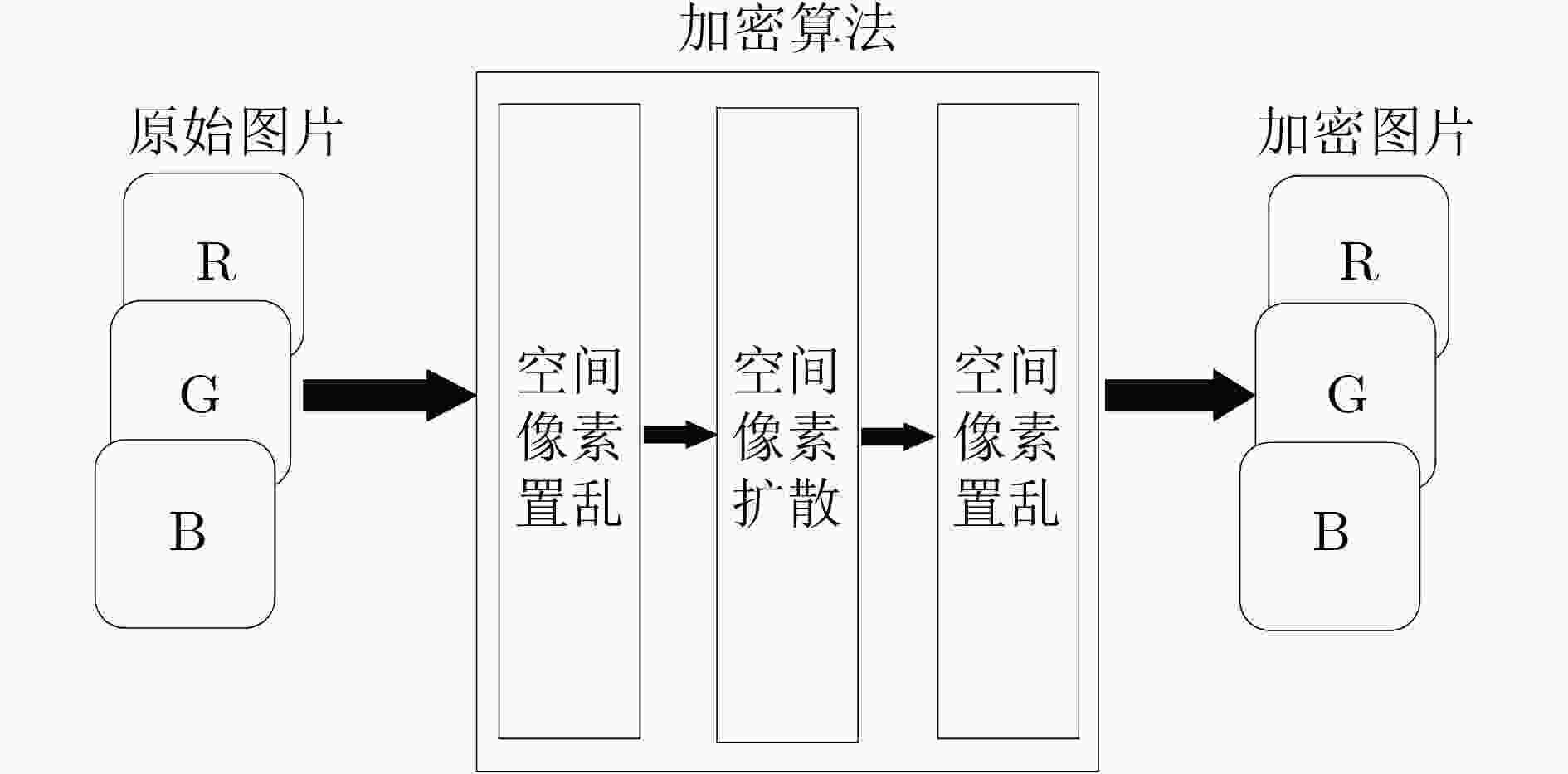
摘要: 为了增强图像数据传输的安全性,该文提出一种新型的2维指数-余弦离散混沌映射系统。该系统通过向1维余弦混沌系统中引入指数和高次幂非线性项来构造新型混沌映射。引入的非线性项对1维余弦混沌系统的迭代过程进行扰动得到更饱满的混沌相轨。利用Lyapunov指数谱、系统分岔图等对该系统的混沌动力学性质进行了验证。基于此混沌映射,该文提出一种新型的混沌图像加密算法。该算法通过“置乱-扩散-置乱”等加密环节,使得加密后的数据具有很好的数据安全性。加密图像数据的安全性分析也表明2维指数-余弦混沌映射具有较强的算法鲁棒性以及加密安全性。Abstract: In order to enhance the security of image data transmission, a novel two-dimensional exponent - cosine chaotic map is proposed. In this system, a new chaotic map is constructed by introducing exponent and high-power nonlinear terms into one dimensional cosine chaotic system. The nonlinear term is introduced to perturb the iterative process of one-dimensional cosine chaotic system to obtain fuller chaotic phase orbits. Lyapunov exponential spectrum and system bifurcation diagram are used to verify the features of chaotic system. Based on the chaotic map, a novel image encryption algorithm is proposed. The encrypted data has good encryption security by following a “scrambling-diffusion-scrambling” strategy. Security analysis of encrypted image data also shows that the two-dimensional exponential - cosine chaotic map has strong robustness and encryption security.
-
表 1 不同参数b对应的李雅普诺夫指数谱及吸引子类型
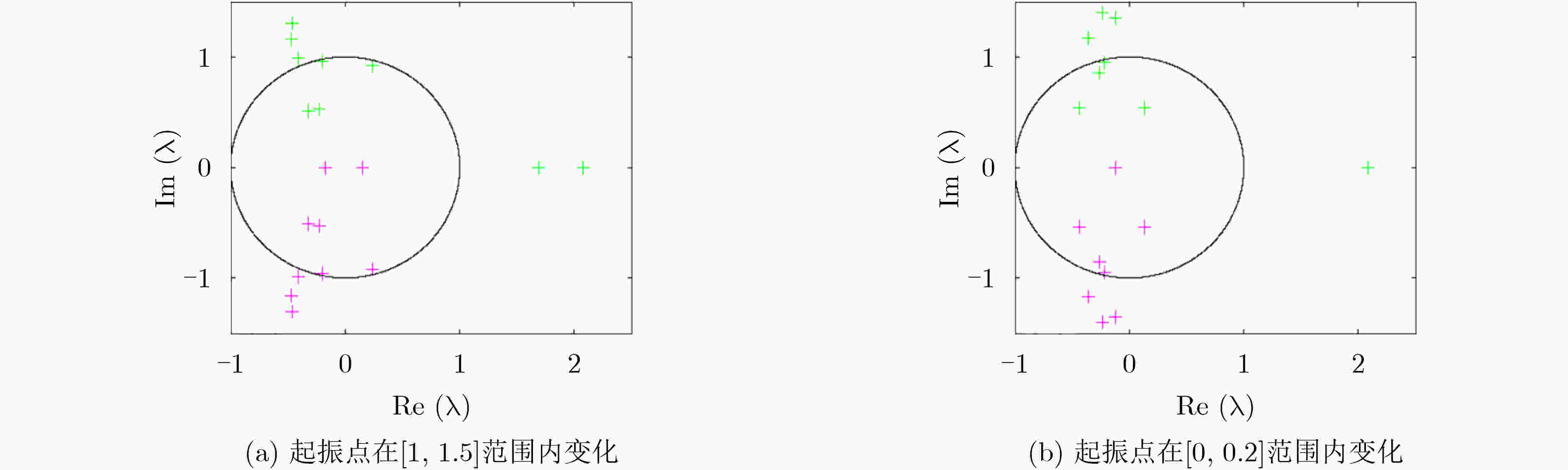
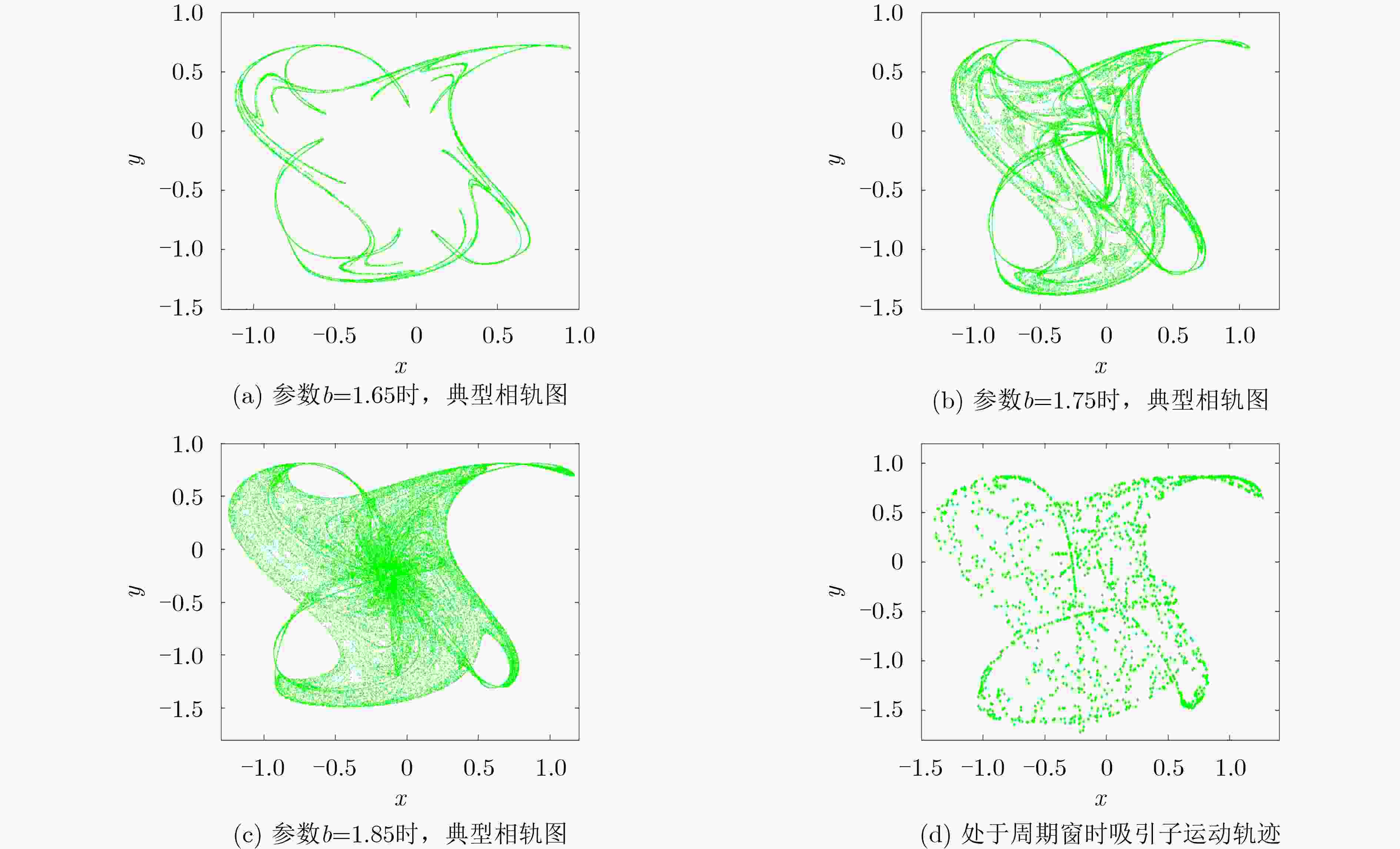
b 李雅普诺夫指数谱 吸引子类型 1.65 0.1301, –0.4619 混沌吸引子 1.75 0.1580, –0.2573 混沌吸引子 1.85 0.1781, –0.2180 混沌吸引子 1.97 –0.1155, –0.1155 离散周期点 表 3 NPCR,UACI参数值(%)
表 4 自相关系数
图像 方位角 原始值 加密后 Boat 左 0.87986 –0.0029753 右 0.87578 0.00099645 上 0.89982 –0.00026347 下 0.89718 –0.00096155 左上 0.81343 –0.00034887 左下 0.82413 –0.00022197 右上 0.83087 0.00025217 右下 0.80362 –2.4882e-05 Cameraman 左 0.91761 0.0006906 右 0.92347 0.00017951 上 0.95272 –0.00039079 下 0.95336 0.00040691 左上 0.88771 –0.00042397 左下 0.90207 3.8675e-05 右上 0.90196 0.00051293 右下 0.88703 0.00053786 Lena 左 0.94044 –0.00029284 右 0.93955 –0.00011737 上 0.96882 0.00014822 下 0.97068 –0.00053991 左上 0.91482 –0.00088227 左下 0.94011 0.00059065 右上 0.93857 –0.00044307 右下 0.91636 1.7261e-05 表 5 图像信息熵
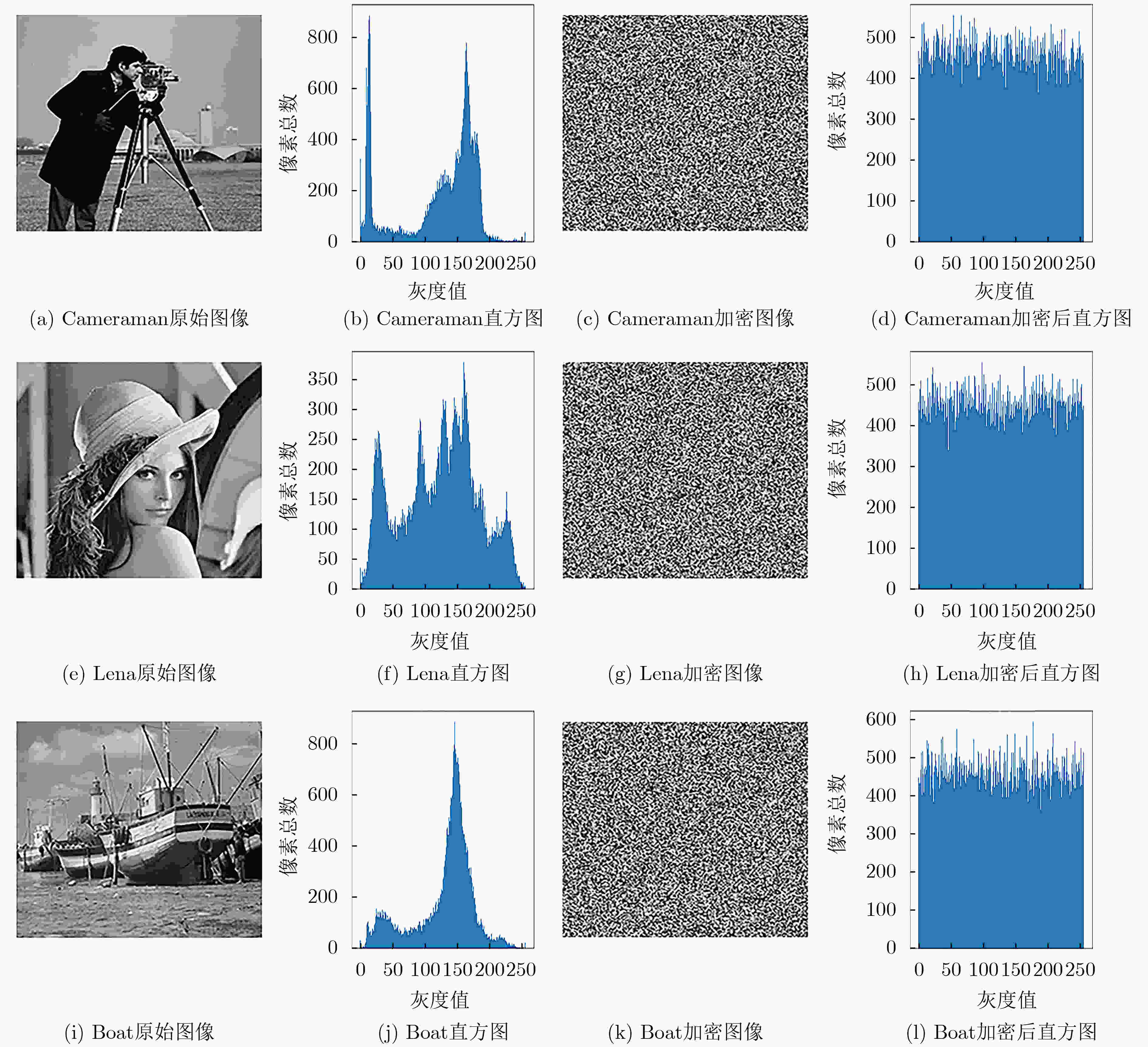
图像 信息熵 Boat 7.9958 Cameraman 7.9944 Lena 7.9951 -
[1] MATTHEWS R. On the derivation of a “Chaotic” encryption algorithm[J]. Cryptologia, 1989, 13(1): 29–42. doi: 10.1080/0161-118991863745 [2] STOYANOV B and KORDOV K. Image encryption using Chebyshev map and rotation equation[J]. Entropy, 2015, 17(4): 2117–2139. doi: 10.3390/e17042117 [3] HUANG Xiaoling. Image encryption algorithm using chaotic chebyshev generator[J]. Nonlinear Dynamics, 2012, 67(4): 2411–2417. doi: 10.1007/s11071-011-0155-7 [4] MURILLO-ESCOBAR M A, CRUZ-HERNÁNDEZ C, ABUNDIZ-PÉREZ F, et al. A RGB image encryption algorithm based on total plain image characteristics and chaos[J]. Signal Processing, 2015, 109: 119–131. doi: 10.1016/j.sigpro.2014.10.033 [5] 吕群, 薛伟. 结合混沌系统和动态S-盒的图像加密算法[J]. 小型微型计算机系统, 2018, 39(3): 607–613. doi: 10.3969/j.issn.1000-1220.2018.03.038LV Qun and XUE Wei. Image encryption algorithm combining chaotic system and dynamic S-boxes[J]. Journal of Chinese Computer Systems, 2018, 39(3): 607–613. doi: 10.3969/j.issn.1000-1220.2018.03.038 [6] HUA Zhongyun and ZHOU Yicong. One-dimensional nonlinear model for producing chaos[J]. IEEE Transactions on Circuits and Systems I:Regular Papers, 2018, 65(1): 235–246. doi: 10.1109/TCSI.2017.2717943 [7] ZHOU Yicong, BAO Long, and CHEN C L P. Image encryption using a new parametric switching chaotic system[J]. Signal Processing, 2013, 93(11): 3039–3052. doi: 10.1016/j.sigpro.2013.04.021 [8] LIU Yang, TONG Xiaojun, and HU Shicheng. A family of new complex number chaotic maps based image encryption algorithm[J]. Signal Processing:Image Communication, 2013, 28(10): 1548–1559. doi: 10.1016/j.image.2013.07.009 [9] HUA Zhongyun and ZHOU Yicong. Image encryption using 2D logistic-adjusted-sine map[J]. Information Sciences, 2016, 339: 237–253. doi: 10.1016/j.ins.2016.01.017 [10] GAN Zhihua, CHAI Xiuli, HAN Daojun, et al. A chaotic image encryption algorithm based on 3-D bit-plane permutation[J]. Neural Computing and Applications, 2019, 31(11): 7111–7130. doi: 10.1007/s00521-018-3541-y [11] YIN Qi and WANG Chunhua. A new chaotic image encryption scheme using breadth-first search and dynamic diffusion[J]. International Journal of Bifurcation and Chaos, 2018, 28(4): 1850047. doi: 10.1142/S0218127418500475 [12] LUO Yuqin, YU Jin, LAI Wenrui, et al. A novel chaotic image encryption algorithm based on improved baker map and logistic map[J]. Multimedia Tools and Applications, 2019, 78(15): 22023–22043. doi: 10.1007/s11042-019-7453-3 [13] KHAN M and MASOOD F. A novel chaotic image encryption technique based on multiple discrete dynamical maps[J]. Multimedia Tools and Applications, 2019, 78(18): 26203–26222. doi: 10.1007/s11042-019-07818-4 [14] YE Guodong, PAN Chen, HUANG Xiaoling, et al. An efficient pixel-level chaotic image encryption algorithm[J]. Nonlinear Dynamics, 2018, 94(1): 745–756. doi: 10.1007/s11071-018-4391-y [15] LIU Lidong, LEI Yuhang, and WANG Dan. A fast chaotic image encryption scheme with simultaneous permutation-diffusion operation[J]. IEEE Access, 2020, 8: 27361–27374. doi: 10.1109/ACCESS.2020.2971759 -





 下载:
下载:

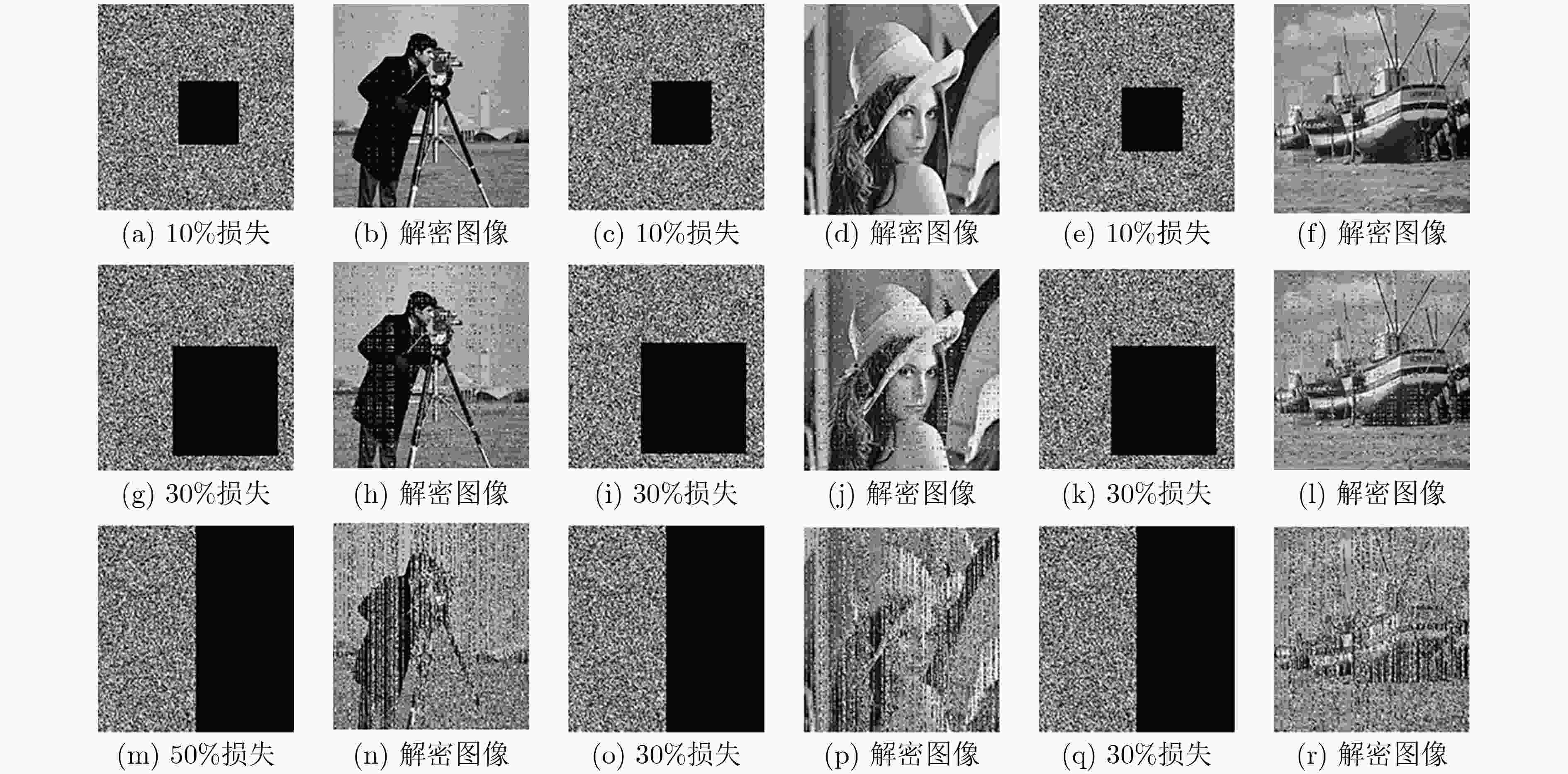
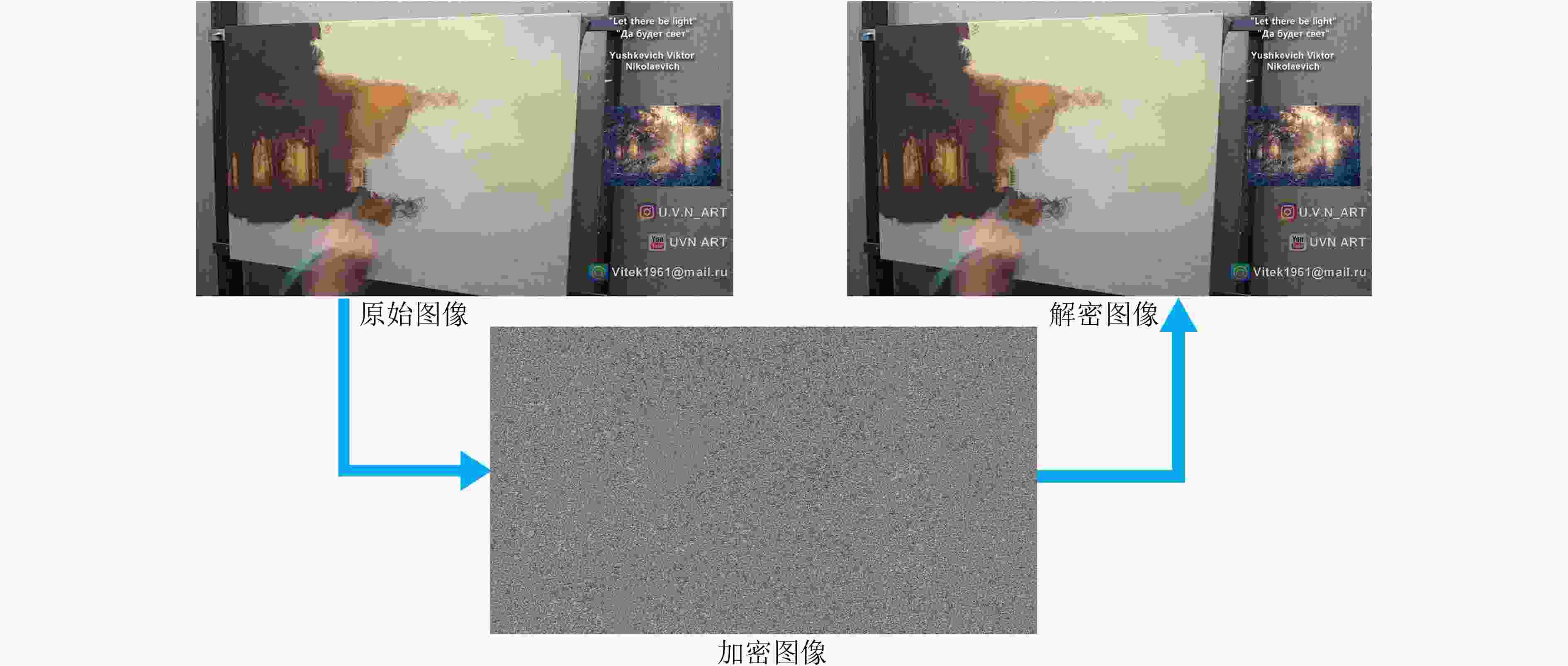


 下载:
下载:
