Multi-carrier Index Modulation Based on Prolate Spheroidal Wave Functions with Dual-mode
-
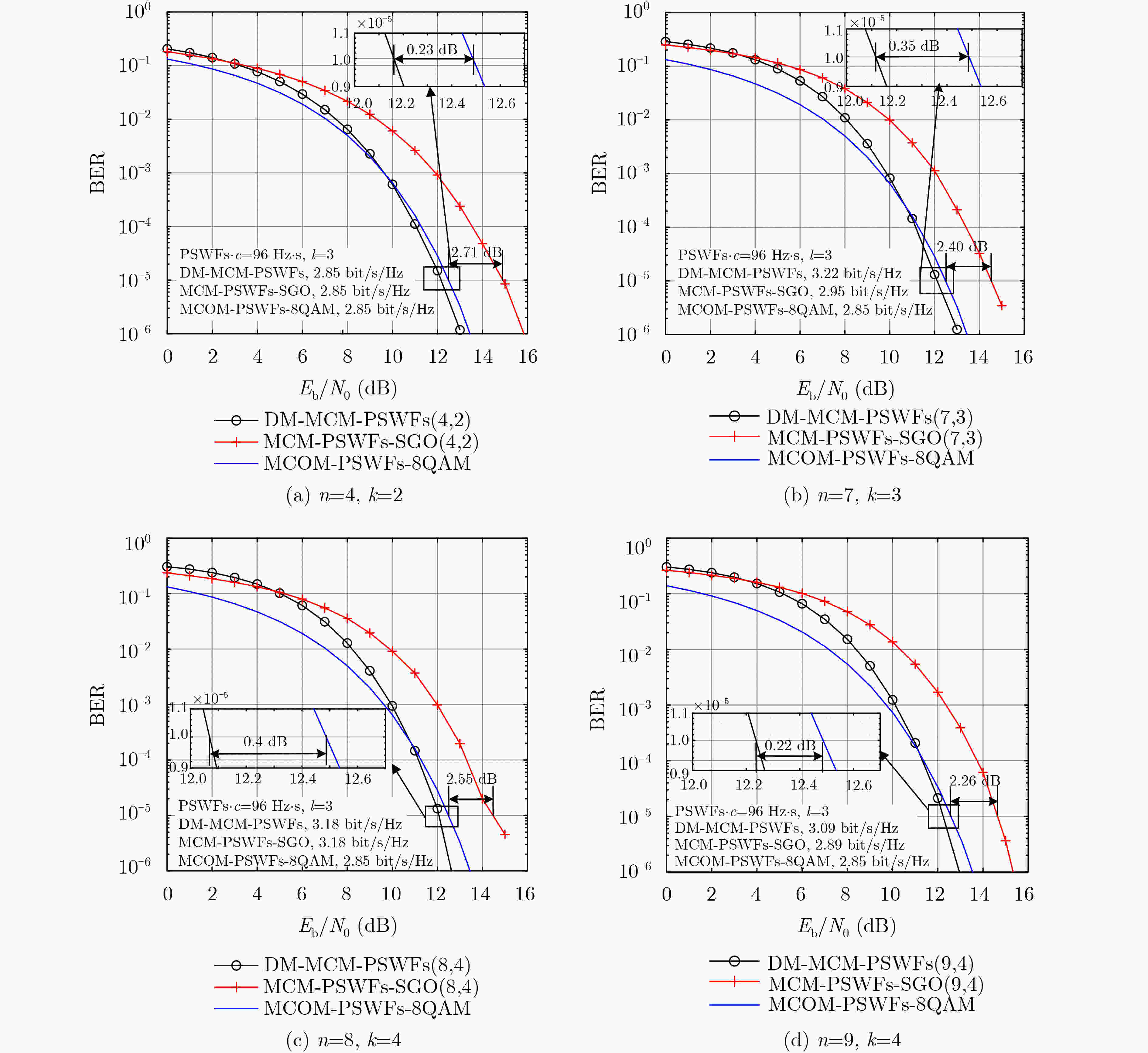
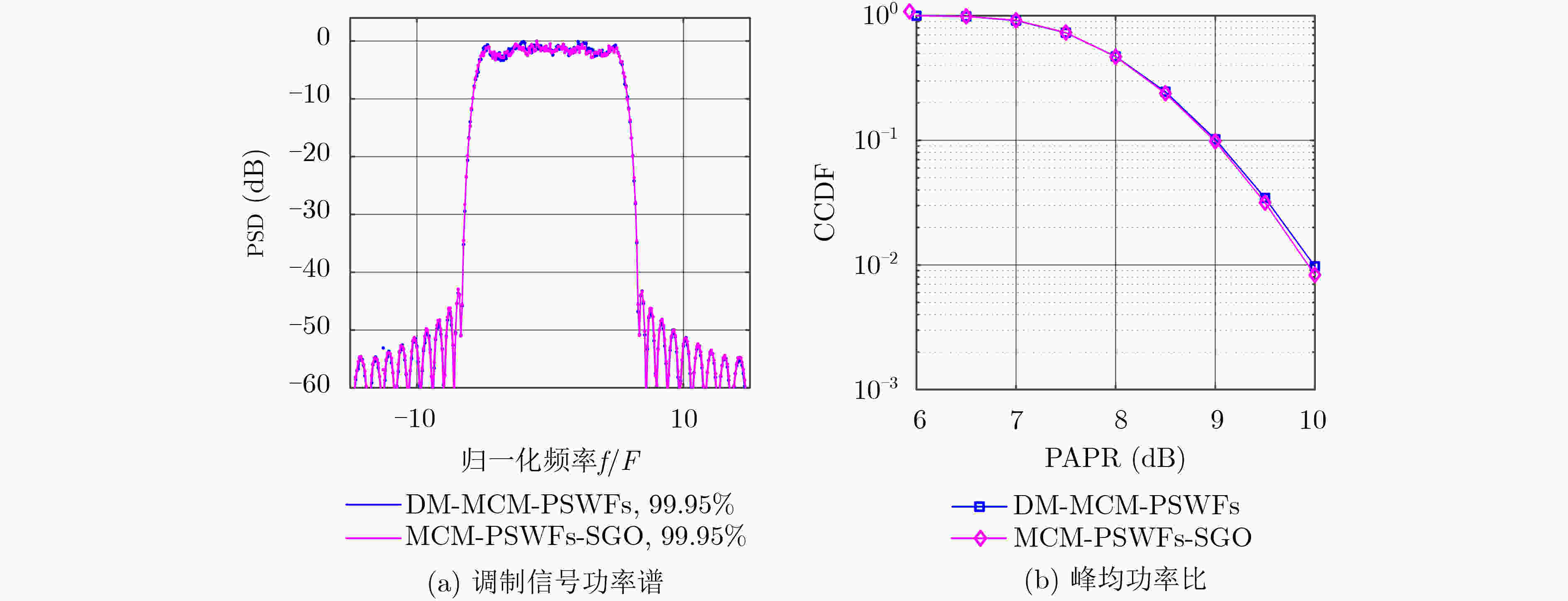
摘要: 该文在基于信号分组优化的椭圆球面波函数(PSWFs)多载波调制的基础上,引入双模索引调制思想,提出了双模PSWFs多载波索引调制解调方法(DM-MCM-PSWFs)。该方法利用未被激活的剩余部分子载波加载第2星座图产生的调制符号,以传输额外的信息比特,实现了基于信号分组优化的PSWFs多载波调制中频谱资源的进一步利用,有效提高了系统频带利用率以及误码性能。理论和仿真分析表明,相较于基于信号分组优化的PSWFs多载波调制,所提方法以适当牺牲系统复杂度为代价,具有更高的系统频带利用率和更优的系统误码性能,当误比特率为1×10–5, n=7, k=3时,所提方法系统频带利用率、误码性能可分别提升9.15%, 2.4 dB。Abstract: With the foundation of multi-carrier modulation based on Prolate Spheroidal Wave Functions (PSWFs) with signal grouping optimization, the idea of dual-mode index modulation is introduced, and proposes a method of Multi-Carrier index Modulation based on PSWFs with Dual-Mode (DM-MCM-PSWFs) is introduced. The remaining sub-carriers that are not activated to load the modulation symbols generated by the second constellation to transmit additional information bits are used in this method, resulting in the improvements of efficiency of spectrum resources in multi-carrier modulation based on PSWFs with signal grouping optimization, and it improves effectively the system spectral efficiency and error performance. Theoretical and simulation analysis indicate that compared with multi-carrier modulation based on PSWFs with signal grouping optimization, the proposed method can attain higher system spectral efficiency and better system error performance at the expense of acceptable sacrifice of system complexity. When the bit rate is 1×10-5, n=7, and k=3, the system spectral efficiency and error performance of the proposed method can increase by 9.15% and 2.4 dB respectively.
-
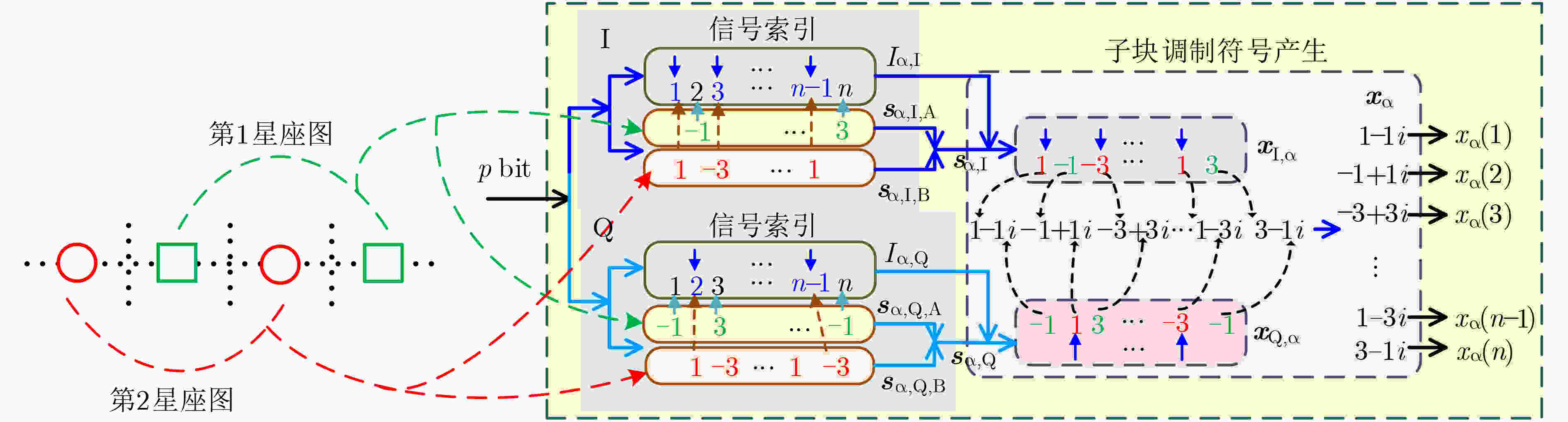
表 1 n=4,k=2时DM-MCM-PSWFs的一种映射方案
比特信号 信号索引 子载波映射 [0,0] {0,0,1,1} {$s_{\rm{I}} ^{\rm{A}} (1)$,$s_{\rm{I}} ^{\rm{A}} (2)$,$s_{\rm{I}} ^{\rm{B}} (1)$,$s_{\rm{I}} ^{\rm{B}} (2)$} [0,1] {0,1,0,1} {$s_{\rm{I}} ^{\rm{A}} (1)$,$s_{\rm{I}} ^{\rm{B}} (1)$,$s_{\rm{I}} ^{\rm{A}} (2)$,$s_{\rm{I}} ^{\rm{B}} (2)$} [1,0] {1,0,0,1} {$s_{\rm{I}} ^{\rm{B}} (1)$,$s_{\rm{I}} ^{\rm{A}} (1)$,$s_{\rm{I}} ^{\rm{A}} (2)$,$s_{\rm{I}} ^{\rm{B}} (2)$} [1,1] {0,1,1,0} {$s_{\rm{I}} ^{\rm{A}} (1)$,$s_{\rm{I}} ^{\rm{B}} (1)$,$s_{\rm{I}} ^{\rm{B}} (2)$,$s_{\rm{I}} ^{\rm{A}} (2)$} 表 2 不同多载波调制方法系统频带利用率
调制方法 g n k SE(bit/s/Hz) ${E_{\rm{b}}}{\rm{/} }{N_{\rm{0} } }$(dB) $\rho $(%) DM-MCM-PSWFs 15 6 3 3.09 11.98 / MCM-PSWFs-SGO-2PAM 9 10 7 2.41 11.05 28.2 MCM-PSWFs-SGO-4PAM 23 4 1 1.90 13.46 62.6 23 4 2 2.85 14.95 8.4 MCOM-PSWFs-8QAM 1 92 92 2.85 12.45 8.4 表 3 信号索引检测乘法运算量
调制方式 运算量 n k 乘法次数(B=1.44 MHz) DM-MCM-PSWFs-ML $O(ng{2^{\left\lfloor {C_n^k} \right\rfloor }})$ 4 1/2/3 368 MCM-PSWFs-SGO-ML $O({\rm{2}}kg{2^{\left\lfloor {C_n^k} \right\rfloor }})$ 4 1 184 4 2 368 4 3 552 MCM-PSWFs-SGO-OS $O(gn{\log _{\rm{2}}}n)$ 4 2 16 -
[1] CAI Yunlong, QIN Zhijin, CUI Fangyu, et al. Modulation and multiple access for 5G networks[J]. IEEE Communications Surveys & Tutorials, 2018, 20(1): 629–646. [2] ZHANG Lin, LIANG Yingchang, and XIAO Ming. Spectrum sharing for internet of things: A survey[J]. IEEE Wireless Communications, 2019, 26(3): 132–139. doi: 10.1109/MWC.2018.1800259 [3] HOU Zhanwei, SHE Changyang, LI Yonghui, et al. Prediction and communication co-design for ultra-reliable and low-latency communications[J]. IEEE Transactions on Wireless Communications, 2020, 19(2): 1196–1209. doi: 10.1109/TWC.2019.2951660 [4] BUZZI S, D’ANDREA C, LI Dejian, et al. MIMO-UFMC transceiver schemes for millimeter-wave wireless communications[J]. IEEE Transactions on Communications, 2019, 67(5): 3323–3336. doi: 10.1109/TCOMM.2019.2896122 [5] MICHAILOW N, MATTHÉ M, GASPAR I S, et al. Generalized frequency division multiplexing for 5th generation cellular network[J]. IEEE Transactions on Communications, 2014, 62(9): 3045–3061. doi: 10.1109/TCOMM.2014.2345566 [6] WANG Hongxing, LU Faping, LIU Chuanhui, et al. Frequency domain multi-carrier modulation based on prolate spheroidal wave functions[J]. IEEE Access, 2020, 8: 99665–99680. doi: 10.1109/ACCESS.2020.2997679 [7] 陆发平, 王红星, 刘传辉, 等. 椭圆球面函数频域调制解调方法[J]. 电子与信息学报, 2020, 42(8): 1888–1895. doi: 10.11999/JEIT190642LU Faping, WANG Hongxing, LIU Chuanhui, et al. PSWFs Frequency Domain Modulation and Demodulation Method[J]. Journal of Electronics &Information Technology, 2020, 42(8): 1888–1895. doi: 10.11999/JEIT190642 [8] KHARE K and GEORGE N. Sampling theory approach to prolate spheroidal wavefunctions[J]. Journal of Physics A: Mathematical and General, 2003, 36(39): 10011–10021. doi: 10.1088/0305-4470/36/39/303 [9] 王红星, 陆发平, 刘传辉, 等. 严格奇偶对称的椭圆球面波函数信号构建与低复杂度检测方法[J]. 中国科学: 信息科学, 2020, 50(5): 766–776. doi: 10.1360/SSI-2019-0121WANG Hongxing, LU Faping, LIU Chuanhui, et al. Strict parity symmetric prolate spheroidal wave functions signal construction and low complexity detection method[J]. Scientia Sinica Informationis, 2020, 50(5): 766–776. doi: 10.1360/SSI-2019-0121 [10] CAI Biao, LIU Aijun, and LIANG Xiaohu. Low-complexity selective mapping methods for multicarrier faster-than-nyquist signaling[J]. IEEE Access, 2020, 8: 31420–31431. doi: 10.1109/ACCESS.2020.2973394 [11] HU Yue, WANG Yafeng, and WANG Haocheng. A decoding method based on RNN for OvTDM[J]. China Communications, 2020, 17(4): 1–10. [12] LI Qiang, WEN Miaowen, CLERCKX B, et al. Subcarrier index modulation for future wireless networks: Principles, applications, and challenges[J]. IEEE Wireless Communications, 2020, 27(3): 118–125. doi: 10.1109/MWC.001.1900335 [13] DANG Shuping, GUO Shuaishuai, COON J P, et al. Enhanced Huffman coded OFDM with index modulation[J]. IEEE Transactions on Wireless Communications, 2020, 19(4): 2489–2503. doi: 10.1109/TWC.2020.2965524 [14] LI Qiang, WEN Miaowen, BASAR E, et al. Index modulated OFDM spread spectrum[J]. IEEE Transactions on Wireless Communications, 2018, 17(4): 2360–2374. doi: 10.1109/TWC.2018.2793238 [15] 王红星, 陆发平, 刘传辉, 等. 基于信号分组优化的椭圆球面波多载波调制解调方法[J]. 中国科学: 信息科学, 2021, 51(7): 1168–1182.WANG Hongxing, LU Faping, LIU Chuanhui, et al. Multi-carrier modulation scheme based on prolate spheroidal wave functions with signal grouping optimization[J]. Scientia Sinence Informationis, 2021, 51(7): 1168–1182. [16] MAO Tianqi, JIANG Rui, and BAI Ruowen. Optical dual-mode index modulation aided OFDM for visible light communications[J]. Optics Communications, 2017, 391: 37–41. doi: 10.1016/j.optcom.2017.01.005 [17] 陆发平, 刘传辉, 康家方. 基于顺序统计量的索引调制信号检测方法[J]. 无线电通信技术, 2019, 45(1): 57–61. doi: 10.3969/j.issn.1003-3114.2019.01.11LU Faping, LIU Chuanhui, and KANG Jiafang. Index modulation signal detection method based on order statistic[J]. Radio Communications Technology, 2019, 45(1): 57–61. doi: 10.3969/j.issn.1003-3114.2019.01.11 -





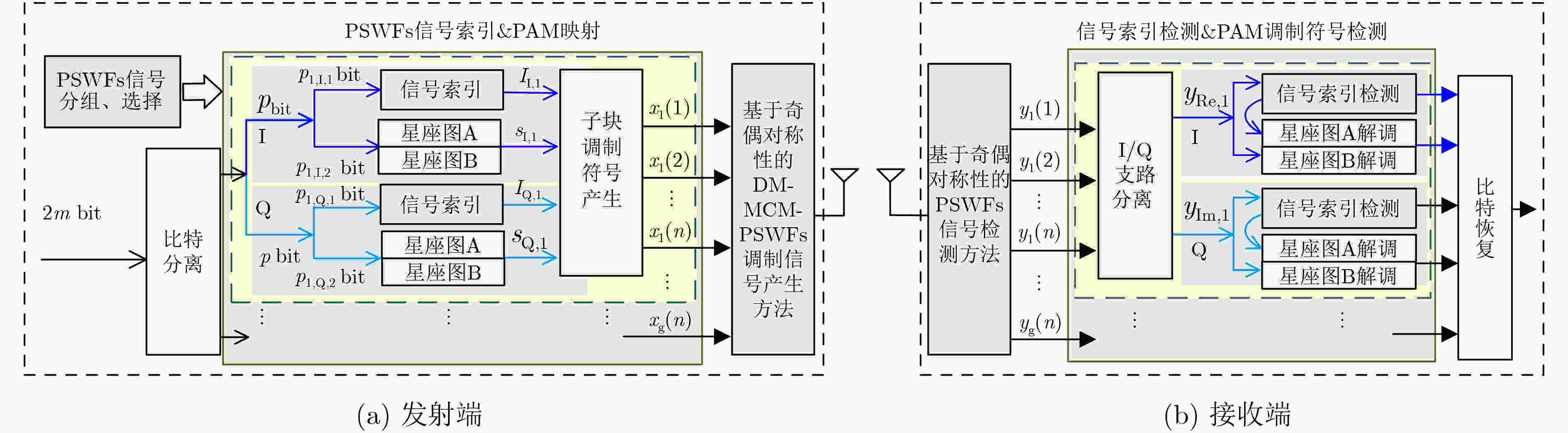
 下载:
下载:



 下载:
下载:
