Signal Compensation of Coaxial Cable Based on Modified Non-negative Tikhonov Regularization Method within Bayesian Inference
-
摘要: 随着信号频率、带宽及传输距离的增大,信号在同轴电缆传输过程中的畸变问题变得越来越严重。特别地,如果同轴电缆在使用过程中还意外地遭受了挤压、拉伸或折叠,信号畸变问题将会变得更加严重。该文基于贝叶斯推理的非负Tikhonov正则化方法,提出一种改进的信号补偿方法。该方法可有效规避逆分析中的病态矩阵问题,利用同轴电缆的冲击响应函数,并结合输出端口的测量信号,即可实现输入信号的重构。并以长度15 m的受挤压同轴电缆为对象,采用此方法对3种不同样式的脉冲信号(双指数脉冲信号、调制方波信号、双极脉冲信号)进行了传输畸变补偿。结果表明:该方法均能实现优异的补偿效果,补偿后信号与输入信号间偏差远远低于传统的衰减补偿法。并且,该方法具有较强的鲁棒性,当信噪比大于30 dB时,即可保持好的稳定性。
-
关键词:
- 信号补偿 /
- 改进的非负Tikhonov正则化方法 /
- 同轴电缆
Abstract: With the increase of signal frequency, bandwidth and transmission distance, the signal distortion problem brought by the coaxial cable becomes serious and can not be ignored. Specifically, if the coaxial cable is accidentally squeezed, stretched or folded during use, the signal distortion problem will become more serious. Herein, a modified signal compensation method is proposed based on the non-negative Tikhonov regularization method with Bayesian inference. This method can effectively avoid the ill-conditioned matrix problem in the inverse analysis. The input signal can be reconstructed by using impulse response function of coaxial cable and measured output signal. Three different types of pulse signals, i.e., double exponential pulse signal, modulated square wave signal, and bipolar pulse signal, transmitted in a 15 m extruded coaxial cable are compensated. The results show that this method can achieve excellent compensation effect, and the deviation between the compensated signal and the input signal is far lower than that of typical attenuation compensation method. Moreover, the modified method exhibits strong robustness. When the signal-to-noise ratio is larger than 30 dB, it can maintain good stability. -
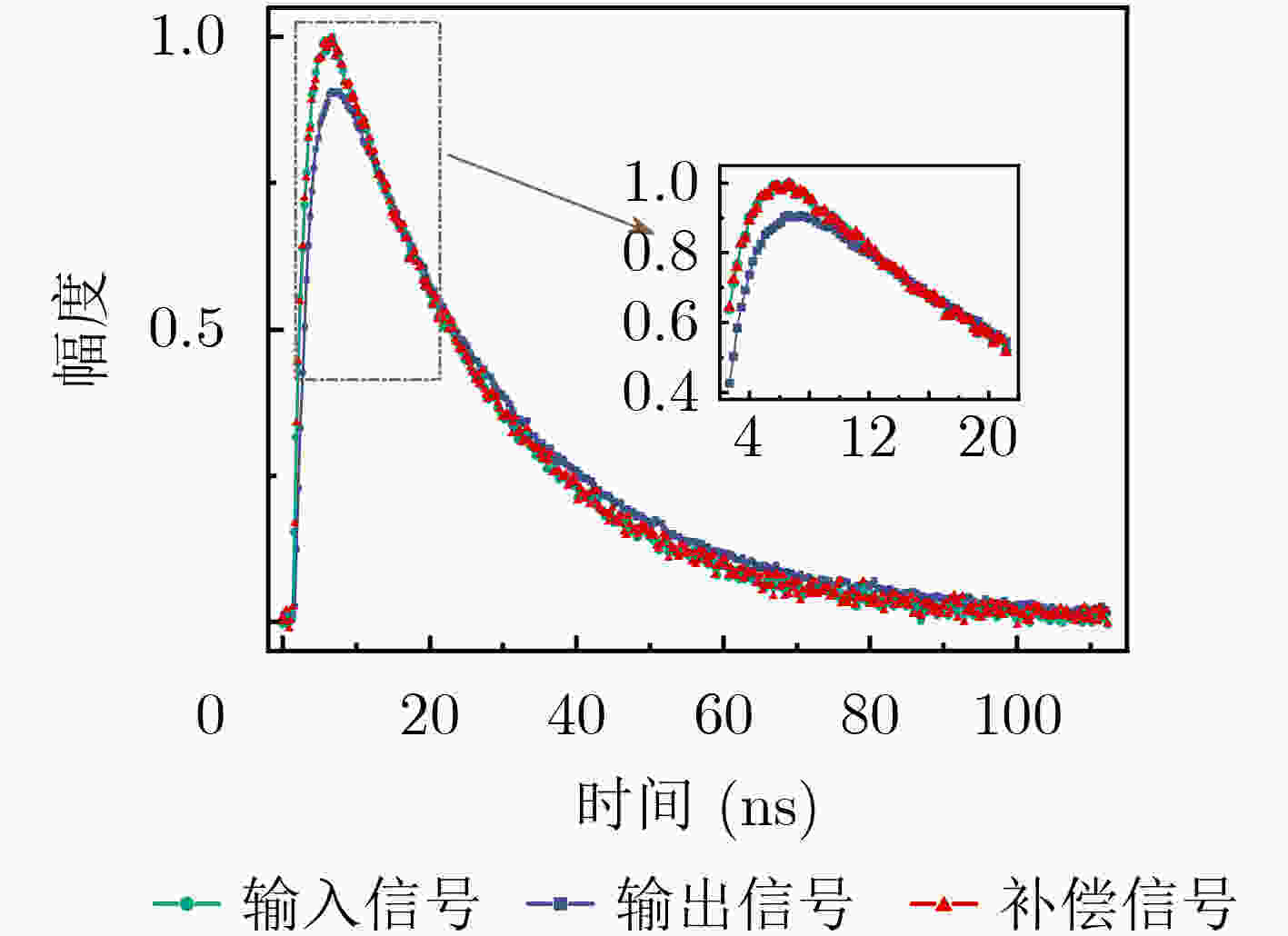
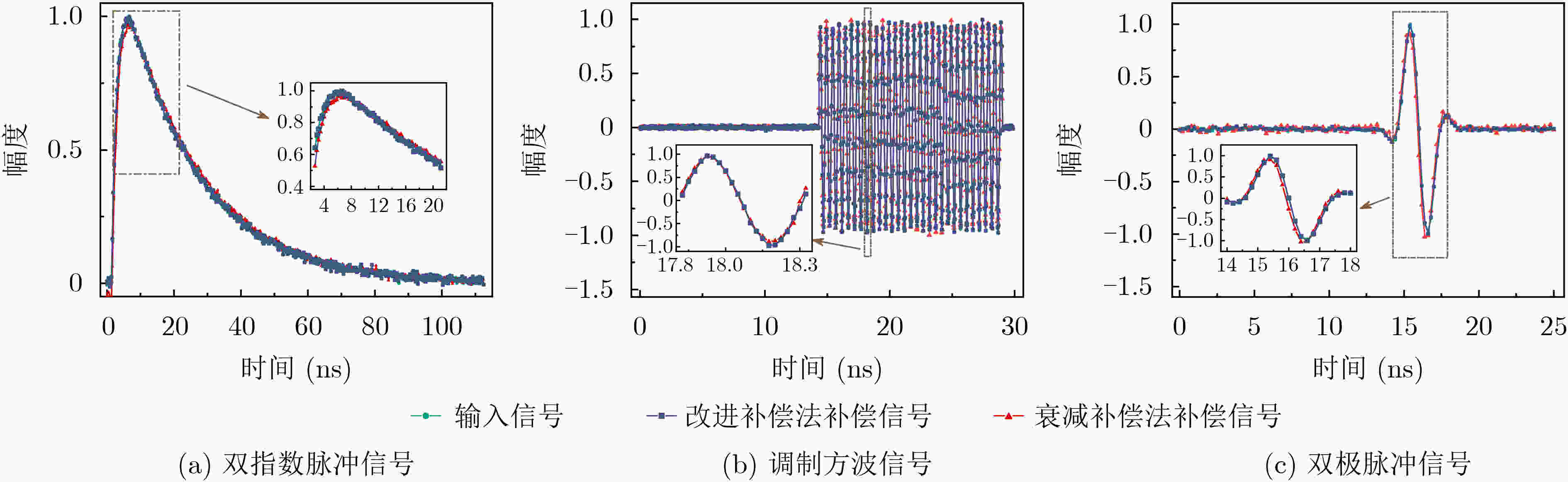
表 1 输出信号、采用改进补偿方法补偿后信号与输入信号间相对偏差(%)
信号样式 输出与输入信号偏差 补偿与输入信号偏差 双指数脉冲信号 16.5 1.5 调制方波信号 24.4 2.4 双极脉冲信号 25.3 2.3 表 2 不同补偿方法补偿后信号与输入信号间相对偏差(%)
信号样式 改进补偿法 衰减补偿法 双指数脉冲信号 1.5 9.0 调制方波信号 2.4 10.5 双极脉冲信号 2.3 13.3 表 3 不同信噪比下改进补偿方法补偿后信号与输入信号间相对偏差(%)
信号样式 45 dB 40 dB 35 dB 30 dB 25 dB 20 dB 双指数脉冲信号 1.5 1.5 1.7 3.4 11.1 17.0 调制方波信号 2.4 2.5 2.8 6.3 19.1 33.1 双极脉冲信号 2.3 2.5 3.0 6.5 18.6 28.3 表 4 不同信噪比下衰减补偿法补偿后信号与输入信号间相对偏差(%)
信号样式 45 dB 40 dB 35 dB 30 dB 25 dB 20 dB 双指数脉冲信号 9.0 9.3 12.0 17.2 22.0 31.0 调制方波信号 10.5 11.1 15.5 22.5 27.5 35.5 双极脉冲信号 13.3 13.7 16.3 24.3 29.3 36.3 -
[1] XIAO Pei, DU Pingan, and ZHANG Bingxue. An analytical method for radiated electromagnetic and shielding effectiveness of braided coaxial cable[J]. IEEE Transactions on Electromagnetic Compatibility, 2019, 61(1): 121–127. doi: 10.1109/TEMC.2018.2814629 [2] 王亚平, 蔡勖. ALICE实验中同轴电缆的信号传输特性的研究[J]. 核电子学与探测技术, 2006, 26(2): 195–198. doi: 10.3969/j.issn.0258-0934.2006.02.019WANG Yaping and CAI Xu. Study of signal transmission properties of a coaxial cable in ALICE experiment[J]. Nuclear Electronics &Detection Technology, 2006, 26(2): 195–198. doi: 10.3969/j.issn.0258-0934.2006.02.019 [3] SATO S, YAMAGUCHI T, NISHIMURA S, et al. Influence of measuring cable on lightning impulse parameters[J]. Electronics and Communications in Japan, 2010, 93(6): 1–7. doi: 10.1002/ecj.10288 [4] 戈弋, 黄华, 袁欢. 温度和机械弯曲引起的同轴电缆相位变化特性[J]. 太赫兹科学与电子信息学报, 2019, 17(4): 621–626. doi: 10.11805/TKYDA201904.0621GE Yi, HUANG Hua, and YUAN Huan. Phase characteristics of coaxial cable caused by temperature and mechanical bending[J]. Journal of Terahertz Science and Electronic Information Technology, 2019, 17(4): 621–626. doi: 10.11805/TKYDA201904.0621 [5] 贾文超, 董冰, 李林. 同轴电缆形变TDR监测技术的研究与实现[J]. 计算机测量与控制, 2007, 15(10): 1276–1278. doi: 10.3969/j.issn.1671-4598.2007.10.004JIA Wenchao, DONG Bing, and LI Lin. Research and realization of coaxial-cable deformation TDR monitoring technology[J]. Computer Measurement &Control, 2007, 15(10): 1276–1278. doi: 10.3969/j.issn.1671-4598.2007.10.004 [6] 陈云敏, 梁志刚, 陈仁朋, 等. 有缺陷同轴电缆的时域反射特性及应用[J]. 浙江大学学报: 工学版, 2005, 39(1): 51–56, 64.CHEN Yunmin, LIANG Zhigang, CHEN Renpeng, et al. Time domain reflectometry property of defective coaxial cable and its applications[J]. Journal of Zhejiang University:Engineering Science, 2005, 39(1): 51–56, 64. [7] CHOO J, CHOO J, and KIM Y H. Evaluation of electromagnetic interference from axially ruptured coaxial cable with multiple dielectrics used in nuclear power plants[J]. IEEE Transactions on Electromagnetic Compatibility, 2019, 61(3): 860–869. doi: 10.1109/TEMC.2018.2835665 [8] 渠红光, 李宪优, 田耕, 等. 基于衰减特性的电缆传输畸变软件补偿[J]. 装备指挥技术学院学报, 2010, 21(2): 87–90.QU Hongguang, LI Xianyou, TIAN Geng, et al. Attenuation compensation by software based on the character of transfers of the cable[J]. Journal of the Academy of Equipment Command &Technology, 2010, 21(2): 87–90. [9] 付佳斌, 卿燕玲, 卫兵, 等. 同轴电缆测量纳秒脉冲信号衰减的数字补偿[J]. 强激光与粒子束, 2011, 23(10): 2826–2830. doi: 10.3788/HPLPB20112310.2826FU Jiabin, QING Yanlin, WEI Bing, et al. Numerical compensation for coaxial cable signal degradation[J]. High Power Laser and Particle Beams, 2011, 23(10): 2826–2830. doi: 10.3788/HPLPB20112310.2826 [10] 黄豹. 新的高频同轴电缆补偿原理[J]. 核电子学与探测技术, 1985, 5(4): 193–198.HUANG Bao. New principle of high frequency coaxial cable equalization[J]. Nuclear Electronics &Detection Technology, 1985, 5(4): 193–198. [11] WHALLEY W B. Color-television coaxial cable termination and equalization[J]. SMPTE Journal 1955, 1995, 64(1): 8–12. [12] SARKAR T K, TSENG F I, RAO S M, et al. Deconvolution of impulse response from time-limited input and output: Theory and experiment[J]. IEEE Transactions on Instrumentation and Measurement, 1985, IM-34(4): 541–546. doi: 10.1109/TIM.1985.4315400 [13] 王园, 朱江淼. 应用反卷积实现脉冲计量中的信号重构与系统辨识[J]. 信号处理, 2013, 29(4): 532–535. doi: 10.3969/j.issn.1003-0530.2013.04.017WANG Yuan and ZHU Jiangmiao. Signal reconstruction and system identification using the deconvolution in pulse metrology[J]. Signal Processing, 2013, 29(4): 532–535. doi: 10.3969/j.issn.1003-0530.2013.04.017 [14] THOMPSON A M and KAY J. On some Bayesian choices of regularization parameter in image restoration[J]. Inverse Problems, 1993, 9(6): 749–761. doi: 10.1088/0266-5611/9/6/011 [15] YAN Gang and SUN Hao. A non-negative Bayesian learning method for impact force reconstruction[J]. Journal of Sound and Vibration, 2019, 457: 354–367. doi: 10.1016/j.jsv.2019.06.013 [16] 骆睿, 刘莉, 佟瑞, 等. 基于Tikhonov正则化的高分辨率群时延测量与计算方法[J]. 电子学报, 2019, 47(5): 1044–1048. doi: 10.3969/j.issn.0372-2112.2019.05.010LUO Rui, LIU Li, TONG Rui, et al. High-resolution group delay measurement and calculation method based on Tikhonov regularization[J]. Acta Electronica Sinica, 2019, 47(5): 1044–1048. doi: 10.3969/j.issn.0372-2112.2019.05.010 [17] 韩玉兵, 吴乐南, 张冬青. 基于正则化处理的超分辨率重建[J]. 电子与信息学报, 2007, 29(7): 1713–1716.HAN Yubing, WU Lenan, and ZHANG Dongqing. Super-resolution reconstruction based on regularization[J]. Journal of Electronics &Information Technology, 2007, 29(7): 1713–1716. [18] 王鹏宇, 宋千, 周智敏. 基于多测量动态聚类的压缩感知增强成像方法[J]. 电子与信息学报, 2013, 35(11): 2664–2671.WANG Pengyu, SONG Qian, and ZHOU Zhimin. Enhanced compressive imaging approach based on multi-measurement and dynamic clustering[J]. Journal of Electronics &Information Technology, 2013, 35(11): 2664–2671. [19] JOHNSTON P R and GULRAJANI R M. Selecting the corner in the L-curve approach to Tikhonov regularization[J]. IEEE Transactions on Biomedical Engineering, 2000, 47(9): 1293–1296. doi: 10.1109/10.867966 [20] 王师萍. 带非负限制的增广Tikhonov正则化方法[D]. [硕士论文], 上海交通大学, 2010.WANG Shiping. Augmented Tikhonov regularization with nonnegative constraints[D]. [Master dissertation], Shanghai Jiao Tong University, 2010. [21] WANG Jingbo and ZABARAS N. Hierarchical Bayesian models for inverse problems in heat conduction[J]. Inverse Problems, 2005, 21(1): 183–206. doi: 10.1088/0266-5611/21/1/012 [22] JIN Bangti and ZOU Jun. A Bayesian inference approach to the ill-posed Cauchy problem of steady-state heat conduction[J]. International Journal for Numerical Methods in Engineering, 2008, 76(4): 521–544. doi: 10.1002/nme.2350 [23] JIN Bangti and ZOU Jun. Augmented Tikhonov regularization[J]. Inverse Problems, 2009, 25(2): 025001. doi: 10.1088/0266-5611/25/2/025001 -





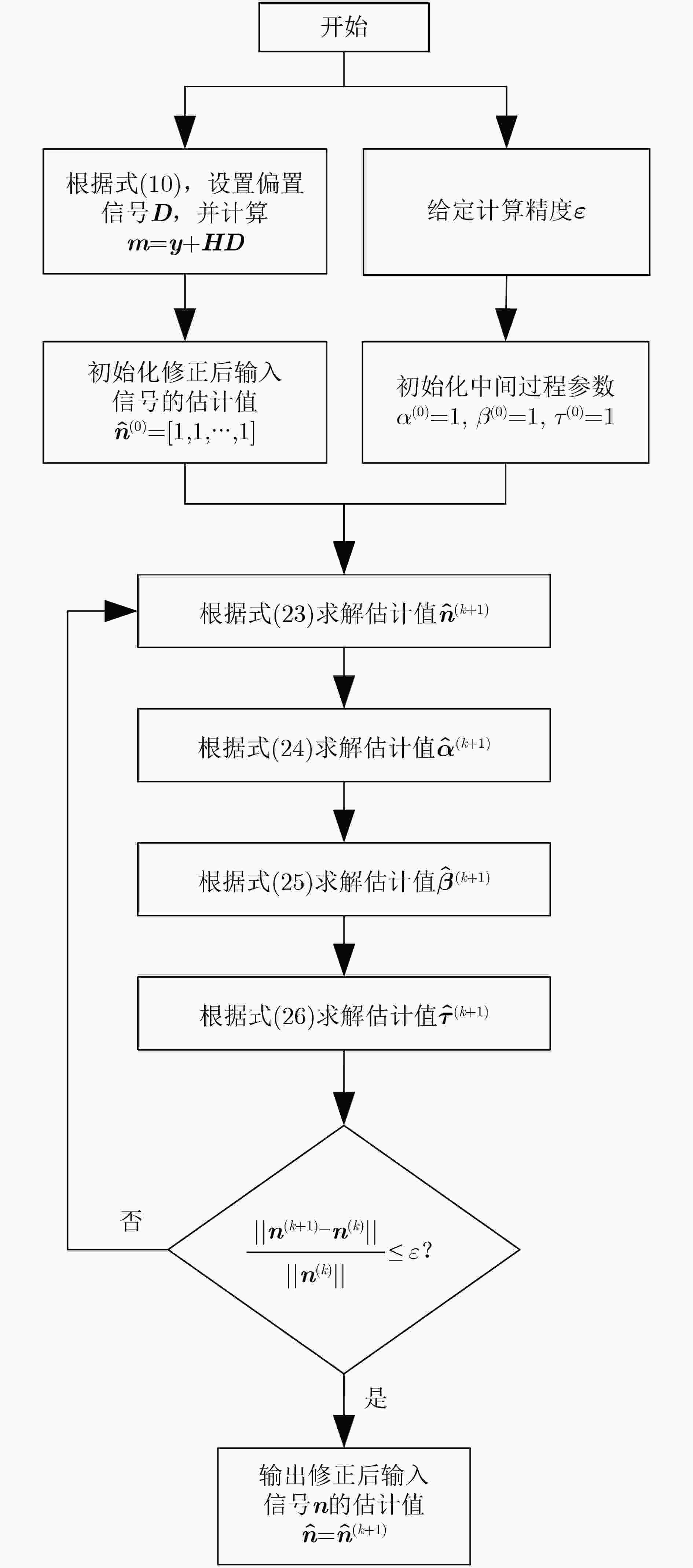
 下载:
下载:
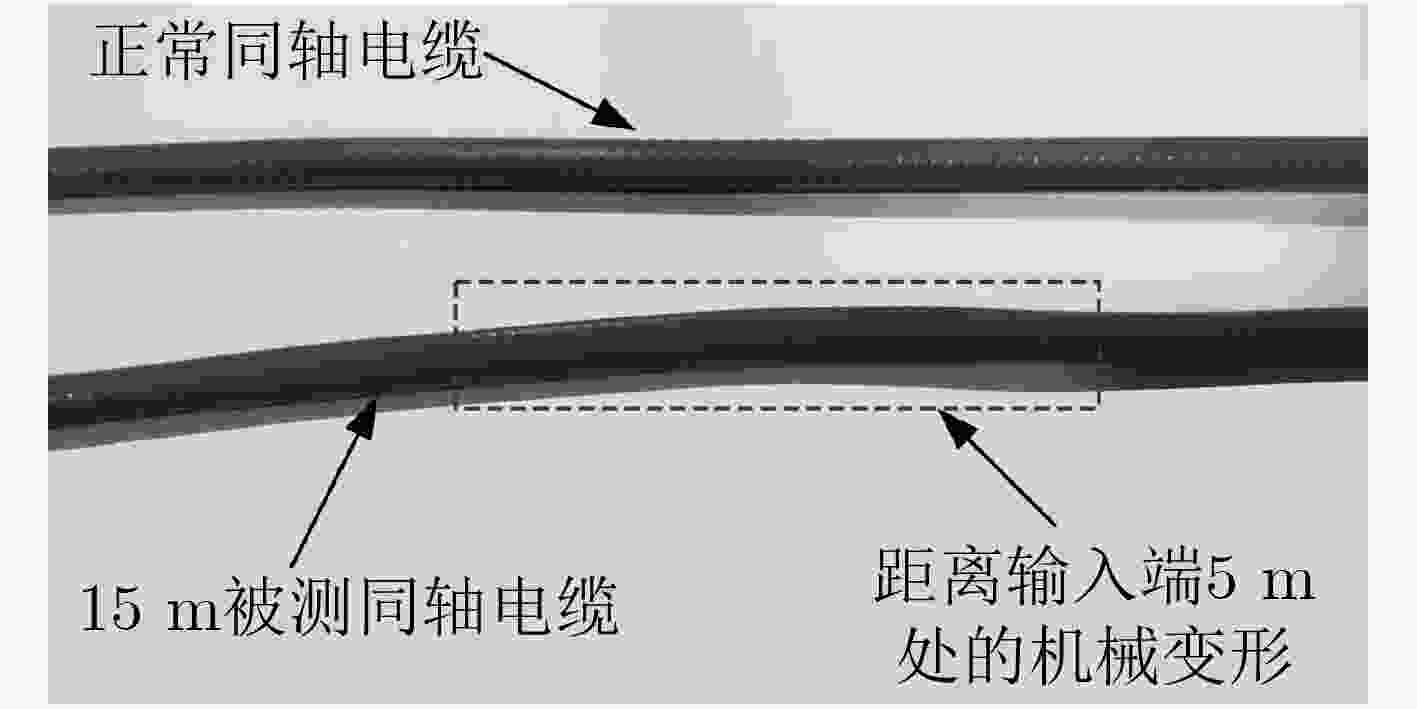
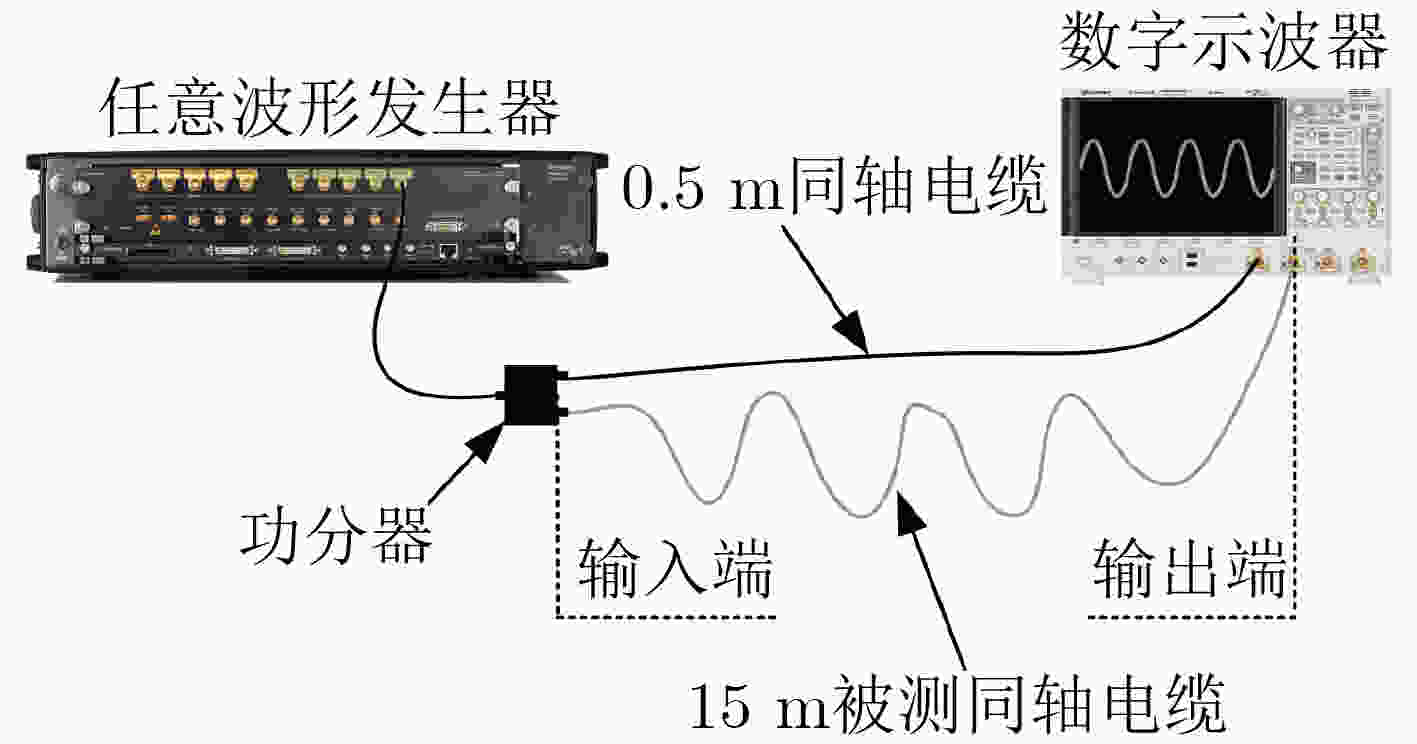
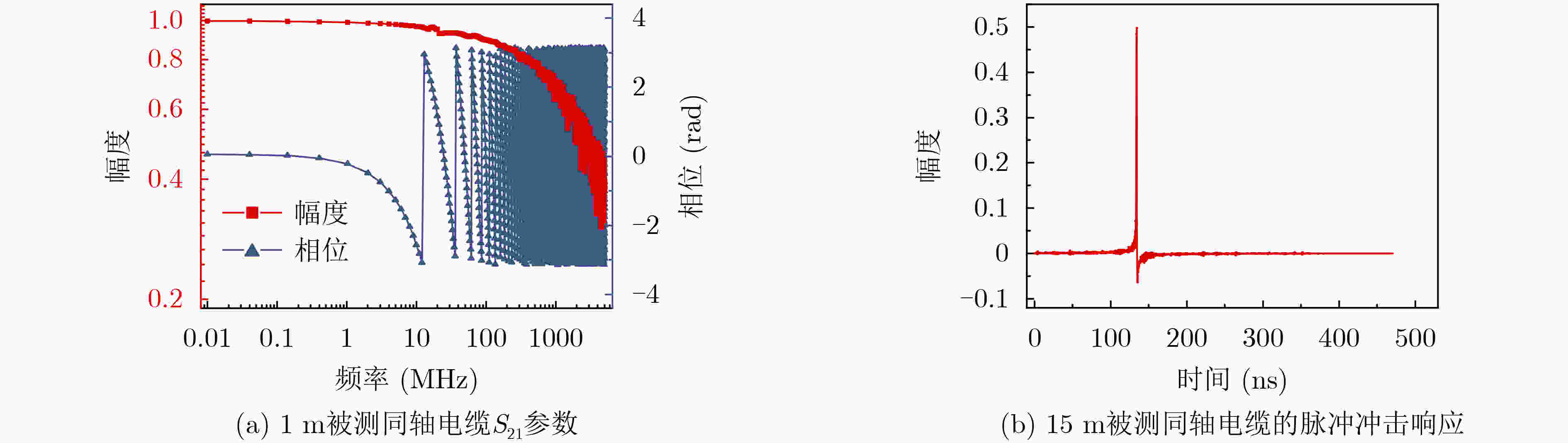



 下载:
下载:
