Orthogonal Multiuser Short Reference High Rate Differential Chaos Shift Keying Communication System
-
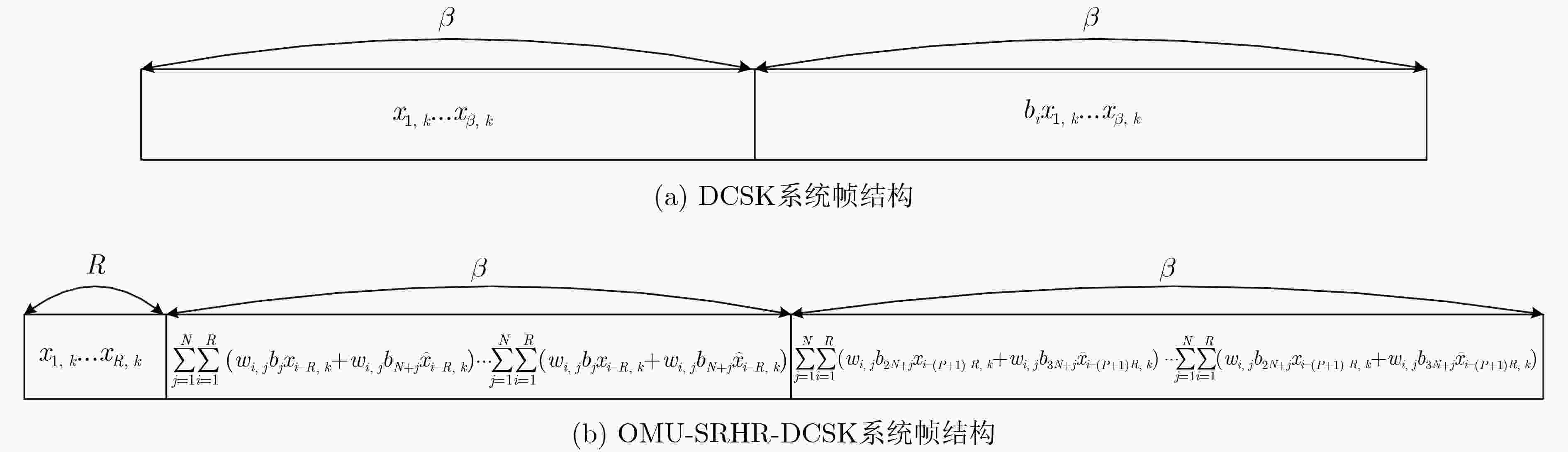
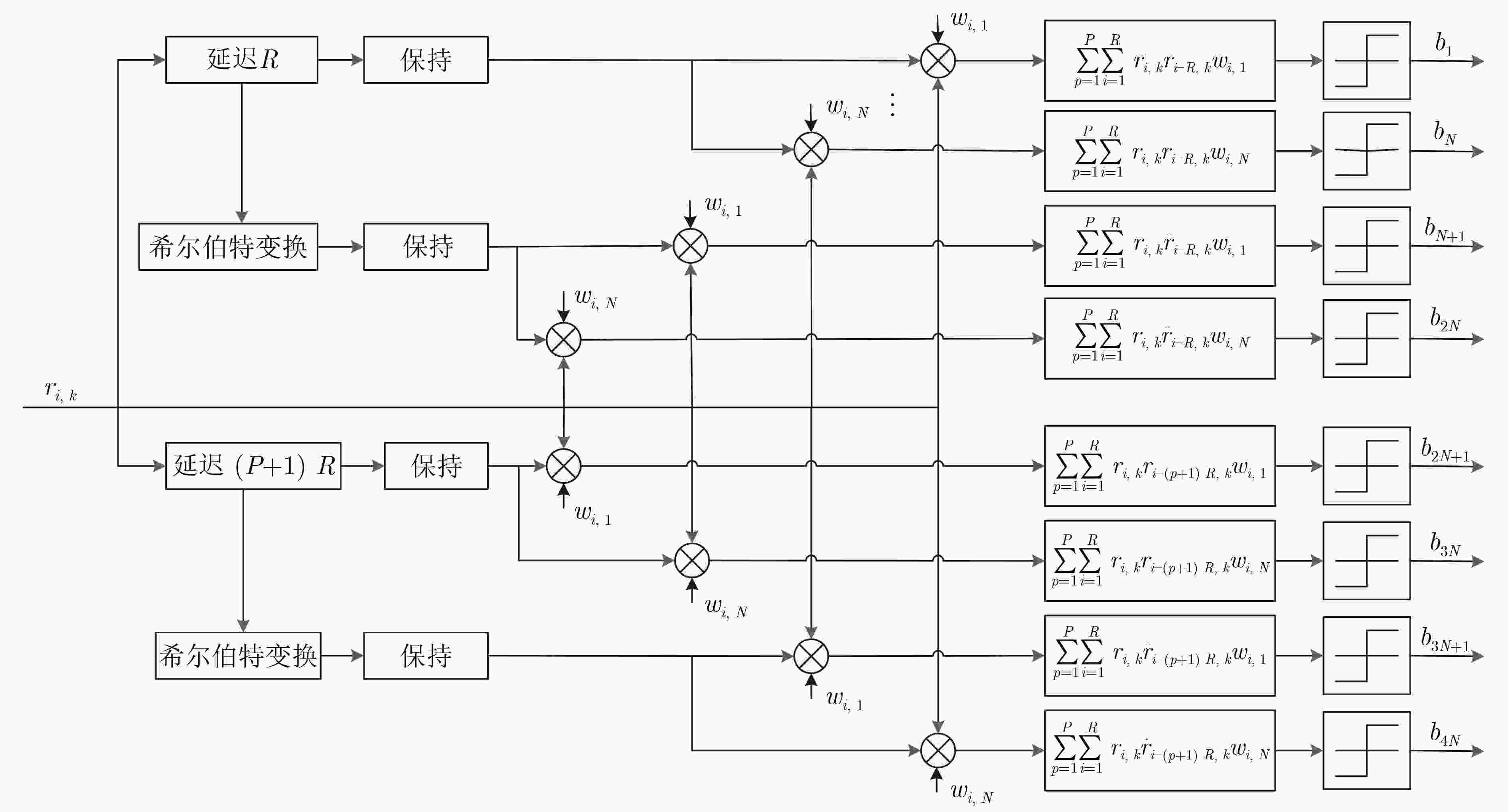
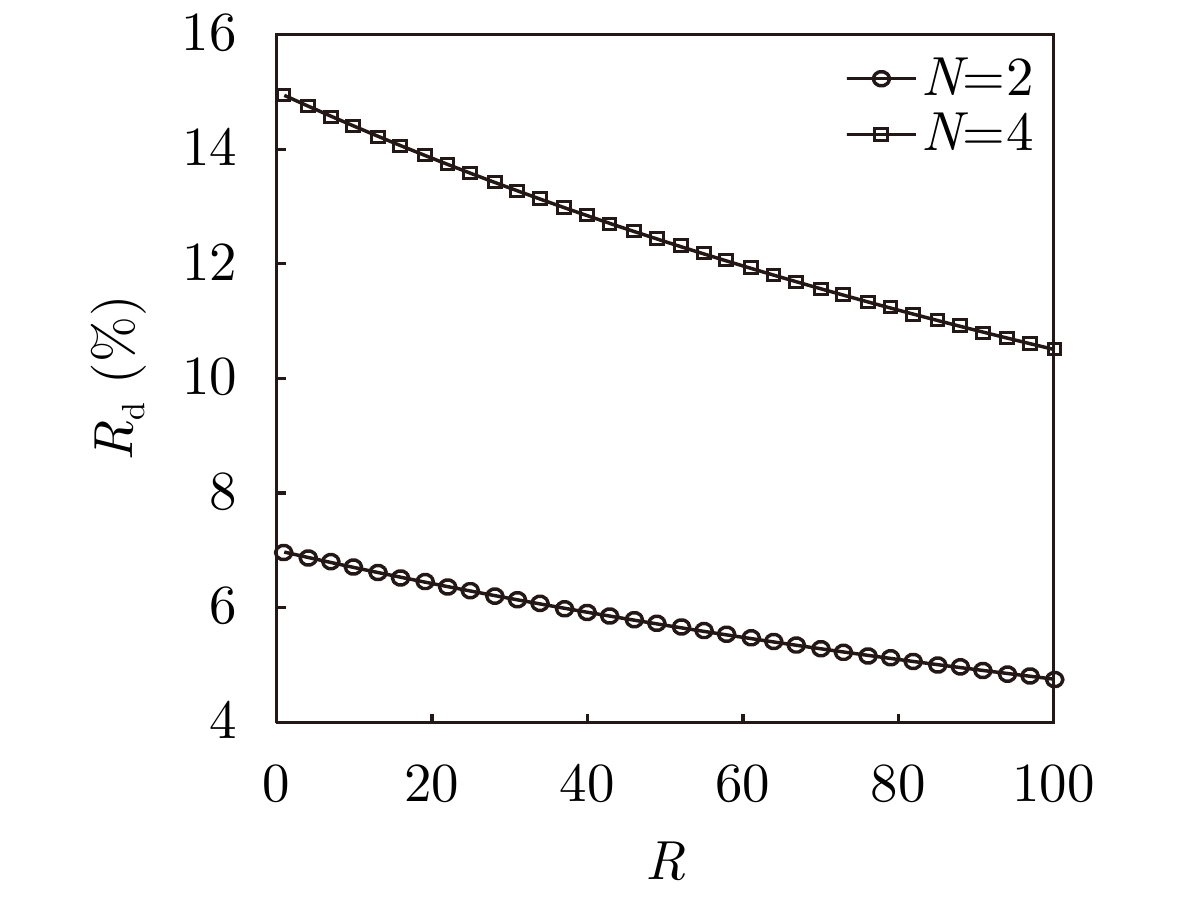
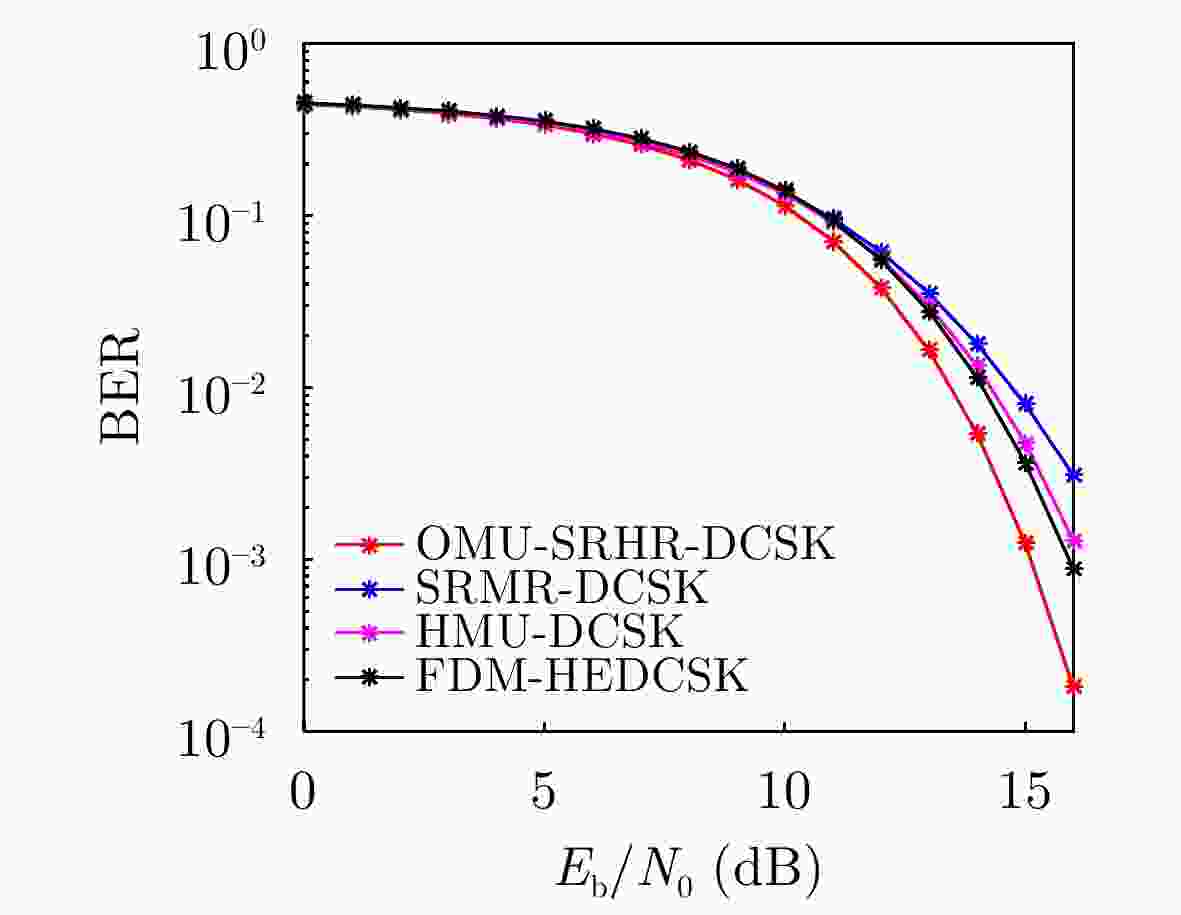
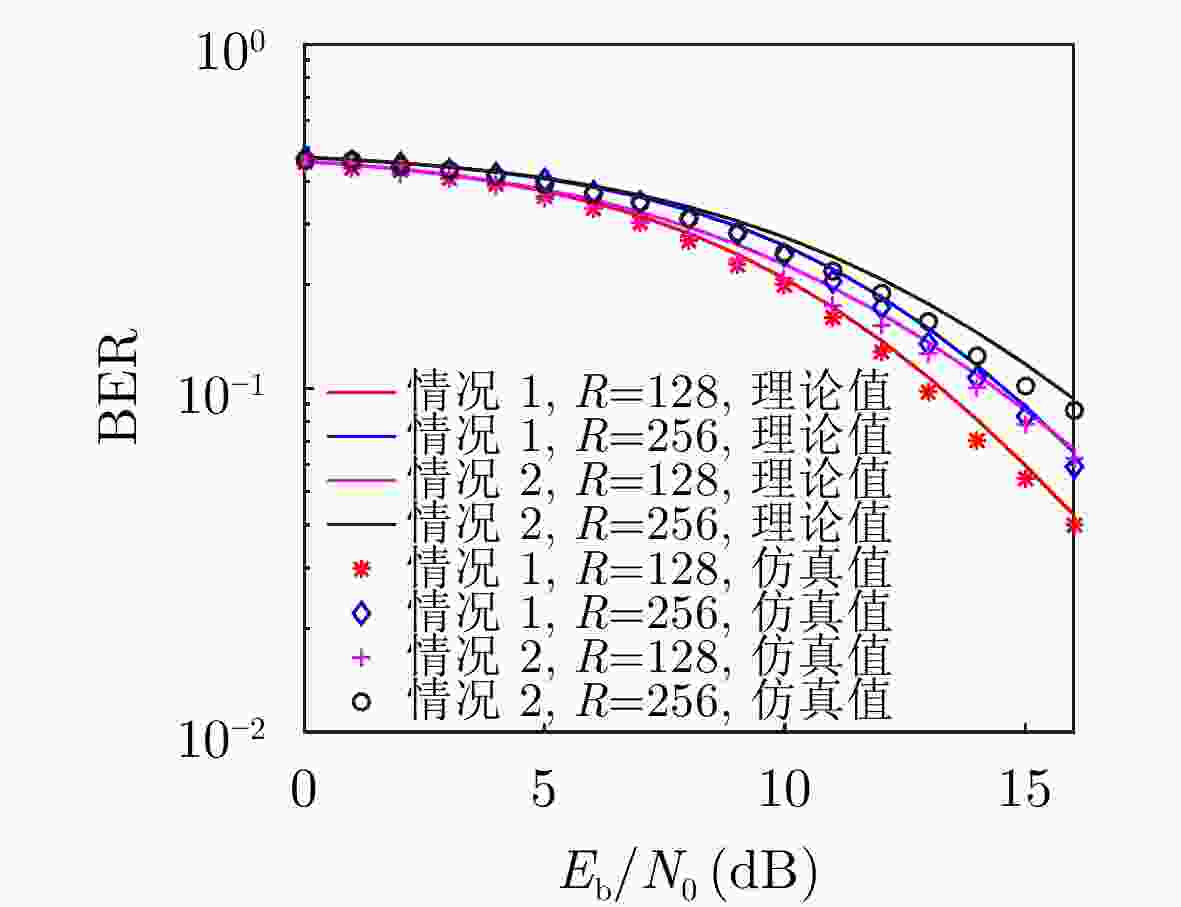
摘要: 针对传统多用户短参考混沌移位键控通信系统传输速率和能量效率低的问题,该文提出了一种正交多用户高速短参考差分混沌移位键控(OMU-SRHR-DCSK)通信系统。系统将参考信号长度缩短为每个数据信号长度的1/P,通过增加两路连续的信息时隙来传输多个用户信息比特,通过希尔伯特变换在每个信息时隙多传输N个用户信息比特,极大地提高了系统的数据传输速率。将希尔伯特变换和Walsh码相结合完全消除了用户间干扰,改善了误码率性能。推导了OMU-SRHR-DCSK系统在加性高斯白噪声(AWGN)信道和多径瑞利衰落信道(RFC)中的比特误码率(BER)公式并进行了实验验证。在两种信道下的仿真值和理论推导值均相等,验证了理论推导的正确性。该系统的传输速率相对于传统多用户短参考系统的传输速率有极大提升,传输比特数相同的条件下系统的误码性能明显优于传统多用户短参考系统,证明了该系统具有优异的实用价值,并为其应用于实际中提供了良好的理论支撑。Abstract: An Orthogonal MultiUser Short Reference High Rate DCSK (OMU-SRHR-DCSK) communication system is proposed to solve the problem of low transmission rate and low energy efficiency of traditional MultiUser Short Reference Differential Chaos Shift Keying communication system. The system shortens reference signal length to 1/P of data signal, transmits multiple users’ information bits by adding two continuous information time slots, and transmits additionally N user’ information bit in each information time slot through Hilbert transform, which greatly improves the data transmission rate of the system. The interference between users is completely eliminated mainly by combining Hilbert transform with Walsh code, thus improving the Bit Error Rate (BER) performance. The theoretical BER formula of OMU-SRHR-DCSK system over Additive White Gaussian Noise (AWGN) and L-path Rayleigh Fading Channel (RFC) are derived and verified by experiments. The experimental simulation and theoretical derivation are consistent in the two channels, which proves the correctness of theoretical derivation. Compared with the traditional multiuser short reference system, the transmission rate of the system is greatly improved, and the BER performance of the system is obviously superior over that of the traditional multiuser short reference system under the condition of the same number of transmission bit. It is proved that the system has excellent practical value and provides a good theoretical support for its application in practice.
-
Key words:
- Chaos communication /
- Multiuser /
- Hilbert transform /
- Walsh code /
- Transmission rate
-
表 1 OMU-SRHR-DCSK, SRMR-DCSK, HMU-DCSK和FDM-HEDCSK系统的传输速率和能量效率
系统 传输速率(R) 能量效率(EE) OMU-SRHR-DCSK $ 4N/(R + 2\beta ) $ $ 4N\beta /(R + 4N\beta ) $ SRMR-DCSK $ N/(R + \beta ) $ $ N\beta /(R + N\beta ) $ HMU-DCSK $ N/\beta $ $ 2N/(1 + 2N) $ FDM-HEDCSK $ 2/\beta $ $ 1/2 $ -
[1] KADDOUM G and GAGNON F. Design of a high-data-rate differential chaos-shift keying system[J]. IEEE Transactions on Circuits and Systems II:Express Briefs, 2012, 59(7): 448–452. doi: 10.1109/TCSII.2012.2198982 [2] HU Wei, WANG Lin, and KADDOUM G. Design and performance analysis of a differentially spatial modulated chaos shift keying modulation system[J]. IEEE Transactions on Circuits and Systems II:Express Briefs, 2017, 64(11): 1302–1306. doi: 10.1109/TCSII.2017.2697456 [3] BAO Jiyu, XU Weikai, WANG Lin, et al. Performance analysis and sub-carriers power allocation for MC-QCSK[C]. International Conference on Wireless Communications & Signal Processing (WCSP), Nanjing, China, 2015: 1–5. doi: 10.1109/WCSP.2015.7341173. [4] LIU Zhaofeng, ZHANG Lin, WU Zhiqiang, et al. A secure and robust frequency and time diversity aided OFDM-DCSK modulation system not requiring channel state information[J]. IEEE Transactions on Communications, 2020, 68(3): 1684–1697. doi: 10.1109/TCOMM.2019.2951512 [5] KADDOUM G, TRAN H V, KONG Long, et al. Design of simultaneous wireless information and power transfer scheme for short reference DCSK communication systems[J]. IEEE Transactions on Communications, 2017, 65(1): 431–443. doi: 10.1109/TCOMM.2016.2619707 [6] CAI Guofa, FANG Yi, HAN Guojun, et al. A new hierarchical M-ary DCSK communication system: Design and analysis[J]. IEEE Access, 2017, 5: 17414–17424. doi: 10.1109/ACCESS.2017.2740973 [7] 贺利芳, 吴雪霜, 张天骐. 正交多载波降噪差分混沌键控通信系统[J]. 电子与信息学报, 2021, 43(4): 1120–1128. doi: 10.11999/JEIT200068HE Lifang, WU Xueshuang, and ZHANG Tianqi. Quadrature multicarrier noise reduction differential chaos shift keying system[J]. Journal of Electronics &Information Technology, 2021, 43(4): 1120–1128. doi: 10.11999/JEIT200068 [8] TALEB F, BENDIMERAD F T, and ROVIRAS D. Very high efficiency differential chaos shift keying system[J]. IET Communications, 2016, 10(17): 2300–2307. doi: 10.1049/iet-com.2016.0411 [9] QUYEN N X. On the study of a quadrature DCSK modulation scheme for cognitive radio[J]. International Journal of Bifurcation and Chaos, 2017, 27(9): 1750135. doi: 10.1142/S0218127417501358 [10] 张琳, 徐位凯, 王琳, 等. 码复用差分混沌键控性能分析与同步算法[J]. 重庆邮电大学学报:自然科学版, 2016, 28(3): 330–336. doi: 10.3979/j.issn.1673-825X.2016.03.008ZHANG Lin, XU Weikai, WANG Lin, et al. Performance analysis and synchronization algorithm for CS-DCSK system[J]. Journal of Chongqing University of Posts and Telecommunications:Natural Science Edition, 2016, 28(3): 330–336. doi: 10.3979/j.issn.1673-825X.2016.03.008 [11] KADDOUM G, SOUJERI E, and NIJSURE Y. Design of a short reference noncoherent chaos-based communication systems[J]. IEEE Transactions on Communications, 2016, 64(2): 680–689. doi: 10.1109/TCOMM.2015.2514089 [12] YANG Hua, TANG W K S, CHEN Guanrong, et al. System design and performance analysis of orthogonal multi-level differential chaos shift keying modulation scheme[J]. IEEE Transactions on Circuits and Systems I:Regular Papers, 2016, 63(1): 146–156. doi: 10.1109/TCSI.2015.2510622 [13] KADDOUM G, RICHARDSON F D, and GAGNON F. Design and analysis of a multi-carrier differential chaos shift keying communication system[J]. IEEE Transactions on Communications, 2013, 61(8): 3281–3291. doi: 10.1109/TCOMM.2013.071013.130225 [14] 张刚, 郝怡曼, 张天骐. 短参倍速差分混沌键控系统[J]. 系统工程与电子技术, 2018, 40(1): 184–190. doi: 10.3969/j.issn.1001-506X.2018.01.27ZHANG Gang, HAO Yiman, and ZHANG Tianqi. Short reference multifold rate differential chaos shift keying[J]. Systems Engineering and Electronics, 2018, 40(1): 184–190. doi: 10.3969/j.issn.1001-506X.2018.01.27 [15] WANG Shaonan, LIU Yingjie, and Ma Weijiao. Design of a novel frequency division scheme for DCSK chaos communication system[C]. International Conference on Information Management, Chengdu, China, 2017: 317–321. doi: 10.1109/INFOMAN.2017.7950400. [16] 张刚, 许嘉平, 张天骐. 基于希尔伯特变换的多用户DCSK通信系统性能分析[J]. 电子与信息学报, 2018, 40(11): 2744–2751. doi: 10.11999/JEIT180110ZHANG Gang, XU Jiaping, and ZHANG Tianqi. Performance analyze for multiuser-DCSK communication system based on Hilbert transform[J]. Journal of Electronics &Information Technology, 2018, 40(11): 2744–2751. doi: 10.11999/JEIT180110 [17] CAI Guofa, WANG Lin, and CHEN Guanrong. Capacity of the non-coherent DCSK system over Rayleigh fading channel[J]. IET Communications, 2016, 10(18): 2663–2669. doi: 10.1049/iet-com.2016.0487 [18] SUSHCHIK M, TSIMRING L S, and VOLKOVSKII A R. Performance analysis of correlation-based communication schemes utilizing chaos[J]. IEEE Transactions on Circuits and Systems I:Fundamental Theory and Applications, 2002, 47(12): 1684–1691. doi: 10.1109/81.899920 -






 下载:
下载:



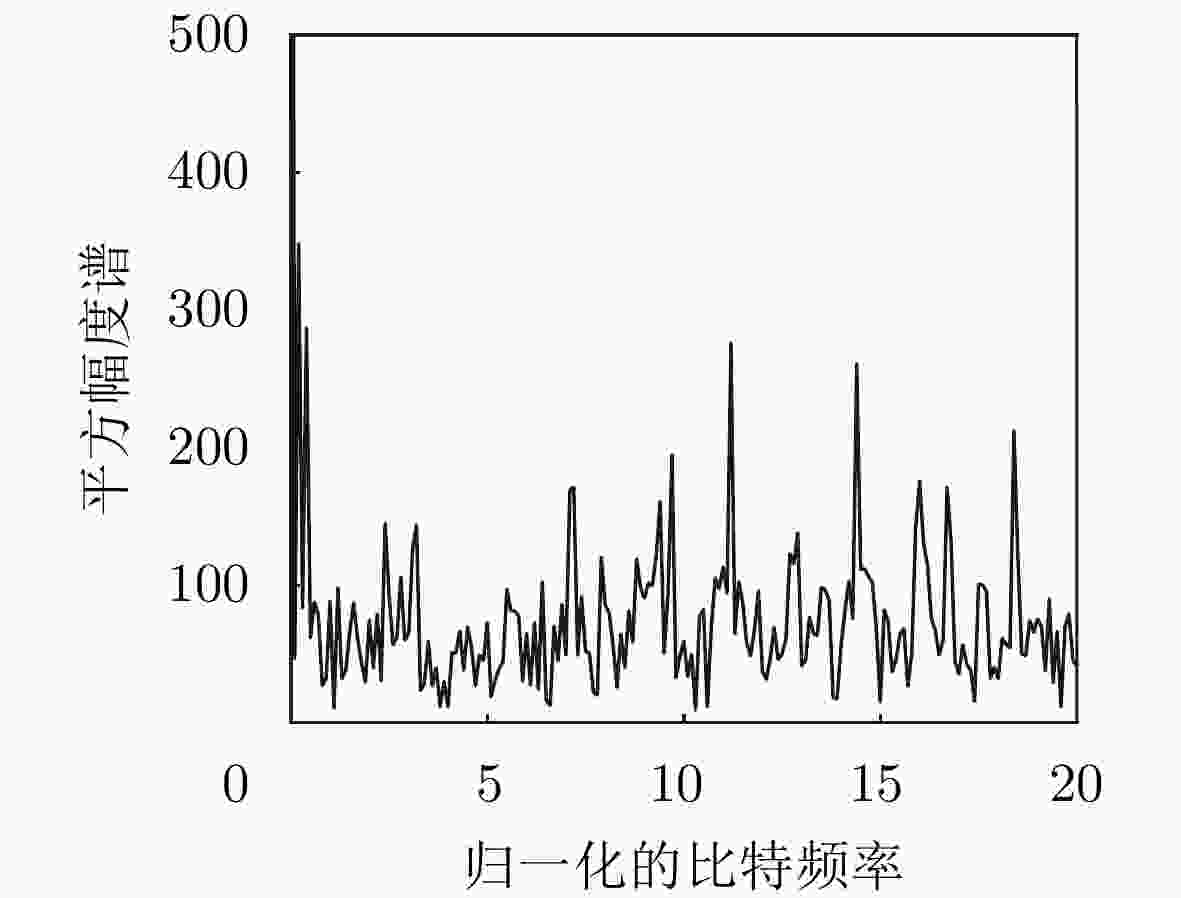
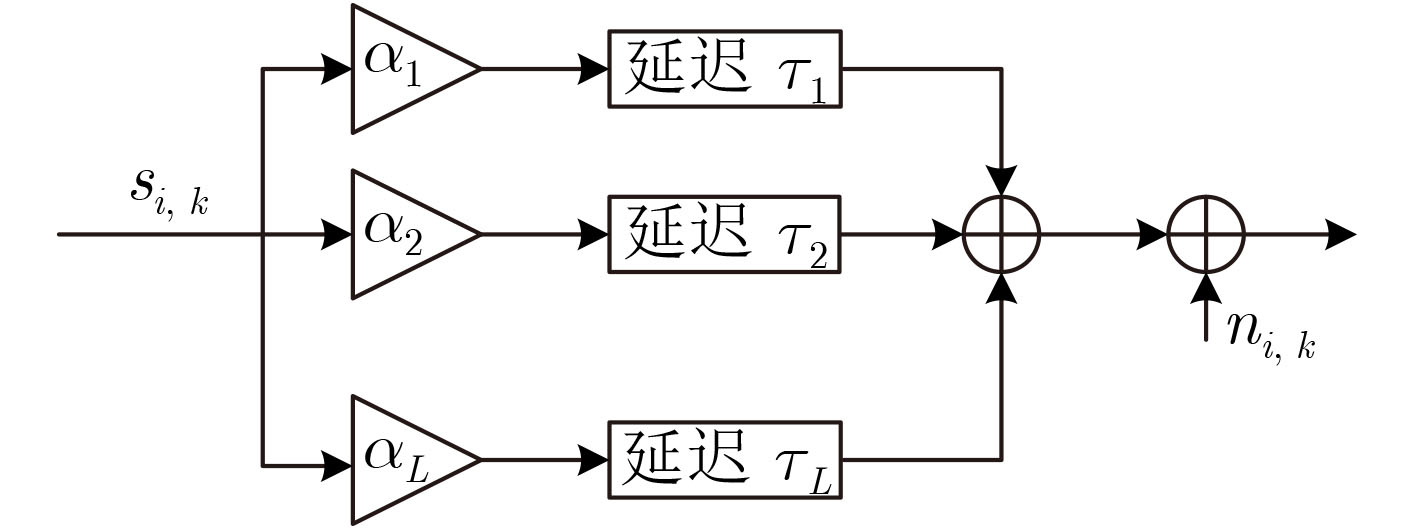


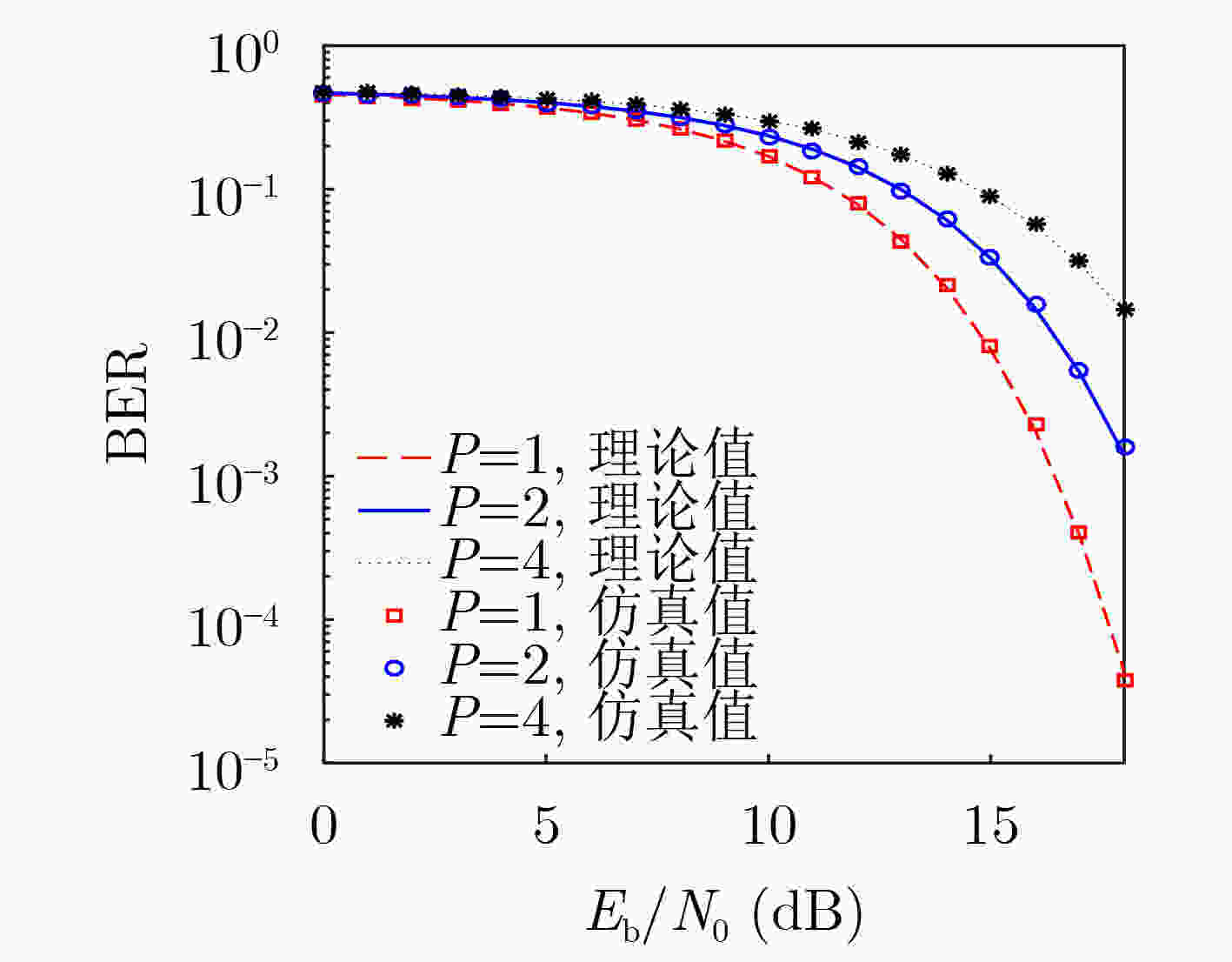





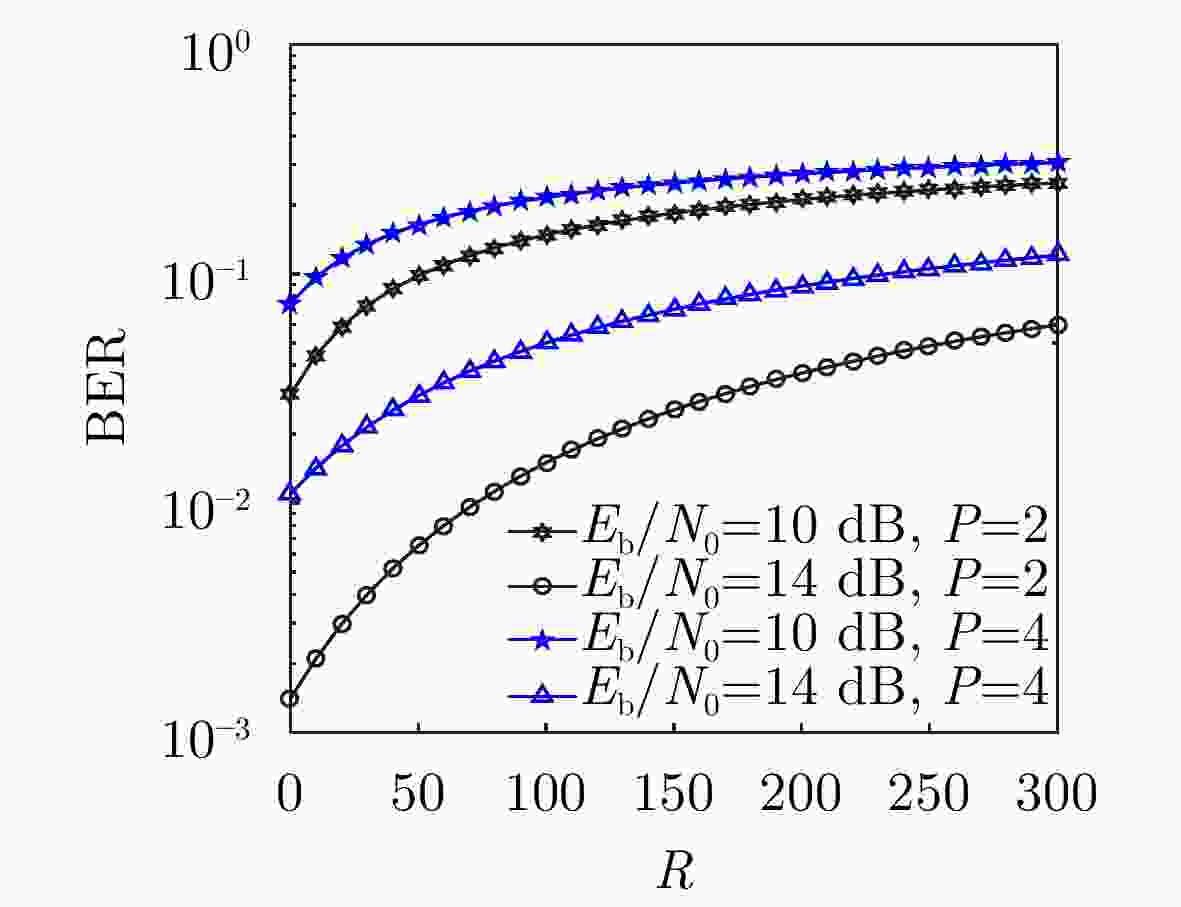




 下载:
下载:
