Robust Energy-efficient Resource Allocation Algorithm in D2D Communication Networks with Imperfect CSI
-
摘要: 针对设备到设备(D2D)直连通信网络传统最优资源分配算法在随机信道时延、信道估计误差影响下鲁棒性弱的问题，该文在考虑参数不确定性影响的条件下，提出D2D用户总能效最大的鲁棒资源分配算法。考虑干扰功率门限、用户最小速率需求、最大传输功率和子信道分配约束，建立了下垫式频谱共享模式下多用户D2D网络资源分配模型。基于有界信道不确定性模型，利用最坏准则方法将原非凸鲁棒资源分配问题转换为确定性的凸优化问题。然后利用拉格朗日对偶理论求得资源分配的解析解。仿真结果表明所提出的算法具有很好的鲁棒性。Abstract: Due to the impact of random channel delays and channel estimation errors, traditional optimal resource allocation algorithms in Device-to-Device (D2D) communication networks have weak robustness. In this paper, a robust resource allocation algorithm for the energy-efficient maximization of D2D users is proposed under parametric uncertainties. Specifically, a multi-user resource allocation model in the D2D network with an underlay spectrum sharing mode is established under the constraints of the interference power threshold, the minimum rate requirement, the maximum transmit power, and the sub-channel allocation. Based on the bounded channel uncertainty models, the original non-convex robust resource allocation problem is converted into a deterministic and convex one by using the worst-case approach. Accordingly, the analytical solution of the robust resource allocation problem is obtained by using Lagrange dual theory. Simulation results demonstrate the proposed algorithm has good robustness.
-
算法1 基于次梯度的鲁棒资源分配算法 1. 初始化系统参数$M$, $N$, ${P_{\rm{c}}}$, $r_n^{\min }$, $I_m^{{\rm{th}}}$, $p_{n,m}^{\max }$, ${\delta _{n,m}}$, $\upsilon _{n,m}^{\rm{C}}$, $\varepsilon _{n,m}^{\rm{D}}$和$\sigma _{\rm{D}}^2$； 2. 初始化外层最大迭代次数${L_{\max }}$和收敛精度${\psi _O}$，初始化能效${\theta ^0}$和传输功率$p_{n,m}^0$，外层迭代次数置零：$l \leftarrow 0$； 3. while $\quad \Big|\displaystyle\sum\nolimits_{n = 1}^N {\displaystyle\sum\nolimits_{m = 1}^M {\hat r_{n,m}^{ {\rm{D} },l} } } \Big/\left(\displaystyle\sum\nolimits_{n = 1}^N {\displaystyle\sum\nolimits_{m = 1}^M { {\alpha _{n,m} }p_{n,m}^l} } + {P_{\rm{c} } }\right)$$ - {\theta ^{l - 1} }| \le {\psi _O} $ or
$l \le {L_{\max } }$, do4. 初始化内层最大迭代次数${T_{\max }}$和内层收敛精度${\psi _I}$，内层迭代次数置零：$t \leftarrow 0$，初始化拉格朗日乘子$\varpi _m^0$, $\varphi _{n,m}^0$, $\beta _n^0$和$\lambda _m^0$；初始化步长$d_{\rm{1}}^0$, $d_2^0$, $d_3^0$和$d_4^0$； 5. while $\left| {{f^{t + 1}} - {f^t}} \right| > {\psi _I}(f = \varpi _m^{},\varphi _{n,m}^{},\beta _n^{},\lambda _m^{})$ or
$t \le {T_{\max } }$, do6. for $n = 1:1:N$ 7. for $m = 1:1:M$ 8. 根据式(24)计算最优传输功率${p_{n,m}}$； 9. 根据式(26)和式(27)更新子载波分配因子${\alpha _{n,m}}$； 10. 根据式(28)-式(31)更新拉格朗日乘子$\varphi _{n,m}^t$, $\varpi _m^t$, $\beta _n^t$和$\lambda _m^t$； 11. end for 12. end for 13. 更新内层迭代次数$t \leftarrow t + 1$； 14. end while 15. 更新外层迭代次数$l \!\leftarrow\! l \!+\! 1$和${\theta ^l} \!=\!\displaystyle\sum\nolimits_{n = 1}^N {\displaystyle\sum\nolimits_{m = 1}^M {\hat r_{n,m}^{ {\rm{D} },l - 1} } } \Big/ $$ \left(\displaystyle\sum\nolimits_{n = 1}^N {\displaystyle\sum\nolimits_{m = 1}^M { {\alpha _{n,m} }p_{n,m}^{l - 1} } } + {P_{\rm{c} } }\right)$； 16. end while 17. output $p_{n,m}^*$, $\alpha _{n,m}^*$和${\theta ^*}$. 
下载: 导出CSV
-
[1] WAQAS M, NIU Yong, LI Yong, et al. A comprehensive survey on mobility-aware D2D communications: Principles, practice and challenges[J]. IEEE Communications Surveys & Tutorials, 2020, 22(3): 1863–1886. doi: 10.1109/COMST.2019.2923708 [2] AHMED M, LI Yong, WAQAS M, et al. A Survey on socially aware device-to-device communications[J]. IEEE Communications Surveys & Tutorials, 2018, 20(3): 2169–2197. doi: 10.1109/COMST.2018.2820069 [3] LIU Xiaonan, LI Zan, ZHAO Nan, et al. Transceiver design and multihop D2D for UAV IoT coverage in disasters[J]. IEEE Internet of Things Journal, 2019, 6(2): 1803–1815. doi: 10.1109/JIOT.2018.2877504 [4] 钱志鸿, 王雪. 面向5G通信网的D2D技术综述[J]. 通信学报, 2016, 37(7): 1–14. doi: 10.11959/j.issn.1000-436x.2016129QIAN Zhihong and WANG Xue. Reviews of D2D technology for 5G communication networks[J]. Journal on Communications, 2016, 37(7): 1–14. doi: 10.11959/j.issn.1000-436x.2016129 [5] LAI Weikuang, WANG Youchiun, LIN H C, et al. Efficient resource allocation and power control for LTE-A D2D communication with pure D2D model[J]. IEEE Transactions on Vehicular Technology, 2020, 69(3): 3202–3216. doi: 10.1109/TVT.2020.2964286 [6] AMJAD M, REHMANI M H, and MAO Shiwen. Wireless multimedia cognitive radio networks: A comprehensive survey[J]. IEEE Communications Surveys & Tutorials, 2018, 20(2): 1056–1103. doi: 10.1109/COMST.2018.2794358 [7] AWIN F, ABDEL-RAHEEM E, and TEPE K. Blind spectrum sensing approaches for interweaved cognitive radio system: A tutorial and short course[J]. IEEE Communications Surveys & Tutorials, 2019, 21(1): 238–259. doi: 10.1109/COMST.2018.2863681 [8] 钱志鸿, 蒙武杰, 王雪, 等. 全负载蜂窝网络下多复用D2D通信功率分配算法研究[J]. 电子与信息学报, 2020, 42(12): 2939–2945. doi: 10.11999/JEIT190974QIAN Zhihong, MENG Wujie, WANG Xue, et al. Research on power allocation algorithm of multi-to-one multiplexing D2D communication underlaying full load cellular networks[J]. Journal of Electronics &Information Technology, 2020, 42(12): 2939–2945. doi: 10.11999/JEIT190974 [9] SULTANA A, ZHAO Lian, and FERNANDO X. Efficient resource allocation in device-to-device communication using cognitive radio technology[J]. IEEE Transactions on Vehicular Technology, 2017, 66(11): 10024–10034. doi: 10.1109/TVT.2017.2743058 [10] WAQAS M, ASLAM S, ALI Z, et al. Resource optimization for cognitive radio based device to device communication under an energy harvesting scenario[J]. IEEE Access, 2020, 8: 24862–24872. doi: 10.1109/ACCESS.2020.2970464 [11] WU Xiaolu, CHEN Yueyun, YUAN Xiaopan, et al. Joint resource allocation and power control for cellular and device-to-device multicast based on cognitive radio[J]. IET Communications, 2014, 8(16): 2805–2813. doi: 10.1049/iet-com.2013.1041 [12] CHENG Peng, DENG Lei, YU Hui, et al. Resource allocation for cognitive networks with D2D communication: An evolutionary approach[C]. 2012 IEEE Wireless Communications and Networking Conference (WCNC), Paris, France, 2012: 2671–2676. [13] 谢显中, 田瑜, 姚鑫凌, 等. 认知网络中D2D全双工通信的速率最大化功率分配算法[J]. 电子与信息学报, 2017, 39(4): 1002–1006. doi: 10.11999/JEIT160593XIE Xianzhong, TIAN Yu, YAO Xinling, et al. Power allocation algorithm of maximizing rate for D2D full-duplex communication in cognitive networks[J]. Journal of Electronics &Information Technology, 2017, 39(4): 1002–1006. doi: 10.11999/JEIT160593 [14] ZAPPONE A, MATTHIESEN B, and JORSWIECK E A. Energy efficiency in MIMO underlay and overlay device-to-device communications and cognitive radio systems[J]. IEEE Transactions on Signal Processing, 2017, 65(4): 1026–1041. doi: 10.1109/TSP.2016.2626249 [15] ZHOU Zhenyu, MA Guifang, XU Chen, et al. Energy-efficient resource allocation in cognitive D2D communications: A game-theoretical and matching approach[C]. 2016 IEEE International Conference on Communications (ICC), Kuala Lumpur, Malaysia, 2016: 814–819. [16] XU Yongjun, ZHAO Xiaohui, and LIANG Yingchang. Robust power control and beamforming in cognitive radio networks: A survey[J]. IEEE Communications Surveys & Tutorials, 2015, 17(4): 1834–1857. doi: 10.1109/COMST.2015.2425040 [17] BEN-TAL A and NEMIROVSKI A. Robust convex optimization[J]. Mathematics of Operations Research, 1998, 23(4): 769–805. doi: 10.1287/moor.23.4.769 [18] PARK M J and KWON O M. Stability and stabilization of discrete-time T-S fuzzy systems with time-varying delay via Cauchy-Schwartz-based summation inequality[J]. IEEE Transactions on Fuzzy Systems, 2017, 25(1): 128–140. doi: 10.1109/TFUZZ.2016.2551290 [19] FANG Fang, CHENG Julian, and DING Zhiguo. Joint energy efficient subchannel and power optimization for a downlink NOMA heterogeneous network[J]. IEEE Transactions on Vehicular Technology, 2019, 68(2): 1351–1364. doi: 10.1109/TVT.2018.2881314 [20] DINKELBACH W. On nonlinear fractional programming[J]. Management Science, 1967, 13(7): 492–498. doi: 10.1287/mnsc.13.7.492 [21] BOYD S and VANDENBERGHE L. Convex Optimization[M]. Cambridge: Cambridge University Press, 2004. [22] ZHANG Haijun, JIANG Chunxiao, BEAULIEU N C, et al. Resource allocation in spectrum-sharing OFDMA femtocells with heterogeneous services[J]. IEEE Transactions on Communications, 2014, 62(7): 2366–2377. doi: 10.1109/TCOMM.2014.2328574 [23] BAI Zhiquan, LI Mengqi, DONG Yanan, et al. Joint fair resource allocation of D2D communication underlaying downlink cellular system with imperfect CSI[J]. IEEE Access, 2018, 6: 63131–63142. doi: 10.1109/ACCESS.2018.2873364 [24] HU Jinming, HENG Wei, LI Xiang, et al. Energy-efficient resource reuse scheme for D2D communications underlaying cellular networks[J]. IEEE Communications Letters, 2017, 21(9): 2097–2100. doi: 10.1109/LCOMM.2017.2711490 -
图(9) / 表(1)
计量
- 文章访问数: 1248
- HTML全文浏览量: 537
- PDF下载量: 108
- 被引次数: 0
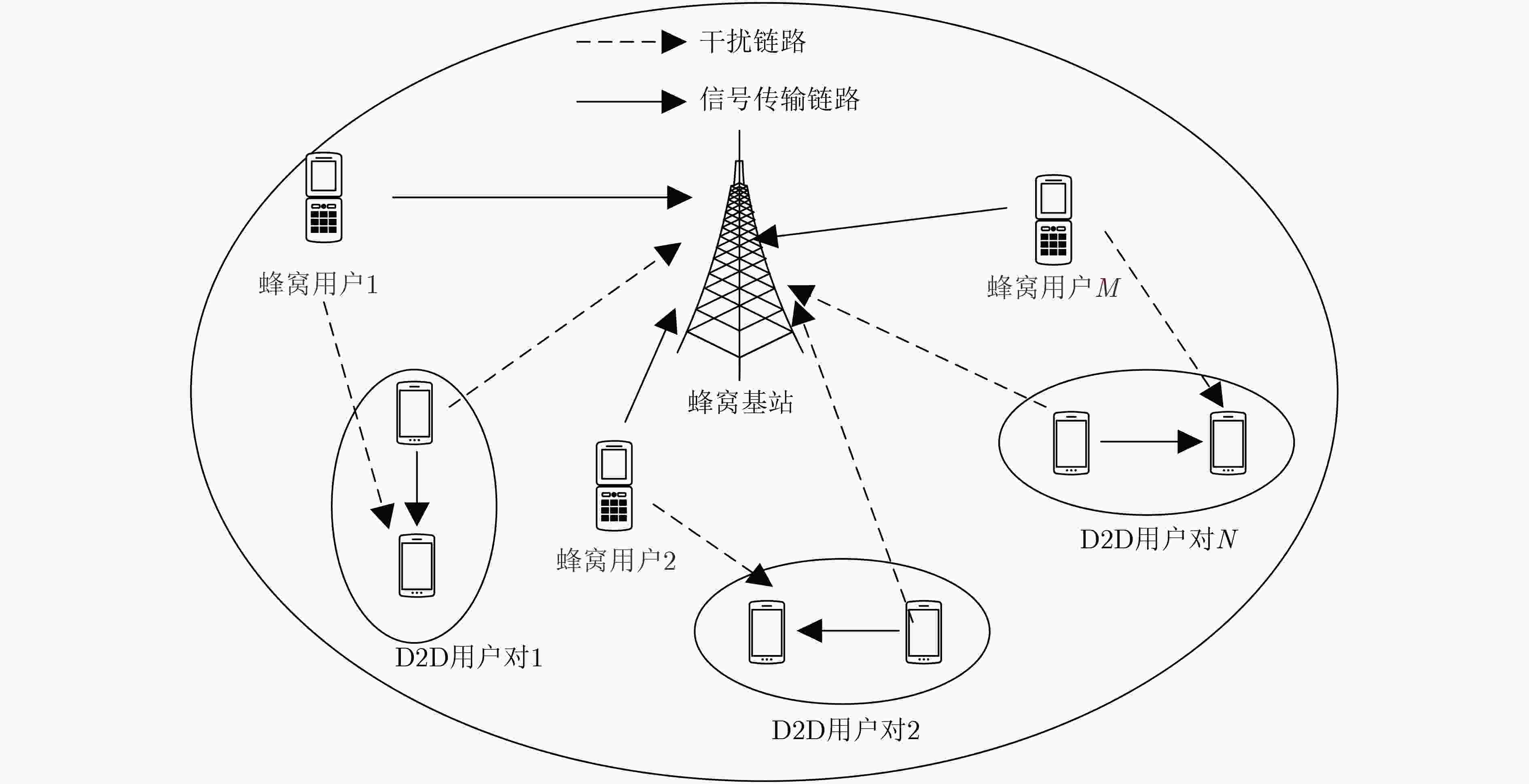
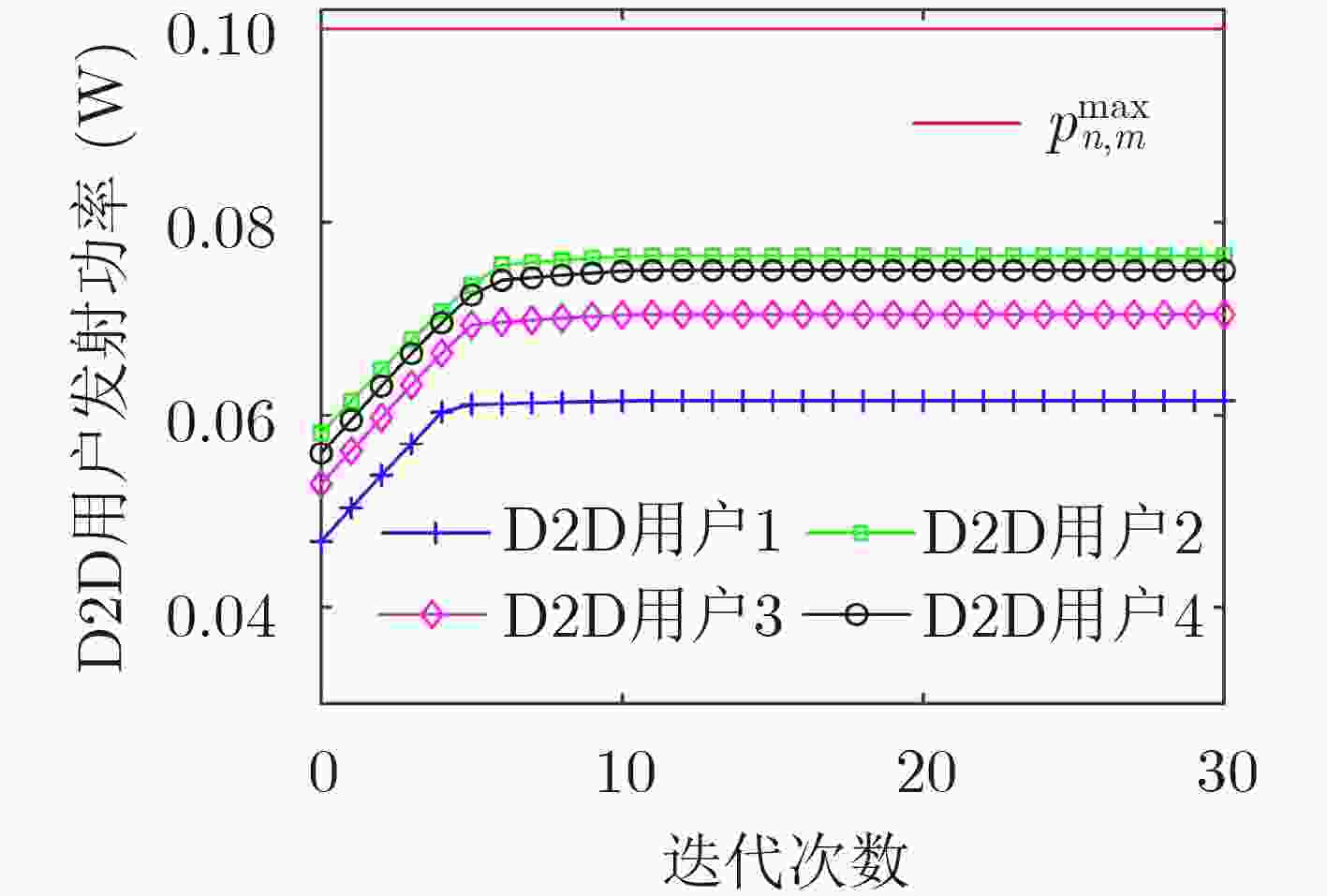
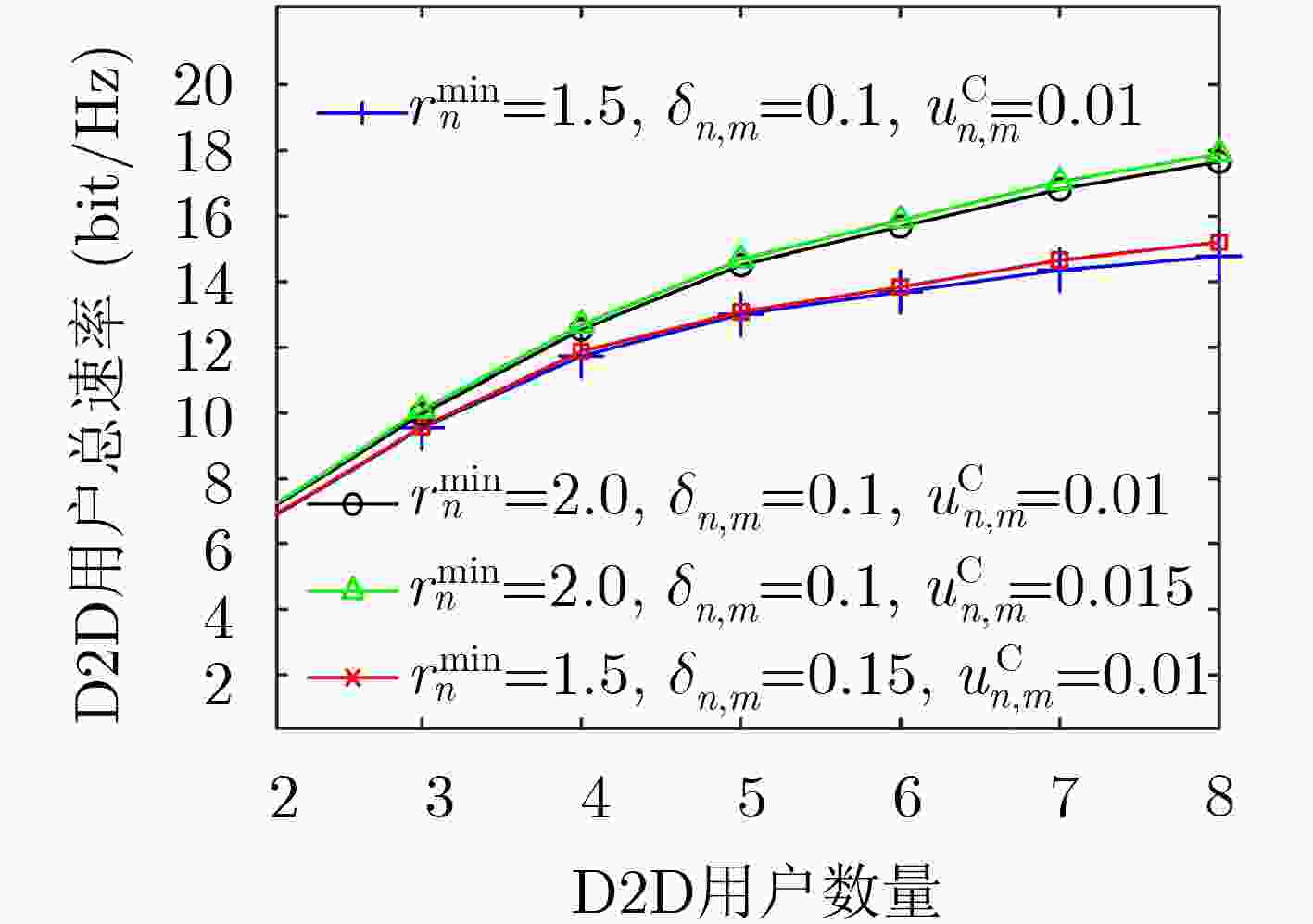
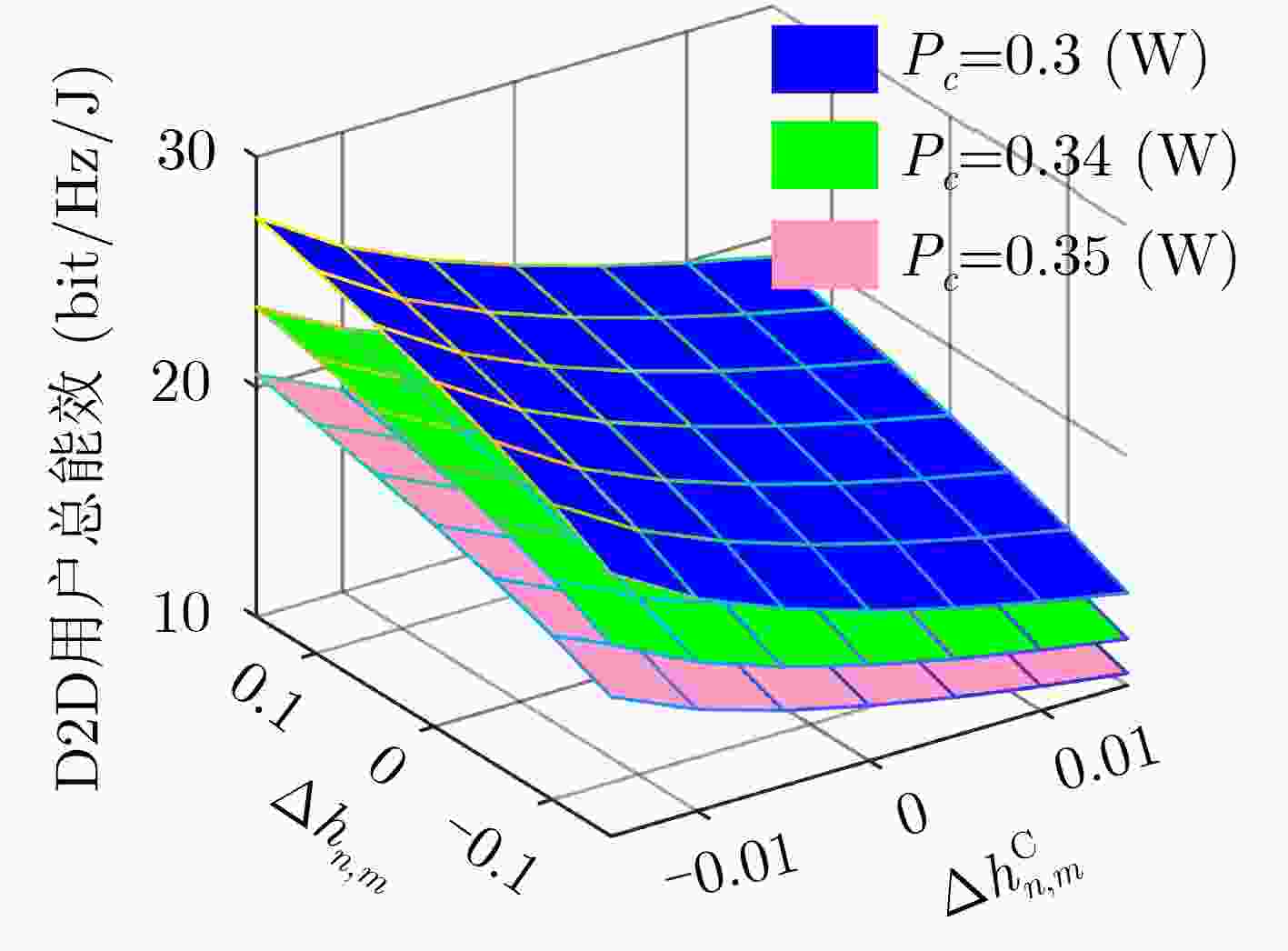
下载:
