One Dimensional High Resolution Range Imaging Method of Stepped Frequency ISAR Based on Atomic Norm Minimization
-
摘要: 针对传统离散化压缩感知方法在网格失配条件下步进频率(SF) ISAR 1维距离成像估计性能下降的问题，该文提出一种基于原子范数最小化(ANM)的高分辨距离成像方法。首先，构建基于原子范数的无网格SF ISAR距离向稀疏表示模型，将1维距离成像问题转化为原子系数以及频率估计问题。然后，利用原子范数半正定性质，将原子范数最小化问题转化为半正定规划问题，并基于交替方向乘子法实现快速求解。最后，利用Vandermonde分解得到最终的1维高分辨距离成像结果。由于避免了网格离散化处理，因此可以实现网格失配、低量测值条件下的高分辨距离成像，且保持了高的距离分辨能力。理论分析与仿真实验验证了所提方法的有效性。Abstract: In order to solve the problem that the performance of one-dimensional range profile synthesis performance of Stepped Frequency (SF) ISAR based on the traditional discrete compressed sensing method declines under the condition of off-grid, a high-resolution range profile synthesis method of SF ISAR based on Atomic Norm Minimization (ANM) is proposed. Firstly, a grid free SF ISAR range sparse representation model based on atomic norm is constructed, and the one-dimensional range synthesis problem is transformed into the atomic coefficient and frequency estimation problem. Then, the atomic norm minimization problem is transformed into a semi-positive definite programming problem by using the semi-positive definite property of the atomic norm, and the fast solvers are performed via the alternating direction method of multipliers. Finally, the final one-dimensional high-resolution range profile imaging results are obtained by Vandermonde decomposition. Because the grid discretization is avoided, the high-resolution range profile imaging can be realized under the condition of grid mismatch and low measurement, and the high range resolution can be maintained. Theoretical analysis and simulation experiments verify the effectiveness of the proposed method.
-
[1] WANG Lei, HUANG Tianyao, and LIU Yimin. Phase compensation and image autofocusing for randomized stepped frequency ISAR[J]. IEEE Sensors Journal, 2019, 19(10): 3784–3796. doi: 10.1109/JSEN.2019.2897014 [2] 陈怡君, 李开明, 张群, 等. 稀疏线性调频步进信号ISAR成像观测矩阵自适应优化方法[J]. 电子与信息学报, 2018, 40(3): 509–516. doi: 10.11999/JEIT170554CHEN Yijun, LI Kaiming, ZHANG Qun, et al. Adaptive measurement matrix optimization for ISAR imaging with sparse frequency-stepped chirp signals[J]. Journal of Electronics &Information Technology, 2018, 40(3): 509–516. doi: 10.11999/JEIT170554 [3] 龙腾, 丁泽刚, 肖枫, 等. 星载高分辨频率步进SAR成像技术[J]. 雷达学报, 2019, 8(6): 782–792. doi: 10.12000/JR19076LONG Teng, DING Zegang, XIAO Feng, et al. Spaceborne high-resolution stepped-frequency SAR imaging technology[J]. Journal of Radars, 2019, 8(6): 782–792. doi: 10.12000/JR19076 [4] GHAFARI M, SABAHI M F, and ZHANG Zhenkai. Difference set coding in stepped frequency radar[C]. 6th Iranian Conference on Radar and Surveillance Systems, Isfahan, Iran, 2019: 1–6. doi: 10.1109/ICRSS48293.2019.9026562. [5] WEI Shaopeng, ZHANG Lei, MA Hui, et al. Sparse frequency waveform optimization for high-resolution ISAR Imaging[J]. IEEE Transactions on Geoscience and Remote Sensing, 2020, 58(1): 546–566. doi: 10.1109/TGRS.2019.2937965 [6] GANGULY S, GHOSH I, RANJAN R, et al. Compressive sensing based off-grid DOA estimation using OMP algorithm[C]. The 6th International Conference on Signal Processing and Integrated Networks (SPIN), Noida, India, 2019. doi: 10.1109/SPIN.2019.8711677. [7] HU Lei, SHI Zhiguang, ZHOU Jianxiong, et al. Compressed sensing of complex sinusoids: An approach based on dictionary refinement[J]. IEEE Transactions on Signal Processing, 2012, 60(7): 3809–3822. doi: 10.1109/TSP.2012.2193392 [8] 王伟, 胡子英, 龚琳舒. MIMO雷达三维成像自适应Off-grid校正方法[J]. 电子与信息学报, 2019, 41(6): 1294–1301. doi: 10.11999/JEIT180145WANG Wei, HU Ziying, and GONG Linshu. Adaptive off-grid calibration method for MIMO radar 3D imaging[J]. Journal of Electronics &Information Technology, 2019, 41(6): 1294–1301. doi: 10.11999/JEIT180145 [9] EKANADHAM C, TRANCHINA D, and SIMONCELLI E P. Recovery of sparse translation-invariant signals with continuous basis pursuit[J]. IEEE Transactions on Signal Processing, 2011, 59(10): 4735–4744. doi: 10.1109/TSP.2011.2160058 [10] HUANG Limei, ZONG Zhulin, HUANG Libing, et al. Off-grid sparse stepped-frequency SAR imaging with adaptive basis[C]. 2019 IEEE International Geoscience and Remote Sensing Symposium, Yokohama, Japan, 2019: 2925–2928. doi: 10.1109/IGARSS.2019.8898543. [11] YANG Zai, XIE Lihua, and ZHANG Cishen. Off-grid direction of arrival estimation using sparse Bayesian inference[J]. IEEE Transactions on Signal Processing, 2013, 61(1): 38–43. doi: 10.1109/TSP.2012.2222378 [12] TANG Gongguo, BHASKAR B N, SHAH P, et al. Compressed sensing off the grid[J]. IEEE Transactions on Information Theory, 2013, 59(11): 7465–7490. doi: 10.1109/TIT.2013.2277451 [13] YANG Zai and XIE Lihua. Continuous compressed sensing with a single or multiple measurement vectors[C]. 2014 IEEE Workshop on Statistical Signal Processing, Gold Coast, Australia, 2014: 288–291. doi: 10.1109/SSP.2014.6884632. [14] CHANDRASEKARAN V, RECHT B, PARRILO P A, et al. The convex algebraic geometry of linear inverse problems[C]. The 48th Annual Allerton Conference on Communication, Control, and Computing, Allerton, USA, 2010: 699–703. doi: 10.1109/ALLERTON.2010.5706975. [15] 吕明久, 陈文峰, 夏塞强, 等. 基于联合块稀疏模型的随机调频步进ISAR成像方法[J]. 电子与信息学报, 2018, 40(11): 2614–2620. doi: 10.11999/JEIT180054LÜ Mingjiu, CHEN Wenfeng, XIA Saiqiang, et al. Random chirp frequency-stepped signal ISAR imaging algorithm based on joint block-sparse model[J]. Journal of Electronics &Information Technology, 2018, 40(11): 2614–2620. doi: 10.11999/JEIT180054 [16] BHASKAR B N, TANG Gongguo, and RECHT B. Atomic norm denoising with applications to line spectral estimation[J]. IEEE Transactions on Signal Processing, 2013, 61(23): 5987–5999. doi: 10.1109/TSP.2013.2273443 [17] HANSEN T L and JENSEN T L. A fast interior-point method for atomic norm soft thresholding[J]. Signal Processing, 2019, 165: 7–19. doi: 10.1016/j.sigpro.2019.06.023 [18] BOYD S, PARIKH N, CHU E, et al. Distributed optimization and statistical learning via the alternating direction method of multipliers[J]. Foundations and Trends® in Machine Learning, 2011, 3(1): 1–122. doi: 10.1561/2200000016 [19] GEORGIOU T T. The Carathéodory-Fejér-Pisarenko decomposition and its multivariable counterpart[J]. IEEE Transactions on Automatic Control, 2007, 52(2): 212–228. doi: 10.1109/TAC.2006.890479 [20] YANG Zai and XIE Lihua. On gridless sparse methods for line spectral estimation from complete and incomplete data[J]. IEEE Transactions on Signal Processing, 2015, 63(12): 3139–3153. doi: 10.1109/tsp.2015.2420541 [21] ZHANG Zhe, WANG Yue, and TIAN Zhi. Efficient two-dimensional line spectrum estimation based on decoupled atomic norm minimization[J]. Signal Processing, 2019, 163: 95–106. doi: 10.1016/j.sigpro.2019.04.024 [22] LI Yinchuan, WANG Xiaodong, and DING Zegang. Multi-dimensional spectral super-resolution with prior knowledge via frequency-selective Vandermonde decomposition and ADMM[EB/OL]. https://arxiv.org/abs/1906.00278, 2019. -
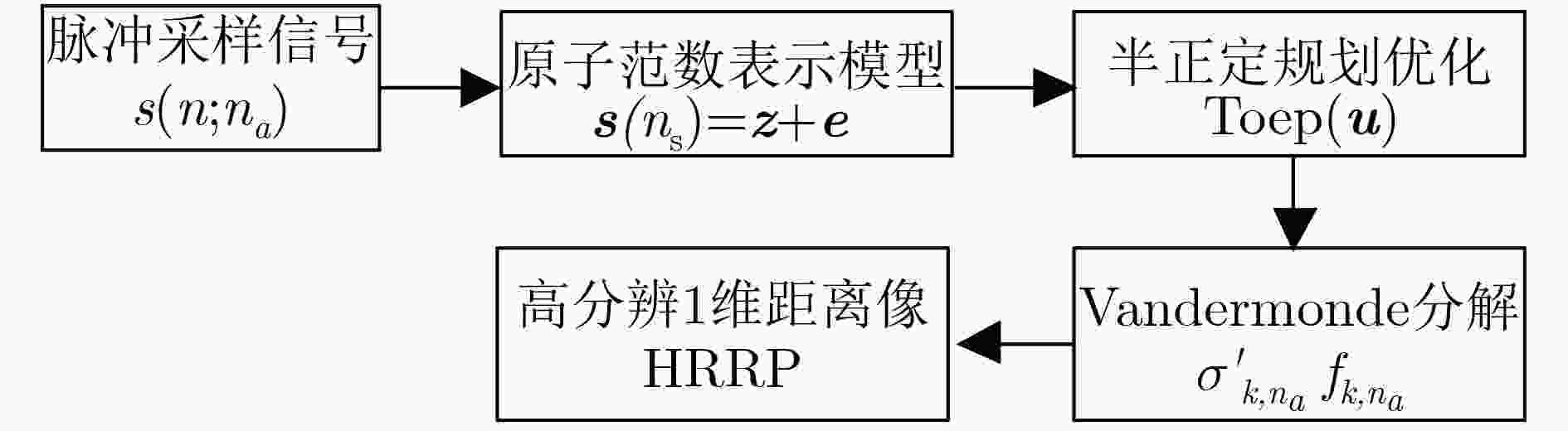
下载:
图(5)
计量
- 文章访问数: 1047
- HTML全文浏览量: 543
- PDF下载量: 96
- 被引次数: 0
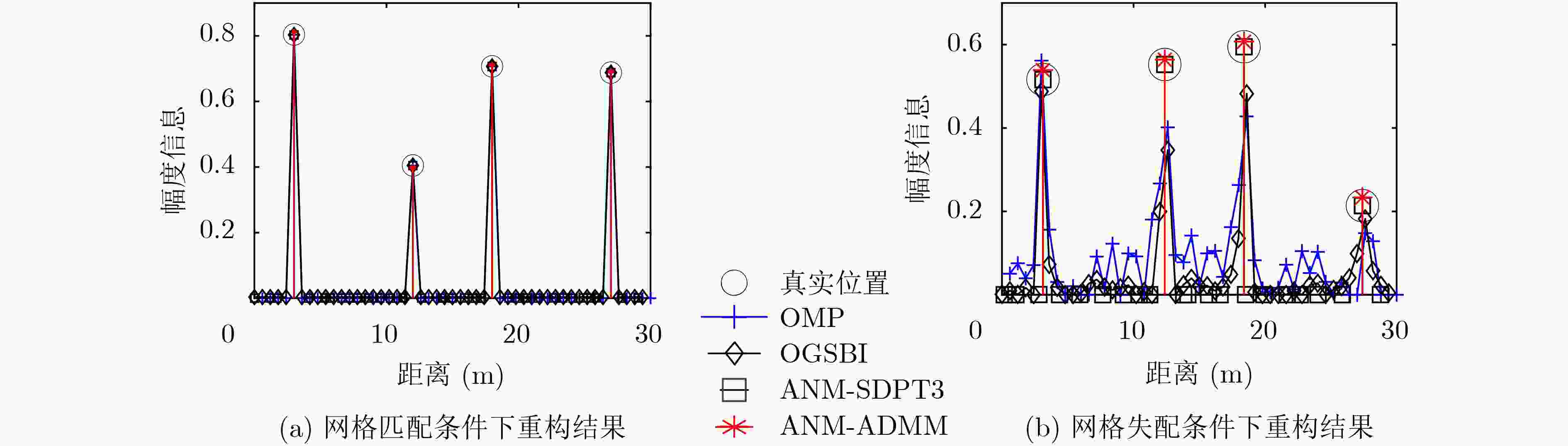
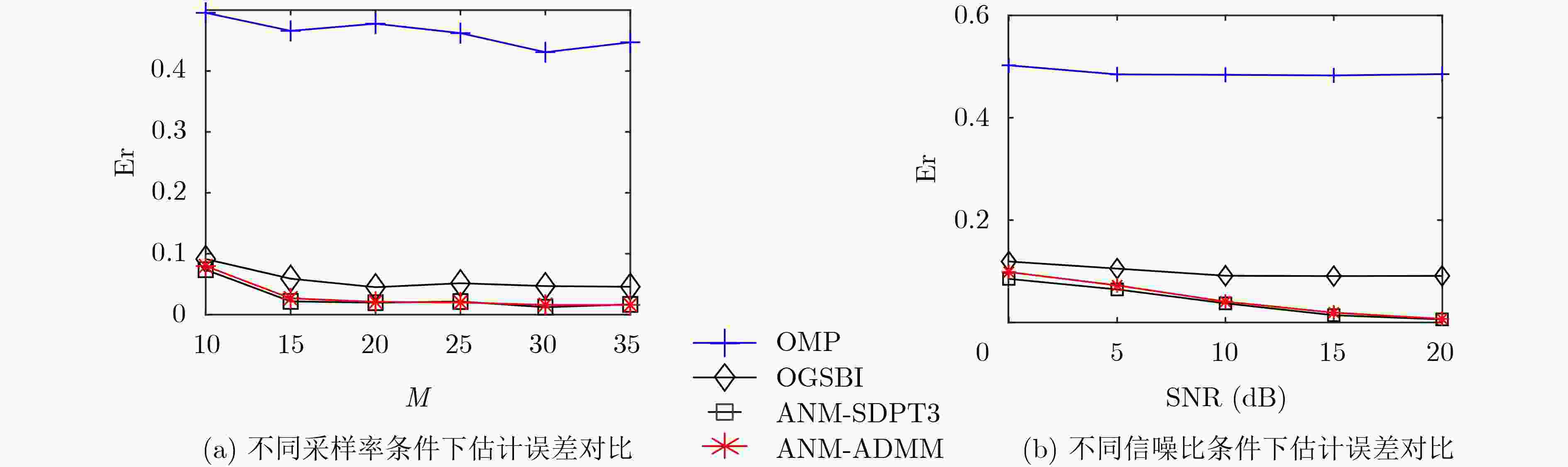
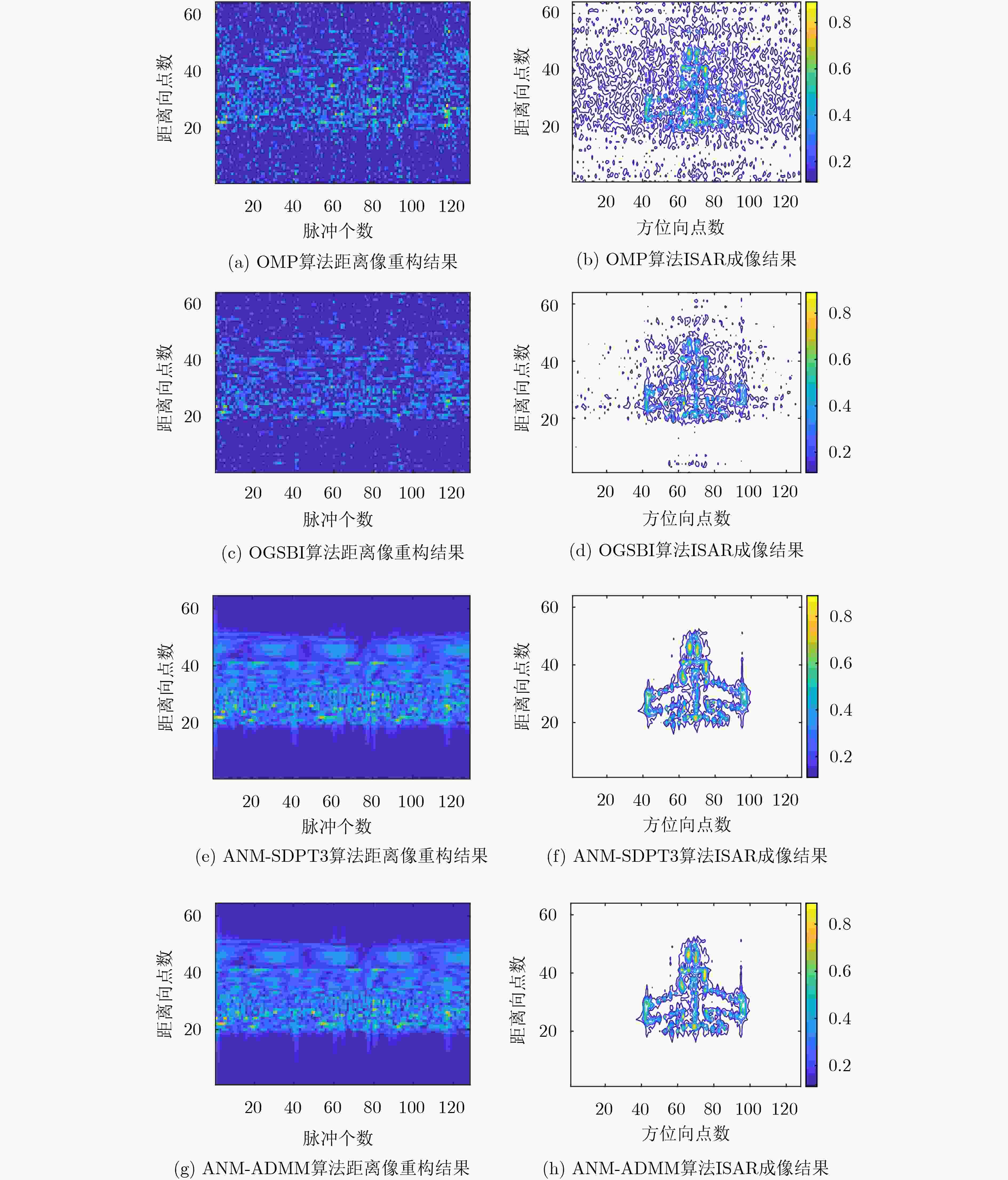
下载:
