A Novel DOA Estimation Method for Coherently Distributed Sources Based on Correntropy in the Impulsive Noise
-
摘要: 针对复杂电磁环境下被动无线监测定位问题，该文提出广义相关熵的概念，推导了广义相关熵的性质，用以抑制阵列输出信号中的脉冲噪声。为了实现脉冲噪声环境下相干分布源中心DOA和扩散角的联合估计，提出基于广义相关熵的DOA估计新方法，并证明了该方法的有界性。为进一步提升算法的鲁棒性，推导了一种仅依赖阵列输出信号的自适应核函数。仿真结果表明，该算法能够实现脉冲噪声环境下相干分布源参数的联合估计，相比已有算法，具有更高的估计精度和鲁棒性。Abstract: To solve the problem of passive wireless monitoring and positioning in complex electromagnetic environments, a generalized auto-correntropy for suppressing the impulsive noise in the array output signals is proposed and its properties are derived. To obtain the estimates of both central Direction Of Arrival (DOA) and angular spread for coherently distributed sources in the impulsive noise, a novel DOA estimation method based on the generalized auto-correntropy is proposed, and its boundedness is proved. To improve the robustness of the proposed algorithm, a new adaptive kernel function, which only depends on the array output signals, is also derived. The simulation results show that the proposed algorithm can obtain the joint estimation for coherently distributed sources under impulsive noise environments, and has higher estimation accuracy and robustness than existing algorithms.
-
SHEN Qing, LIU Wei, CUI Wei, et al. Underdetermined DOA estimation under the compressive sensing framework: A review[J]. IEEE Access, 2016, 4: 8865–8878. doi: 10.1109/ACCESS.2016.2628869 ZHAI Hui, ZHANG Xiaofei, ZHENG Wang, et al. DOA estimation of noncircular signals for unfolded coprime linear array: Identifiability, DOF and algorithm (May 2018)[J]. IEEE Access, 2018, 6: 29382–29390. doi: 10.1109/ACCESS.2018.2835563 LIN Jianfeng and ZHANG Xiaofei. Direction of arrival estimation of quasi-stationary signals using unfolded coprime array[J]. IEEE Access, 2017, 5: 6538–6545. doi: 10.1109/ACCESS.2017.2695581 SHU Feng, QIN Yaolu, LIU Tingting, et al. Low-complexity and high-resolution DOA estimation for hybrid analog and digital massive MIMO receive array[J]. IEEE Transactions on Communications, 2018, 66(6): 2487–2501. doi: 10.1109/TCOMM.2018.2805803 SCHMIDT R. Multiple emitter location and signal parameter estimation[J]. IEEE Transactions on Antennas and Propagation, 1986, 34(3): 276–280. doi: 10.1109/TAP.1986.1143830 TSAKALIDES P and NIKIAS C L. Robust space-time adaptive processing (STAP) in non-Gaussian clutter environments[J]. IEE Proceedings–Radar, Sonar and Navigation, 1999, 146(2): 84–93. doi: 10.1049/ip-rsn:19990233 NIKIAS C L and SHAO Min. Signal Processing with Alpha-stable Distributions and Applications[M]. New York, USA: John Wiley & Sons, Inc., 1995: 20–55. 马济通, 邱天爽, 李蓉, 等. 脉冲噪声下基于Renyi熵的分数低阶双模盲均衡算法[J]. 电子与信息学报, 2018, 40(2): 378–385. doi: 10.11999/JEIT170366MA Jitong, QIU Tianshuang, LI Rong, et al. Dual-mode blind equalization algorithm based on Renyi entropy and fractional lower order statistics under impulsive noise[J]. Journal of Electronics &Information Technology, 2018, 40(2): 378–385. doi: 10.11999/JEIT170366 TSAKALIDES P and NIKIAS C L. High-resolution autofocus techniques for SAR imaging based on fractional lower-order statistics[J]. IEE Proceedings - Radar, Sonar and Navigation, 2001, 148(5): 267–276. doi: 10.1049/ip-rsn:20010457 LIU T H and MENDEL J M. A subspace-based direction finding algorithm using fractional lower order statistics[J]. IEEE Transactions on Signal Processing, 2001, 49(8): 1605–1613. doi: 10.1109/78.934131 BELKACEMI H and MARCOS S. Robust subspace-based algorithms for joint angle/Doppler estimation in non-Gaussian clutter[J]. Signal Processing, 2007, 87(7): 1547–1558. doi: 10.1016/j.sigpro.2006.12.015 LIU Weifeng, POKHAREL P P, and PRINCIPE J C. Correntropy: Properties and applications in non-Gaussian signal processing[J]. IEEE Transactions on Signal Processing, 2007, 55(11): 5286–5298. doi: 10.1109/TSP.2007.896065 ZHANG Jinfeng, QIU Tianshuang, SONG Aimin, et al. A novel correntropy based DOA estimation algorithm in impulsive noise environments[J]. Signal Processing, 2014, 104: 346–357. doi: 10.1016/j.sigpro.2014.04.033 VALAEE S, CHAMPAGNE B, and KABAL P. Parametric localization of distributed sources[J]. IEEE Transactions on Signal Processing, 1995, 43(9): 2144–2153. doi: 10.1109/78.414777 MENG Y, STOICA P, and WONG K M. Estimation of the directions of arrival of spatially dispersed signals in array processing[J]. IEE Proceedings–Radar, Sonar and Navigation, 1996, 143(1): 1–9. doi: 10.1049/ip-rsn:19960170 邱天爽. 相关熵与循环相关熵信号处理研究进展[J]. 电子与信息学报, 2020, 42(1): 105–118. doi: 10.11999/JEIT190646QIU Tianshuang. Development in signal processing based on correntropy and cyclic correntropy[J]. Journal of Electronics &Information Technology, 2020, 42(1): 105–118. doi: 10.11999/JEIT190646 GREENE W H. Econometric Analysis[M]. Englewood Cliffs, NJ, US: Prentice Hall Inc., 2011: 80–120. CHIANI M, DARDARI D, and SIMON M K. New exponential bounds and approximations for the computation of error probability in fading channels[J]. IEEE Transactions on Wireless Communications, 2003, 2(4): 840–845. doi: 10.1109/TWC.2003.814350 CHANG S H, COSMAN P C, and MILSTEIN L B. Chernoff-type bounds for the Gaussian error function[J]. IEEE Transactions on Communications, 2011, 59(11): 2939–2944. doi: 10.1109/TCOMM.2011.072011.100049 TSAKALIDES P and NIKIAS C L. Maximum likelihood localization of sources in noise modeled as a stable process[J]. IEEE Transactions on Signal Processing, 1995, 43(11): 2700–2713. doi: 10.1109/78.482119 ZHANG Q T. Probability of resolution of the MUSIC algorithm[J]. IEEE Transactions on Signal Processing, 1995, 43(4): 978–987. doi: 10.1109/78.376849 TIAN Quan, QIU Tianshuang, LI Jingchun, et al. Robust adaptive DOA estimation method in an impulsive noise environment considering coherently distributed sources[J]. Signal Processing, 2019, 165: 343–356. doi: 10.1016/j.sigpro.2019.07.014 -
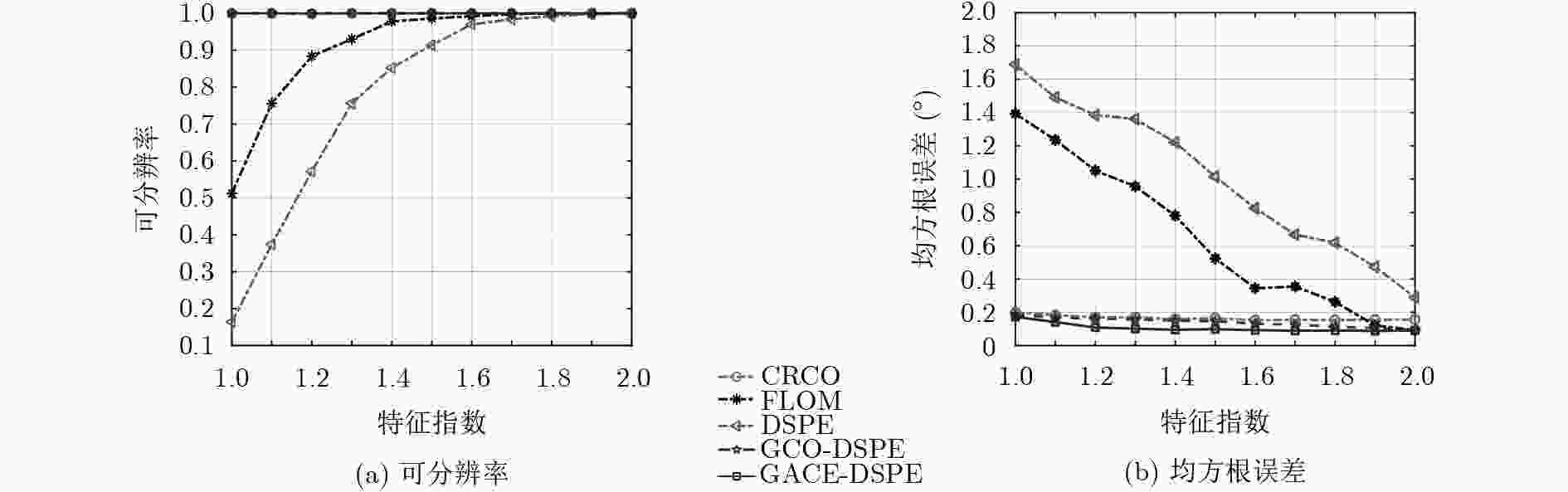
下载:
图(3)
计量
- 文章访问数: 1020
- HTML全文浏览量: 341
- PDF下载量: 79
- 被引次数: 0
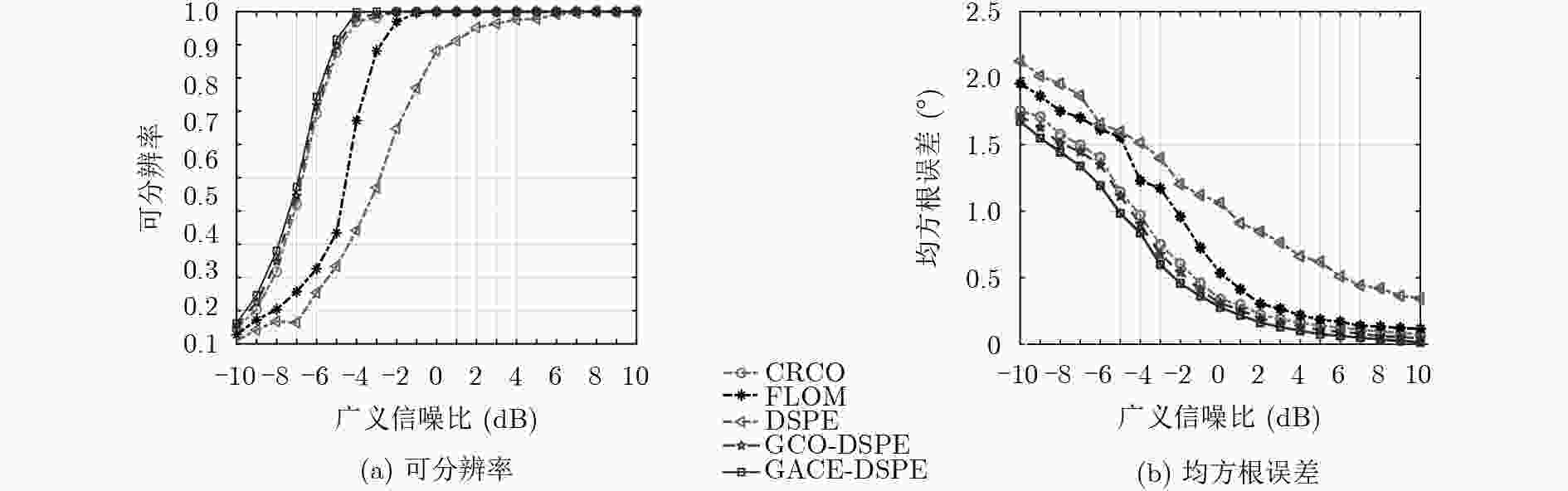
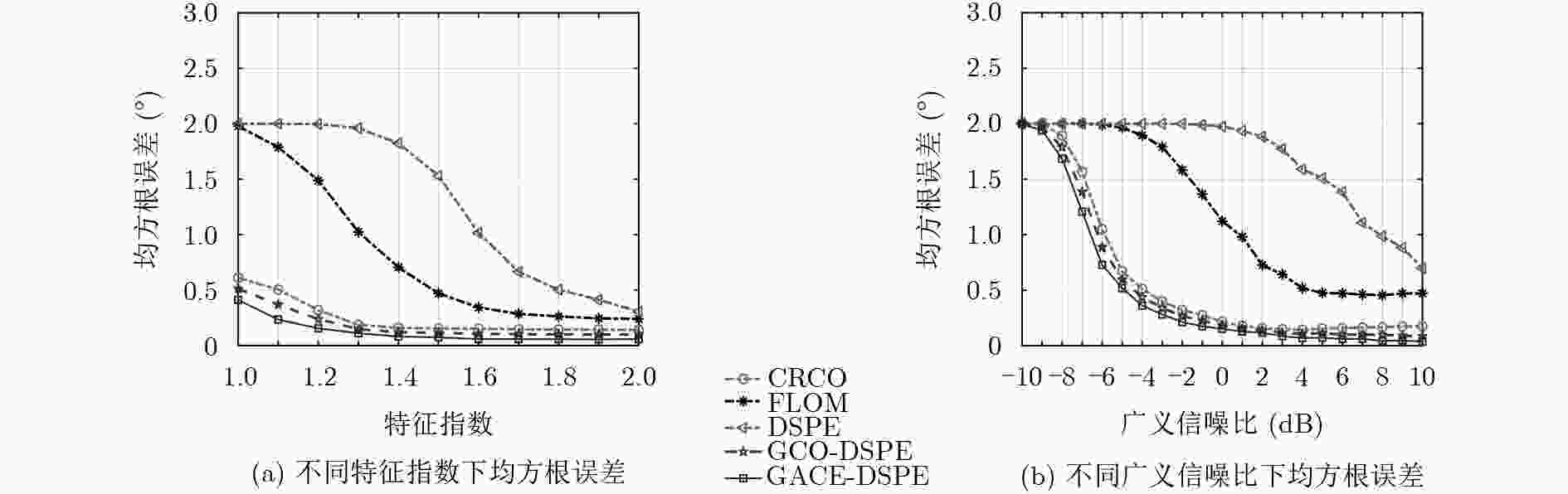
下载:
