| [1] |
POLUEKTOV A, PINOMAA A, ROMANENKO A, et al. Sensitivity analysis of a PLC-based DSSS anti-islanding system in power distribution grids[J]. International Journal of Electrical Power & Energy Systems, 2019, 113: 739–747. doi: 10.1016/j.ijepes.2019.06.022
|
| [2] |
FENG Jining, YANG Xiaobo, and WANG Jun. Novel LFM jammer suppression algorithm for DSSS in FRFT domain[J]. Journal of Beijing Institute of Technology, 2019, 28(4): 783–789. doi: 10.15918/j.jbit1004-0579.18088
|
| [3] |
张天骐, 袁帅, 刘董华, 等. 高动态环境下高阶双二进制偏移载波信号的精确捕获[J]. 电子与信息学报, 2018, 40(11): 2728–2735. doi: 10.11999/JEIT180087ZHANG Tianqi, YUAN Shuai, LIU Donghua, et al. Accurate acquisition of High order double binary offset carrier signals for high dynamic environment[J]. Journal of Electronics &Information Technology, 2018, 40(11): 2728–2735. doi: 10.11999/JEIT180087
|
| [4] |
王航, 郭静波, 王赞基. 混沌多进制直接序列扩频信号的盲解扩[J]. 清华大学学报: 自然科学版, 2009, 49(1): 13–16.WANG Hang, GUO Jingbo, and WANG Zanji. Chaotic M-ary direct sequence spread spectrum signals blind despreading[J]. Journal of Tsinghua University:Science and Technology, 2009, 49(1): 13–16.
|
| [5] |
ZHOU Feng, LIU Bing, NIE Donghu, et al. M-ary cyclic shift keying spread spectrum underwater acoustic communications based on virtual time-reversal mirror[J]. Sensors, 2019, 19(16): 3577. doi: 10.3390/s19163577
|
| [6] |
LIU Fang and JIA Shiyao. Decomposition-combination correlation decision mechanism for MBOK signals[J]. Wireless Personal Communications, 2020, 110(1): 207–221. doi: 10.1007/s11277-019-06721-3
|
| [7] |
朱亮, 裴玉奎, 葛宁, 等. 超宽带信道下MBOK调制系统可达速率分析[J]. 清华大学学报: 自然科学版, 2011, 51(3): 400–404.ZHU Liang, PEI Yukui, GE Ning, et al. Achievable rate analysis of MBOK modulation system over UWB channels[J]. Journal of Tsinghua University:Natural and Technology, 2011, 51(3): 400–404.
|
| [8] |
BAE J N, KIM J Y, KIM G, et al. Performance of TZCD-MBOK watermarking scheme in T-DMB systems[J]. Multimedia Tools and Applications, 2012, 57(2): 359–372. doi: 10.1007/s11042-011-0740-2
|
| [9] |
齐琳, 郭黎利. 并行组合扩频非等概超宽带系统误码性能研究[J]. 系统工程与电子技术, 2011, 33(3): 659–664. doi: 10.3969/j.issn.1001-506X.2011.03.38QI Lin and GUO Lili. BER performance study of non-equal probability UWB system based on parallel combinatory spread spectrum[J]. Systems Engineering and Electronics, 2011, 33(3): 659–664. doi: 10.3969/j.issn.1001-506X.2011.03.38
|
| [10] |
刘明夺, 郭黎利, 姜晓斐. 并行组合扩频系统中Gold序列代数和相关性及优选[J]. 北京邮电大学学报, 2013, 36(4): 33–38. doi: 10.13190/jbupt.201304.31.liumdLIU Mingduo, GUO Lili, and JIANG Xiaofei. Correlation of superposition Gold sequences and optimized sequences selection in parallel combinatory spread spectrum system[J]. Journal of Beijing University of Posts and Telecommunications, 2013, 36(4): 33–38. doi: 10.13190/jbupt.201304.31.liumd
|
| [11] |
BASAR E. Index modulation techniques for 5G wireless networks[J]. IEEE Communications Magazine, 2016, 54(7): 168–175. doi: 10.1109/MCOM.2016.7509396
|
| [12] |
JAIN M, AGARWAL A, RAWAL D, et al. Adaptive bit and power allocation for dual mode index modulation based OFDM system[J]. Physical Communication, 2020, 40: 101093. doi: 10.1016/j.phycom.2020.101093
|
| [13] |
DATTA T, ESHWARAIAH H S, and CHOCKALINGAM A. Generalized space-and-frequency index modulation[J]. IEEE Transactions on Vehicular Technology, 2016, 65(7): 4911–4924. doi: 10.1109/TVT.2015.2451095
|
| [14] |
KADDOUM G, AHMED M F A, and NIJSURE Y. Code index modulation: A high data rate and energy efficient communication system[J]. IEEE Communications Letters, 2015, 19(2): 175–178. doi: 10.1109/LCOMM.2014.2385054
|
| [15] |
KADDOUM G and SOUJERI E. On the comparison between code-index modulation and spatial modulation techniques[C]. 2015 International Conference on Information and Communication Technology Research, Abu Dhabi, United Arab Emirates, 2015: 24–27. doi: 10.1109/ICTRC.2015.7156412.
|
| [16] |
KADDOUM G, NIJSURE Y, and TRAN H. Generalized code index modulation technique for high-data-rate communication systems[J]. IEEE Transactions on Vehicular Technology, 2016, 65(9): 7000–7009. doi: 10.1109/TVT.2015.2498040
|
| [17] |
XU Weikai, HUANG Tingting, and WANG Lin. Code-shifted differential chaos shift keying with code index modulation for high data rate transmission[J]. IEEE Transactions on Communications, 2017, 65(10): 4285–4294. doi: 10.1109/TCOMM.2017.2725261
|
| [18] |
TAN Yunsheng, XU Weikai, HUANG Tingting, et al. A multilevel code shifted differential chaos shift keying scheme with code index modulation[J]. IEEE Transactions on Circuits and Systems II: Express Briefs, 2018, 65(11): 1743–1747. doi: 10.1109/TCSII.2017.2764916
|
| [19] |
葛利嘉, 江治林, 冯胜, 等. 非正交-码索引调制方法[J]. 电子与信息学报, 2018, 40(10): 2331–2336. doi: 10.11999/JEIT180023GE Lijia, JIANG Zhilin, FENG Sheng, et al. Non-orthogonal-code index modulation[J]. Journal of Electronics &Information Technology, 2018, 40(10): 2331–2336. doi: 10.11999/JEIT180023
|
| [20] |
冯胜, 杨勤, 郑鹤, 等. 广义空—码联合索引调制[J]. 计算机应用研究, 2019, 36(7): 2112–2115. doi: 10.19734/j.issn.1001-3695.2018.01.0046.FENG Sheng, YANG Qin, ZHENG He, et al. Generalized space code joint index modulation[J]. Application Research of Computers, 2019, 36(7): 2112–2115. doi: 10.19734/j.issn.1001-3695.2018.01.0046.
|





 下载:
下载:




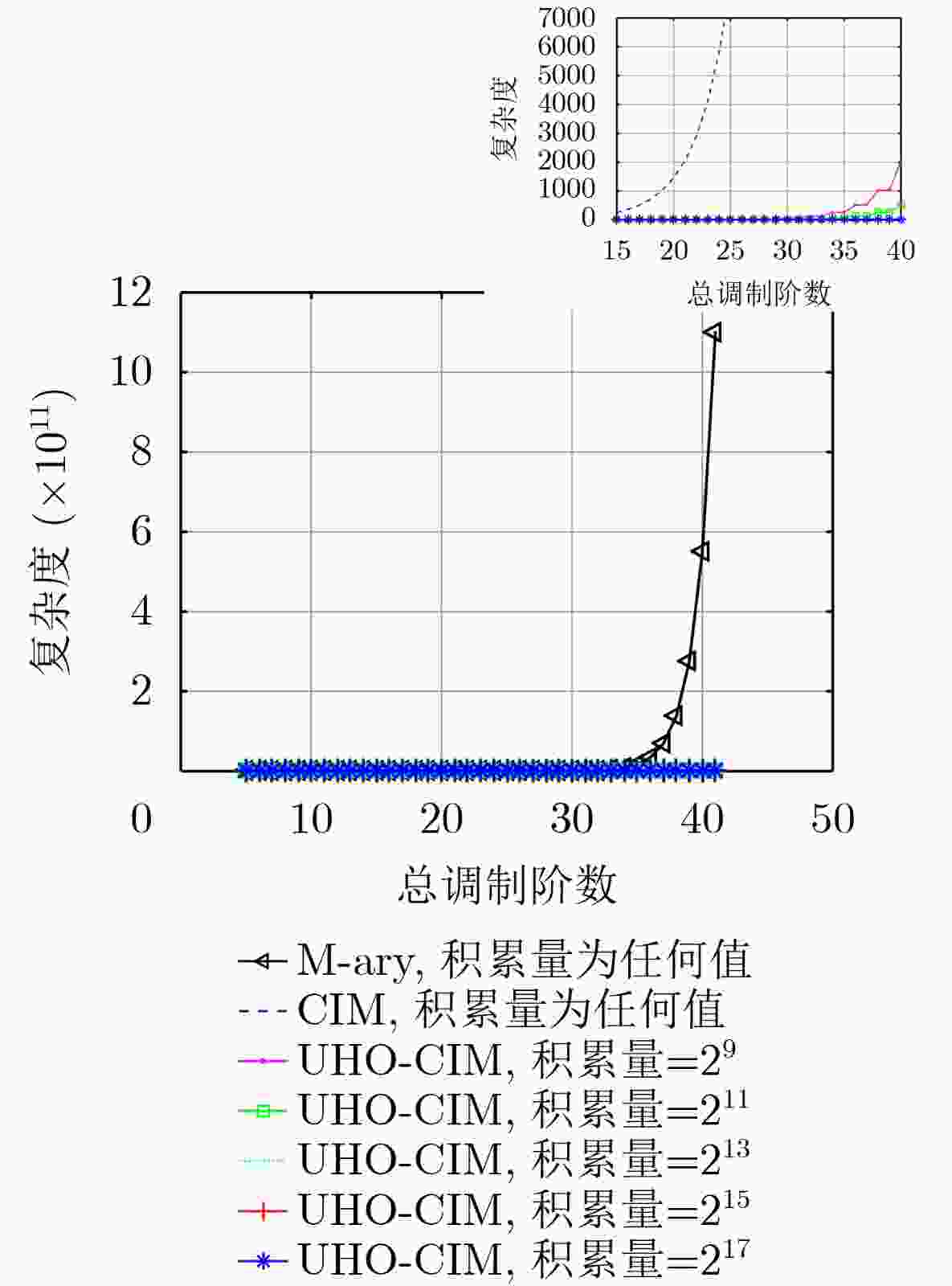

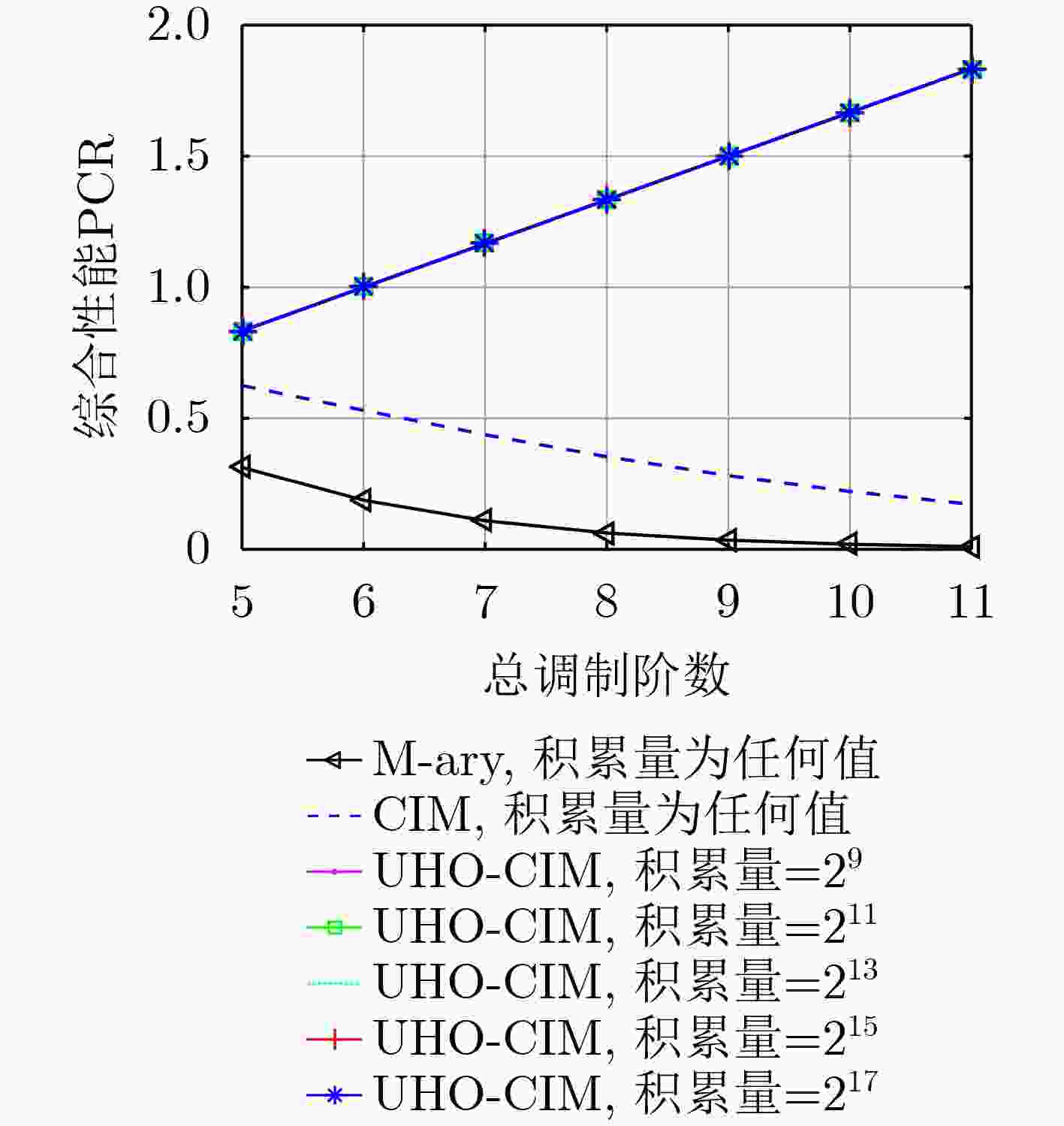

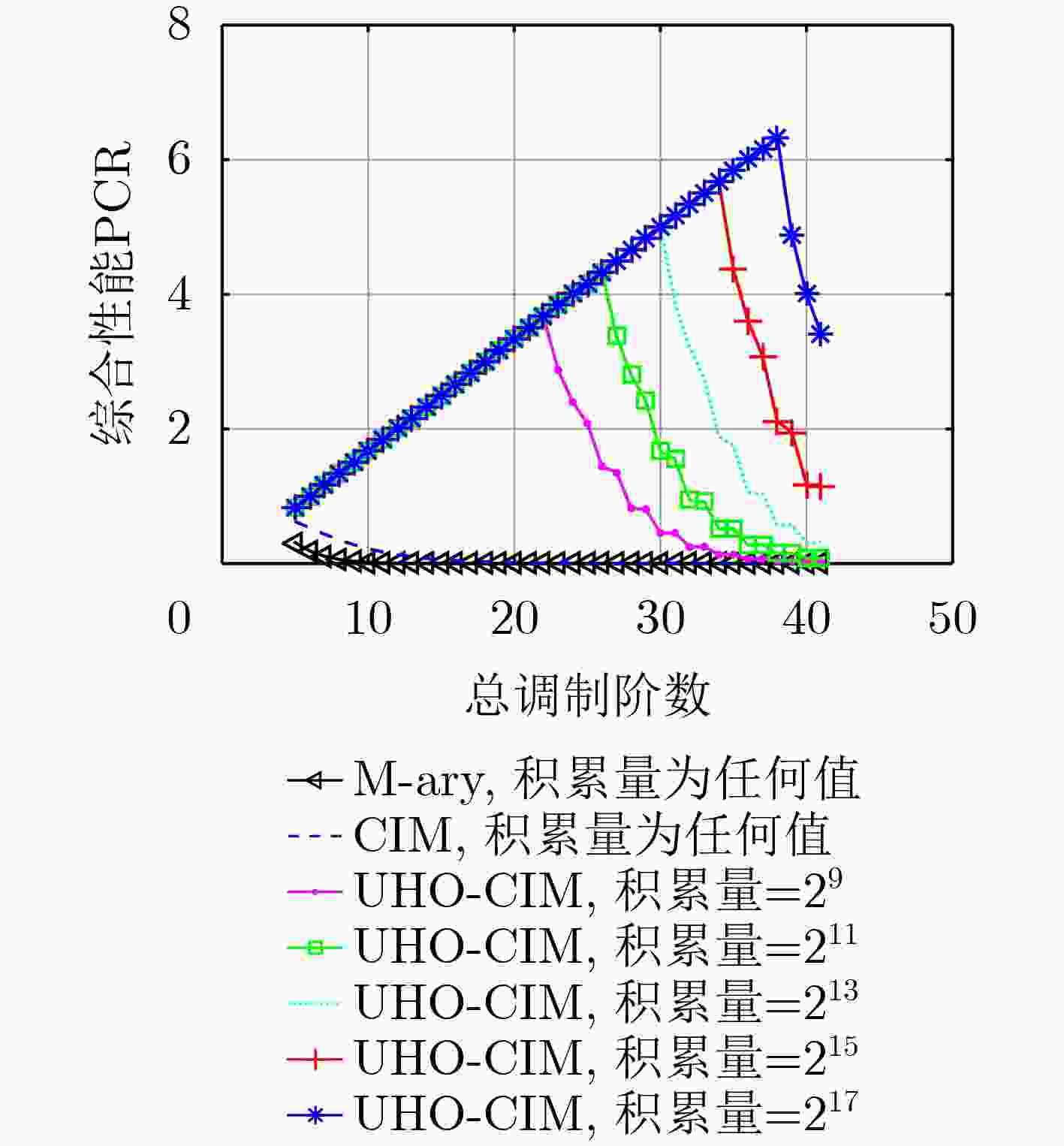

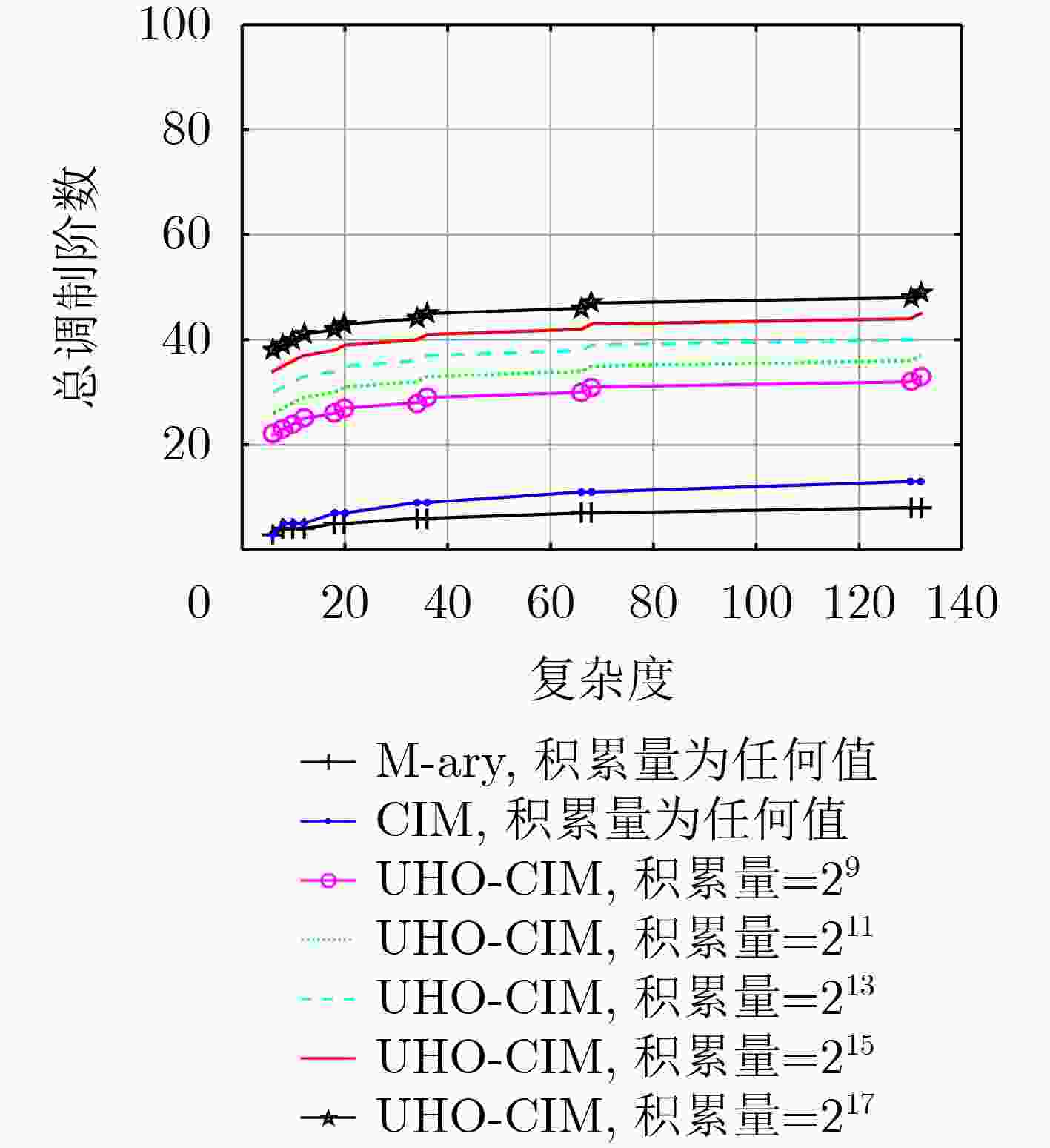


 下载:
下载:
