Asynchronous Anti-bias Track Association Algorithm of Radar and Electronic Support Measurements
-
摘要: 针对雷达与电子支援措施(ESM)存在系统误差、航迹异步等复杂条件下的航迹关联问题，该文提出一种基于区间序列离散度的异步抗差航迹关联算法。定义混合区间序列的离散信息度量，给出系统误差的区间化方法，通过计算区间离散度并利用经典分配法进行关联判定。与传统算法相比，可在系统误差存在的前提下无需时域配准对异步航迹直接关联，且对噪声分布不敏感。仿真结果表明，所提算法具有良好的抗差性且不受目标运动位置的影响，适用于传感器同地或异地配置等多种情况。Abstract: To address track association problem of radar and Electronic Support Measurements (ESM) under complex conditions such as system biases and asynchronous track, an asynchronous anti-bias track association algorithm based on discrete degree of interval sequence is proposed. The discrete information measurement of mixed interval sequence is defined and the interval method of system biases is given. The association determination is performed by calculating the interval discrete degree and using classical assignment method. Compared with the traditional algorithm, the asynchronous track can be directly correlated without time-domain registration in the presence of system errors and it is not sensitive to noise distribution. The simulation results show that the algorithm has good robust performance and is not affected by the target moving position. The algorithm can be applied to the configuration of sensors at same or different sites.
-
Key words:
- Radar /
- Electronic Support Measurements (ESM) /
- Track association /
- System bias /
- Asynchronous track /
- Discrete degree
-
表 1 雷达与ESM最大系统误差取值表
实验编号 1 2 3 4 5 6 $\Delta \theta _{\rm{m}}^s(^\circ )$ 0.5 0.5 1.0 1.5 0.5 0.5 $\Delta \theta _{\rm{m}}^w(^\circ )$ 0.5 0.5 0.5 0.5 1.0 1.5 $\Delta \rho _{\rm{m}}^s({\rm{m)}}$ 500 1000 500 500 500 500 
下载: 导出CSV
表 2 不同系统误差下的算法关联率对比
实验编号 本文算法 文献[9]算法 文献[13]算法 文献[14]算法 Ec Ee Es Ec Ee Es Ec Ee Es Ec Ee Es 1 0.937 0.056 0.007 0.743 0.075 0.182 0.936 0.028 0.036 0.866 0.023 0.111 2 0.932 0.060 0.008 0.698 0.049 0.253 0.935 0.033 0.032 0.858 0.024 0.118 3 0.914 0.054 0.032 0.504 0.175 0.321 0.900 0.044 0.056 0.817 0.078 0.105 4 0.892 0.056 0.052 0.445 0.243 0.312 0.881 0.058 0.061 0.772 0.026 0.202 5 0.944 0.047 0.009 0.506 0.090 0.404 0.937 0.023 0.040 0.840 0.048 0.112 6 0.933 0.056 0.011 0.456 0.234 0.310 0.925 0.038 0.037 0.787 0.051 0.162
下载: 导出CSV
表 4 不同采样周期和开机时延的正确关联率
ESM开机时延(s) 雷达，ESM采样周期(时间间隔)(s) t1=0.1,t2=0.3 t1=0.3,t2=0.5 t1=0.5,t2=1.1 Δt=0.1 0.913 0.913 0.834 Δt=0.2 0.922 0.891 0.844 Δt=0.3 0.928 0.890 0.820 Δt=0.4 0.927 0.881 0.831
下载: 导出CSV
-
[1] 何友, 王国宏, 关欣, 等. 信息融合理论及应用[M]. 北京: 电子工业出版社, 2010: 124–146.HE You, WANG Guohong, GUAN Xin, et al. Information Fusion Theory with Applications[M]. Beijing: Publishing House of Electronics Industry, 2010: 124–146. [2] TU Yongiun, HUANG Gaoming, LI Jinghui, et al. Performance analysis of radar and ESM track association algorithms[J]. Applied Mechanics and Materials, 2011, 130–134: 1925–1928. doi: 10.4028/www.scientific.net/AMM.130-134.1925 [3] WANG Guohong, MAO Shiyi, and HE You. Analytical performance evaluation of association of active and passive tracks for airborne sensors[J]. Signal Processing, 2003, 83(5): 973–981. doi: 10.1016/s0165-1684(02)00500-5 [4] OFFER C R. Performance of bearing-only ESM-radar track association[C]. Proceedings of the 9th IET Data Fusion & Target Tracking Conference, London, UK, 2012: 1–6. doi: 10.1049/cp.2012.0409. [5] WANG Guohong, MAO Shiyi, HE You, et al. Triple-threshold radar-to-ESM correlation algorithm when each radar track is specified by different number of measurements[J]. IEE Proceedings - Radar, Sonar and Navigation, 2000, 147(4): 177–181. doi: 10.1049/ip-rsn:20000094 [6] WANG Guohong, HE You, MAO Shiyi, et al. Quadri-threshold algorithm of co-located radar-to-ESM correlation[J]. Chinese Journal of Aeronautics, 2000, 13(2): 86–90. [7] 陈中华, 王国宏, 刘德浩, 等. 基于几何法的雷达与ESM航迹关联算法[J]. 电光与控制, 2012, 19(4): 10–12, 22. doi: 10.3969/j.issn.1671-637X.2012.04.003CHEN Zhonghua, WANG Guohong, LIU Dehao, et al. Radar-ESM track correlation based on geometric method[J]. Electronics Optics &Control, 2012, 19(4): 10–12, 22. doi: 10.3969/j.issn.1671-637X.2012.04.003 [8] 关欣, 彭彬彬, 衣晓. 基于区间重合度的雷达与ESM航迹关联算法[J]. 雷达科学与技术, 2017, 15(1): 61–67. doi: 10.3969/j.issn.1672-2337.2017.01.011GUAN Xin, PENG Binbin, and YI Xiao. Track association algorithm of radar and ESM based on the interval overlap ratio[J]. Radar Science and Technology, 2017, 15(1): 61–67. doi: 10.3969/j.issn.1672-2337.2017.01.011 [9] 宋振宇, 张翔宇, 张光轶. 系统误差对异地配置的雷达和ESM航迹关联的影响[J]. 电光与控制, 2014, 21(3): 42–46. doi: 10.3969/j.issn.1671-637X.2014.03.010SONG Zhenyu, ZHANG Xiangyu, and ZHANG Guangyi. Effect of systematic errors on radar-to-ESM track association at different sites[J]. Electronics Optics &Control, 2014, 21(3): 42–46. doi: 10.3969/j.issn.1671-637X.2014.03.010 [10] 张翔宇, 王国宏, 王娜, 等. 系统误差下异地配置的雷达和电子支援测量航迹关联[J]. 电光与控制, 2012, 19(3): 30–35, 43. doi: 10.3969/j.issn.1671-637X.2012.03.007ZHANG Xiangyu, WANG Guohong, WANG Na, et al. Track association of radar and ESM sensors with systematic errors at different sites[J]. Electronics Optics &Control, 2012, 19(3): 30–35, 43. doi: 10.3969/j.issn.1671-637X.2012.03.007 [11] ZHANG Feihu and KNOLL A. Systematic error modeling and bias estimation[J]. Sensors, 2016, 16(5): 729. doi: 10.3390/s16050729 [12] WANG Guohong, ZHANG Xiangyu, and TAN Shuncheng. Effect of biased estimation on radar-to-ESM track association[J]. Journal of Systems Engineering and Electronics, 2012, 23(2): 188–194. doi: 10.1109/JSEE.2012.00024 [13] 李保珠, 张林, 董云龙, 等. 基于航迹矢量分级聚类的雷达与电子支援措施抗差关联算法[J]. 电子与信息学报, 2019, 41(6): 1310–1316. doi: 10.11999/JEIT180714LI Baozhu, ZHANG Lin, DONG Yunlong, et al. Anti-bias track association algorithm of radar and electronic support measurements based on track vectors hierarchical clustering[J]. Journal of Electronics &Information Technology, 2019, 41(6): 1310–1316. doi: 10.11999/JEIT180714 [14] 关欣, 彭彬彬, 衣晓. 修正极坐标系下雷达与ESM航迹对准关联[J]. 航空学报, 2017, 38(5): 320668. doi: 10.7527/S1000-6893.2016.0287GUAN Xin, PENG Binbin, and YI Xiao. Track alignment-association of radar and ESM in MPC[J]. Acta Aeronautica et Astronautica Sinica, 2017, 38(5): 320668. doi: 10.7527/S1000-6893.2016.0287 [15] 李保珠, 关键, 董云龙. 基于航迹矢量检测的雷达与电子支援设施抗差关联算法[J]. 电子与信息学报, 2019, 41(1): 123–129. doi: 10.11999/JEIT180303LI Baozhu, GUAN Jian, and DONG Yunlong. Anti-bias track association algorithm of radar and electronic support measurements based on track vectors detection[J]. Journal of Electronics &Information Technology, 2019, 41(1): 123–129. doi: 10.11999/JEIT180303 [16] ZHU Hongyan and WANG Chen. Joint track-to-track association and sensor registration at the track level[J]. Digital Signal Processing, 2015, 41: 48–59. doi: 10.1016/j.dsp.2015.03.012 [17] 左燕, 陈志猛, 蔡立平. 基于约束总体最小二乘的单站DOA/TDOA联合误差校正与定位算法[J]. 电子与信息学报, 2019, 41(6): 1317–1323. doi: 10.11999/JEIT180655ZUO Yan, CHEN Zhimeng, and CAI Liping. Single-observer DOA/TDOA Registration and passive localization based on constrained total least squares[J]. Journal of Electronics &Information Technology, 2019, 41(6): 1317–1323. doi: 10.11999/JEIT180655 [18] 衣晓, 杜金鹏. 基于分段序列离散度的异步航迹关联算法[J]. 航空学报, 2020, 41(7): 323694. doi: 10.7527/S1000-6893.2020.23694YI Xiao and DU Jinpeng. Asynchronous track-to-track association algorithm based on discrete degree of segmented sequence[J]. Acta Aeronautica et Astronautica Sinica, 2020, 41(7): 323694. doi: 10.7527/S1000-6893.2020.23694 -
图(6) / 表(5)
计量
- 文章访问数: 1020
- HTML全文浏览量: 472
- PDF下载量: 64
- 被引次数: 0
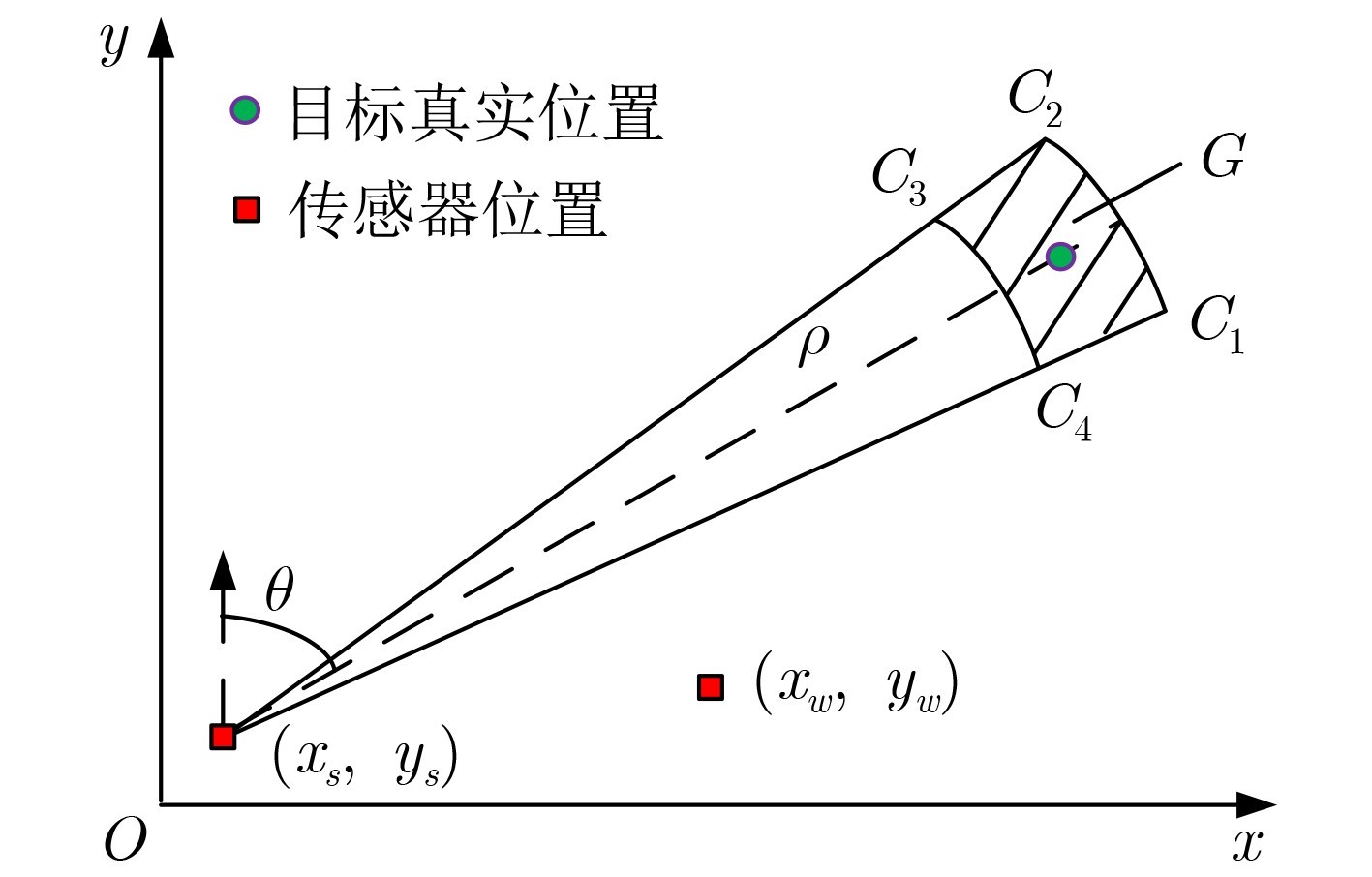
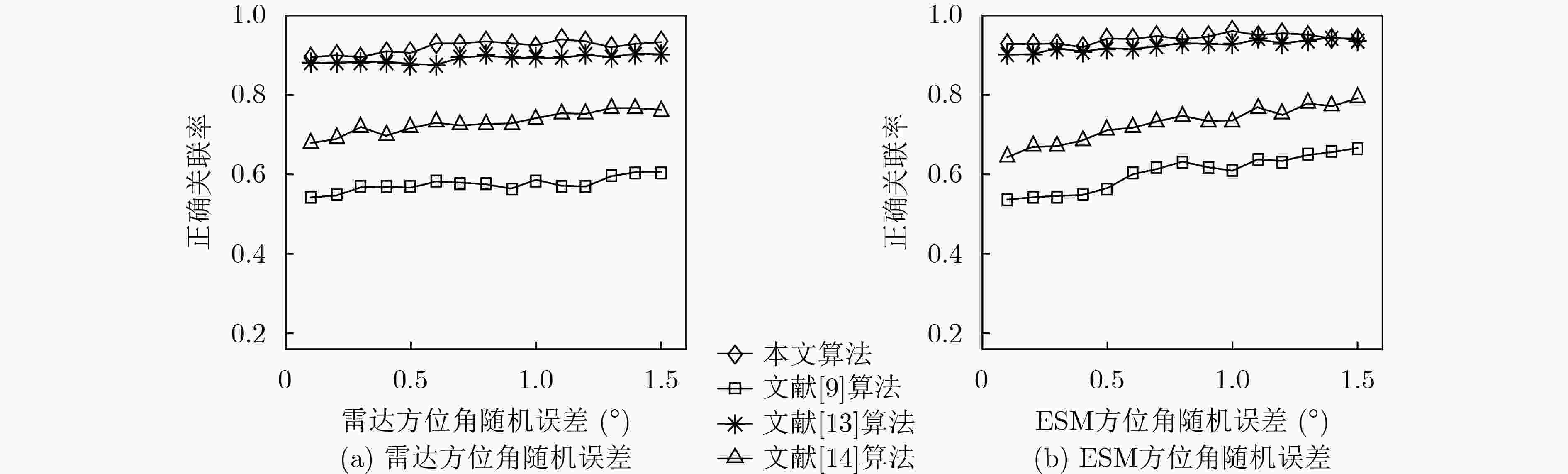
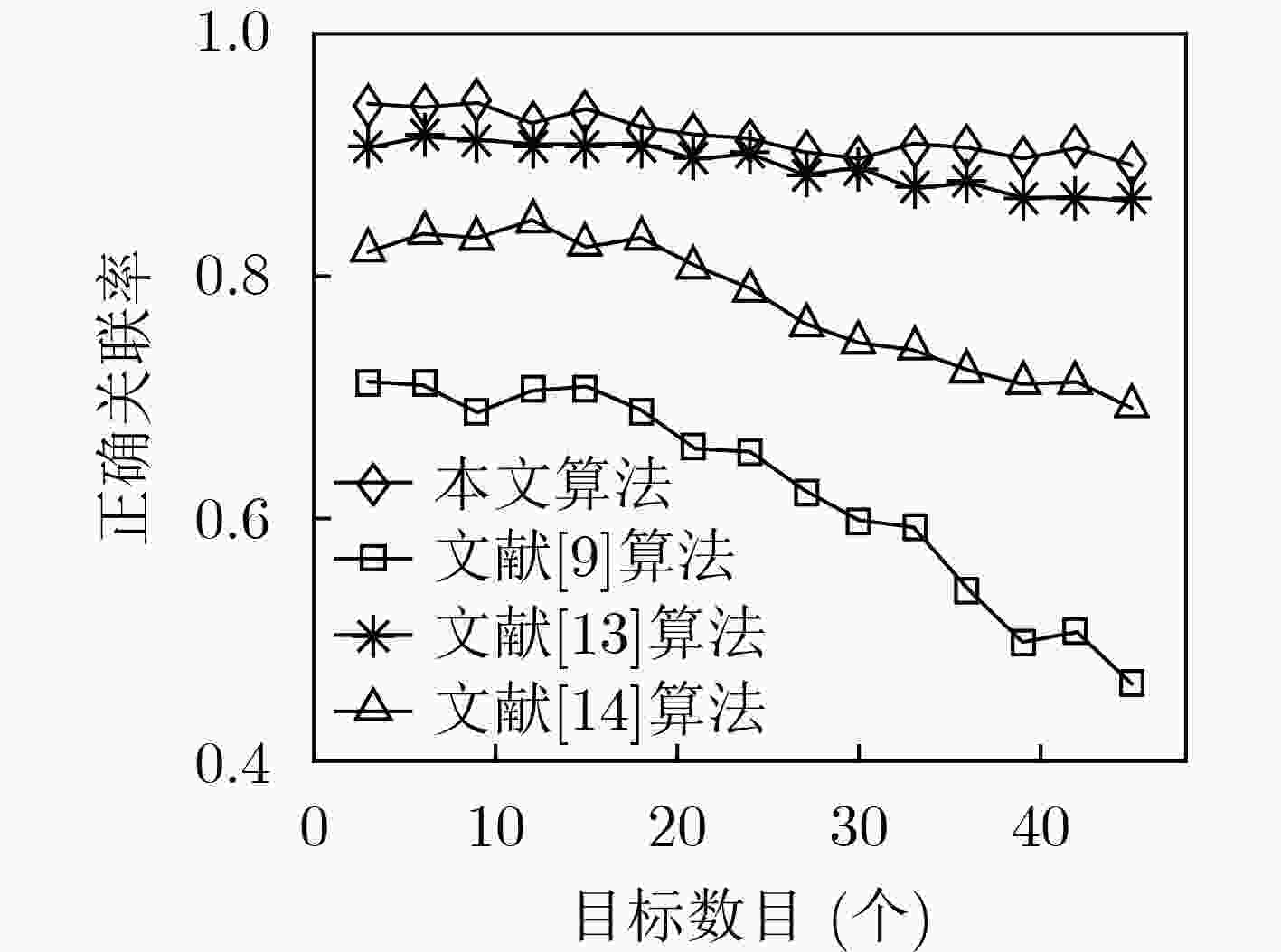
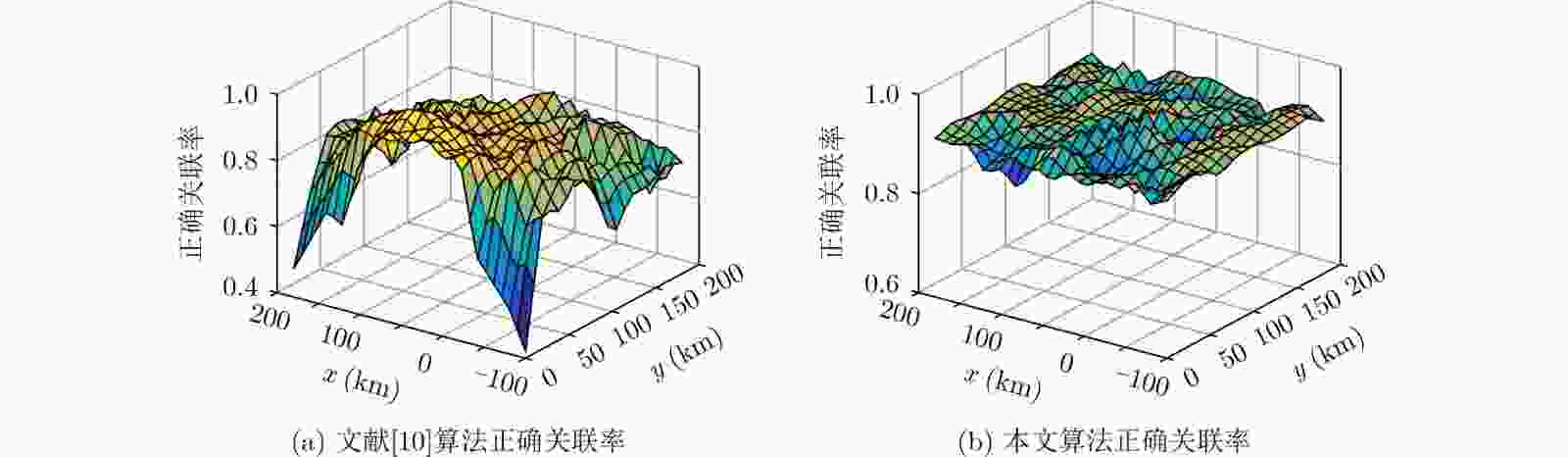
下载:
