Decision Threshold-aided Fast Z-Forward in Wireless Multirelay Communications
-
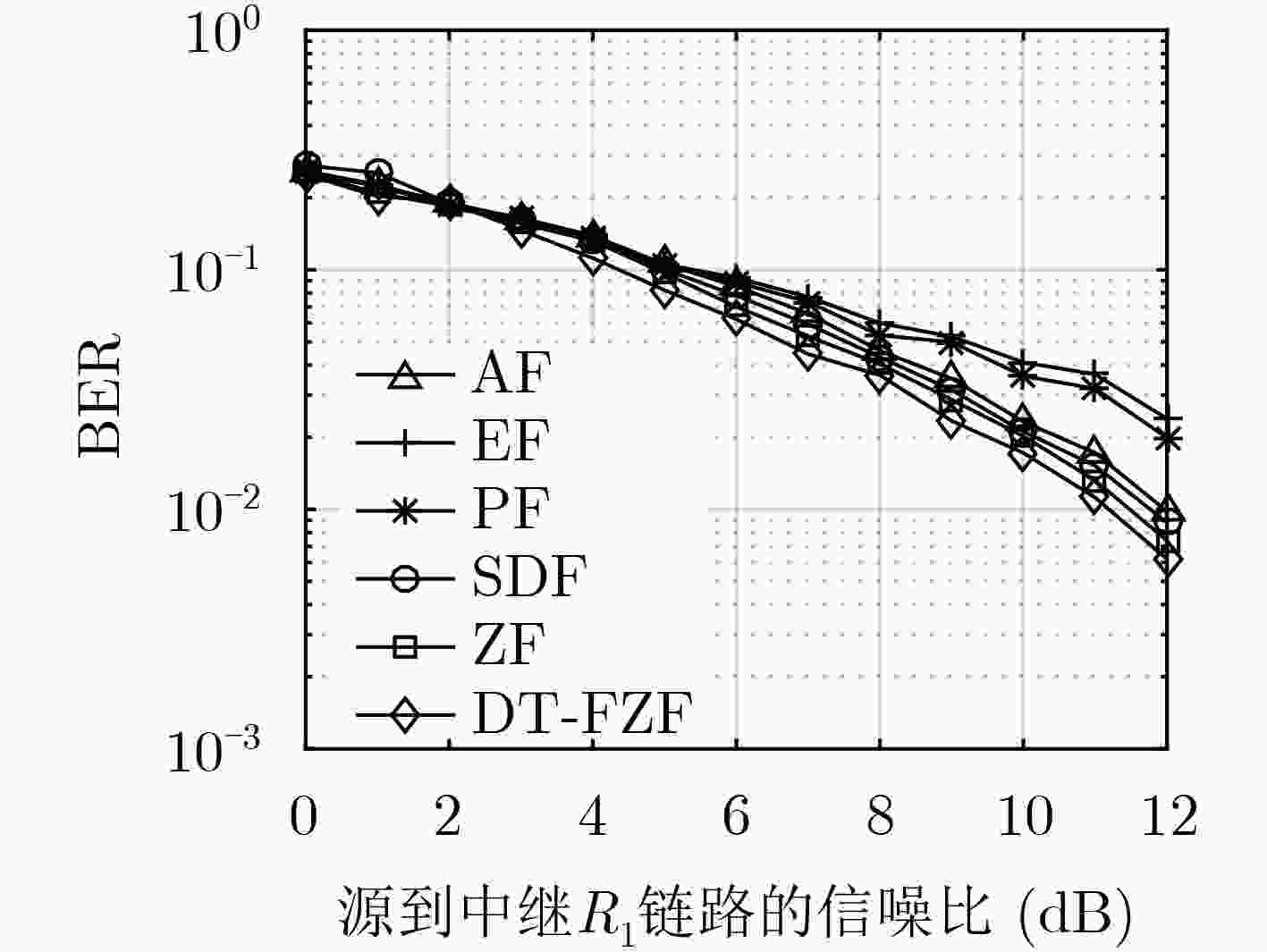
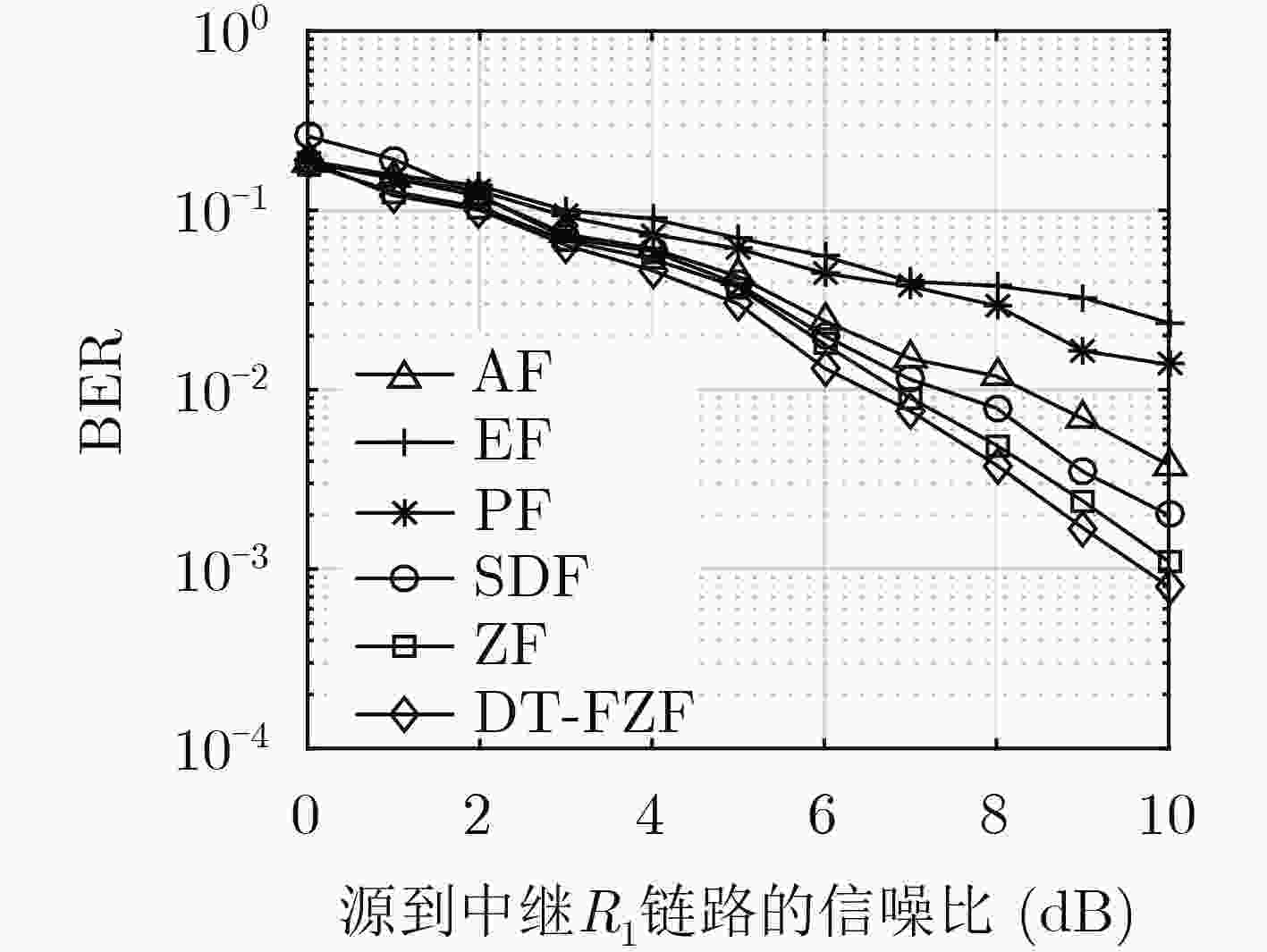
摘要: 针对Z向转发(ZF)协作所有中继节点均参与协作转发导致的能耗利用不合理问题, 该文提出了一种适用于多中继场景下的门限辅助判决快速Z转发(DT-FZF)协作。当中继节点处接收信号对数似然比(LLR)的绝对值小于门限时,中继节点不参与协作转发;否则中继节点协作转发经截断后的对数似然比(LLR)。放大转发(AF)、译码转发(DF)、分段转发(PF)和ZF协作可看作DT-FZF协作的特殊情况。在三中继系统,误比特率(BER)为10–3时,相比ZF协作,所提协作可获得约0.8 dB的性能增益。Abstract: In consideration of improper power allocation and insufficient relay selection in the current Z-Forward (ZF) scheme, an efficient Decision Threshold-aided Fast Z-Forward (DT-FZF) scheme is proposed to improve power and transmission efficiency. When the absolute value of the Log-Likelihood Ratio (LLR) of a source-relay reception is less than the decision threshold, the relay remains quiet. Otherwise, it directly sends the truncated LLR to the destination. In addition, the proposed DT-FZF scheme incorporates the Amplify-Forward(AF), Decode-Forward(DF), Piecewise-Forward(PF) and ZF schemes, all of which can be the special case of the proposed scheme. At a Bit Error Rate (BER) is of 10–3, the DT-FZF scheme outperforms the ZF scheme by approximately 0.8 dB in a three-relay system.
-
Key words:
- Multirelay networks /
- Cooperative communication /
- Z-Forward(ZF) /
- Decision threshold
-
表 1 采用DT-FZF协作的双中继系统θ2i最优取值,θ21=θ22
SNRSR(dB) 0 1 2 3 4 5 6 7 8 9 10 θ21 0.25 0.25 0.30 0.30 0.30 0.30 0.30 0.35 0.35 0.35 0.40 表 2 采用DT-FZF协作的双中继系统θ2i最优取值,θ21≠θ22
SNRSR(dB) 0 1 2 3 4 5 (θ21,θ22) (0.15, 0.25) (0.15, 0.25) (0.15, 0.25) (0.20, 0.30) (0.20,0.30) (0.25, 0.30) SNRSR(dB) 6 7 8 9 10 (θ21,θ22) (0.25,0.30) (0.25,0.35) (0.30,0.35) (0.30,0.35) (0.30,0.40) 表 3 采用DT-FZF协作的三中继系统θ21最优取值,θ21=θ22=θ23
SNRSR(dB) 0 1 2 3 4 5 6 7 8 9 10 θ21 0.30 0.30 0.30 0.35 0.35 0.35 0.35 0.35 0.40 0.40 0.45 -
[1] SENDONARIS A, ERKIP E, and AAZHANG B. User cooperation diversity. Part I. System description[J]. IEEE Transactions on Communications, 2003, 51(11): 1927–1938. doi: 10.1109/TCOMM.2003.818096 [2] SENDONARIS A, ERKIP E, and AAZHANG B. User cooperation diversity. Part II. Implementation aspects and performance analysis[J]. IEEE Transactions on Communications, 2003, 51(11): 1939–1948. doi: 10.1109/TCOMM.2003.819238 [3] VAN DER MEULEN E. A survey of multi-way channels in information theory: 1961–1976[J]. IEEE Transactions on Information Theory, 1977, 23(1): 1–37. doi: 10.1109/TIT.1977.1055652 [4] COVER T and GAMAL A E. Capacity theorems for the relay channel[J]. IEEE Transactions on Information Theory, 1979, 25(5): 572–584. doi: 10.1109/TIT.1979.1056084 [5] LANEMAN J N, TSE D N C, and WORNELL G W. Cooperative diversity in wireless networks: Efficient protocols and outage behavior[J]. IEEE Transactions on Information Theory, 2004, 50(2): 3062–3080. doi: 10.1109/TIT.2004.838089 [6] WANG Ning, LIN Xuehong, WANG Junyi, et al. Cooperative diversity through network turbo-coding[C]. 2007 International Conference on Communications, Circuits and Systems, Kokura, Japan, 2007: 92–94. doi: 10.1109/ICCCAS.2007.6247581. [7] ALVES H and SOUZA R D. Selective decode-and-forward using fixed relays and packet accumulation[J]. IEEE Communications Letters, 2011, 15(7): 707–709. doi: 10.1109/LCOMM.2011.050311.110416 [8] ELSAID L, RANJBAR M, RAYMONDI N, et al. Full-duplex decode-and-forward relaying: Secrecy rates and optimal power allocation[C]. 2017 IEEE 85th Vehicular Technology Conference, Sydney, Australia, 2017: 1–6. doi: 10.1109/VTCSpring.2017.8108359. [9] LIU Yingting, PAN Zhengwei, SHEN Jianmei, et al. Outage performance analysis for a DF based hybrid scheme over log-normal fading channels[C]. 2019 IEEE/CIC International Conference on Communications Workshops in China, Changchun, China, 2019: 114–119. doi: 10.1109/ICCChinaW.2019.8849966. [10] LIU Tianxi, SONG Lingyang, LI Yonghui, et al. Performance analysis of hybrid relay selection in cooperative wireless systems[J]. IEEE Transactions on Communications, 2012, 60(3): 779–788. doi: 10.1109/TCOMM.2012.011312.110015 [11] RIBEIRO A, CAI Xiaodong, and GIANNAKIS G B. Symbol error probabilities for general Cooperative links[J]. IEEE Transactions on Wireless Communications, 2005, 4(3): 1264–1273. doi: 10.1109/TWC.2005.846989 [12] 徐少毅, 张鹏. D2D协作通信网络中基于社交信息的中继选择和功率分配[J]. 电子与信息学报, 2017, 39(5): 1142–1149. doi: 10.11999/JEIT160746XU Shaoyi and ZHANG Peng. Social network information based relay selection and power allocation in D2D communication systems[J]. Journal of Electronics &Information Technology, 2017, 39(5): 1142–1149. doi: 10.11999/JEIT160746 [13] 田雨, 马林华, 唐红, 等. 基于虚拟MIMO的协作通信节点选择算法[J]. 电子与信息学报, 2014, 36(4): 797–803. doi: 10.3724/SP.J.1146.2013.01008TIAN Yu, MA Linhua, TANG Hong, et al. Cooperative communication node selection algorithm based on virtual MIMO[J]. Journal of Electronics &Information Technology, 2014, 36(4): 797–803. doi: 10.3724/SP.J.1146.2013.01008 [14] BASTAMI A H and OLFAT A. Optimal incremental relaying in cooperative diversity systems[J]. IET Communications, 2013, 7(2): 152–168. doi: 10.1049/iet-com.2012.0178 [15] HOSNI I and HAMDI N. Joint optimization of switching threshold and power allocation in one way incremental amplify and forward cooperative networks[C]. 2013 International Conference on Electrical Engineering and Software Applications, Hammamet, 2013: 1–5. doi: 10.1109/ICEESA.2013.6578420. [16] BAO Xingkai and LI Jing. Efficient message relaying for wireless user cooperation: Decode-amplify-forward (DAF) and hybrid DAF and coded-cooperation[J]. IEEE Transactions on Wireless Communications, 2007, 6(11): 3975–3984. doi: 10.1109/TWC.2007.06117 [17] GOMADAM K S and JAFAR S A. Optimal relay functionality for SNR maximization in memoryless relay networks[J]. IEEE Journal on Selected Areas in Communications, 2007, 25(2): 390–401. doi: 10.1109/JSAC.2007.070214 [18] TIAN Shuang, LI Yonghui, and VUCETIC B. Piecewise-and-forward relaying in wireless relay networks[J]. IEEE Signal Processing Letters, 2011, 18(5): 323–326. doi: 10.1109/LSP.2011.2125788 [19] LU Xuanxuan, LI Jing, and LIU Yang. A parametric approach to optimal soft signal relaying in wireless parallel-relay systems[C]. 2014 IEEE International Conference on Acoustics, Speech and Signal Processing, Florence, Italy, 2014: 2744–2748. doi: 10.1109/ICASSP.2014.6854099. -






 下载:
下载:





 下载:
下载:
