| [1] |
LI Ning, MARTÍNEZ-ORTEGA J F, DÍAZ V H, et al. A new high-efficiency multilevel frequency-modulation different chaos shift keying communication system[J]. IEEE Systems Journal, 2018, 12(4): 3334–3345. doi: 10.1109/jsyst.2017.2715661
|
| [2] |
ZHANG Bangquan, XU Weikai, WU Yunfeng, et al. Design and performance analysis of multilevel code-shifted M -ary differential chaos shift keying system[J]. IEEE Transactions on Circuits and Systems II: Express Briefs, 2019, 66(7): 1257–1261. doi: 10.1109/TCSII.2018.2880779
|
| [3] |
CHEND Guixian, WANG Lin, XU Weikai, et al. Carrier index differential chaos shift keying modulation[J]. IEEE Transactions on Circuits and Systems II: Express Briefs, 2017, 64(8): 907–911. doi: 10.1109/TCSII.2016.2613093
|
| [4] |
曹学鹏, 吕毅博, 黄婷婷, 等. 不同混沌调制方式在基于超宽带系统的体内信道下的性能表现[J]. 重庆邮电大学学报: 自然科学版, 2016, 28(1): 72–77. doi: 10.3979/j.issn.1673-825X.2016.01.011CAO Xuepeng, LÜ Yibo, HUANG Tingting, et al. Performance of different DCSK schemes over the UWB in-body channel[J]. Journal of Chongqing University of Posts and Telecommunications:Natural Science Edition, 2016, 28(1): 72–77. doi: 10.3979/j.issn.1673-825X.2016.01.011
|
| [5] |
张刚, 赵畅畅, 张天骐. 短参考正交多用户差分混沌键控方案的性能分析[J]. 电子与信息学报, 2019, 41(9): 2055–2062. doi: 10.11999/JEIT181038ZHANG Gang, ZHAO Changchang, and ZHANG Tianqi. Performance analysis of short reference orthogonal multiuser differential chaotic shift keying scheme[J]. Journal of Electronics &Information Technology, 2019, 41(9): 2055–2062. doi: 10.11999/JEIT181038
|
| [6] |
贺利芳, 陈俊, 张天骐. 短参考多用户差分混沌移位键控通信系统性能分析[J]. 电子与信息学报, 2020, 42(8): 1902–1909. doi: 10.11999/JEIT190117HE Lifang, CHEN Jun, and ZHANG Tianqi. Performance analysis of short reference multi-user differential chaos shift keying communication system[J]. Journal of Electronics &Information Technology, 2020, 42(8): 1902–1909. doi: 10.11999/JEIT190117
|
| [7] |
MIN X, XU W, WANG L, et al. Promising performance of a frequency-modulated differential chaos shift keying ultra-wideband system under indoor environments[J]. IET Communications, 2010, 4(2): 125–134. doi: 10.1049/iet-com.2008.0658
|
| [8] |
KOLUMBAN G. UWB technology: Chaotic communications versus noncoherent impulse radio[C]. The 2005 European Conference on Circuit Theory and Design, 2005, Cork, Ireland, 2005: II/79-II/82. doi: 10.1109/ECCTD.2005.1522997.
|
| [9] |
HERCEG M, KADDOUM G, VRANJEŠ D, et al. Permutation index DCSK modulation technique for secure multiuser high-data-rate communication systems[J]. IEEE Transactions on Vehicular Technology, 2018, 67(4): 2997–3011. doi: 10.1109/TVT.2017.2774108
|
| [10] |
KADDOUM G, SOUJERI E, and NIJSURE Y. Design of a short reference noncoherent chaos-based communication systems[J]. IEEE Transactions on Communications, 2016, 64(2): 680–689. doi: 10.1109/TCOMM.2015.2514089
|
| [11] |
KOLUMBAN G, VIZVARI B, SCHWARZ W, et al. Differential chaos shift keying: A robust coding for chaos communication[C]. The 4th International Workshop on Nonlinear Dynamics of Electronic Systems, Seville, Spain, 1996: 87–92.
|
| [12] |
YANG Hua, TANG W K S, CHEN Guanrong, et al. System design and performance analysis of orthogonal multi-level differential chaos shift keying modulation scheme[J]. IEEE Transactions on Circuits and Systems I: Regular Papers, 2016, 63(1): 146–156. doi: 10.1109/TCSI.2015.2510622
|
| [13] |
KADDOUM G. Design and performance analysis of a multiuser OFDM based differential chaos shift keying communication system[J]. IEEE Transactions on Communications, 2016, 64(1): 249–260. doi: 10.1109/TCOMM.2015.2502259
|
| [14] |
KADDOUM G and GAGNON F. Performance analysis of STBC-CSK communication system over slow fading channel[J]. Signal Processing, 2013, 93(7): 2055–2060. doi: 10.1016/j.sigpro.2012.12.020
|
| [15] |
QUYEN N X, DUONG T Q, and NALLANATHAN A. Modelling, analysis and performance comparison of two direct sampling DCSK receivers under frequency non-selective fading channels[J]. IET Communications, 2016, 10(11): 1263–1272. doi: 10.1049/iet-com.2015.1103
|
| [16] |
张刚, 徐联冰, 张天骐. 无信号内干扰的MAMU-CDSK混沌通信系统[J]. 系统工程与电子技术, 2019, 41(4): 906–913. doi: 10.3969/j.issn.1001-506X.2019.04.29ZHANG Gang, XU Lianbing, and ZHANG Tianqi. MAMU-CDSK chaotic communication system with no intra-signal interference[J]. Systems Engineering and Electronics, 2019, 41(4): 906–913. doi: 10.3969/j.issn.1001-506X.2019.04.29
|
| [17] |
KADDOUM G, RICHARDSON F D, and GAGNON F. Design and analysis of a multi-carrier differential chaos shift keying communication system[J]. IEEE Transactions on Communications, 2013, 61(8): 3281–3291. doi: 10.1109/TCOMM.2013.071013.130225
|
| [18] |
YANG Hua, JIANG Guoping, TANG W K S, et al. Multi-carrier differential chaos shift keying system with subcarriers allocation for noise reduction[J]. IEEE Transactions on Circuits and Systems II: Express Briefs, 2018, 65(11): 1733–1737. doi: 10.1109/TCSII.2017.2752754
|
| [19] |
QUYEN N X and PHAM C K. Quadrature multi-carrier DCSK: A high-efficiency scheme for radio communications[C]. 2017 International Conference on Advanced Technologies for Communications, Quy Nhon, Vietnam, 2017: 186–191. doi: 10.1109/ATC.2017.8167614.
|
| [20] |
YANG Hua, TANG W K S, CHEN Guanrong, et al. Multi-carrier chaos shift keying: System design and performance analysis[J]. IEEE Transactions on Circuits and Systems I: Regular Papers, 2017, 64(8): 2182–2194. doi: 10.1109/TCSI.2017.2685344
|
| [21] |
VENKATESH S and SINGH P. Performance analysis of OCV based non coherent MA chaotic communication system with adaptive multi user receivers[C]. 2011 International Conference on Devices and Communications (ICDeCom), Mesra, India, 2011: 1–5. doi: 10.1109/ICDECOM.2011.5738462.
|





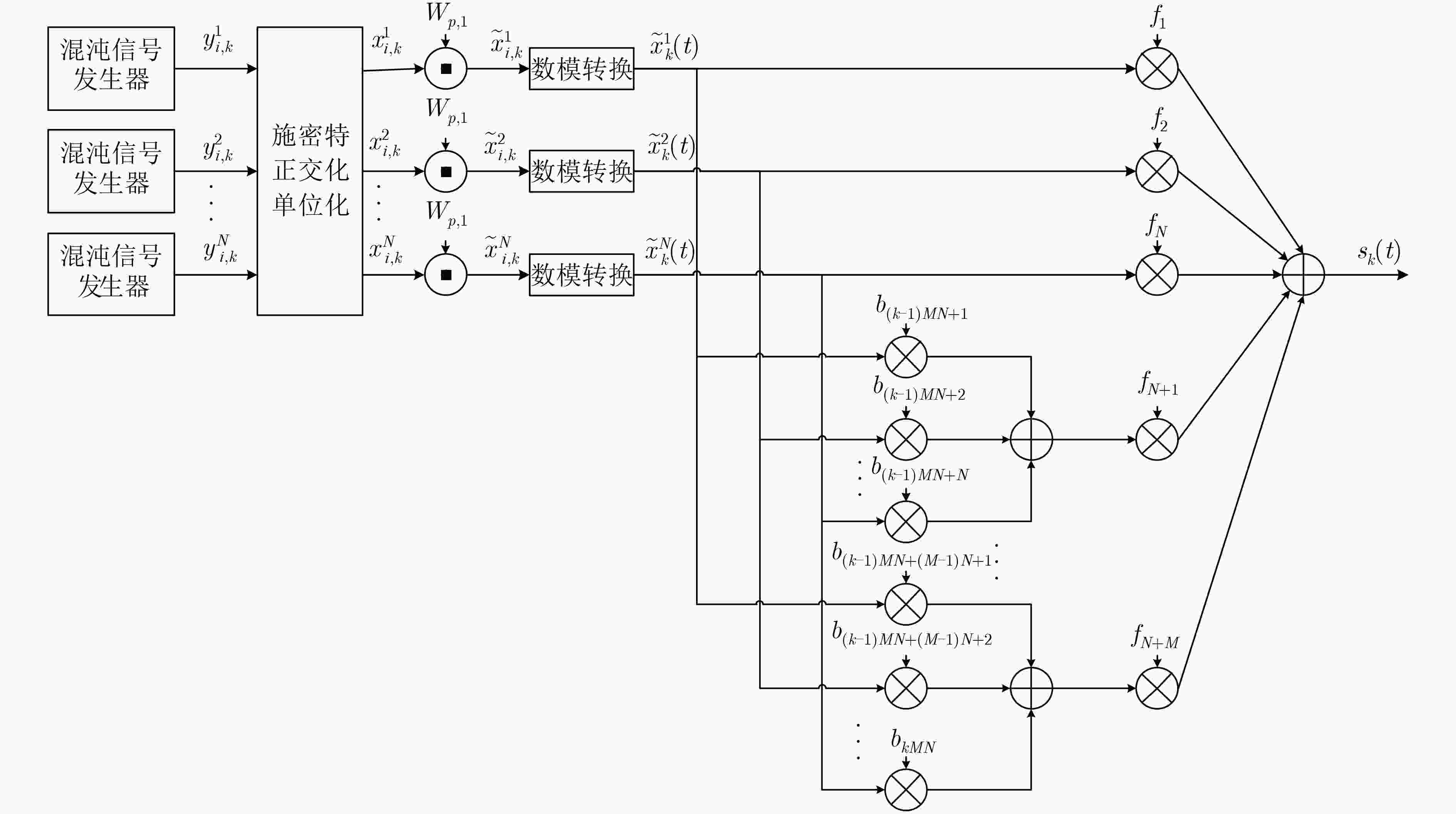
 下载:
下载:
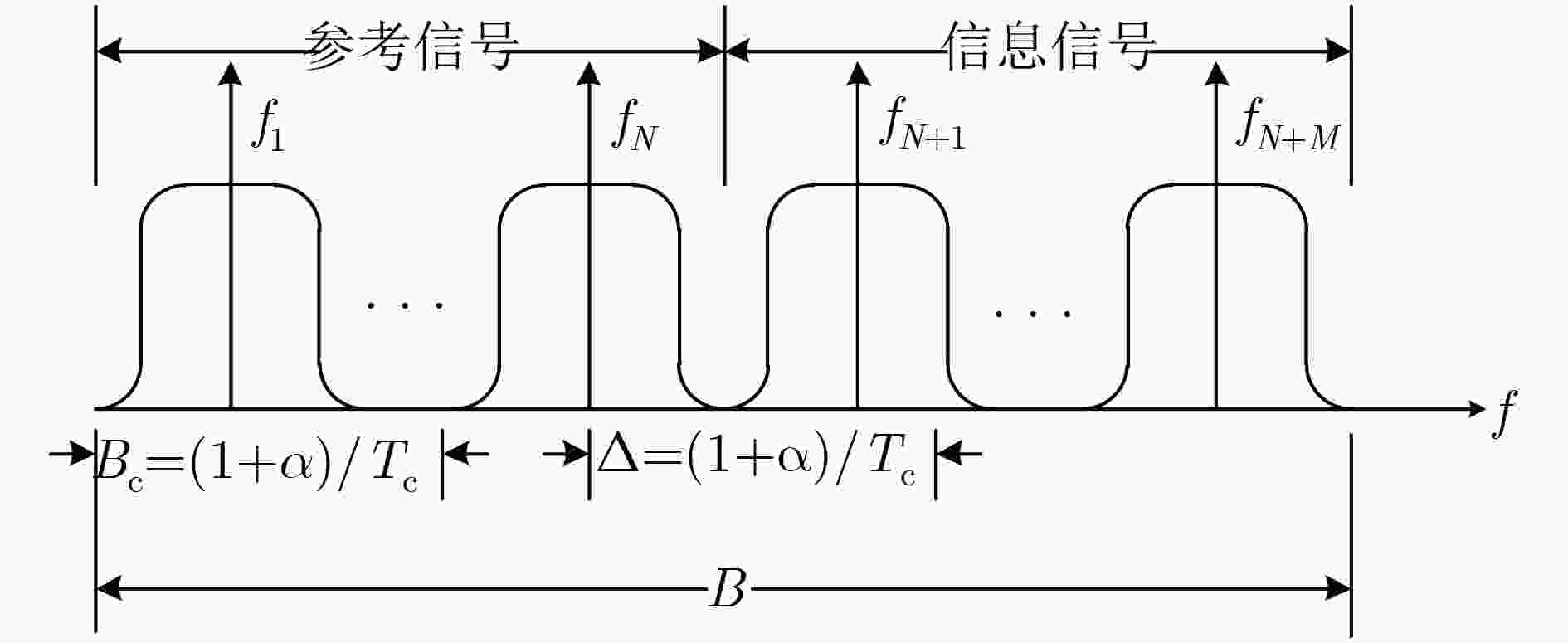

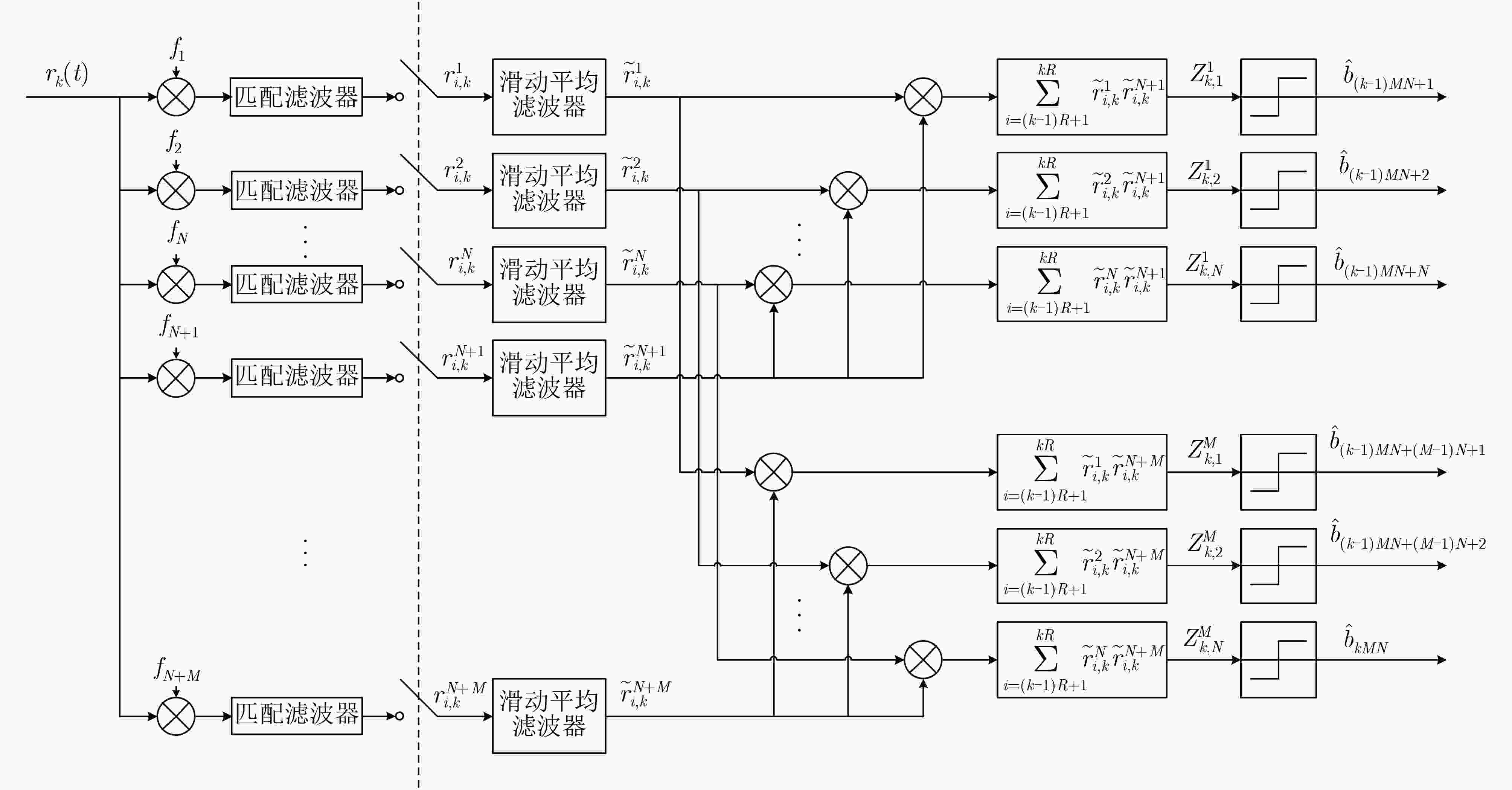
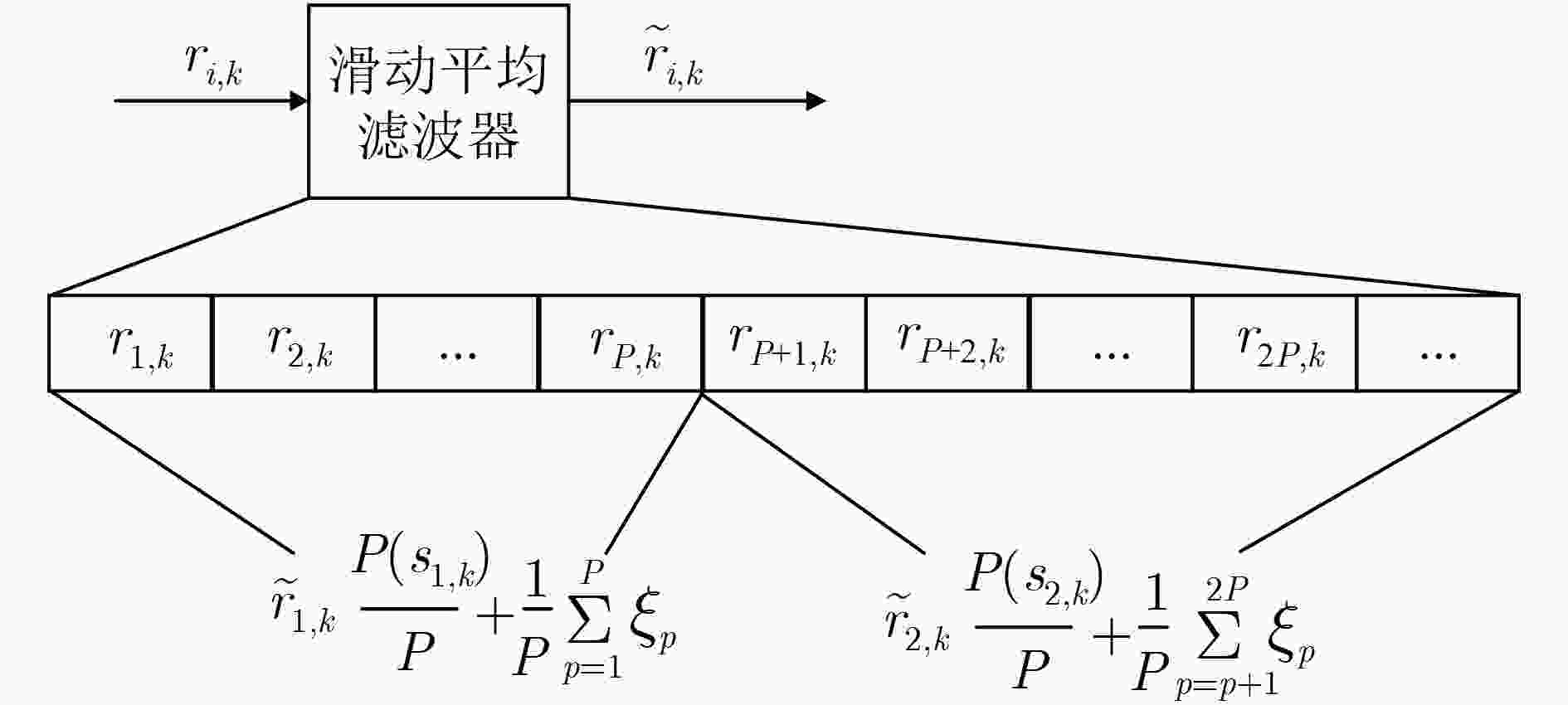







 下载:
下载:
