Research on Image Optimization Technology Based on Compressed Sensing
-
摘要:
压缩感知(CS)理论是目前信息工程相关领域研究的前沿热点之一。它打破了传统的奈奎斯特采样定理,相比于其要求的最小采样频率,CS理论证明了能够从更低数目的采样中以高概率完整地恢复原始信号,在保证信息特征不丢失的前提下节省了数据采集和处理的时间成本。压缩感知理论本质上可以视为处理线性信号恢复问题的工具,因此在求解信号和图像的逆问题上有着显而易见的优势。图像退化问题便是其中之一,恢复相应的高质量图像的过程即为图像优化。为推动压缩感知理论的学术研究与实际应用,该文介绍了其基本原理与方法。根据图像优化技术的现存研究工作,分别从去噪、去模糊和超分辨三大主流方面研究了基于CS理论的优化技术。最后探讨了所面临的问题和挑战,分析了未来的发展趋势,为将来研究工作的展开提供借鉴与帮助。
Abstract:Compressed Sensing (CS) theory is one of the most active research fields in electronic information engineering. CS theory overcomes the limits dictated by Nyquist sampling theorem. Compared to the required minimum sampling quantity, CS proves that the original signal can be restored with high probability by fewer measurements, which saves the time cost of data acquisition and processing without losing information features. CS theory can essentially be regarded as a tool for dealing with linear signal recovery problems, so it has obvious advantages in solving inverse problems of signals and images. Image degradation is one of them, and the process of restoring high-quality images is image optimization. In order to promote the academic research and practical application of CS theory, the basic principle of CS is introduced. Based on the previous research, this paper studies on CS-based image optimization technology in three main aspects: denoising, deblurring and super resolution. Finally, the problems and challenges are discussed, and the current trends are analyzed to provide reference and help for future work.
-
Key words:
- Image processing /
- Compressed Sensing (CS) /
- Image denoising /
- Image deblurring /
- Super resolution
-
表 1 基于小波方法和基于曲波方法对比
评价指标 PSNR(dB) MSE MAE 小波方法 22.36 289.16 13.52 曲波方法 22.97 254.52 12.97 表 2 主流稀疏去噪方法PSNR对比(dB)
去噪算法 BM3D LSSC NCSR SSC-GSM Monarch图像 32.46 32.15 32.34 32.52 Barbara图像 33.27 32.96 33.02 33.32 Straw图像 29.13 28.95 29.13 29.16 表 3 多种超分辨方法的信息熵与平均梯度对比
超分辨算法 原始图像 Bicubic插值 单帧CS 多帧CS 信息熵 6.162 6.473 6.487 6.532 平均梯度 4.355 3.951 4.986 5.282 -
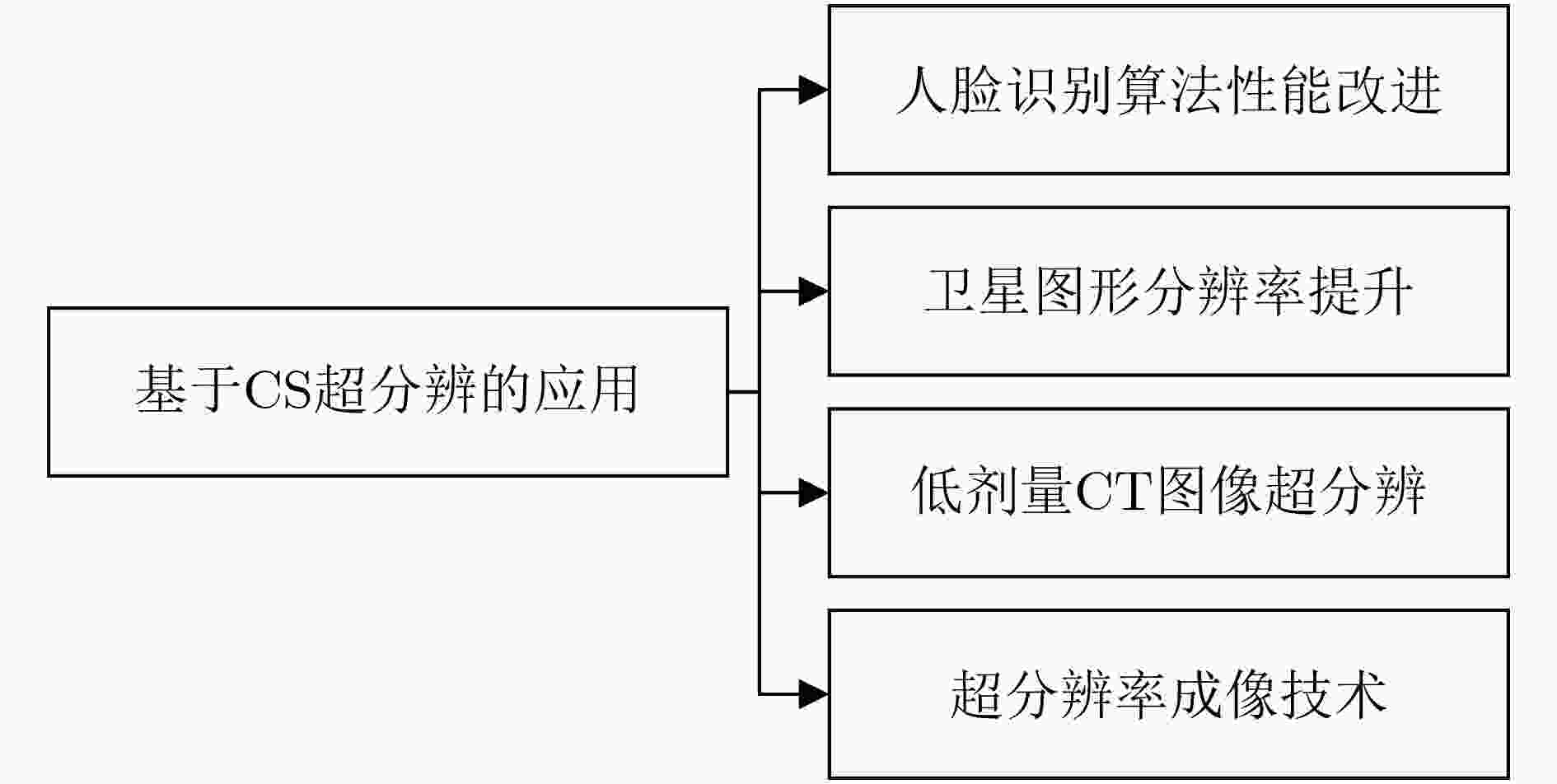
BANHAM M R and KATSAGGELOS A K. Digital image restoration[J]. IEEE Signal Processing Magazine, 1997, 14(2): 24–41. doi: 10.1109/79.581363 JIANG Jielin, ZHANG Lei, and YANG Jian. Mixed noise removal by weighted encoding with sparse nonlocal regularization[J]. IEEE Transactions on Image Processing, 2014, 23(6): 2651–2662. doi: 10.1109/TIP.2014.2317985 RODRÍGUEZ P, ROJAS R, and WOHLBERG B. Mixed Gaussian-impulse noise image restoration via total variation[C]. 2012 IEEE International Conference on Acoustics, Speech and Signal Processing, Kyoto, Japan, 2012: 1077–1080. doi: 10.1109/ICASSP.2012.6288073. ZHANG Jian, XIONG Ruiqin, ZHAO Chen, et al. Exploiting image local and nonlocal consistency for mixed Gaussian-impulse noise removal[C]. 2012 IEEE International Conference on Multimedia and Expo, Melbourne, Australia, 2012: 592–597. doi: 10.1109/ICME.2012.109. CAI Jianfeng, CHAN R H, and NIKOLOVA M. Two-phase approach for deblurring images corrupted by impulse plus Gaussian noise[J]. Inverse Problems and Imaging, 2008, 2(2): 187–204. doi: 10.3934/ipi.2008.2.187 FERGUS R, SINGH B, HERTZMANN A, et al. Removing camera shake from a single photograph[J]. ACM Transactions on Graphics, 2006, 25(3): 787–794. doi: 10.1145/1141911.1141956 SHAN Qi, JIA Jiaya, and AGARWALA A. High-quality motion deblurring from a single image[J]. ACM Transactions on Graphics, 2008, 27(3): 73. doi: 10.1145/1360612.1360672 ZHENG Shicheng, XU Li, and JIA Jiaya. Forward motion deblurring[C]. 2013 IEEE International Conference on Computer Vision, Sydney, Australia, 2013: 1465–1472. doi: 10.1109/ICCV.2013.185. GLASNER D, BAGON S, and IRANI M. Super-resolution from a single image[C]. The 2009 IEEE 12th International Conference on Computer Vision, Kyoto, Japan, 2009: 349–356. doi: 10.1109/ICCV.2009.5459271. PARK S C, PARK M K, and KANG M G. Super-resolution image reconstruction: A technical overview[J]. IEEE Signal Processing Magazine, 2003, 20(3): 21–36. doi: 10.1109/MSP.2003.1203207 YANG Jianchao, WRIGHT J, HUANG T S, et al. Image super-resolution via sparse representation[J]. IEEE Transactions on Image Processing, 2010, 19(11): 2861–2873. doi: 10.1109/TIP.2010.2050625 CHEN Yongyong, GUO Yanwen, WANG Yongli, et al. Denoising of hyperspectral images using nonconvex low rank matrix approximation[J]. IEEE Transactions on Geoscience and Remote Sensing, 2017, 55(9): 5366–5380. doi: 10.1109/TGRS.2017.2706326 MANJÓN J V, CARBONELL-CABALLERO J, LULL J J, et al. MRI denoising using non-local means[J]. Medical Image Analysis, 2008, 12(4): 514–523. doi: 10.1016/j.media.2008.02.004 PENG Yigang, GANESH A, WRIGHT J, et al. RASL: Robust alignment by sparse and low-rank decomposition for linearly correlated images[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 2012, 34(11): 2233–2246. doi: 10.1109/TPAMI.2011.282 WANG Naiyan and YEUNG D Y. Bayesian robust matrix factorization for image and video processing[C]. 2013 IEEE International Conference on Computer Vision, Sydney, Australia, 2013: 1785–1792. doi: 10.1109/ICCV.2013.224. DONOHO D L. Compressed sensing[J]. IEEE Transactions on Information Theory, 2006, 52(4): 1289–1306. doi: 10.1109/TIT.2006.871582 CANDES E J, ROMBERG J, and TAO T. Robust uncertainty principles: Exact signal reconstruction from highly incomplete frequency information[J]. IEEE Transactions on Information Theory, 2006, 52(2): 489–509. doi: 10.1109/TIT.2005.862083 CANDES E J and TAO T. Near-optimal signal recovery from random projections: Universal encoding strategies?[J]. IEEE Transactions on Information Theory, 2006, 52(12): 5406–5425. doi: 10.1109/TIT.2006.885507 MALLAT S G. A Wavelet Tour of Signal Processing[M]. San Diego, USA: Academic Press, 1999: 263-376. CANDÈS E J and DONOHO D L. Ridgelets: A key to higher-dimensional intermittency?[J]. Philosophical Transactions of the Royal Society B, 1999, 357(1760): 2495–2509. doi: 10.1098/rsta.1999.0444 CANDES E J and DONOHO D L. Curvelets, multiresolution representation, and scaling laws[J]. SPIE, 2000, 4119: 1–12. doi: 10.1117/12.408568. DONOHO D L. Wedgelets: Nearly minimax estimation of edges[J]. The Annals of Statistics, 1999, 27(3): 859–897. doi: 10.1214/aos/1018031261 DO M N and VETTERLI M. The contourlet transform: An efficient directional multiresolution image representation[J]. IEEE Transactions on Image Processing, 2005, 14(12): 2091–2106. doi: 10.1109/TIP.2005.859376 PENNEC L E and MALLAT S. Bandelet representations for image compression[C]. The 2001 International Conference on Image Processing, Thessaloniki, Greece, 2001: 12. doi: 10.1109/ICIP.2001.958939. ELAD M and AHARON M. Image denoising via sparse and redundant representations over learned dictionaries[J]. IEEE Transactions on Image Processing, 2006, 15(12): 3736–3745. doi: 10.1109/TIP.2006.881969 AHARON M, ELAD M, and BRUCKSTEIN A. K-SVD: An algorithm for designing overcomplete dictionaries for sparse representation[J]. IEEE Transactions on Signal Processing, 2006, 54(11): 4311–4322. doi: 10.1109/TSP.2006.881199 CANDES E J and TAO T. Decoding by linear programming[J]. IEEE Transactions on Information Theory, 2005, 51(12): 4203–4215. doi: 10.1109/TIT.2005.858979 CANDES E J and WAKIN M B. An introduction to compressive sampling[J]. IEEE Signal Processing Magazine, 2008, 25(2): 21–30. doi: 10.1109/MSP.2007.914731 CANDÈS E J. The restricted isometry property and its implications for compressed sensing[J]. Comptes Rendus Mathematique, 2008, 346(9/10): 589–592. doi: 10.1016/j.crma.2008.03.014 LIU Xinji, XIA Shutao, and DAI Tao. Deterministic constructions of binary measurement matrices with various sizes[C]. 2015 IEEE International Conference on Acoustics, Speech and Signal Processing, Brisbane, Australia, 2015: 3641–3645. doi: 10.1109/ICASSP.2015.7178650. BRUCKSTEIN A M, DONOHO D L, and ELAD M. From sparse solutions of systems of equations to sparse modeling of signals and images[J]. SIAM Review, 2009, 51(1): 34–81. doi: 10.1137/060657704 TROPP J A and GILBERT A C. Signal recovery from random measurements via orthogonal matching pursuit[J]. IEEE Transactions on Information Theory, 2007, 53(12): 4655–4666. doi: 10.1109/tit.2007.909108 RUDIN L I, OSHER S, and FATEMI E. Nonlinear total variation based noise removal algorithms[J]. Physica D: Nonlinear Phenomena, 1992, 60(1/4): 259–268. doi: 10.1016/0167-2789(92)90242-F FRIEDMAN N and RUSSELL S. Image segmentation in video sequences: A probabilistic approach[C]. The 13th Conference on Uncertainty in Artificial Intelligence, Providence, USA, 1997: 175–181. VETTERLI M and KOVACEVIC J. Wavelets and Subband Coding[M]. Englewood Cliffs, USA: Prentice Hall, 1995: 414–445. DONOHO D L. De-noising by soft-thresholding[J]. IEEE Transactions on Information Theory, 1995, 41(3): 613–627. doi: 10.1109/18.382009 DONOHO D L and JOHNSTONE J M. Ideal spatial adaptation by wavelet shrinkage[J]. Biometrika, 1994, 81(3): 425–455. doi: 10.1093/biomet/81.3.425 PORTILLA J, STRELA V, WAINWRIGHT M J, et al. Image denoising using scale mixtures of Gaussians in the wavelet domain[J]. IEEE Transactions on Image Processing, 2003, 12(11): 1338–1351. doi: 10.1109/TIP.2003.818640 DABOV K, FOI A, KATKOVNIK V, et al. Image denoising by sparse 3-D transform-domain collaborative filtering[J]. IEEE Transactions on Image Processing, 2007, 16(8): 2080–2095. doi: 10.1109/TIP.2007.901238 SANTOS C A N, MARTINS D L N, and MASCARENHAS N D A. Ultrasound image despeckling using stochastic distance-based BM3D[J]. IEEE Transactions on Image Processing, 2017, 26(6): 2632–2643. doi: 10.1109/TIP.2017.2685339 LI Yingjiang, ZHANG Jiangwei, and WANG Maoning. Improved BM3D denoising method[J]. IET Image Processing, 2017, 11(12): 1197–1204. doi: 10.1049/iet-ipr.2016.1110 SICA F, COZZOLINO D, ZHU Xiaoxiang, et al. InSAR-BM3D: A nonlocal filter for SAR interferometric phase restoration[J]. IEEE Transactions on Geoscience and Remote Sensing, 2018, 56(6): 3456–3467. doi: 10.1109/TGRS.2018.2800087 QIAO Tong, REN Jinchang, WANG Zheng, et al. Effective denoising and classification of hyperspectral images using curvelet transform and singular spectrum analysis[J]. IEEE Transactions on Geoscience and Remote Sensing, 2017, 55(1): 119–133. doi: 10.1109/TGRS.2016.2598065 MAIRAL J, ELAD M, and SAPIRO G. Sparse representation for color image restoration[J]. IEEE Transactions on Image Processing, 2008, 17(1): 53–69. doi: 10.1109/TIP.2007.911828 CHATTERJEE P and MILANFAR P. Clustering-based denoising with locally learned dictionaries[J]. IEEE Transactions on Image Processing, 2009, 18(7): 1438–1451. doi: 10.1109/TIP.2009.2018575 MAIRAL J, BACH F, PONCE J, et al. Non-local sparse models for image restoration[C]. The 12th IEEE International Conference on Computer Vision, Kyoto, Japan, 2009: 2272–2279. doi: 10.1109/ICCV.2009.5459452. KATKOVNIK V, FOI A, EGIAZARIAN K, et al. From local kernel to nonlocal multiple-model image denoising[J]. International Journal of Computer Vision, 2010, 86(1): 1. doi: 10.1007/s11263-009-0272-7 DONG Weisheng, LI Xin, ZHANG Lei, et al. Sparsity-based Image denoising via dictionary learning and structural clustering[C]. CVPR 2011, Providence, USA, 2011: 457–464. doi: 10.1109/CVPR.2011.5995478. DONG Weisheng, ZHANG Lei, SHI Guangming, et al. Nonlocally centralized sparse representation for image restoration[J]. IEEE Transactions on Image Processing, 2013, 22(4): 1620–1630. doi: 10.1109/TIP.2012.2235847 DONG Weisheng, SHI Guangming, MA Yi, et al. Image restoration via simultaneous sparse coding: Where structured sparsity meets Gaussian scale mixture[J]. International Journal of Computer Vision, 2015, 114(2/3): 217–232. doi: 10.1007/s11263-015-0808-y ZHA Zhiyuan, ZHANG Xinggan, WANG Qiong, et al. Group sparsity residual with non-local samples for image denoising[C]. 2018 IEEE International Conference on Acoustics, Speech and Signal Processing, Calgary, Canada, 2018: 1353–1357. doi: 10.1109/ICASSP.2018.8461388. NEEDELL D and TROPP J A. CoSaMP: Iterative signal recovery from incomplete and inaccurate samples[J]. Applied and Computational Harmonic Analysis, 2009, 26(3): 301–321. doi: 10.1016/j.acha.2008.07.002 WANG Jian, KWON S, and SHIM B. Generalized orthogonal matching pursuit[J]. IEEE Transactions on Signal Processing, 2012, 60(12): 6202–6216. doi: 10.1109/tsp.2012.2218810 EMERSON T H, OLSON C C, and DOSTER T. Path-based dictionary augmentation: A framework for improving k -sparse image processing[J]. IEEE Transactions on Image Processing, 2020, 29: 1259–1270. doi: 10.1109/TIP.2019.2927331 JIN Y, KU B, AHN J, et al. Nonhomogeneous noise removal from side-scan sonar images using structural sparsity[J]. IEEE Geoscience and Remote Sensing Letters, 2019, 16(8): 1215–1219. doi: 10.1109/LGRS.2019.2895843 HAN Jing, YUE Jiang, ZHNAG Yi, et al. Local sparse structure denoising for low-light-level image[J]. IEEE Transactions on Image Processing, 2015, 24(12): 5177–5192. doi: 10.1109/TIP.2015.2447735 XIE Ting, LI Shutao, and SUN Bin. Hyperspectral images denoising via nonconvex regularized low-rank and sparse matrix decomposition[J]. IEEE Transactions on Image Processing, 2020, 29: 44–56. doi: 10.1109/TIP.2019.2926736 SONG Pingfan, WEIZMAN L, MOTA J F C, et al. Coupled dictionary learning for multi-contrast MRI reconstruction[C]. The 25th IEEE International Conference on Image Processing, Athens, Greece, 2018: 2880–2884. doi: 10.1109/ICIP.2018.8451341. RICHARDSON W H. Bayesian-based iterative method of image restoration[J]. Journal of the Optical Society of America, 1972, 62(1): 55–59. doi: 10.1364/JOSA.62.000055 LUCY L B. An iterative technique for the rectification of observed distributions[J]. Astronomical Journal, 1974, 79: 745–754. doi: 10.1086/111605 LOU Yifei, BERTOZZI A L, and SOATTO S. Direct sparse deblurring[J]. Journal of Mathematical Imaging and Vision, 2011, 39(1): 1–12. doi: 10.1007/s10851-010-0220-8 YUAN Lu, SUN Jian, QUAN Long, et al. Image deblurring with blurred/noisy image pairs[J]. ACM Transactions on Graphics, 2007, 26(3): 1. doi: 10.1145/1276377.1276379 唐述, 谢显中. 多正则化混合约束的模糊图像盲复原方法[J]. 电子与信息学报, 2015, 37(4): 770–776. doi: 10.11999/JEIT140949TANG Shu and XIE Xianzhong. Multi-regularization hybrid constraints method for blind image restoration[J]. Journal of Electronics &Information Technology, 2015, 37(4): 770–776. doi: 10.11999/JEIT140949 HU Zhe, HUANG Jiabin, and YANG M H. Single image deblurring with adaptive dictionary learning[C]. The 2010 IEEE International Conference on Image Processing, Hong Kong, China, 2010: 1169–1172. doi: 10.1109/ICIP.2010.5651892. YU Jing, CHANG Zhenchun, XIAO Chuangbai, et al. Blind image deblurring based on sparse representation and structural self-similarity[C]. 2017 IEEE International Conference on Acoustics, Speech and Signal Processing, New Orleans, USA, 2017: 1328–1332. doi: 10.1109/ICASSP.2017.7952372. ROSTAMI M, MICHAILOVICH O, and WANG Zhou. Image deblurring using derivative compressed sensing for optical imaging application[J]. IEEE Transactions on Image Processing, 2012, 21(7): 3139–3149. doi: 10.1109/TIP.2012.2190610 CHEN Jia, YUAN Lu, TANG C K, et al. Robust dual motion deblurring[C]. 2008 IEEE Conference on Computer Vision and Pattern Recognition, Anchorage, USA, 2008: 1–8. doi: 10.1109/CVPR.2008.4587830. ZHU Xiang, ŠROUBEK F, and MILANFAR P. Deconvolving PSFs for a better motion deblurring using multiple images[C]. The 12th European Conference on Computer Vision, Florence, Italy, 2012: 636–647. doi: 10.1007/978-3-642-33715-4_46. ZHANG Haichao, WIPF D, and ZHANG Yanning. Multi-image blind deblurring using a coupled adaptive sparse prior[C]. 2013 IEEE Conference on Computer Vision and Pattern Recognition, Portland, USA, 2013: 1051–1058. doi: 10.1109/CVPR.2013.140. XIANG Fengtao, HUANG Yumin, GU Xueqiang, et al. A restoration method of infrared image based on compressive sampling[C]. The 8th International Conference on Intelligent Human-machine Systems and Cybernetics, Hangzhou, China, 2016: 493–496. doi: 10.1109/IHMSC.2016.98. ZHANG Yan, PU Haitao, and LIAN Jian. Quick response barcode deblurring via l0-regularisation based sparse optimisation[J]. IET Image Processing, 2019, 13(8): 1254–1258. doi: 10.1049/iet-ipr.2018.5738 LEE H, JUNG C, and KIM C. Blind deblurring of text images using a text-specific hybrid dictionary[J]. IEEE Transactions on Image Processing, 2020, 29: 710–723. doi: 10.1109/TIP.2019.2933739 吴敏, 邢孟道, 张磊. 基于压缩感知的二维联合超分辨ISAR成像算法[J]. 电子与信息学报, 2014, 36(1): 187–193. doi: 10.3724/SP.J.1300.2012.20093WU Min, XING Mengdao, and ZHANG Lei. Two dimensional joint super-resolution ISAR imaging algorithm based on compressive sensing[J]. Journal of Electronics &Information Technology, 2014, 36(1): 187–193. doi: 10.3724/SP.J.1300.2012.20093 DEKA B, GORAIN K K, KALITA N, et al. Single image super-resolution using compressive sensing with learned overcomplete dictionary[C]. The 2013 4th National Conference on Computer Vision, Pattern Recognition, Image Processing and Graphics, Jodhpur, India, 2013: 1–5. doi: 10.1109/NCVPRIPG.2013.6776176. SUN Yicheng, GU Guohua, SUI Xiubao, et al. Single image super-resolution using compressive sensing with a redundant dictionary[J]. IEEE Photonics Journal, 2015, 7(2): 6900411. doi: 10.1109/JPHOT.2015.2409063 SUN Yicheng, GU Guohua, SUI Xiubao, et al. Compressive superresolution imaging based on local and nonlocal regularizations[J]. IEEE Photonics Journal, 2016, 8(1): 6900112. doi: 10.1109/JPHOT.2016.2516251 XIAO Aoran, SHAO Zhenfeng, and WANG Zhongyuan. Sparse coding for super-resolution via K-means classification[C]. 2017 IEEE International Conference on Multimedia & Expo Workshops, Hong Kong, China, 2017: 363–368. doi: 10.1109/ICMEW.2017.8026254. ZHOU Ruofei, WANG Gang, ZHAO Donglai, et al. Super-resolution of low-quality images based on compressed sensing and sequence information[C]. The 90th IEEE Vehicular Technology Conference, Honolulu, USA, 2019: 1–5. doi: 10.1109/VTCFall.2019.8891073. LIAO Haibin, DAI Wenhua, ZHOU Qianjin, et al. Non-local similarity dictionary learning based face super-resolution[C]. The 12th International Conference on Signal Processing, Hangzhou, China, 2014: 88-93. doi: 10.1109/ICOSP.2014.7014975. RANA S, SINGH H, and KUMAR A. Comparative analysis of single and multi frame super resolution in satellite imagery[C]. 2018 IEEE International Geoscience and Remote Sensing Symposium, Valencia, Spain, 2018: 7220–7223. doi: 10.1109/IGARSS.2018.8517329. GU Peijian and ZHENG Lin. Fast low-dose computed tomography image super-resolution reconstruction via sparse coding and random forests[C]. The 8th IEEE Joint International Information Technology and Artificial Intelligence Conference, Chongqing, China, 2019: 1400–1403. doi: 10.1109/ITAIC.2019.8785482. SUN Yicheng, GU Guohua, SUI Xiubao, et al. Super-resolution imaging using compressive sensing and binary pure-phase annular filter[J]. IEEE Photonics Journal, 2017, 9(3): 7802409. doi: 10.1109/JPHOT.2017.2696519 MARCUS G. Deep learning: A critical appraisal[J]. arXiv: 1801.00631, 2018. -





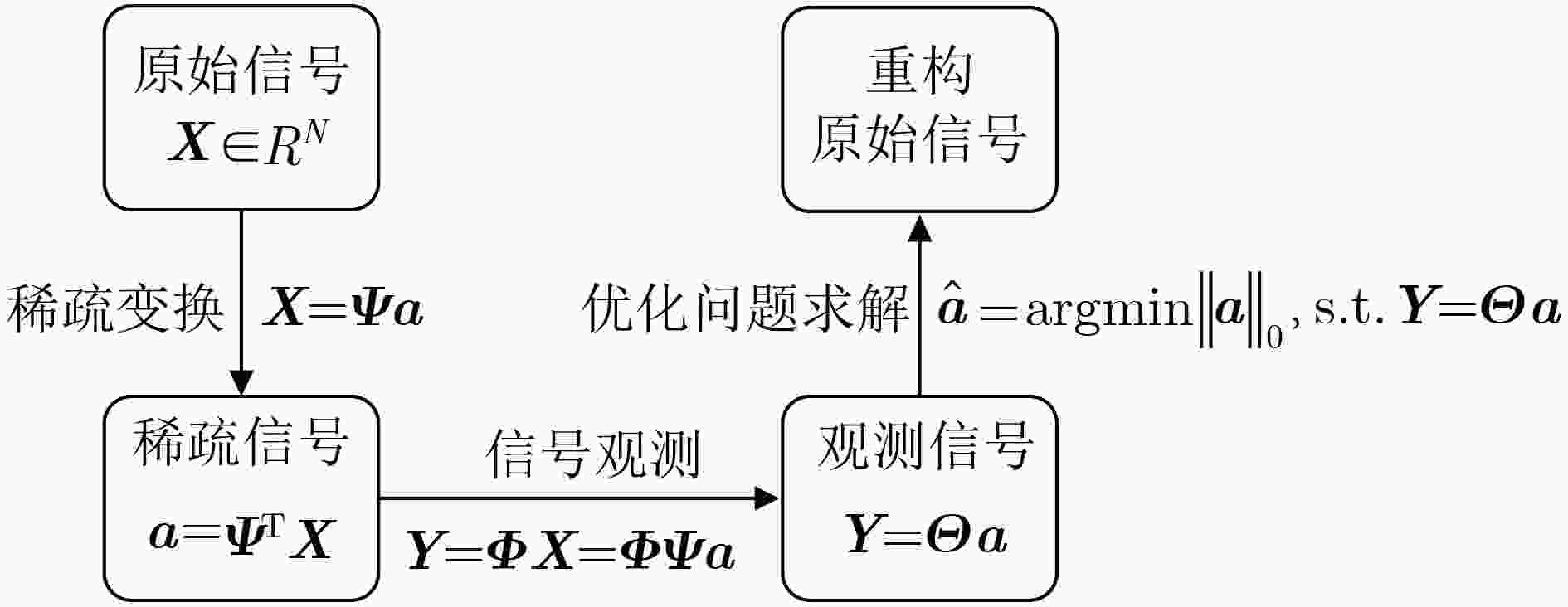
 下载:
下载:

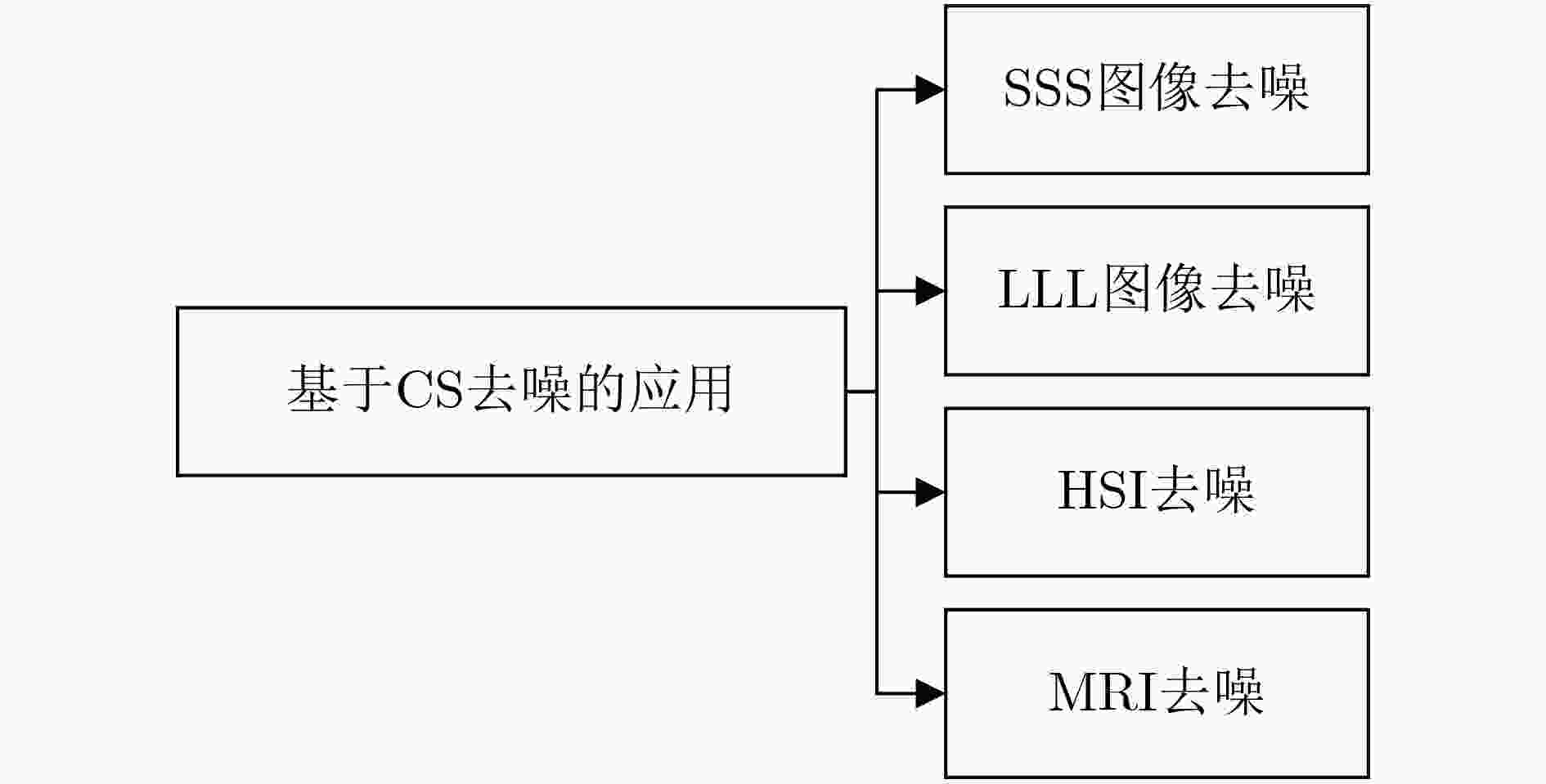
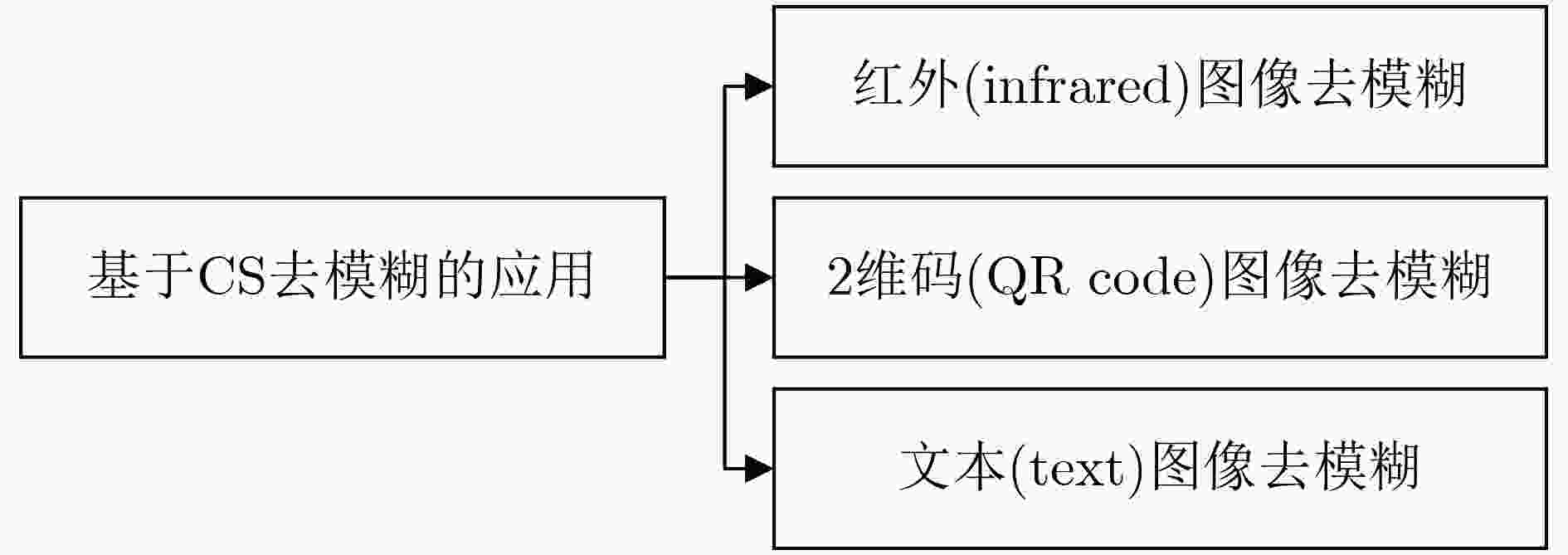
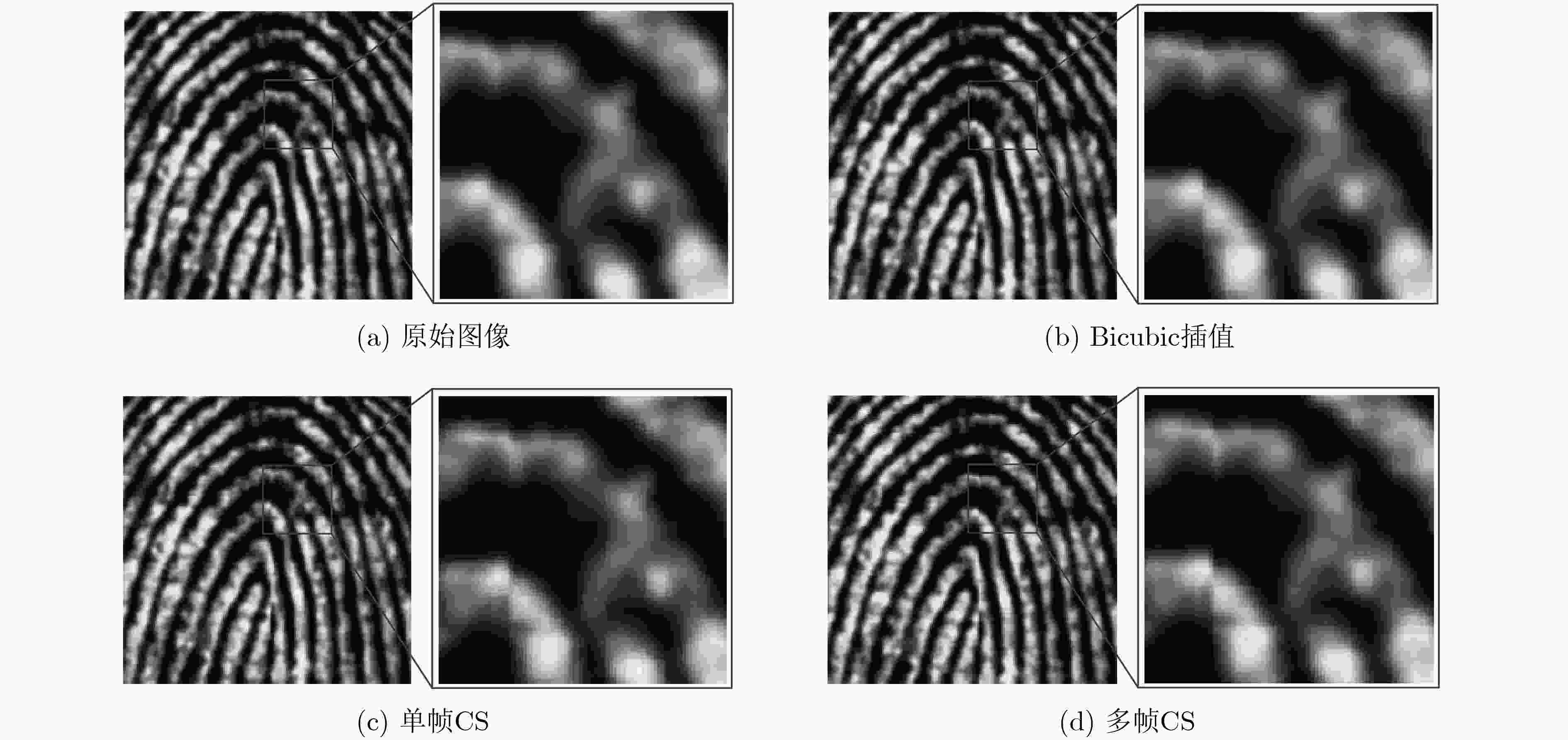


 下载:
下载:
