Wideband DOA Estimation via Cyclic Correntropy and Sparse Reconstruction in the Presence of Impulsive Noise
-
摘要: 针对脉冲噪声与同频带干扰并存时宽带信号的波达方向(DOA)估计问题，该文提出一种结合循环相关熵(CCE)与稀疏重构的算法。首先，分析了宽带信源的接收信号模型，并利用循环相关熵的性质构造出对脉冲噪声与同频带干扰具有抑制能力的宽带信号虚拟输出阵列。随后对该虚拟输出阵列进行稀疏表示，并通过归一化迭代硬阈值(NIHT)算法进行稀疏重构，从而估计宽带信号的波达方向。实验结果表明，该算法对脉冲噪声和同频带干扰具有很好的抑制作用，并且相较已有算法在估计性能方面有明显的改善。Abstract: To deal with wideband band Direction Of Arrival (DOA) estimation in the presence of impulsive noise and co-channel interferences, a novel method is proposed with the help of Cyclic CorrEntropy (CCE) and sparse reconstruction. Firstly, the received signal model of wideband sources is analyzed and a virtual array output is constructed, which shows resistance to impulsive noise and co-channel interferences via the characteristics of CCE. Then, to extract the DOA of wideband signals, the virtual array output with a sparse structure is represented and the Normalized Iterative Hard Thresholding (NIHT) is utilized to solve the sparse reconstruction problem. Comprehensive simulation results demonstrate that the proposed method has efficient suppression on impulsive noise and co-channel interference and it can improve both accuracy and efficiency than existing methods.
-
Key words:
- Wideband DOA /
- Impulsive noise /
- Cyclic CorrEntropy (CCE) /
- Sparse reconstruction
-
KRIM H and VIBERG M. Two decades of array signal processing research: The parametric approach[J]. IEEE Signal Processing Magazine, 1996, 13(4): 67–94. doi: 10.1109/79.526899 SCHMIDT R. Multiple emitter location and signal parameter estimation[J]. IEEE Transactions on Antennas and Propagation, 1986, 34(3): 276–280. doi: 10.1109/TAP.1986.1143830 ROY R and KAILATH T. ESPRIT-estimation of signal parameters via rotational invariance techniques[J]. IEEE Transactions on Acoustics, Speech, and Signal Processing, 1989, 37(7): 984–995. doi: 10.1109/29.32276 MALIOUTOV D, CETIN M, and WILLSKY A S. A sparse signal reconstruction perspective for source localization with sensor arrays[J]. IEEE Transactions on Signal Processing, 2005, 53(8): 3010–3022. doi: 10.1109/tsp.2005.850882 HU Na, YE Zhongfu, XU Xu, et al. DOA estimation for sparse array via sparse signal reconstruction[J]. IEEE Transactions on Aerospace and Electronic Systems, 2013, 49(2): 760–773. doi: 10.1109/TAES.2013.6494379 WU Xiaohuan, ZHU Weiping, YAN Jun, et al. Two sparse-based methods for off-grid direction-of-arrival estimation[J]. Signal Processing, 2018, 142: 87–95. doi: 10.1016/j.sigpro.2017.07.004 郭英, 东润泽, 张坤峰, 等. 基于稀疏贝叶斯学习的多跳频信号DOA估计方法[J]. 电子与信息学报, 2019, 41(3): 516–522. doi: 10.11999/JEIT180435GUO Ying, DONG Runze, ZHANG Kunfeng, et al. Direction of arrival estimation for multiple frequency hopping signals based on sparse bayesian learning[J]. Journal of Electronics &Information Technology, 2019, 41(3): 516–522. doi: 10.11999/JEIT180435 XU G and KAILATH T. Direction-of-arrival estimation via exploitation of cyclostationary-a combination of temporal and spatial processing[J]. IEEE Transactions on Signal Processing, 1992, 40(7): 1775–1786. doi: 10.1109/78.143448 XIN Jingmin and SANO A. MSE-based regularization approach to direction estimation of coherent narrowband signals using linear prediction[J]. IEEE Transactions on Signal Processing, 2001, 49(11): 2481–2497. doi: 10.1109/78.960396 LIU Zhangmeng, HUANG Zhitao, and ZHOU Yiyu. Direction-of-arrival estimation of wideband signals via covariance matrix sparse representation[J]. IEEE Transactions on Signal processing, 2011, 59(9): 4256–4270. doi: 10.1109/tsp.2011.2159214 HE Zhenqing, SHI Zhiping, HUANG Lei, et al. Underdetermined DOA estimation for wideband signals using robust sparse covariance fitting[J]. IEEE Signal Processing Letters, 2015, 22(4): 435–439. doi: 10.1109/LSP.2014.2358084 BUTTON M D, GARDINER J G, and GLOVER I A. Measurement of the impulsive noise environment for satellite-mobile radio systems at 1.5 GHz[J]. IEEE Transactions on Vehicular Technology, 2002, 51(3): 551–560. doi: 10.1109/tvt.2002.1002503 BLACKARD K L, RAPPAPORT T S, and BOSTIAN C W. Measurements and models of radio frequency impulsive noise for indoor wireless communications[J]. IEEE Journal on Selected Areas in Communications, 1993, 11(7): 991–1001. doi: 10.1109/49.233212 NIKIAS C L and SHAO Min. Signal Processing with Alpha-Stable Distributions and Applications[M]. New York: Wiley & Sons, 1995. JIN Fangxiao, QIU Tianshuang, LUAN Shengyang, et al. Joint Estimation of the DOA and the number of sources for wideband signals using cyclic correntropy[J]. IEEE Access, 2019, 7: 42482–42494. doi: 10.1109/ACCESS.2019.2904287 LUAN Shengyang, QIU Tianshuang, ZHU Yongjie, et al. Cyclic correntropy and its spectrum in frequency estimation in the presence of impulsive noise[J]. Signal Processing, 2016, 120: 503–508. doi: 10.1016/j.sigpro.2015.09.023 BLUMENSATH T and DAVIES M E. Normalized iterative hard thresholding: Guaranteed stability and performance[J]. IEEE Journal of Selected Topics in Signal Processing, 2010, 4(2): 298–309. doi: 10.1109/JSTSP.2010.2042411 -
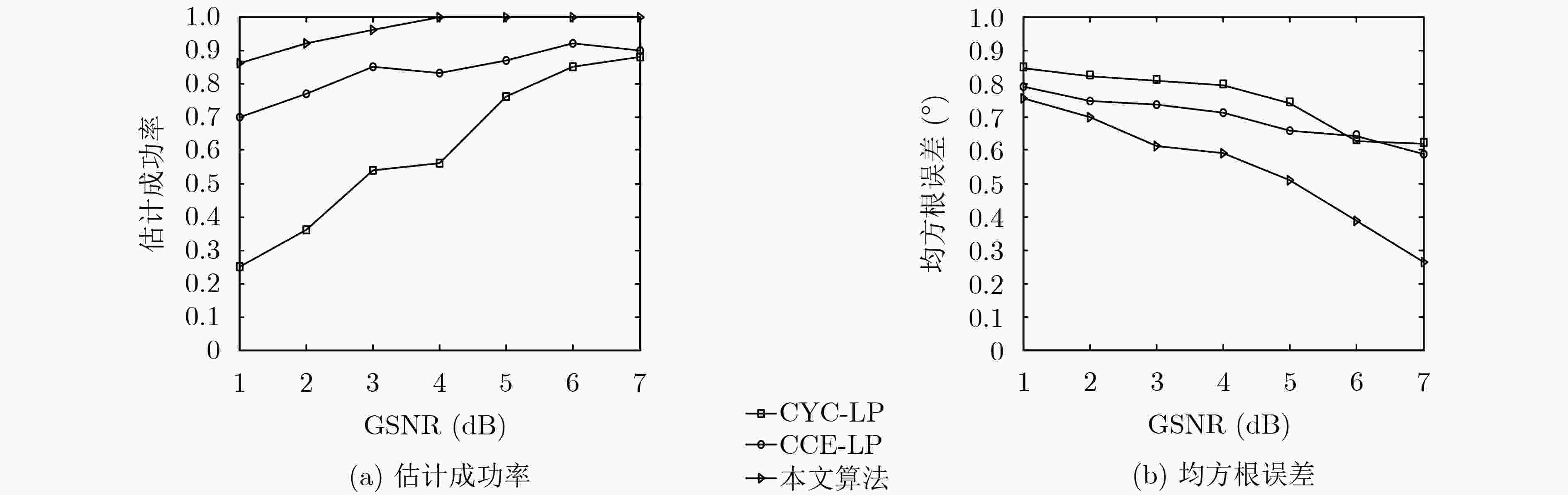
下载:
图(3)
计量
- 文章访问数: 1214
- HTML全文浏览量: 228
- PDF下载量: 96
- 被引次数: 0
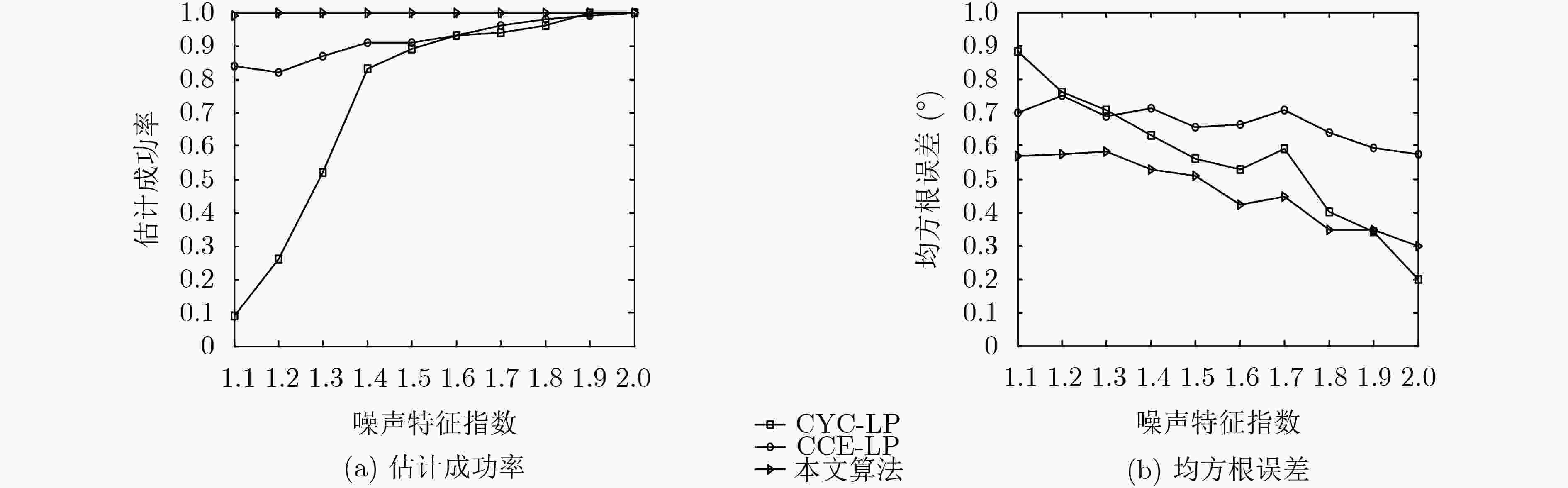
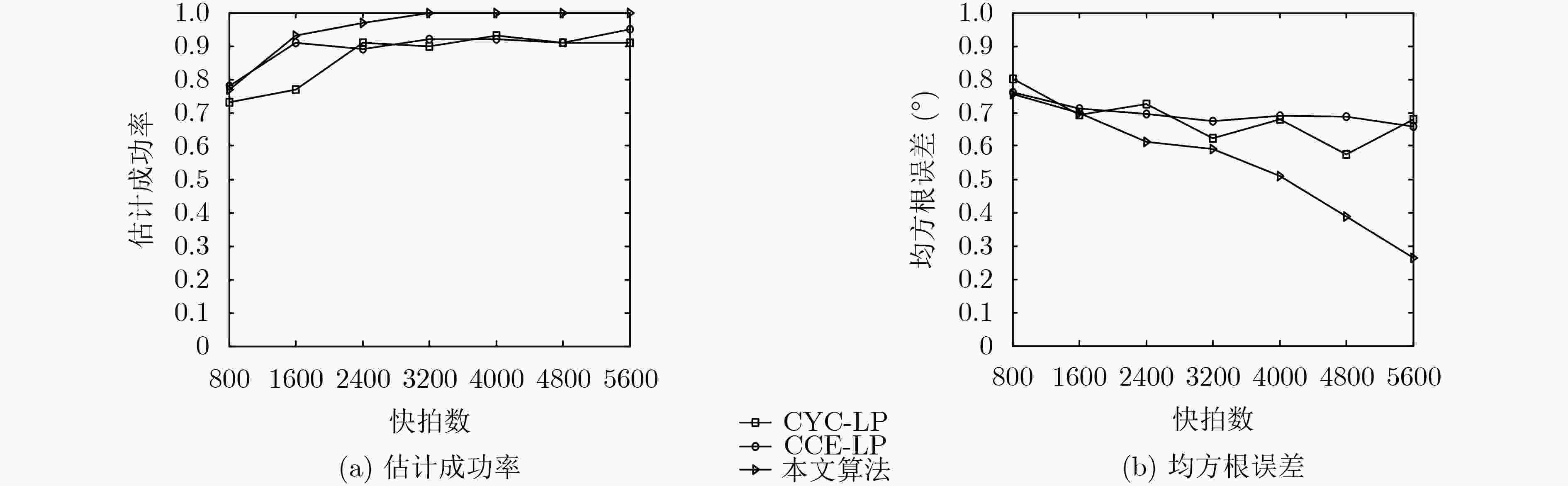
下载:
