Joint Transmitting Subarray Partition and Beamforming Design Method Based on Two-Dimensional Phased-MIMO Radar
-
摘要:
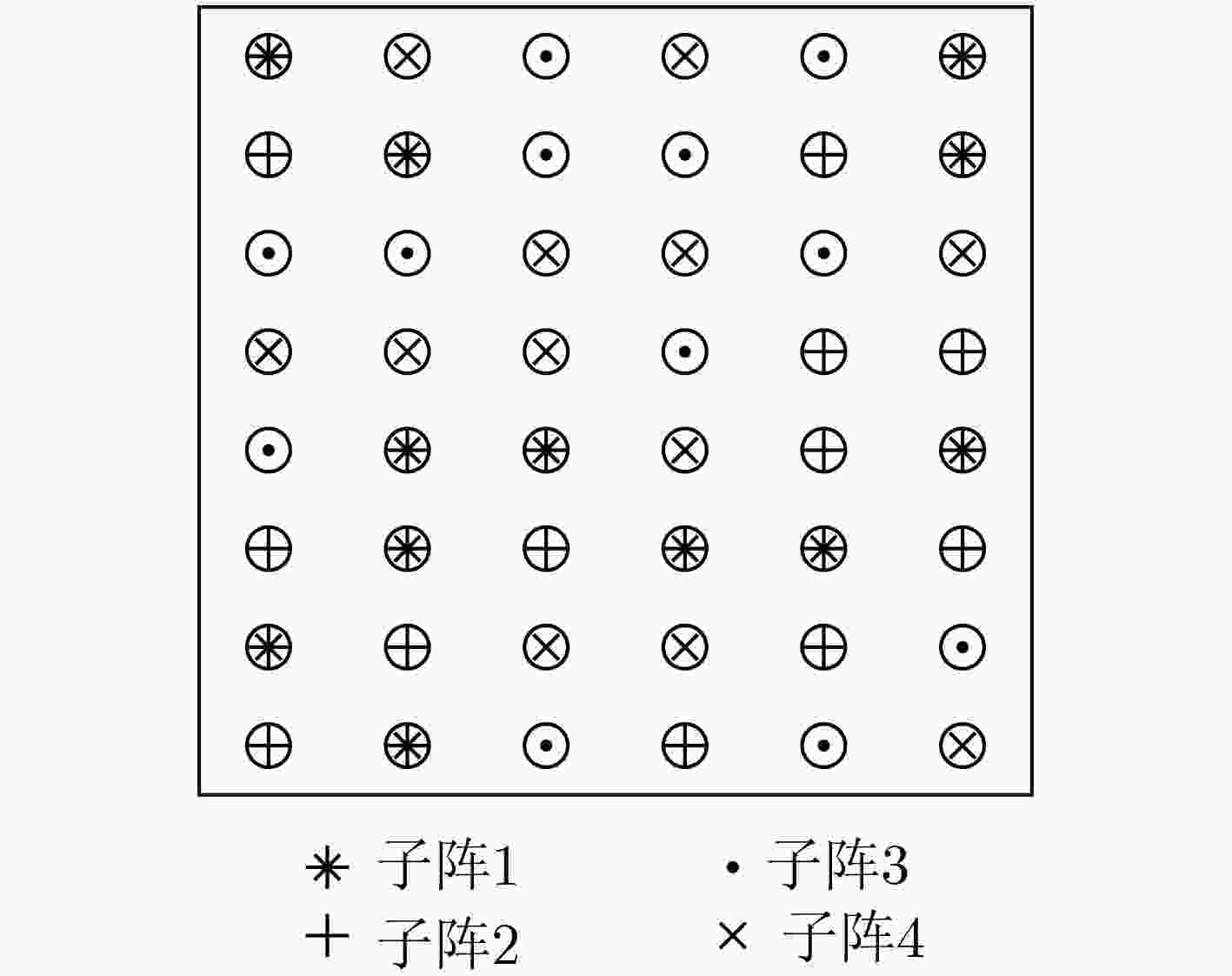
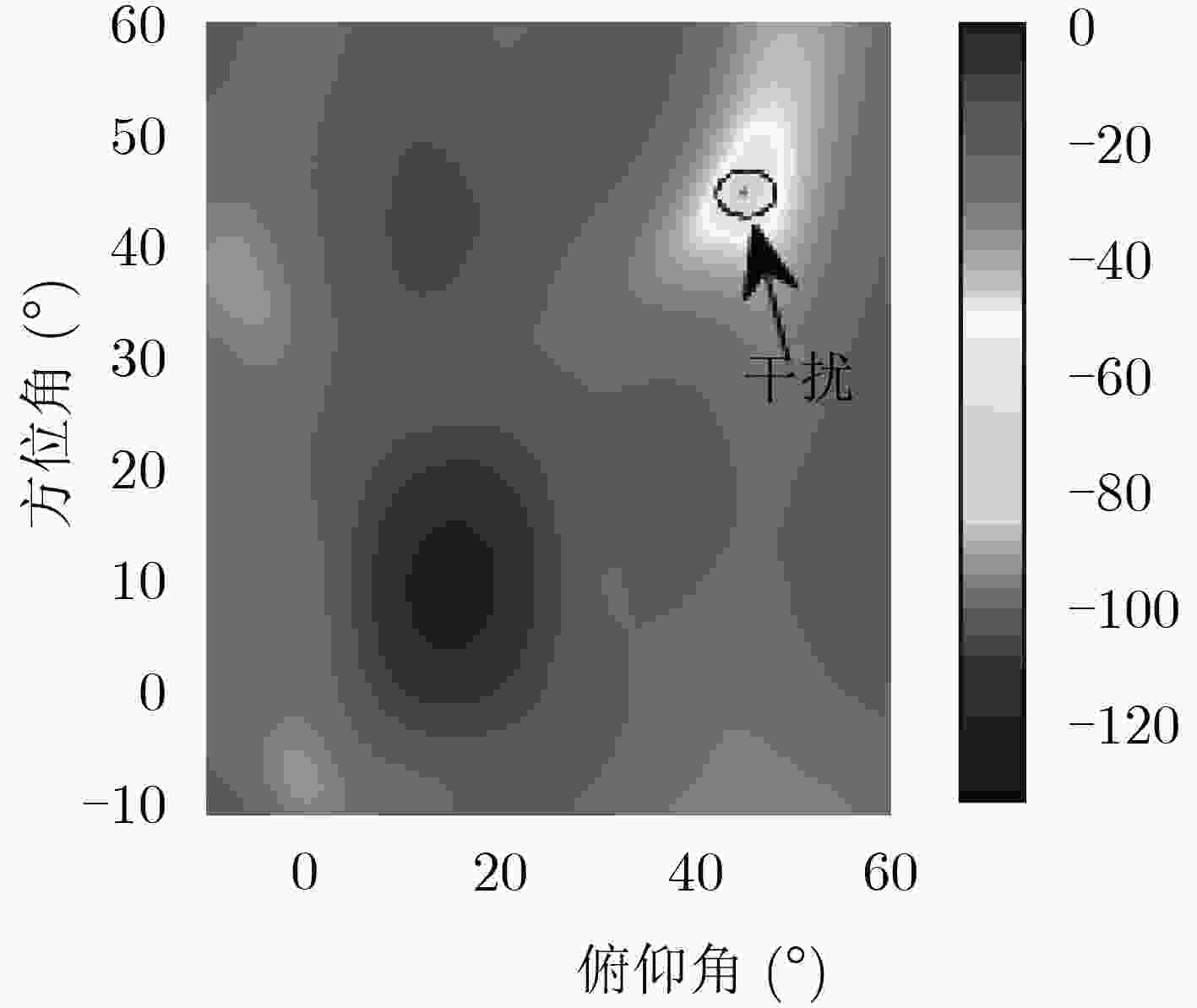
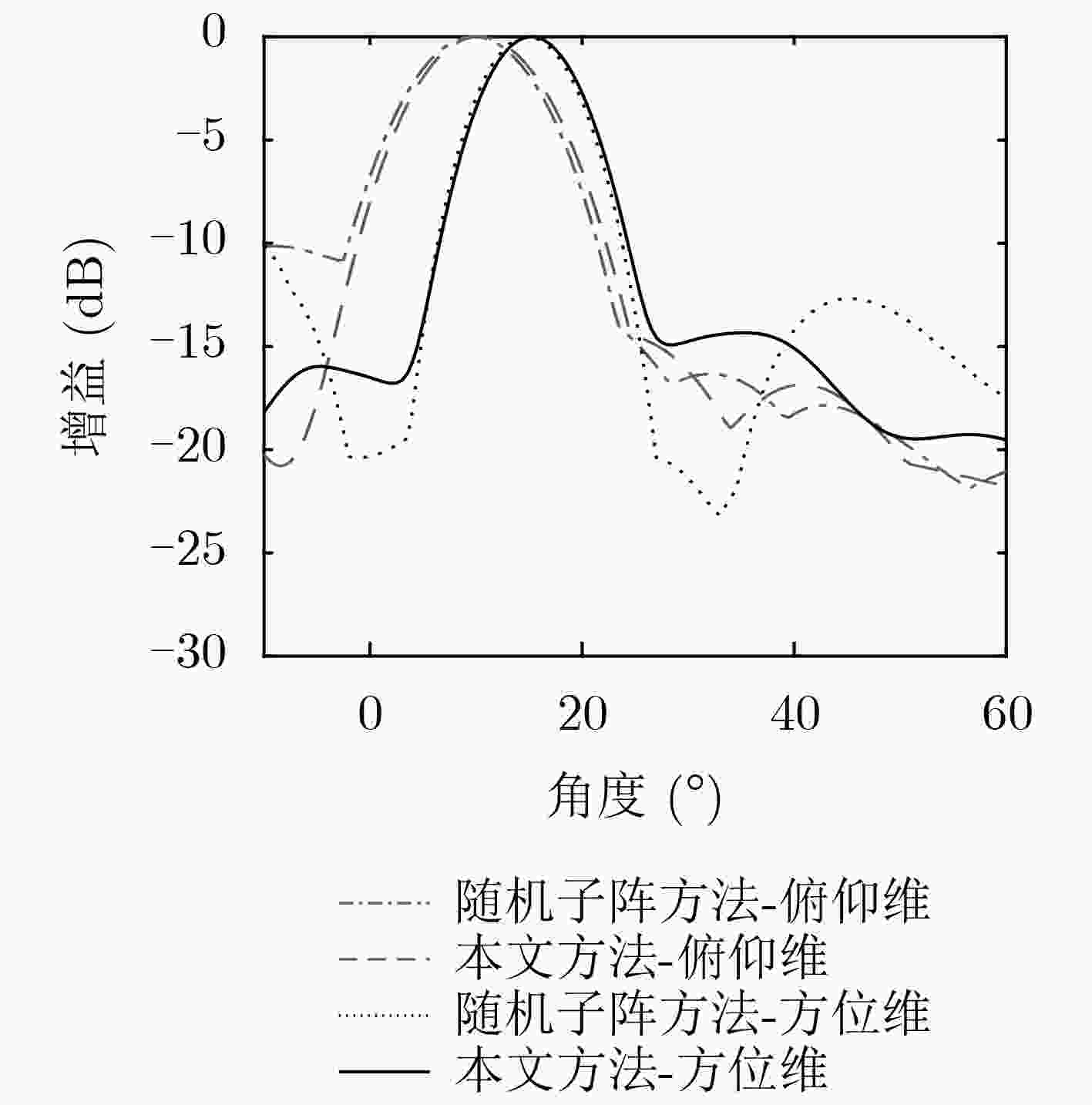
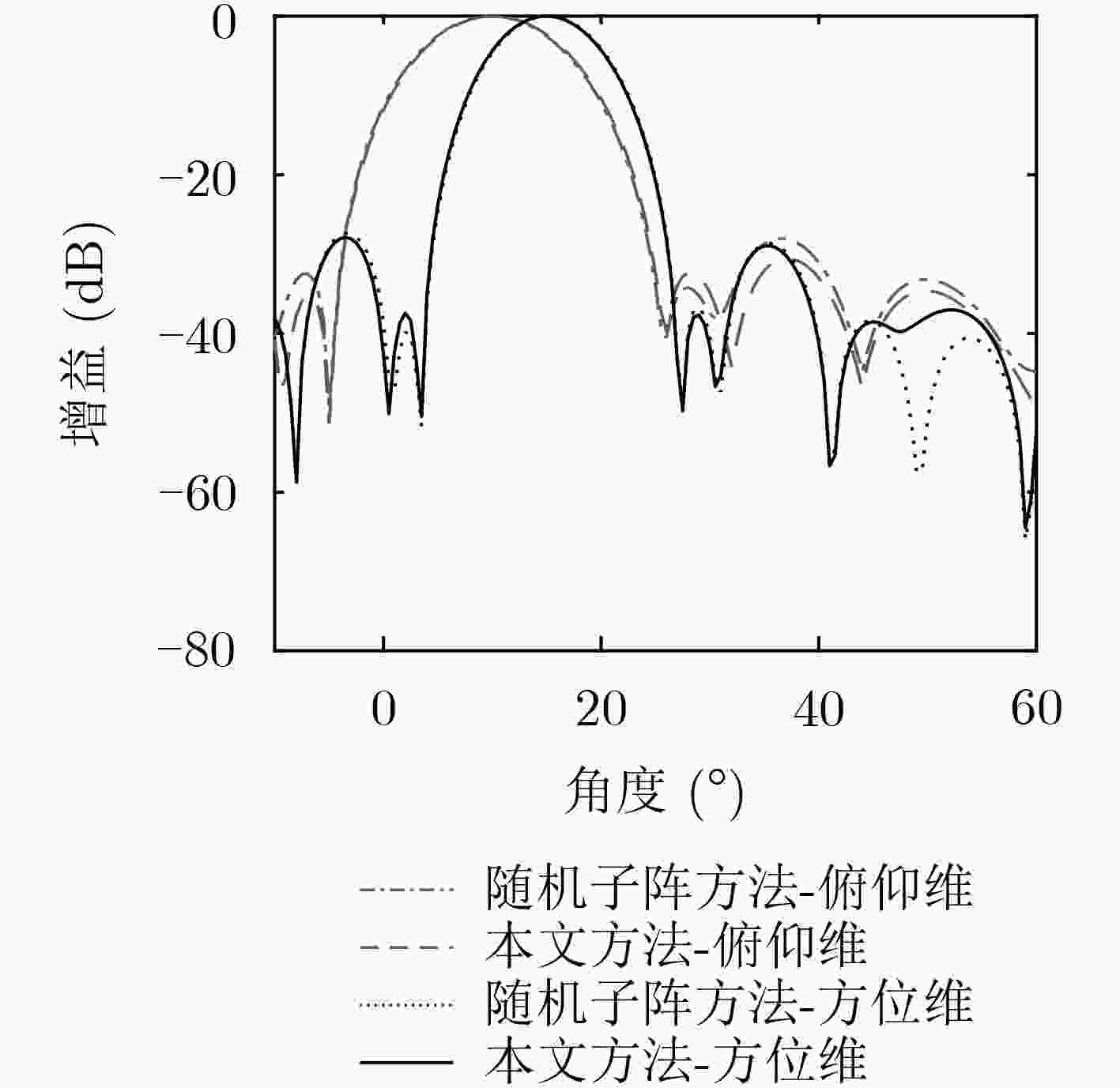
为了有效地抑制干扰信号并进一步提高雷达系统的性能,该文提出一种基于2维相控阵-MIMO雷达的联合发射子阵划分和波束形成设计方法。该方法首先将MIMO雷达系统的发射阵列等分成一定数目的非重叠子阵并给每个天线分配相同的发射能量,以确保发射信号具有恒模特性;其次,在一定的约束条件下,以最大化接收波束形成器的输出信干噪比为准则建立关于子阵结构、每个子阵对应的发射波束形成权矢量以及接收波束形成权矢量的优化模型,并采用循环迭代方法进行求解。仿真结果证实了所提方法的正确性和有效性。
-
关键词:
- 相控阵-MIMO雷达 /
- 干扰抑制 /
- 发射子阵划分 /
- 波束形成
Abstract:In order to suppress effectively the interference signal and improve further the performance of radar system, a joint transmitting subarray partition and beamforming design method based on two-dimensional phased-MIMO radar is proposed. Firstly, the transmitting array of MIMO radar system is equally partitioned into a number of non-overlapping subarrays and the transmit power of each antenna is equal, so as to guarantee that the transmit signal has constant modulus characteristic. Then, the optimization model for subarray structure of transmitting array, transmit beamformer weight vectors and receive beamformer weight vector is established by maximizing the output signal-to-interference-plus-noise ratio of the receive beamformer under certain constraint conditions. Simulation results demonstrate the correctness and effectiveness of the proposed method.
-
表 1 循环迭代算法流程
初始化:初始化子阵个数$K$,目标空间位置$({\theta _0},{\phi _0})$和散射系数${\gamma _0}$, $Q$个依赖于雷达系统发射波形的干扰的空间位置$\{ ({\theta _q},{\phi _q})\} _{q = 1}^Q$和散射
系数$\{ {\gamma _q}\} _{q = 1}^Q$, $P$个不依赖于雷达系统发射波形的干扰的空间位置$\{ ({\theta _p},{\phi _p})\} _{p = 1}^P$和功率$\{ \gamma _p^2\} _{p = 1}^P$,子阵结构${{{F}}^0}$,发射波束形成权矢量
$\{ \bar {{w}}_k^0\} _{k = 1}^K$,系统发射总能量$\eta $,终止阈值$\beta $;步骤 1 固定子阵结构${{{F}}^v}$和发射波束形成权矢量$\{ \bar {{w}}_k^v\} _{k = 1}^K$,根据式(19)计算接收波束形成权矢量${{{g}}^{v + 1}}$; 步骤 2 固定子阵结构${{{F}}^v}$和接收波束形成权矢量${{{g}}^{v + 1}}$,根据式(24)计算发射波束形成权矢量$\{ \bar {{w}}_k^{v + 1}\} _{k = 1}^K$; 步骤 3 固定发射波束形成权矢量$\{ \bar {{w}}_k^{v + 1}\} _{k = 1}^K$和接收波束形成权矢量${{{g}}^{v + 1}}$,根据式(29)和式(30)计算子阵结构${{{F}}^{v + 1}}$; 步骤 4 判断终止条件$|{\rm{SIN} }{ {\rm{R} }^{v + 1} } - {\rm{SIN} }{ {\rm{R} }^v}| \le \beta $是否满足,满足则终止,否则令$v = v+1$并重复步骤1至步骤4。 -
申滨, 赵书锋, 金纯. 基于迭代并行干扰消除的低复杂度大规模MIMO信号检测算法[J]. 电子与信息学报, 2018, 40(12): 2970–2978. doi: 10.11999/JEIT180111SHEN Bin, ZHAO Shufeng, and JIN Chun. Low complexity iterative parallel interference cancellation detection algorithms for massive MIMO systems[J]. Journal of Electronics &Information Technology, 2018, 40(12): 2970–2978. doi: 10.11999/JEIT180111 李慧, 赵永波, 程增飞. 基于线性调频时宽的MIMO雷达正交波形设计[J]. 电子与信息学报, 2018, 40(5): 1151–1158. doi: 10.11999/JEIT170426LI Hui, ZHAO Yongbo, and CHENG Zengfei. MIMO radar orthogonal waveform set design based on chirp durations[J]. Journal of Electronics &Information Technology, 2018, 40(5): 1151–1158. doi: 10.11999/JEIT170426 王昊, 马启明. 修正主模抑制稳健自适应波束形成算法[J]. 电子与信息学报, 2017, 39(11): 2620–2626. doi: 10.11999/JEIT170236WANG Hao and MA Qiming. Modified dominant mode rejection robust adaptive beamforming algorithm[J]. Journal of Electronics &Information Technology, 2017, 39(11): 2620–2626. doi: 10.11999/JEIT170236 ZHOU Gongjian, GUO Zhengkun, CHEN Xi, et al. Statically fused converted measurement kalman filters for phased-array radars[J]. IEEE Transactions on Aerospace and Electronic Systems, 2018, 54(2): 554–568. doi: 10.1109/TAES.2017.2760798 XIONG Jie, WANG Wenqin, and GAO Kuandong. FDA-MIMO radar range-angle estimation: CRLB, MSE, and resolution analysis[J]. IEEE Transactions on Aerospace and Electronic Systems, 2018, 54(1): 284–294. doi: 10.1109/TAES.2017.2756498 HUANG Yan, LIAO Guisheng, XU Jingwei, et al. GMTI and parameter estimation for MIMO SAR system via fast interferometry RPCA method[J]. IEEE Transactions on Geoscience and Remote Sensing, 2018, 56(3): 1774–1787. doi: 10.1109/TGRS.2017.2768243 王宇卓, 朱圣棋, 许京伟. MIMO双基地机载雷达距离模糊杂波抑制方法[J]. 雷达学报, 2018, 7(2): 202–211. doi: 10.12000/JR18016WANG Yuzhuo, ZHU Shengqi, and XU Jingwei. A range-ambiguous clutter suppression method for MIMO bistatic airborne radar[J]. Journal of Radars, 2018, 7(2): 202–211. doi: 10.12000/JR18016 施君南, 纠博, 刘宏伟, 等. 一种基于先验信息的机载MIMO雷达发射方向图设计方法[J]. 电子与信息学报, 2015, 37(5): 1038–1043. doi: 10.11999/JEIT140911SHI Junnan, JIU Bo, LIU Hongwei, et al. A beampattern design method for airborne MIMO radar based on prior information[J]. Journal of Electronics &Information Technology, 2015, 37(5): 1038–1043. doi: 10.11999/JEIT140911 BERGIN J, MCNEIL S, FOMUNDAM L, et al. MIMO phased-array for SMTI radar[C]. 2018 IEEE Aerospace Conference, Big Sky, USA, 2008: 1–7. doi: 10.1109/AERO.2008.4526416. HASSANIEN A and VOROBYOV S A. Why the phased-MIMO radar outperforms the phased-array and MIMO radars[C]. The 18th European Signal Processing Conference, Aalborg, Denmark, 2010: 1234–1238. doi: 10.5281/zenodo.42010. HASSANIEN A and VOROBYOV S A. Phased-MIMO radar: A tradeoff between phased-array and MIMO radars[J]. IEEE Transactions on Signal Processing, 2010, 58(6): 3137–3151. doi: 10.1109/TSP.2010.2043976 LI Hongbin and HIMED B. Transmit subaperturing for MIMO radars with co-located antennas[J]. IEEE Journal of Selected Topics in Signal Processing, 2010, 4(1): 55–65. doi: 10.1109/JSTSP.2009.2038967 DELIGIANNIS A, LAMBOTHARAN S, and CHAMBERS J A. Beamforming for fully-overlapped two-dimensional Phased-MIMO radar[C]. 2015 IEEE Radar Conference, Arlington, USA, 2015: 599–604. doi: 10.1109/RADAR.2015.7131068. KHAN W, QURESHI I M, and SULTAN K. Ambiguity function of phased-MIMO radar with colocated antennas and its properties[J]. IEEE Geoscience and Remote Sensing Letters, 2014, 11(7): 1220–1224. doi: 10.1109/LGRS.2013.2290010 MILLER S L and O'DEA R J. Peak power and bandwidth efficient linear modulation[J]. IEEE Transactions on Communications, 1998, 46(12): 1639–1648. doi: 10.1109/26.737402 ALIELDIN A, HUANG Yi, and SAAD W M. Optimum partitioning of a phased-MIMO radar array antenna[J]. IEEE Antennas and Wireless Propagation Letters, 2017, 16: 2287–2290. doi: 10.1109/LAWP.2017.2714866 LUO Zhiquan, MA W, SO A M C, et al. Semidefinite relaxation of quadratic optimization problems[J]. IEEE Signal Processing Magazine, 2010, 27(3): 20–34. doi: 10.1109/MSP.2010.936019 GRANT M and BOYD S. CVX: Matlab software for disciplined convex programming, version 2.2[OL]. http://cvxr.com/cvx, 2020. BOYD S and VANDENBERGHE L. Convex Optimization[M]. Cambridge: Cambridge University Press, 2004: 291–342. HELMBERG C and RENDL F. Solving quadratic (0, 1)-problems by semidefinite programs and cutting planes[J]. Mathematical Programming, 1998, 82(3): 291–315. doi: 10.1007/bf01580072 -





 下载:
下载:





 下载:
下载:
