Algebraic Solution for 3D Localization of Multistatic Passive Radar in the Presence of Sensor Position Errors
-
摘要:
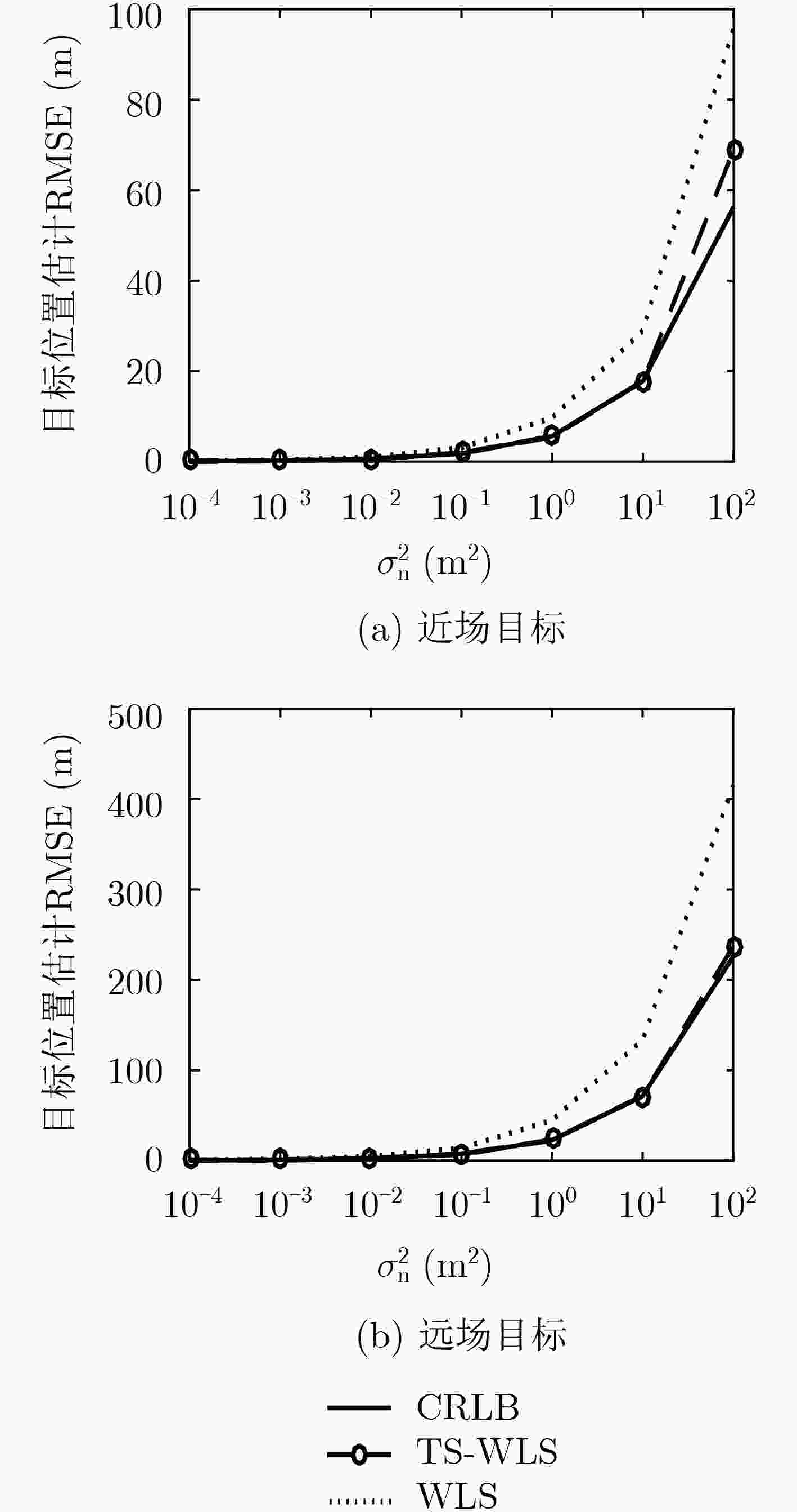
机载外辐射源雷达系统中,部署在飞机上的观测站传感器位置无法精确获知,观测站位置误差将严重影响目标定位精度。对此,该文提出一种观测站位置误差下多基外辐射源雷达3维定位代数解算法。该算法首先利用辅助变量将非线性双基距离和差(BRD)观测方程进行线性化,构造伪线性目标估计模型。然后将观测站位置量测噪声的统计特性融入定位算法,提出一种改进两步加权最小二乘(TS-WLS)算法实现观测站位置误差下外辐射源雷达目标定位。最后推导了克拉美罗下界(CRLB)和算法的理论误差。仿真结果显示,在适中的BRD量测误差和观测站位置误差下,所提算法的目标定位性能能够达到CRLB。
-
关键词:
- 外辐射源雷达 /
- 观测站位置误差 /
- 双基距离和差(BRD) /
- 两步加权最小二乘(TS-WLS)
Abstract:An observer is placed on the airborne in the multistatic passive radar localization system. The error in observer position may seriously affect the localization accuracy. An algebraic closed-form solution is proposed for 3D localization of multistatic passive radar in the presence of sensor position errors. Firstly, the nonlinear Bistatic Range Difference (BRD) measurement equations are linearized by proper additional parameters and a pseudo-linear estimation model is given accordingly. Then a modified Two-Step Weighted Least Squares (TS-WLS) algorithm is developed with considering the statistic characteristics of the observer position measurement noises. Finally the Cramer-Rao Lower Bound (CRLB) and the theoretical error of the algorithm are derived. Simulation results show that the proposed algorithm can achieve the CRLB in a moderate level of noises.
-
LIU Jun, LI Hongbin, and HIMED B. On the performance of the cross-correlation detector for passive radar applications[J]. Signal Processing, 2015, 113: 32–37. doi: 10.1016/j.sigpro.2015.01.006 INGGS M, TONG C, NADJIASNGAR R, et al. Planning and design phases of a commensal radar system in the FM broadcast band[J]. IEEE Aerospace and Electronic Systems Magazine, 2014, 29(7): 50–63. doi: 10.1109/MAES.2014.130165 CHOI S, CROUSE D, WILLETT P, et al. Multistatic target tracking for passive radar in a DAB/DVB network: Initiation[J]. IEEE Transactions on Aerospace and Electronic Systems, 2015, 51(3): 2460–2469. doi: 10.1109/TAES.2015.130270 GASSIER G, CHABRIEL G, BARRÈRE J, et al. A unifying approach for disturbance cancellation and target detection in passive radar using OFDM[J]. IEEE Transactions on Signal Processing, 2016, 64(22): 5959–5971. doi: 10.1109/TSP.2016.2600511 王鼎, 魏帅. 基于外辐射源的约束总体最小二乘定位算法及其理论性能分析[J]. 中国科学: 信息科学, 2015, 45(11): 1466–1489. doi: 10.1360/N112014-00397WANG Ding and WEI Shuai. The constrained-total-least-squares localization algorithm and performance analysis based on an external illuminator[J]. Scientia Sinica Informationis, 2015, 45(11): 1466–1489. doi: 10.1360/N112014-00397 MALANOWSKI M and KULPA K. Two methods for target localization in multistatic passive radar[J]. IEEE Transactions on Aerospace and Electronic Systems, 2012, 48(1): 572–580. doi: 10.1109/TAES.2012.6129656 NOROOZI A and SEBT M A. Target localization in multistatic passive radar using SVD approach for eliminating the nuisance parameters[J]. IEEE Transactions on Aerospace and Electronic Systems, 2017, 53(4): 1660–1671. doi: 10.1109/TAES.2017.2669558 CHAN Y T and HO K C. A simple and efficient estimator for hyperbolic location[J]. IEEE Transactions on Signal Processing, 1994, 42(8): 1905–1915. doi: 10.1109/78.301830 WANG Yue and HO K C. TDOA source localization in the presence of synchronization clock bias and sensor position errors[J]. IEEE Transactions on Signal Processing, 2013, 61(18): 4532–4544. doi: 10.1109/TSP.2013.2271750 曲付勇, 孟祥伟. 基于约束总体最小二乘方法的到达时差到达频差无源定位算法[J]. 电子与信息学报, 2014, 36(5): 1075–1081. doi: 10.3724/SP.J.1146.2013.01019QU Fuyong and MENG Xiangwei. Source localization using TDOA and FDOA measurements based on constrained total least squares algorithm[J]. Journal of Electronics &Information Technology, 2014, 36(5): 1075–1081. doi: 10.3724/SP.J.1146.2013.01019 GODRICH H, HAIMOVICH A M, and BLUM R S. Target localization accuracy gain in MIMO radar-based systems[J]. IEEE Transactions on Information Theory, 2010, 56(6): 2783–2803. doi: 10.1109/tit.2010.2046246 ALAM M and JAMIL K. Maximum likelihood (ML) based localization algorithm for multi-static passive radar using range-only measurements[C]. 2015 IEEE Radar Conference, Johannesburg, South Africa, 2015: 180–184. doi: 10.1109/RadarConf.2015.7411876. NOROOZI A and SEBT M A. Target localization from bistatic range measurements in multi-transmitter multi-receiver passive radar[J]. IEEE Signal Processing Letters, 2015, 22(12): 2445–2449. doi: 10.1109/LSP.2015.2491961 NOROOZI A and SEBT M A. Weighted least squares target location estimation in multi-transmitter multi-receiver passive radar using bistatic range measurements[J]. IET Radar, Sonar & Navigation, 2016, 10(6): 1088–1097. doi: 10.1049/iet-rsn.2015.0446 AMIRI R, BEHNIA F, and ZAMANI H. Asymptotically efficient target localization from bistatic range measurements in distributed MIMO radars[J]. IEEE Signal Processing Letters, 2017, 24(3): 299–303. doi: 10.1109/LSP.2017.2660545 AMIRI R and BEHNIA F. An efficient weighted least squares estimator for elliptic localization in distributed MIMO radars[J]. IEEE Signal Processing Letters, 2017, 24(6): 902–906. doi: 10.1109/LSP.2017.2697500 NOROOZI A, OVEIS A H, and SEBT M A. Iterative target localization in distributed MIMO radar from bistatic range measurements[J]. IEEE Signal Processing Letters, 2017, 24(11): 1709–1713. doi: 10.1109/LSP.2017.2747479 AMIRI R, BEHNIA F, and SADR M A M. Exact solution for elliptic localization in distributed MIMO radar systems[J]. IEEE Transactions on Vehicular Technology, 2018, 67(2): 1075–1086. doi: 10.1109/TVT.2017.2762631 赵勇胜, 赵拥军, 赵闯, 等. 一种新的分布式MIMO雷达系统运动目标定位代数解算法[J]. 电子与信息学报, 2018, 40(3): 548–556. doi: 10.11999/JEIT170510ZHAO Yongsheng, ZHAO Yongjun, ZHAO Chuang, et al. New algebraic algorithm for moving target localization in distributed MIMO radar systems[J]. Journal of Electronics &Information Technology, 2018, 40(3): 548–556. doi: 10.11999/JEIT170510 ZHAO Yongsheng, ZHAO Yongjun, and ZHAO Chuang. A novel algebraic solution for moving target localization in multi-transmitter multi-receiver passive radar[J]. Signal Processing, 2018, 143: 303–310. doi: 10.1016/j.sigpro.2017.09.014 -





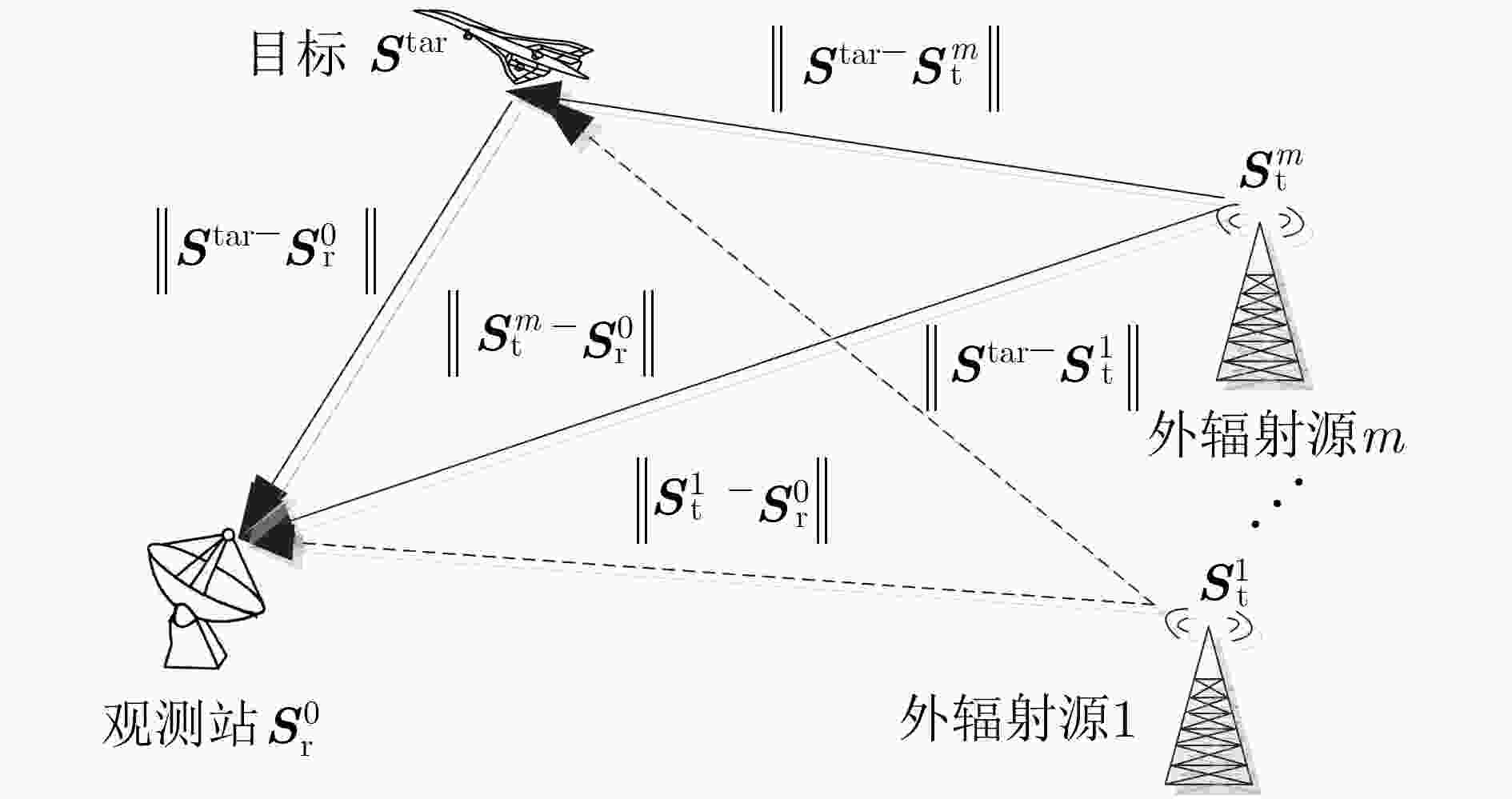
 下载:
下载:

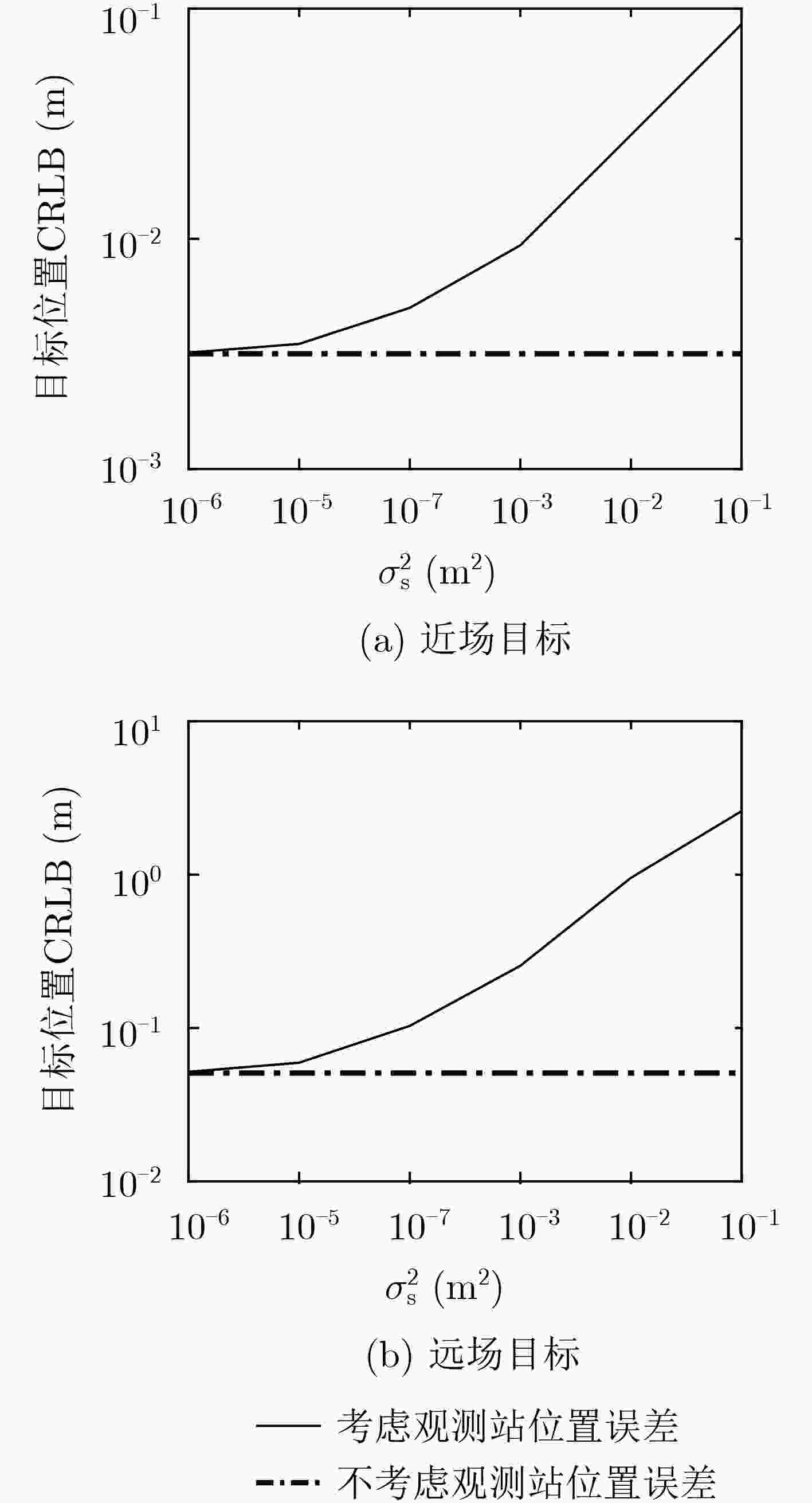
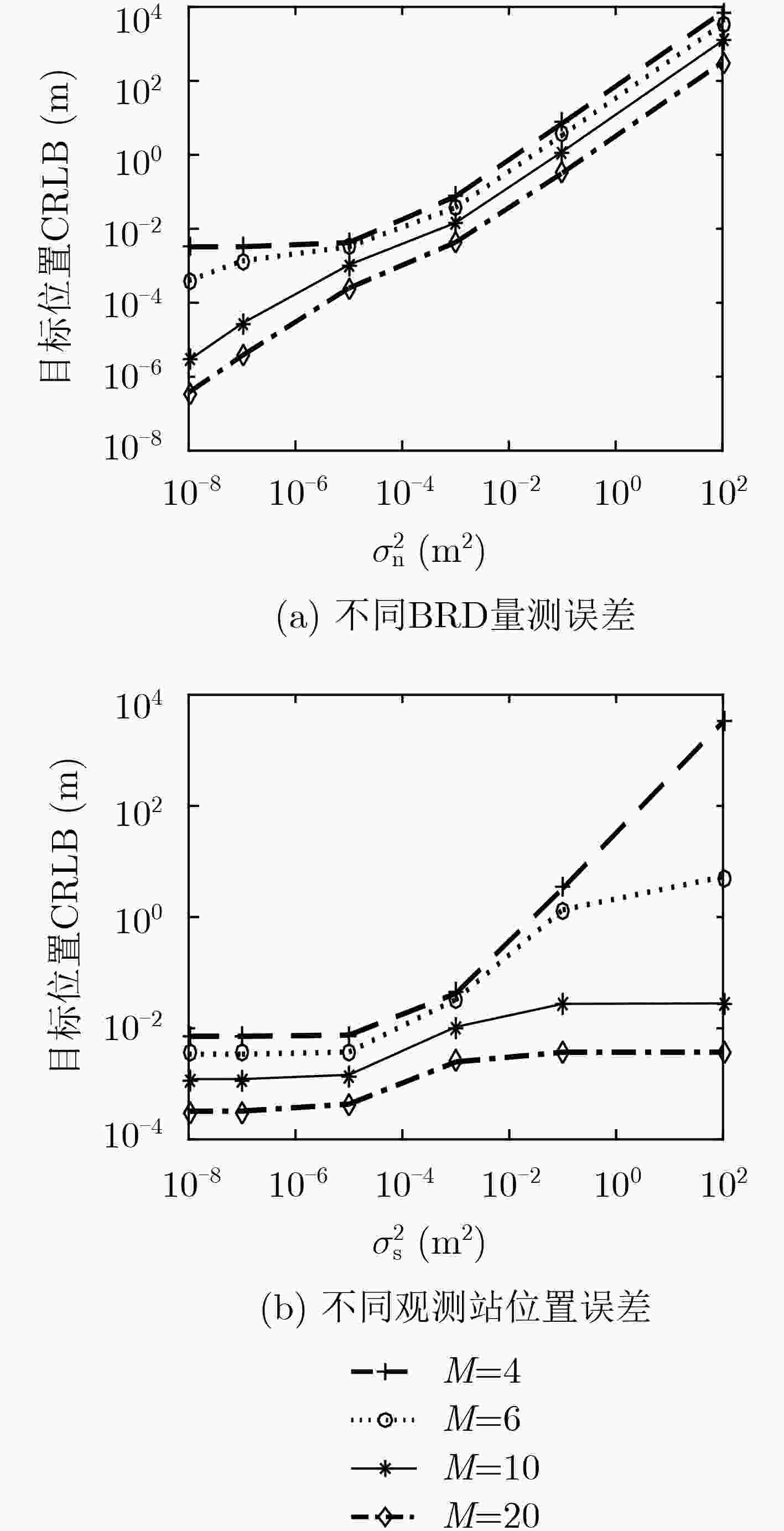
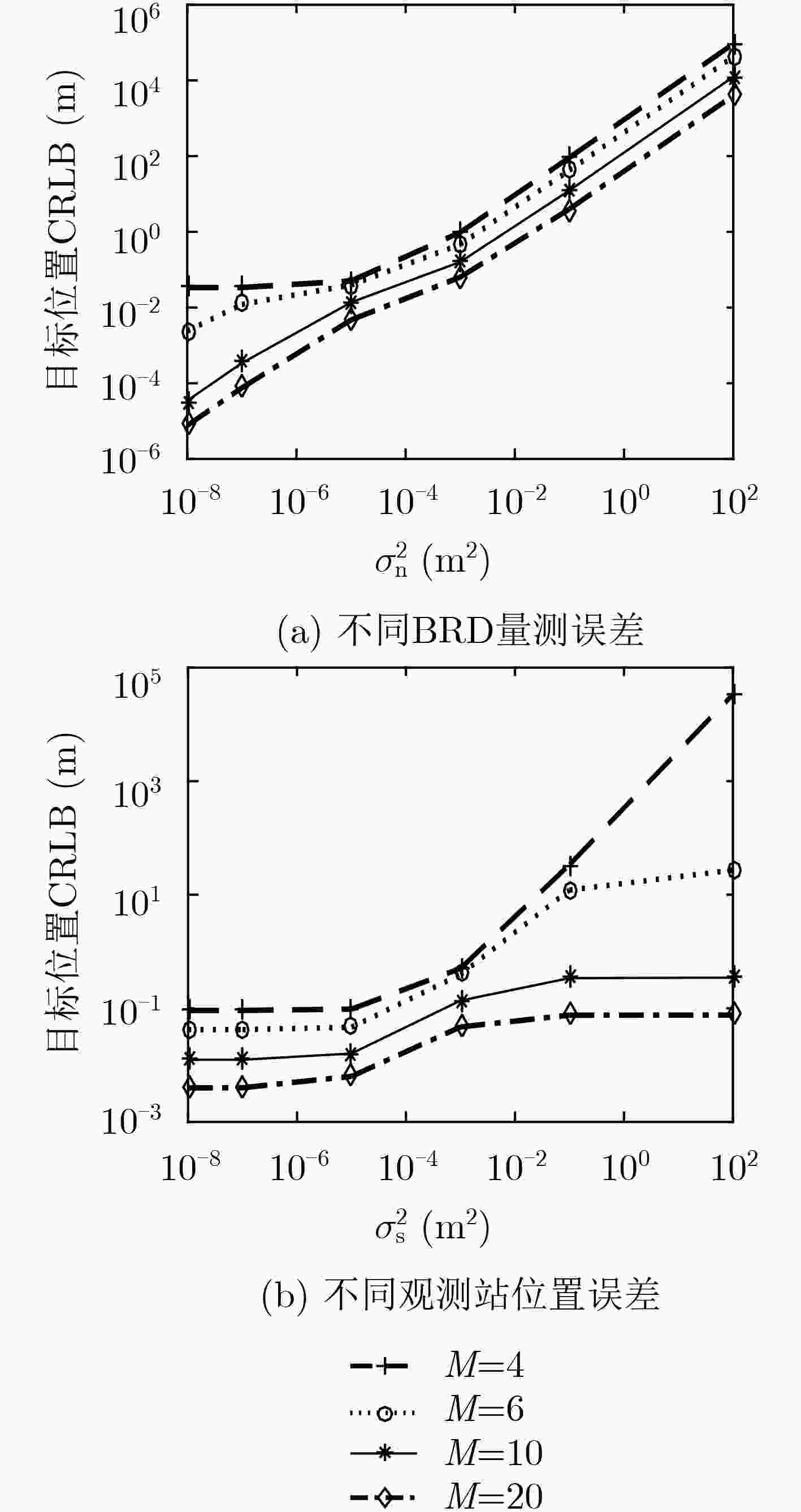



 下载:
下载:
