Individual Recognition Algorithm of IFF Radiation Sources Based on Ensemble Intrinsic Time-scale Decomposition
-
摘要:
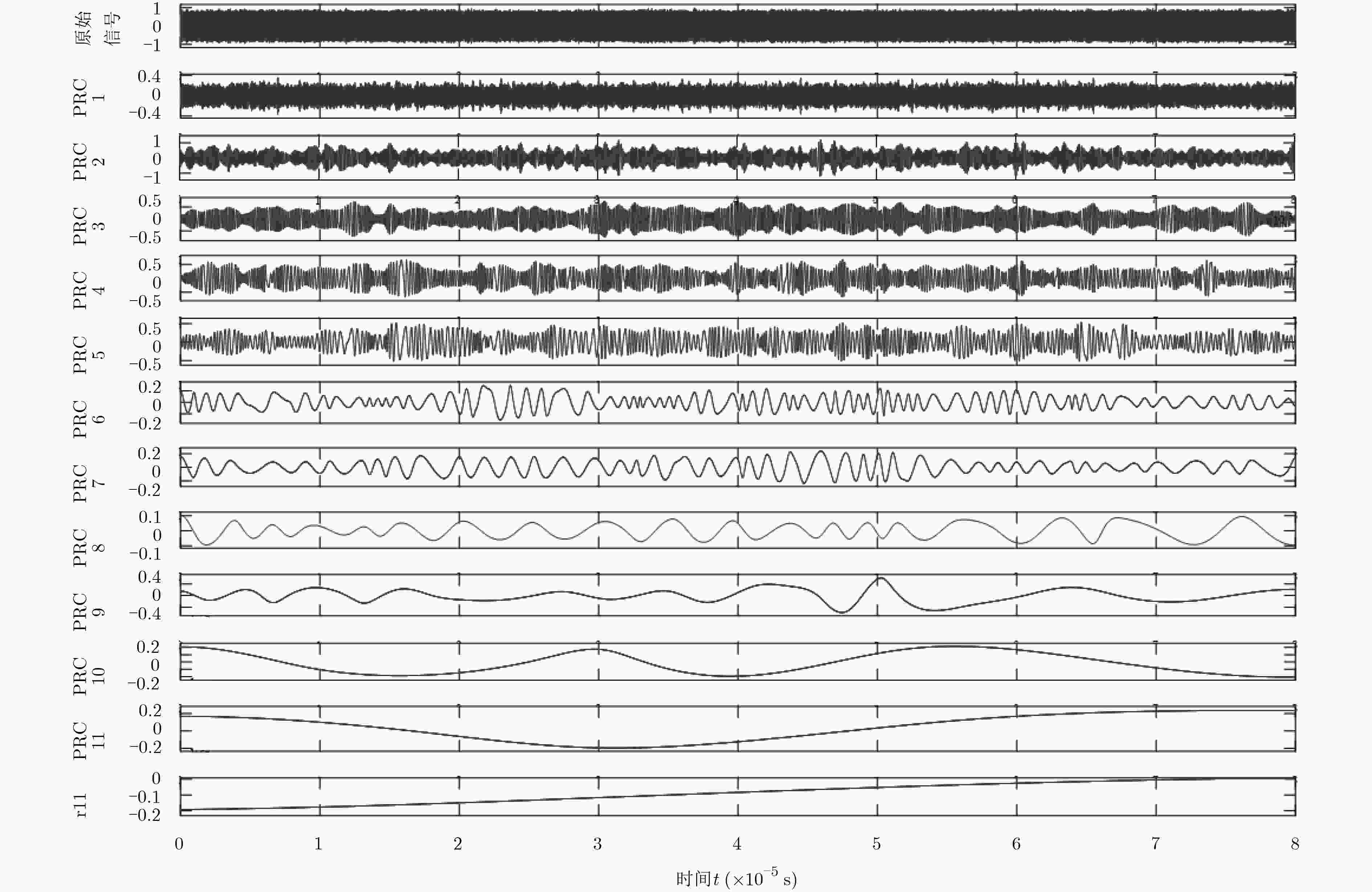
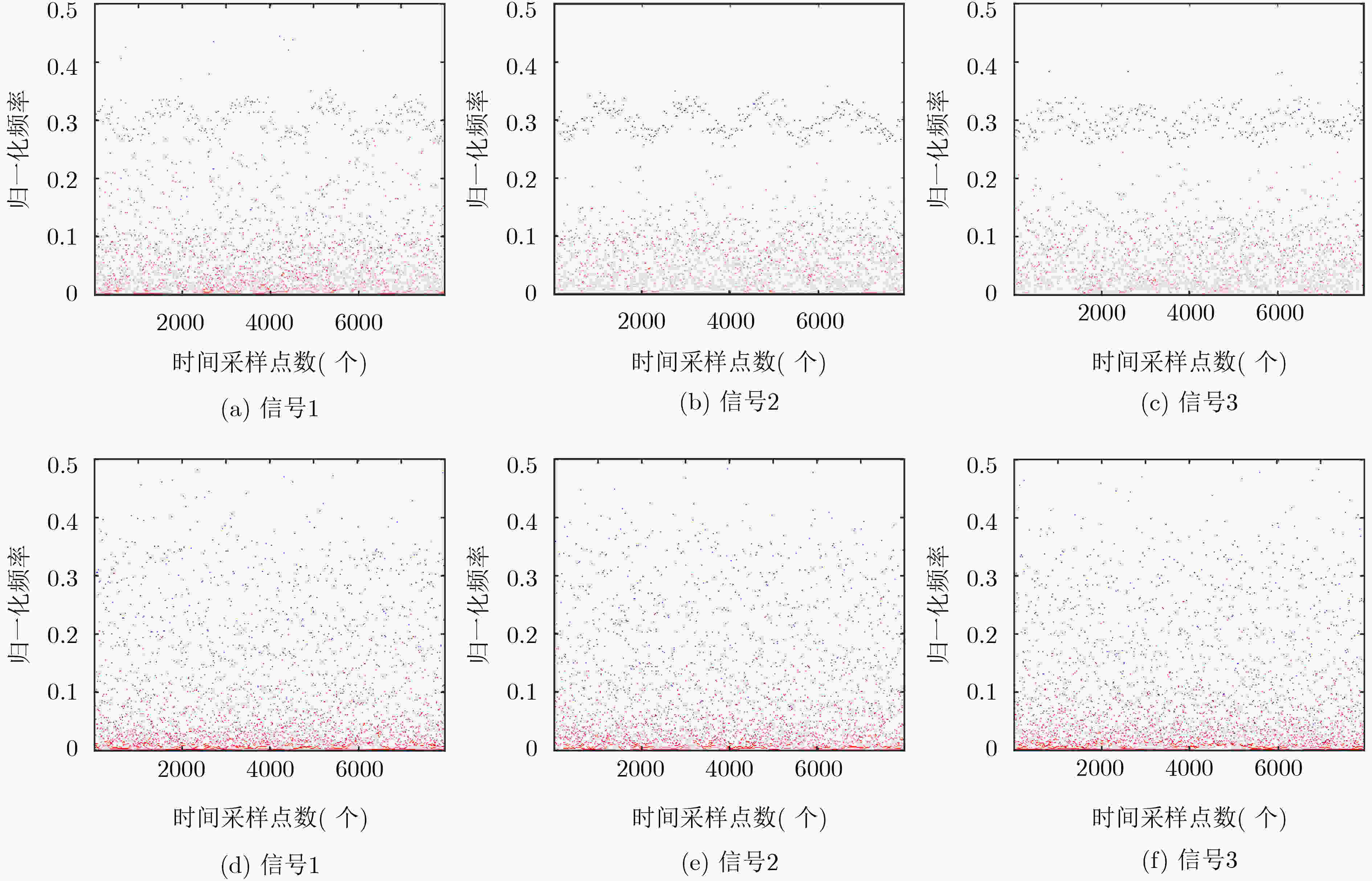
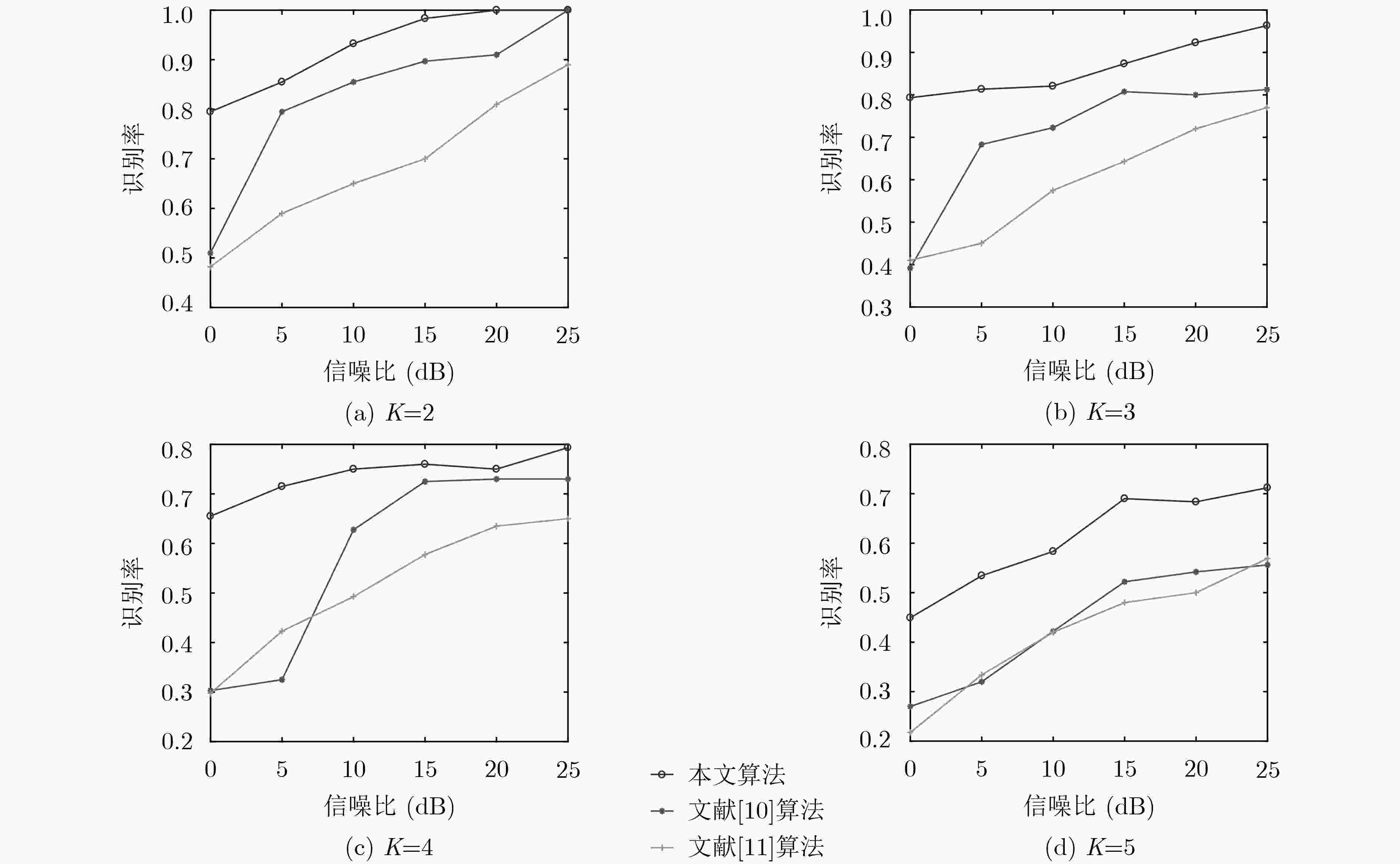
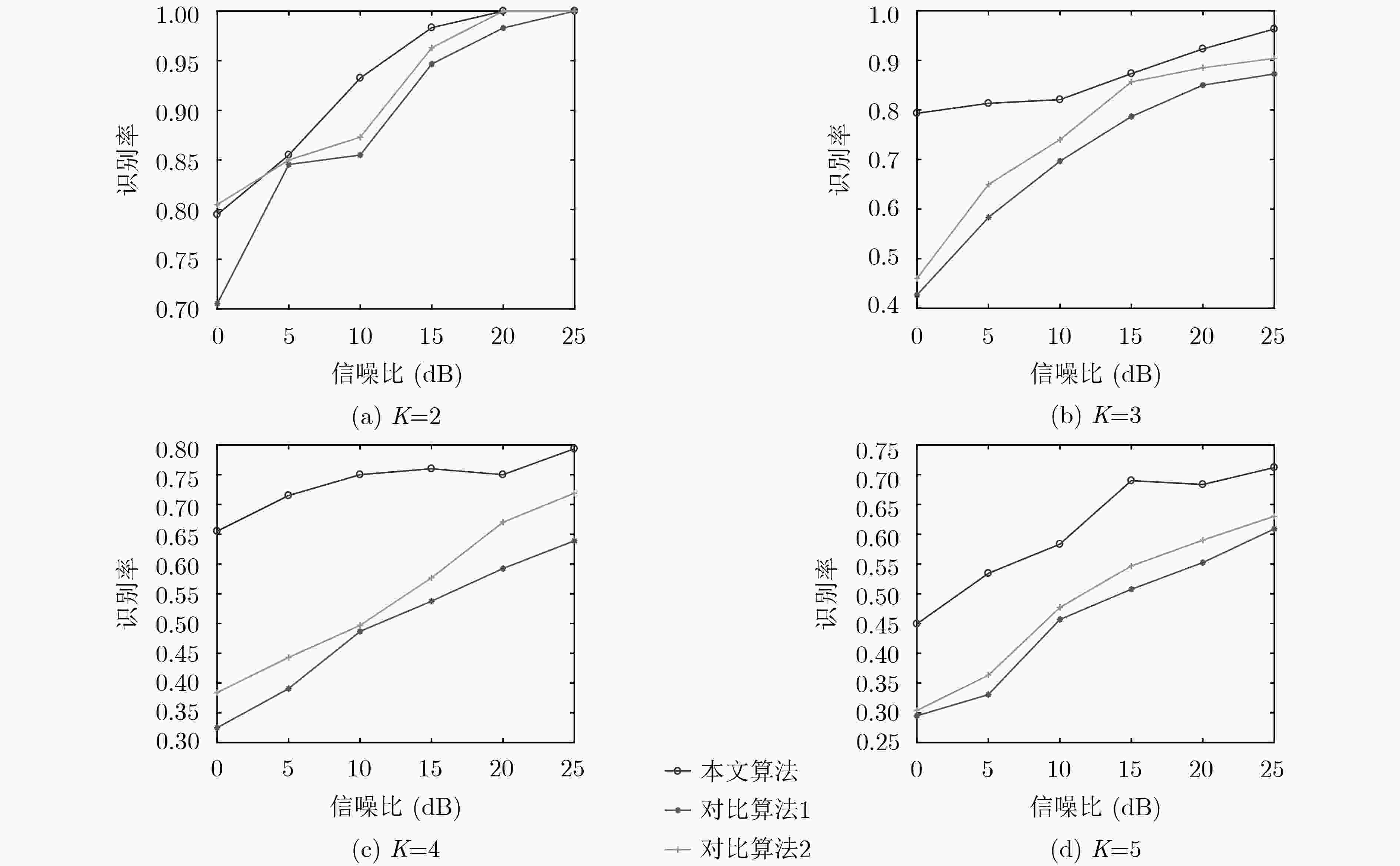
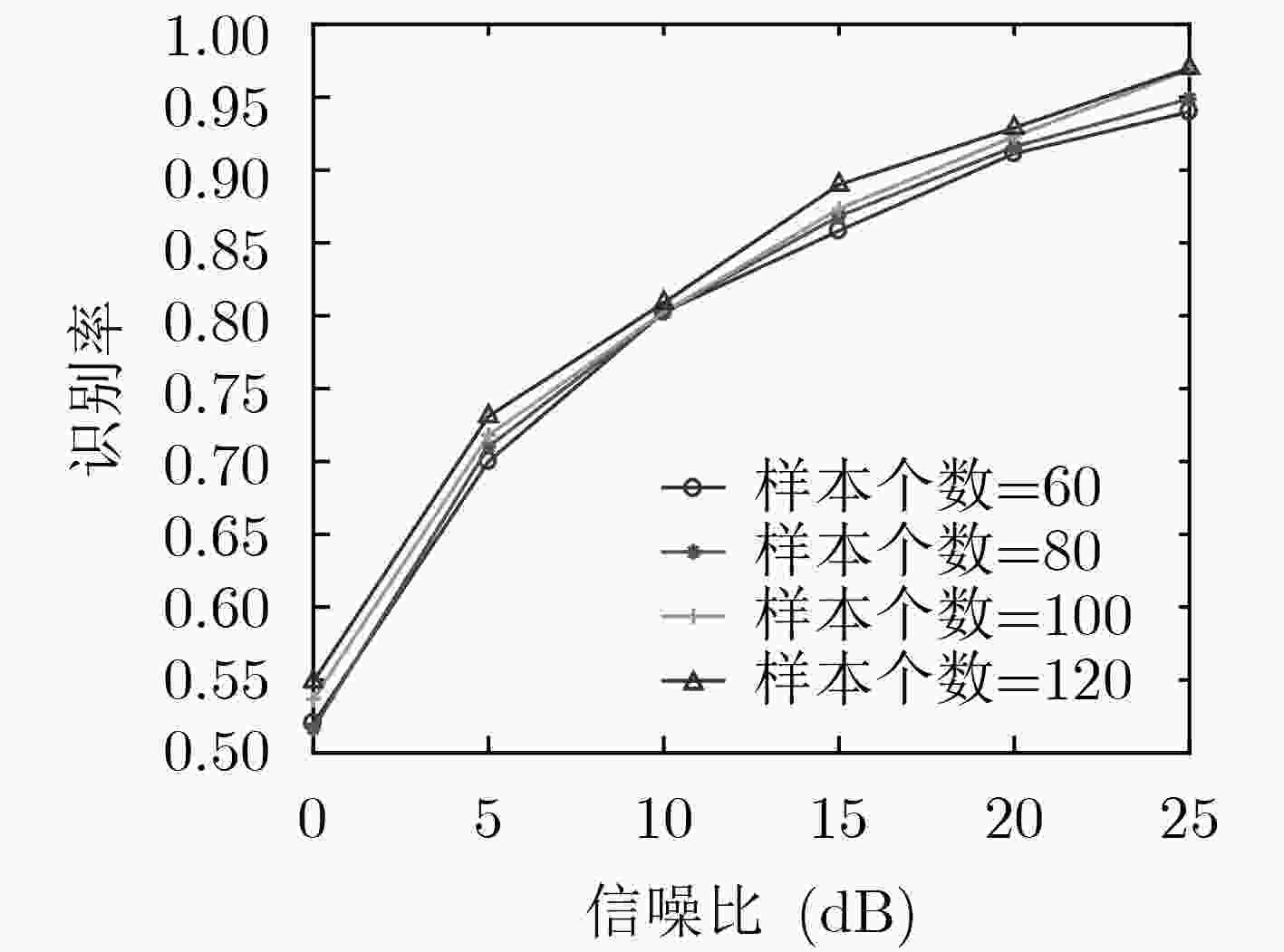
为研究敌我识别(IFF)辐射源信号的细微特征,针对目前在复杂噪声环境中IFF辐射源个体识别研究不足的问题,该文提出一种基于集成固有时间尺度分解的IFF辐射源个体识别算法。该算法应用集成固有时间尺度分解(EITD)将采样信号自适应划分为若干有实际意义的信号分量并求取IFF辐射源信号在时频域的能量分布图。通过对时频能量谱的纹理分析,以图像的纹理特征表征辐射源信号的无意调制特征,送入支持向量机(SVM)中进行分类识别。实验表明,所提算法相较于基于希尔伯特-黄变换(HHT)、基于固有时间尺度分解(ITD)的辐射源个体识别方法在识别准确度上有较大提升。
Abstract:In order to study the subtle feature recognition of Identification Foe or Friend (IFF) radiation source signals, this paper proposes an IFF individual recognition method based on ensemble intrinsic time-scale decomposition to solve the problem of insufficient research on individual identification of IFF radiation source in complex noise environment. In this algorithm, the Ensemble Intrinsic Time-scale Decomposition (EITD) is applied to dividing the sampled signals into several practical signal components and obtaining the energy distribution diagram of the IFF radiation source signals in time-frequency domain. Through the texture analysis of time-frequency energy spectrum, the unintentional modulation feature of the radiation source signals is represented by the texture features of the image, which are sent to the Support Vector Machine (SVM) for classification and recognition. Experiments show that the proposed method is more accurate than the Hilbert-Huang Transform (HHT) and Inherent Time scale Decomposition (ITD) based method.
-
表 1 各方法的平均运算时间(ms)
方法 ITD EMD EITD EITD (迭代次数5) 运算时间 8.4 19.0 21.3 10.2 -
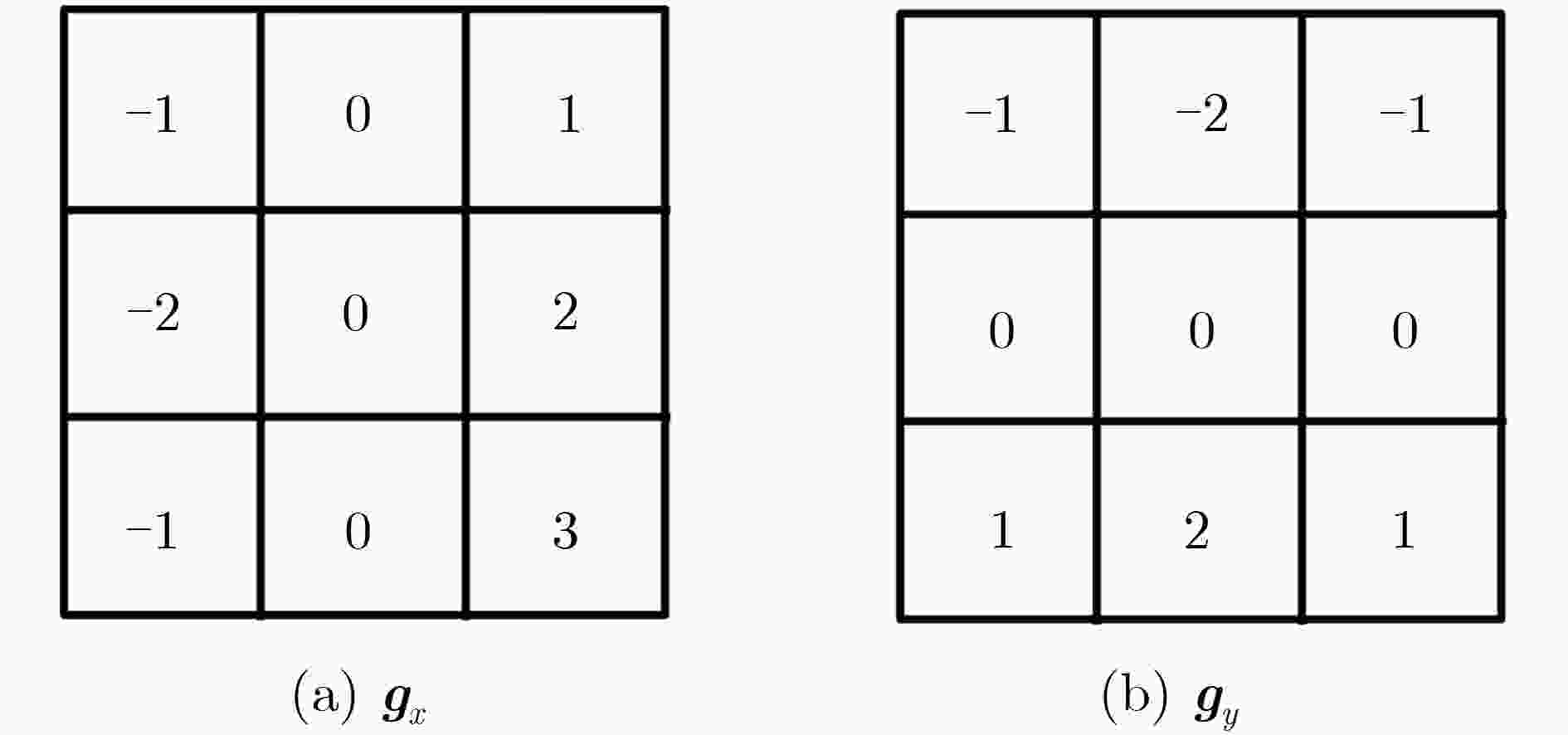
谭源泉, 李胜强, 王厚军. 西方体制Mark XIIA的Mode5数据格式分析[J]. 电子科技大学学报, 2011, 40(4): 532–536. doi: 10.3969/j.issn.1001-0548.2011.04.011TAN Yuanquan, LI Shengqiang, and WANG Houjun. Analysis on data format of Mode 5 in western Mark XIIA[J]. Journal of University of Electronic Science and Technology of China, 2011, 40(4): 532–536. doi: 10.3969/j.issn.1001-0548.2011.04.011 LIU Mingwei and DOHERTY J F. Nonlinearity estimation for specific emitter identification in multipath channels[J]. IEEE Transactions on Information Forensics and Security, 2011, 6(3): 1076–1085. doi: 10.1109/TIFS.2011.2134848 龙慧敏. 基于时域调制域特征的 IFF 模式5信号识别[J]. 电讯技术, 2014, 54(7): 910–914. doi: 10.3969/j.issn.1001-893x.2014.07.009LONG Huimin. Identification of IFF Mode 5 signals based on time & modulation domain characteristics[J]. Telecommunication Engineering, 2014, 54(7): 910–914. doi: 10.3969/j.issn.1001-893x.2014.07.009 李维科. 基于时域和编码特征的Mark XIIA IFF信号识别方法[J]. 兵器装备工程学报, 2016, 37(7): 153–157. doi: 10.11809/scbgxb2016.07.033LI Weike. Identification of Mark XIIA IFF signals based on time domain and coding characteristics[J]. Journal of Ordnance Equipment Engineering, 2016, 37(7): 153–157. doi: 10.11809/scbgxb2016.07.033 许程成, 周青松, 张剑云, 等. 导数约束平滑条件下基于模糊函数特征的雷达辐射源信号识别方法[J]. 电子学报, 2018, 46(7): 1663–1668. doi: 10.3969/j.issn.0372-2112.2018.07.018XU Chengcheng, ZHOU Qingsong, ZHANG Jianyun, et al. Radar emitter recognition based on ambiguity function features with derivative constraint on smoothing[J]. Acta Electronica Sinica, 2018, 46(7): 1663–1668. doi: 10.3969/j.issn.0372-2112.2018.07.018 WANG Xuebao, HUANG Gaoming, ZHOU Zhiwen, et al. Radar emitter recognition based on the energy cumulant of short time fourier transform and reinforced deep belief network[J]. Sensors, 2018, 18(9): 3103. doi: 10.3390/s18093103 LIU Shaokun, YAN Xiaopeng, LI Ping, et al. Radar emitter recognition based on SIFT position and scale features[J]. IEEE Transactions on Circuits and Systems II: Express Briefs, 2018, 65(12): 2062–2066. doi: 10.1109/TCSII.2018.2819666 赵越, 陈之纯, 纠博, 等. 一种基于时频分析的窄带雷达飞机目标分类特征提取方法[J]. 电子与信息学报, 2017, 39(9): 2225–2231. doi: 10.11999/JEIT161204ZHAO Yue, CHEN Zhichun, JIU Bo, et al. Narrowband aircraft targets feature extraction and classification based on time-frequency analysis[J]. Journal of Electronics &Information Technology, 2017, 39(9): 2225–2231. doi: 10.11999/JEIT161204 张天骐, 全盛荣, 强幸子, 等. 基于多尺度Chirplet稀疏分解和Wigner-Ville变换的时频分析方法[J]. 电子与信息学报, 2017, 39(6): 1333–1339. doi: 10.11999/JEIT160750ZHANG Tianqi, QUAN Shengrong, QIANG Xingzi, et al. Time-frequency analysis method based on multi-scale Chirplet sparse decomposition and Wigner-Ville transform[J]. Journal of Electronics &Information Technology, 2017, 39(6): 1333–1339. doi: 10.11999/JEIT160750 任东方, 张涛, 韩洁, 等. 基于ITD与纹理分析的特定辐射源识别方法[J]. 通信学报, 2017, 38(12): 160–168. doi: 10.11959/j.issn.1000-436x.2017299REN Dongfang, ZHANG Tao, HAN Jie, et al. Specific emitter identification based on ITD and texture analysis[J]. Journal on Communications, 2017, 38(12): 160–168. doi: 10.11959/j.issn.1000-436x.2017299 ZHANG Jingwen, WANG Fanggang, DOBRE O A, et al. Specific emitter identification via Hilbert-Huang transform in single-hop and relaying scenarios[J]. IEEE Transactions on Information Forensics and Security, 2016, 11(6): 1192–1205. doi: 10.1109/tifs.2016.2520908 WU Zhaohua and HUANG N E. Ensemble empirical mode decomposition: A noise-assisted data analysis method[J]. Advances in Adaptive Data Analysis, 2009, 1(1): 1–41. doi: 10.1142/S1793536909000047 FREI M G and OSORIO I. Intrinsic time-scale decomposition: Time-frequency-energy analysis and real-time filtering of non-stationary signals[J]. The Royal Society A: Mathematical, Physical and Engineering Sciences, 2007, 463(2078): 321–342. doi: 10.1098/rspa.2006.1761 HU Aijun, YAN Xiaoan, and XIANG Ling. A new wind turbine fault diagnosis method based on ensemble intrinsic time-scale decomposition and WPT-fractal dimension[J]. Renewable Energy, 2015, 83: 767–778. doi: 10.1016/j.renene.2015.04.063 HU Aijun, XIANG Ling, and GAO Nan. Fault diagnosis for the gearbox of wind turbine combining ensemble intrinsic time-scale decomposition with Wigner bi-spectrum entropy[J]. Journal of Vibroengineering, 2017, 19(3): 1759–1770. doi: 10.21595/jve.2017.17465 丛蕊, 高光甫, 樊瑞筱, 等. 基于灰度-梯度共生矩阵和模糊核聚类的振动图形识别方法[J]. 振动与冲击, 2012, 32(21): 73–76. doi: 10.3969/j.issn.1000-3835.2012.21.015CONG Rui, GAO Guangfu, FAN Ruixiao, et al. Vibration image recognition method based on gray-gradient CO-occurrence matrix and kernel·-based fuzzy clustering[J]. Journal of Vibration and Shock, 2012, 32(21): 73–76. doi: 10.3969/j.issn.1000-3835.2012.21.015 -






 下载:
下载:


 下载:
下载:
