非局部多尺度分数阶微分图像增强算法研究

黄果; 许黎; 陈庆利; 蒲亦非

doi:10.11999/JEIT190032

非局部多尺度分数阶微分图像增强算法研究

doi: 10.11999/JEIT190032

黄果¹,
许黎^{2, 3, ,},
陈庆利¹,
蒲亦非³

1.
乐山师范学院互联网自然语言智能处理四川省高等学校重点实验室乐山 614000
2.
乐山师范学院物理与电子工程学院乐山 614000
3.
四川大学计算机科学学院成都 610064

基金项目: 国家自然科学基金(61201438)，四川省科技厅应用基础项目(2016JY0238)，四川省教育厅重点项目(18ZA0235)，四川省教育厅一般项目(18ZB0268, 18ZB0266)，乐山师范学院科研项目(JG2018-1-04)

详细信息

作者简介:
黄果：男，1980年生，博士，副教授，主要研究领域为分数阶微积分理论、数字信号处理、模式识别

许黎：女，1982年生，博士生，讲师，研究领域为分数阶微积分理论、数字信号处理、分数阶忆阻

陈庆利：男，1975年生，博士，副教授，主要研究领域为分数阶微积分理论、数字信号处理、模式识别

蒲亦非：男，1975年生，博士，教授，主要研究领域为分数阶微积分理论、数字信号处理、模式识别、分数阶忆阻

通讯作者:
许黎　79017771@qq.com

中图分类号: TN391
计量
- 文章访问数: 2467
- HTML全文浏览量: 1207
- PDF下载量: 113
- 被引次数: 0
出版历程
- 收稿日期: 2019-01-15
- 修回日期: 2019-07-31
- 网络出版日期: 2019-08-30
- 刊出日期: 2019-12-01

Research on Non-local Multi-scale Fractional Differential Image Enhancement Algorithm

Guo HUANG¹,
Li XU^{2, 3
, ,},
Qingli CHEN¹,
Yifei Pu³

1.
Sichuan Province University Key Laboratory of Internet Natural Language Intelligent Processing, Le shan Normal University, Leshan 614000, China
2.
School of Physics and Electronics, Le shan Normal University, Leshan 614000, China
3.
School of Computer Science, Sichuan University, Chengdu 610064, China

Funds: The National Natural Science Foundation of China (61201438), The Sichuan Province Science and Technology Department Application Foundation Project (2016JY0238), The Sichuan Province Education Department Key Projects (18ZA0235), The Sichuan Province Education Department General Project (18ZB0268, 18ZB0266), The Research Fund of Leshan Normal University (JG2018-1-04)

摘要

摘要: 为了更好增强图像中的有用信息，改善图像视觉效果，该文提出了一种基于非局部多尺度分数阶微分图像增强算子(NMFD)。该算子首先将图像分成若干块子图像，计算每一块子图像的边缘强度系数、熵值和粗糙度等细节特征,将得到的特征数据在全局图像范围进行统一尺度的归一化，然后对这些归一化的数据进行加权求和作为图像的非局部特征值，最后利用指数函数建立图像细节特征和分数阶微分算子阶次之间的非线性量化关系，在不同的图像子块区域，确定不同尺度的分数阶微分阶次，实现图像的非局部多尺度增强。
- 图像增强 /
- 非局部多尺度分数阶微分算子 /
- 图像熵值 /
- 图像对比度
Abstract: In order to enhance the useful information in the image and improve the visual effect of the image, a Non-local Multi-scale Fractional Differential(NMFD) image enhancement operator is proposed. The operator divides the image into several sub-images and calculates the edge intensity coefficient, entropy value and roughness of each sub-image, and the obtained feature data are normalized in a unified scale in the global image range. Then, the normalized data are weighted to be the non-local eigenvalues of the image. Finally, an exponential function is used to establish the non-linear quantization relationship between image detail features and the value of fractional order. Thus, the fractional order of different scales can be determined in different image sub-block regions, so that the non-local multi-scale image enhancement model is realized.
- Image enhancement /
- Non-local Multi-scale Fractional Differential (NMFD) operator /
- Image entropy /
- Image contrast

HTML全文

图 1 2维信号不同阶次分数阶微分算子的幅频特性曲面

下载: 全尺寸图片幻灯片

图 2 窗口描述

下载: 全尺寸图片幻灯片

图 3 分数阶微分G-L定义下的掩模算子

下载: 全尺寸图片幻灯片

图 4 NMFD增强模型在不同窗口尺寸下的增强效果

下载: 全尺寸图片幻灯片

图 5 不同方法的增强模型增强Lena图像的效果对比

下载: 全尺寸图片幻灯片

图 6 不同方法的增强模型增强lena图像后的纹理特征对比

下载: 全尺寸图片幻灯片

表 1 NMFD增强模型在不同窗口尺寸下实验数据对比

窗口数量	平均梯度	边缘保持系数	对比度	熵
2×2	11.3139	1.7529	0.8152	7.5301
4×4	11.6058	1.8011	1.0725	7.5524
8×8	12.4049	2.0808	1.0893	7.5829
16×16	13.5831	2.4982	1.8659	7.5966
32×32	15.6209	2.9672	1.9856	7.5686

下载: 导出CSV

表 2 不同方法的图像增强模型增强lena图像的实验数据对比

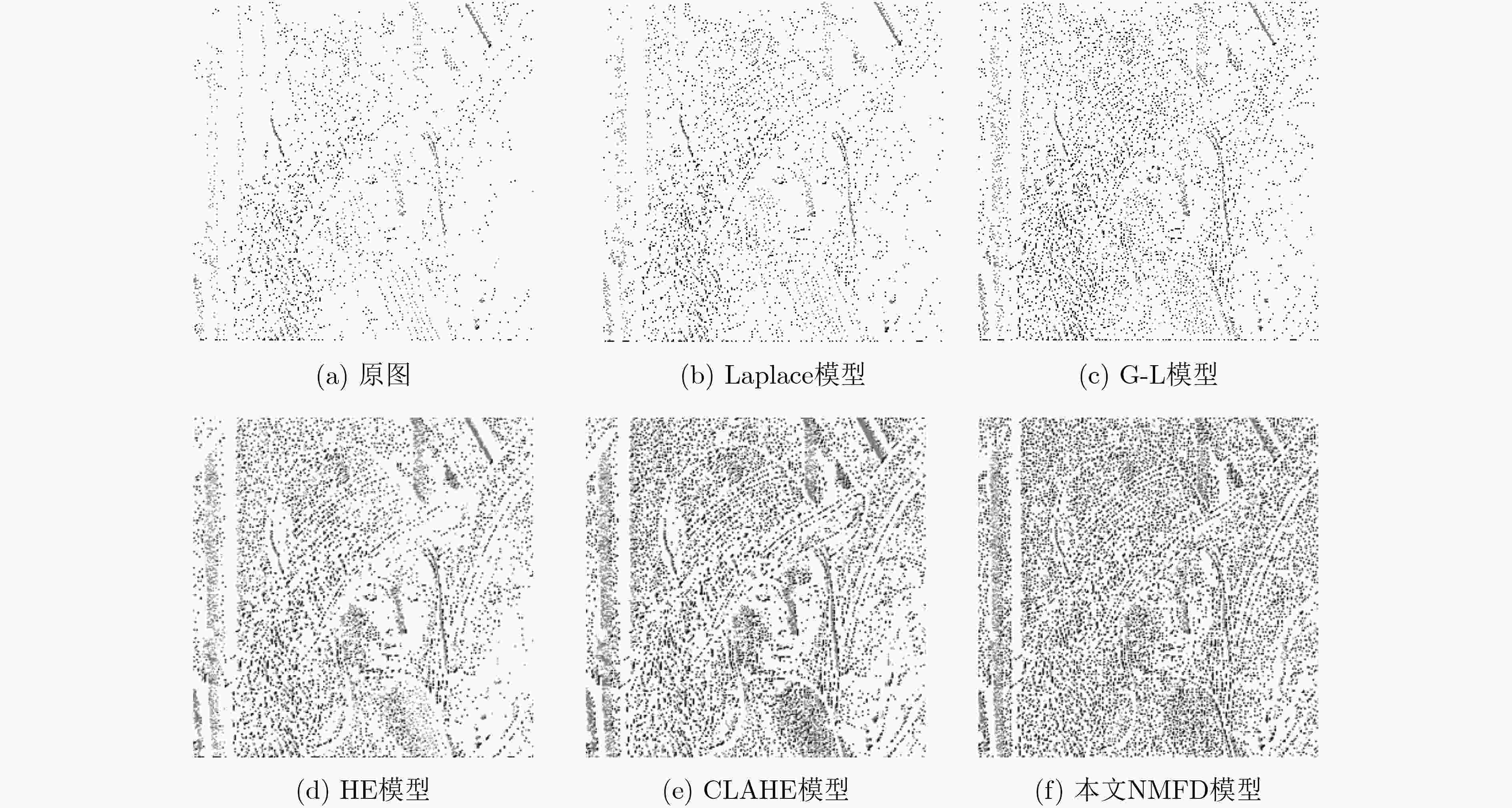
增强类型	平均梯度	边缘保持系数	对比度	熵
Laplace	10.9327	2.1957	1.3521	7.1963
G-L	10.8623	1.7908	0.9982	6.8723
HE	10.7522	1.4451	0.7538	5.9849
CLAHE	12.4368	1.8789	0.9823	7.3692
NMFD	14.2039	2.2321	1.3640	7.7404

下载: 导出CSV

参考文献(16)

MANDELBROT B B. The Fractal Geometry of Nature[M]. New York: W. H. Freeman and Company, 1983.

AZARANG A and GHASSEMIAN H. Application of fractional-order differentiation in multispectral image fusion[J]. Remote Sensing Letters, 2017, 9(1): 91–100. doi: 10.1080/2150704X.2017.1395963

LI Bo and XIE Wei. Adaptive fractional differential approach and its application to medical image enhancement[J]. Computers & Electrical Engineering, 2015, 45: 324–335. doi: 10.1016/j.compeleceng.2015.02.013

BAI Jian and FENG Xiangchu. Fractional-order anisotropic diffusion for image denoising[J]. IEEE Transactions on Image Processing, 2007, 16(10): 2492–2502. doi: 10.1109/TIP.2007.904971

HU Fuyuan, SI Shaohui, WONG H S, et al. An adaptive approach for texture enhancement based on a fractional differential operator with non-integer step and order[J]. Neurocomputing, 2015, 158: 295–306. doi: 10.1016/j.neucom.2014.10.013

HE Ning, WANG Jinbao, ZHANG Lulu, et al. An improved fractional-order differentiation model for image denoising[J]. Signal Processing, 2015, 112: 180–188. doi: 10.1016/j.sigpro.2014.08.025

YUAN Jianjun and LIU Lipei. Anisotropic diffusion model based on a new diffusion coefficient and fractional order differential for image denoising[J]. International Journal of Image and Graphics, 2016, 16(1): 1650003. doi: 10.1142/S0219467816500030

JALAB H A, IBRAHIM R W, and AHMED A. Image denoising algorithm based on the convolution of fractional Tsallis entropy with the Riesz fractional derivative[J]. Neural Computing and Applications, 2017, 28(S1): 217–223. doi: 10.1007/s00521-016-2331-7

PU Yifei, ZHOU Jiliu, and YUAN Xiao. Fractional differential mask: A fractional differential-based approach for multiscale texture enhancement[J]. IEEE Transactions on Image Processing, 2010, 19(2): 491–511. doi: 10.1109/TIP.2009.2035980

PU Yifei, SIARRY P, CHATTERJEE A, et al. A fractional-order variational framework for retinex: Fractional-order partial differential equation-based formulation for multi-scale nonlocal contrast enhancement with texture preserving[J]. IEEE Transactions on Image Processing, 2018, 27(3): 1214–1229. doi: 10.1109/TIP.2017.2779601

牛为华, 孟建良, 崔克彬, 等. 利用Grümwald-Letnikov分数阶方向导数的图像增强方法[J]. 计算机辅助设计与图形学学报, 2016, 28(1): 129–137. doi: 10.3969/j.issn.1003-9775.2016.01.016

NIU Weihua, MENG Jianliang, CUI Kebin, et al. Image enhancement method using grümwald -letnikov fractional directional differential[J]. Journal of Computer-Aided Design &Computer Graphics, 2016, 28(1): 129–137. doi: 10.3969/j.issn.1003-9775.2016.01.016

GAO Caobang, ZHOU Jiliu, HU Jingrong, et al. Edge detection of colour image based on quaternion fractional differential[J]. IET Image Processing, 2011, 5(3): 261–272. doi: 10.1049/iet-ipr.2009.0409

李博, 谢巍. 基于自适应分数阶微积分的图像去噪与增强算法[J]. 系统工程与电子技术, 2016, 38(1): 185–192. doi: 10.3969/j.issn.1001-506X.2016.01.29

LI Bo and XIE Wei. Image enhancement and denoising algorithms based on adaptive fractional differential and integral[J]. Systems Engineering and Electronics, 2016, 38(1): 185–192. doi: 10.3969/j.issn.1001-506X.2016.01.29

陈庆利, 黄果, 门涛, 等. 数字图像的局部分数阶微分增强[J]. 四川大学学报: 工程科学版, 2016, 48(4): 115–122. doi: 10.15961/j.jsuese.2016.04.016

CHEN Qingli, HUANG Guo, MEN Tao, et al. Local fractional differential algorithm for image enhancement[J]. Journal of Sichuan University:Engineering Science Edition, 2016, 48(4): 115–122. doi: 10.15961/j.jsuese.2016.04.016

姒绍辉, 胡伏原, 付保川, 等. 自适应非整数步长的分数阶微分掩模的图像纹理增强算法[J]. 计算机辅助设计与图形学学报, 2014, 26(9): 1438–1449.

SI Shaohui, HU Fuyuan, FU Baochuan, et al. An algorithm for texture enhancement based on fractional differential mask using adaptive non-integer step[J]. Journal of Computer-Aided Design &Computer Graphics, 2014, 26(9): 1438–1449.

ZHAO Mengdan, GAO Xuzhen, PAN Yue, et al. Image encryption based on fractal-structured phase mask in fractional Fourier transform domain[J]. Journal of Optics, 2018, 20(4): 045703. doi: 10.1088/2040-8986/aab247

施引文献

资源附件(0)

访问统计