Doppler Frequency Estimation Method Based on Chinese Remainder Theorem with Spectrum Correction
-
摘要: 脉冲多普勒(PD)雷达能够检测目标多普勒频率和有效抑制杂波,该优势使得PD雷达得到了广泛应用。但速度模糊的存在,往往对PD目标检测带来困难。该文紧密结合PD雷达体制的特点,在基于PD雷达参差重频模式下,提出一种基于全相位离散傅里叶变换(DFT)相位差频谱校正的最优余数封闭式鲁棒中国余数定理(CFRCRT)的多普勒频率估计算法。理论分析和仿真实验表明该文算法在测量精度和实时性能上可以满足工程上应用的需求。Abstract: It makes the Pulse Doppler (PD) radar widely applied that the PD radar has the obvious advantages of detecting the Doppler frequency of the target and suppressing the clutter effectively. However, it is difficult for the PD radar to detect the target due to velocity ambiguity. Combining with the characteristic and stagger-period model of the PD radar, a Doppler frequency estimation method based on all phase DFT Closed-Form Robust Chinese Remainder Theorem (CFRCRT) with spectrum correction is proposed. Both theoretical analysis and simulation experiment demonstrate that the proposed method can satisfy the engineering demand in measure accuracy and real-time performance.
-
表 1 基于谱校正的中国余数定理的方案测量结果(Hz)
$F$ ${\hat f_{{\rm{r}}1}}$ 余数理论值 ${\hat f_{{\rm{r}}2}}$ 余数理论值 ${\hat f_{{\rm{r}}3}}$ 余数理论值 $\Delta F\;$ ${\rm{5}}{\rm{.5122}} \times {\rm{1}}{{\rm{0}}^{\rm{3}}}$ ${\rm{512}}{\rm{.60}}$ $512$ ${\rm{5512}}{\rm{.65}}$ $5512$ ${\rm{5512}}{\rm{.90}}$ $5512$ ${\rm{1}}.57 \times {\rm{1}}{{\rm{0}}^{{\rm{ - 1}}}}$ $512.58$ $5512.57$ $5512.58$ $1.66 \times {10^{ - 1}}$ $515.04$ $5516.01$ $5516.97$ $3.41 \times {10^0}$ ${\rm{3}}{\rm{.1157}} \times {\rm{1}}{{\rm{0}}^{\rm{5}}}$ $1569.27$ $1570$ $3569.69$ $3570$ $5569.80$ $5570$ $2.03 \times {10^{ - 2}}$ $1568.72$ $3568.70$ $5568.95$ $4.99 \times {10^{ - 2}}$ $1574.25$ $3566.42$ $5574.39$ $8.42 \times {10^{ - 1}}$ -
CHUANG Tingwei, CHEN Chaur-Chin, and CHIEN Betty. Image sharing and recovering based on Chinese remainder theorem[C]. International Symposium on Computer, Consumer and Control, Xi’an, China, 2016: 817–820. XIAO Hanshen, HUANG Yufeng, YE Yu, et al. Robustness in Chinese remainder theorem for multiple numbers and remainder coding[J]. IEEE Transactions on Signal Processing, 2018, 66(16): 4347–4361. doi: 10.1109/TSP.2018.2846228 LU Dianjun, WANG Yu, ZHANG Xiaoqin, et al. A threshold secret sharing scheme based on LMCA and Chinese remainder theorem[C]. The 9th International Symposium on Computational Intelligence and Design, Hangzhou, China, 2016: 439–442. CHEN Jinrui, LIU Kesheng, YAN Xuehu, et al. An information hiding scheme based on Chinese remainder theorem[C]. The 3rd IEEE International Conference on Image, Vision and Computing, Chongqing, China, 2018: 785–790. LIN E and MONTE L. Joint frequency and angle of arrival estimation using the Chinese remainder theorem[C]. 2017 IEEE Radar Conference, Seattle, USA, 2017: 1547–1551. JIANG Zhibiao, WANG Jian, SONG Qian, et al. A closed-form robust Chinese remainder theorem based Multibaseline phase unwrapping[C]. 2017 International Conference on Circuits, Devices and Systems, Chengdu, China, 2017: 115–119. JIANG Zhibiao, WANG Jian, SONG Qian, et al. Multibaseline phase unwrapping through robust Chinese remainder theorem[C]. The 7th IEEE International Symposium on Microwave, Antenna, Propagation, and EMC Technologies, Xi’an, China, 2017: 462–466. SILVA Band FRAIDENRAICH G. Performance analysis of the classic and robust Chinese remainder theorems in pulsed Doppler radars[J]. IEEE Transactions on Signal Processing, 2018, 66(18): 4898–4903. doi: 10.1109/TSP.2018.2863667 LI Xiaoping, WANG Wenjie, YANG Bin, et al. Distance estimation based on phase detection with robust Chinese remainder theorem[C]. 2014 IEEE International Conference on Acoustics, Speech and Signal Processing, Florence, Italy, 2014: 4204–4208. WANG Qian, YAN Xiao, and QIN Kaiyu. Parameters estimation algorithm for the exponential signal by the interpolated all-phase DFT Approach[C]. The 11th International Computer Conference on Wavelet Active Media Technology and Information Processing, Chengdu, China, 2014: 37–41. 王文杰, 李小平. 鲁棒的闭式中国余数定理及其在欠采样频率估计中的应用[J]. 信号处理, 2013, 29(9): 1206–1211. doi: 10.3969/j.issn.1003-0530.2013.09.017WANG Wenjie and LI Xiaoping. The closed-form robust Chinese remainder theorem and its application in frequency estimation with Undersampling[J]. Journal of Signal Processing, 2013, 29(9): 1206–1211. doi: 10.3969/j.issn.1003-0530.2013.09.017 CANDAN Ç. A method for fine resolution frequency estimation from three DFT samples[J]. IEEE Signal Processing Letters, 2011, 18(6): 351–354. doi: 10.1109/LSP.2011.2136378 CANDAN Ç. Analysis and further improvement of fine resolution frequency estimation method from three DFT samples[J]. IEEE Signal Processing Letters, 2013, 20(9): 913–916. doi: 10.1109/LSP.2013.2273616 ABOUTANIOS E and MULGREW B. Iterative frequency estimation by interpolation on Fourier coefficients[J]. IEEE Transactions on Signal Processing, 2005, 53(4): 1237–1242. doi: 10.1109/TSP.2005.843719 BELEGA D, PETRI D, and DALLET D. Iterative sine-wave frequency estimation by generalized Fourier interpolation algorithms[C]. The 11th International Symposium on Electronics and Telecommunications, Timisoara, Romania, 2014: 1–4. GAO Yue, ZHANG Xiong, and SONG Jun. Modified algorithm of sinusoid signal frequency estimation based on Quinn and Aboutanios iterative algorithms[C]. The 13th International Conference on Signal Processing, Chengdu, China, 2016: 232–235. LU Xinning and ZHANG Yonghui. Phase detection algorithm and precision analysis based on all phase FFT[C]. The International Conference on Automatic Control and Artificial Intelligence, Xiamen, China, 2012: 1564–1567. LI Xiaowei, LIANG Hong, and XIA Xianggen. A robust Chinese remainder theorem with its applications in frequency estimation from undersampled waveforms[J]. IEEE Transactions on Signal Processing, 2009, 57(11): 4314–4322. doi: 10.1109/TSP.2009.2025079 WANG Wei, LI Xiaoping, XIA Xianggen, et al. The largest dynamic range of a generalized Chinese remainder theorem for two integers[J]. IEEE Signal Processing Letters, 2015, 22(2): 254–258. doi: 10.1109/LSP.2014.2322200 XIAO Li, XIA Xianggen. A generalized Chinese remainder theorem for two integers[J]. IEEE Signal Processing Letters, 2014, 21(1): 55–59. doi: 10.1109/LSP.2013.2289326 -






 下载:
下载:
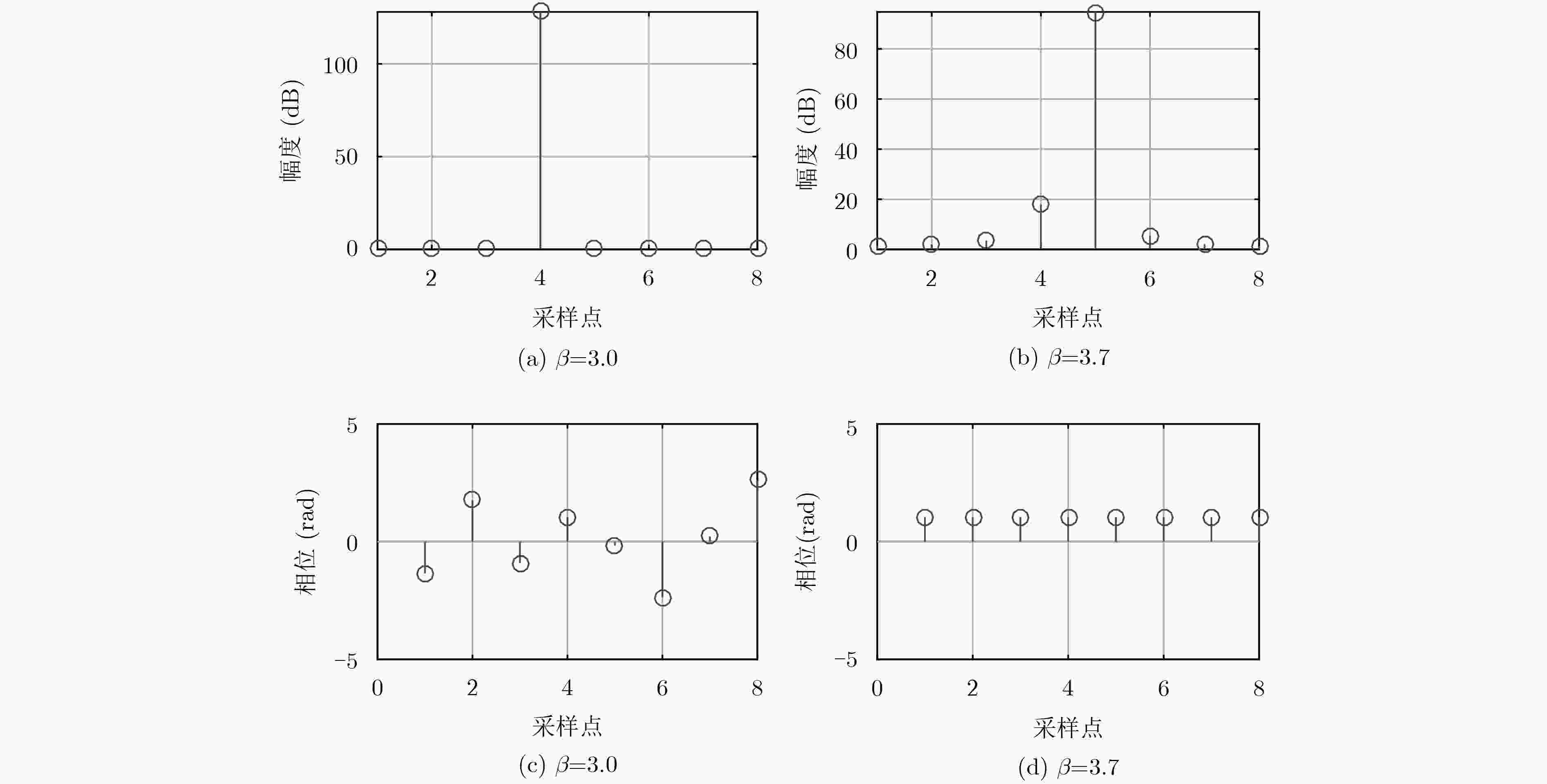
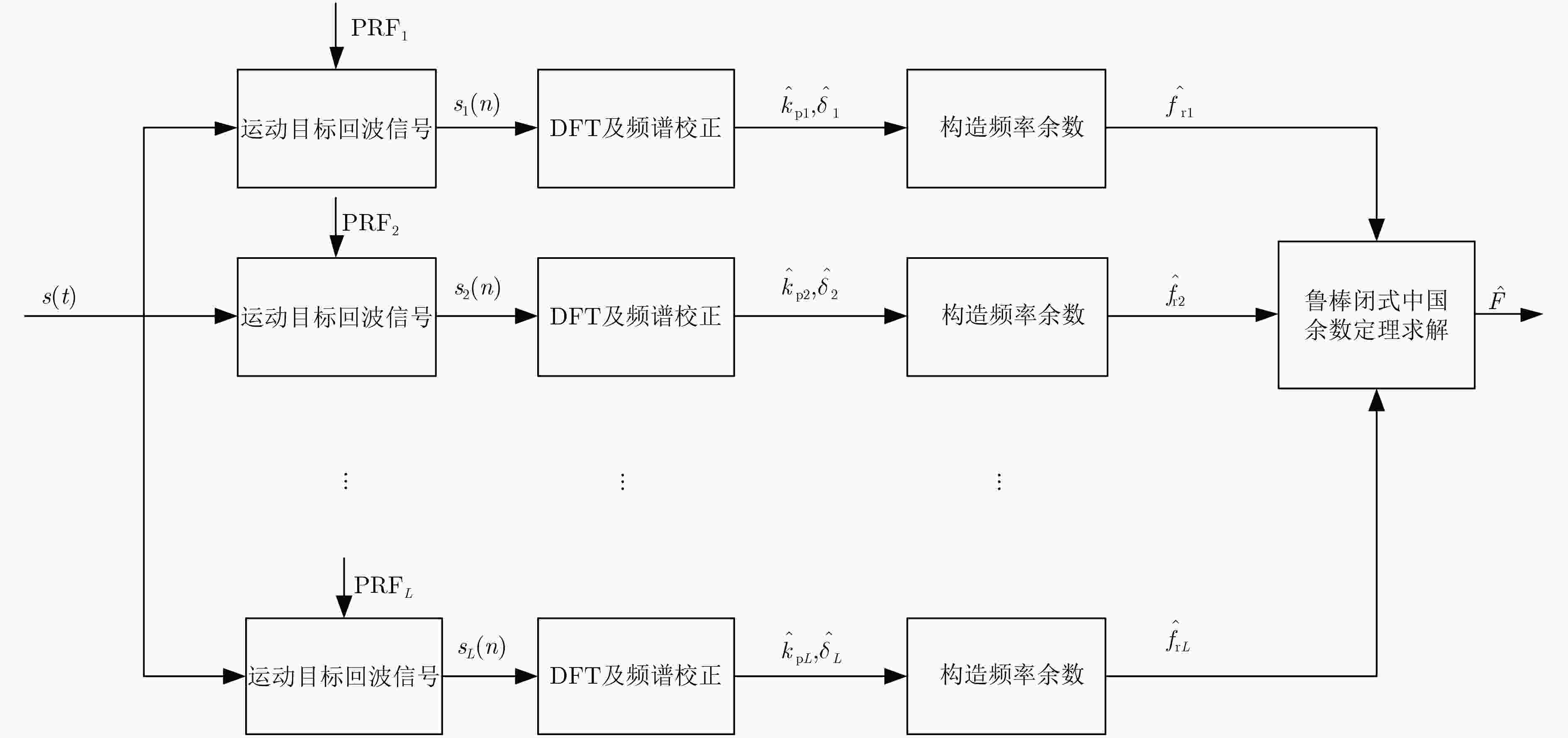
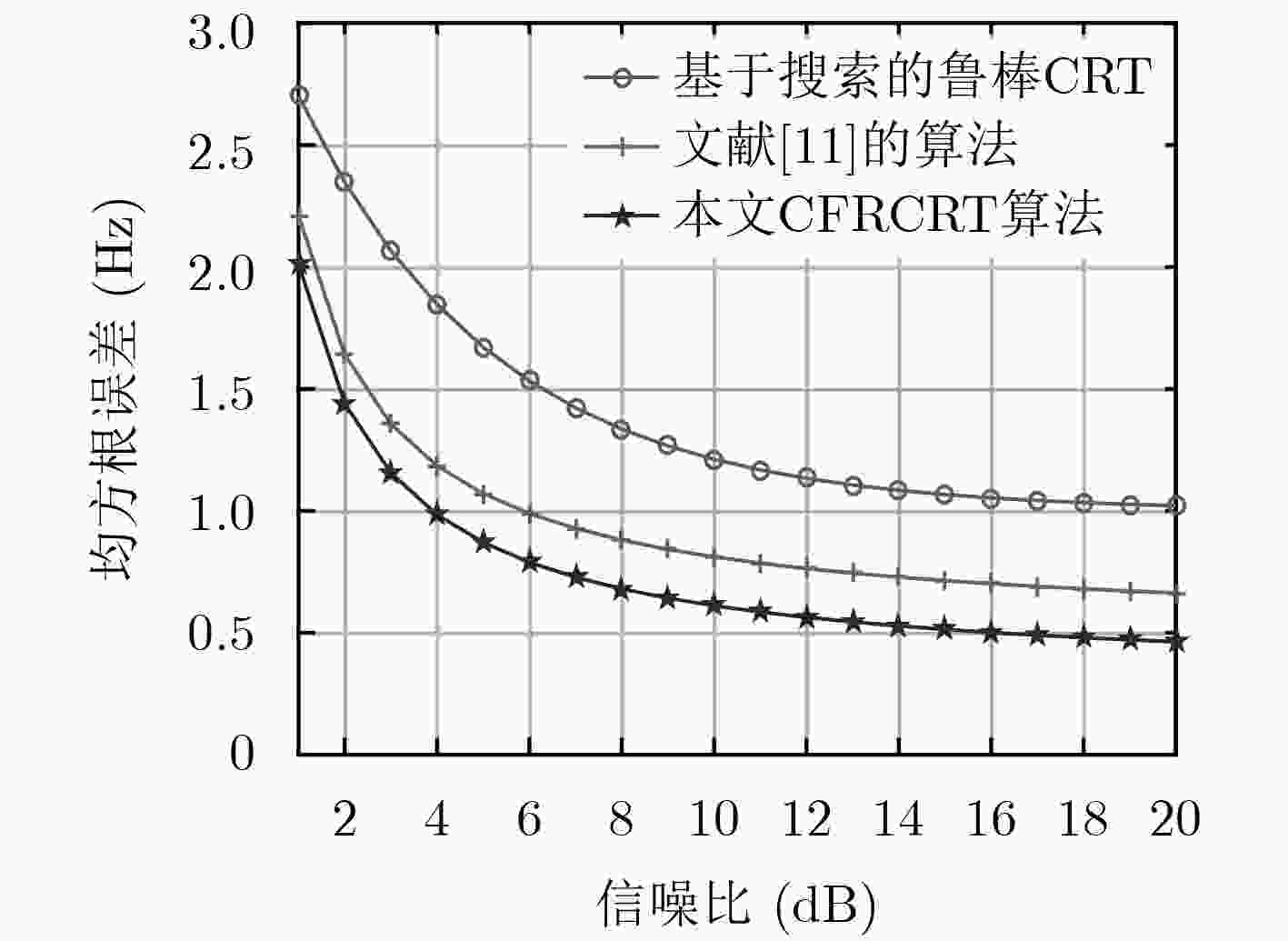

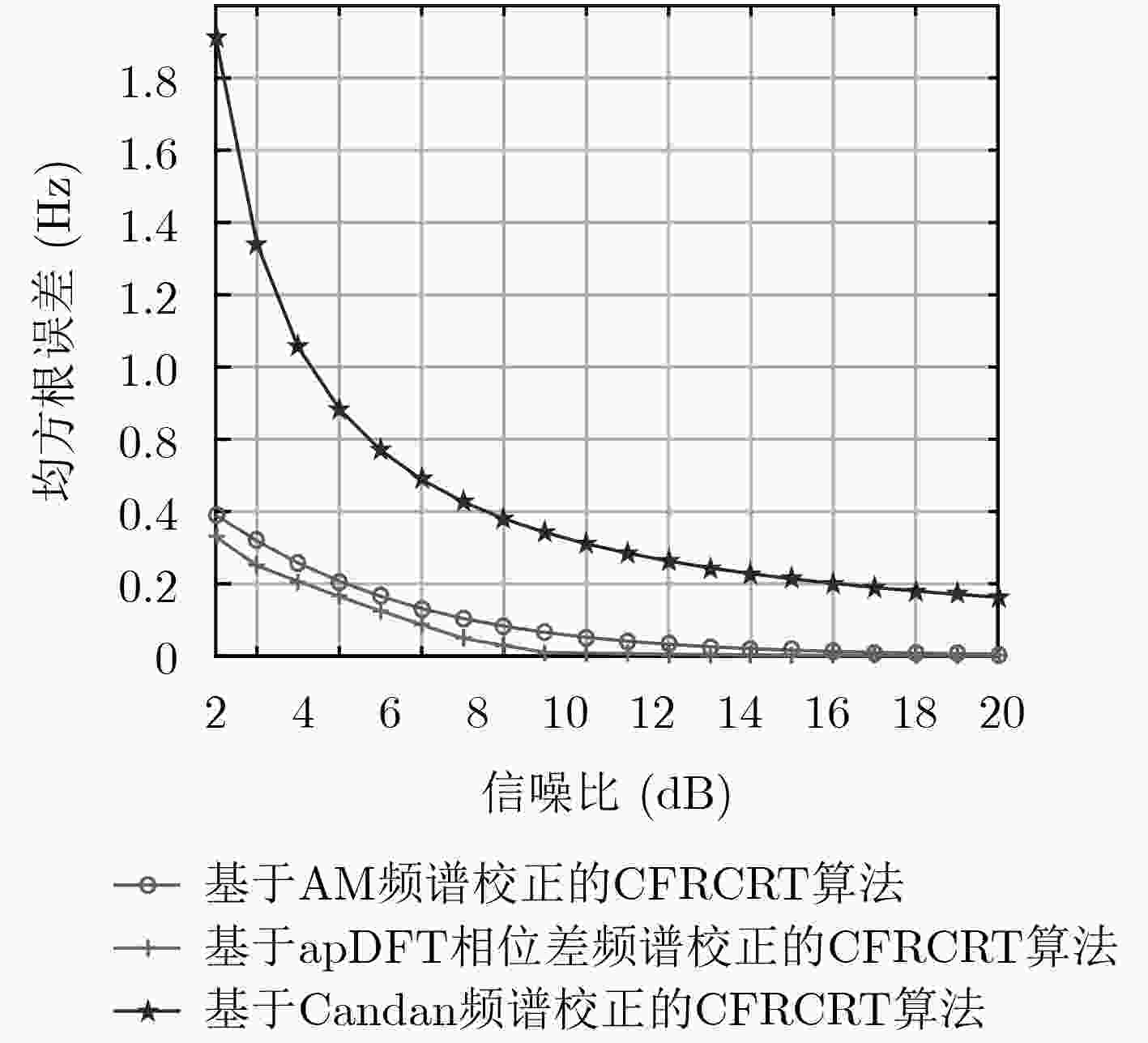



 下载:
下载:
