一种基于短合成孔径的双星干涉精确定位方法

孙光才; 王裕旗; 高昭昭; 江帆; 邢孟道; 保铮

doi:10.11999/JEIT180940

一种基于短合成孔径的双星干涉精确定位方法

doi: 10.11999/JEIT180940

1.
西安电子科技大学雷达信号处理国家重点实验室西安 710071
2.
电子信息控制重点实验室成都 610036

基金项目: 电子信息控制重点实验室基金(20170105)

详细信息

作者简介:
孙光才：男，1984年生，博士，副教授，研究方向为合成孔径雷达成像和动目标检测

王裕旗：男，1994年生，博士生，研究方向为合成孔径雷达成像

高昭昭：男，1982年生，博士，高级工程师，研究方向为雷达信号处理和毫米波无源成像探测

江帆：女，1988年生，博士，高级工程师，研究方向为阵列信号处理与微波光子

邢孟道：男，1975年生，博士，教授，研究方向为雷达成像和目标检测与识别

保铮：男，1927年生，中国科学院院士，研究方向为雷达系统与雷达信号处理、动目标检测、阵列信号处理、空时自适应信号处理等

通讯作者:
孙光才　rsandsgc@126.com

中图分类号: TN958
计量
- 文章访问数: 2748
- HTML全文浏览量: 868
- PDF下载量: 95
- 被引次数: 0
出版历程
- 收稿日期: 2018-10-09
- 修回日期: 2019-07-18
- 网络出版日期: 2019-09-17
- 刊出日期: 2020-02-19

A Dual Satellite Interferometric Precise Localization Method Based on Short Synthetic Aperture

1.
National Laboratary of Radar Signal Processing, Xidian University, Xi’an 710071, China
2.
Science and Technology on Electronic Information Control Laboratory, Chengdu 610036, China

Funds: The Foundation of Science and Technology on Electronic Information Control Laboratory (20170105)

摘要

摘要:
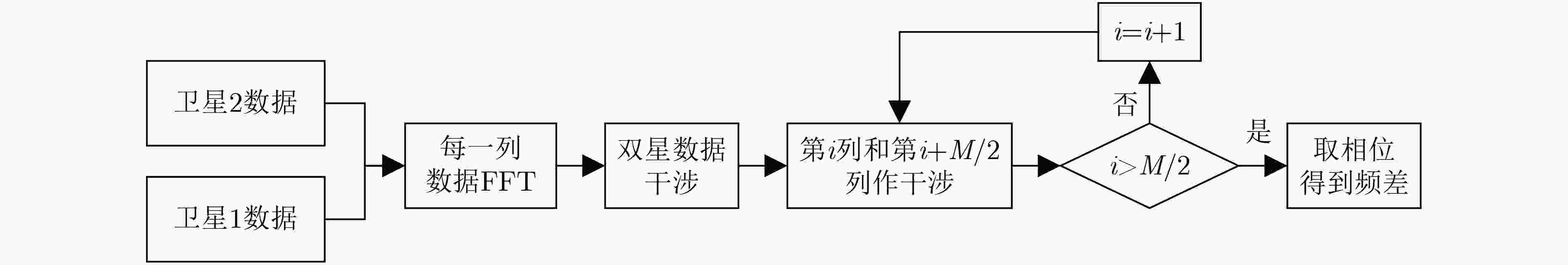
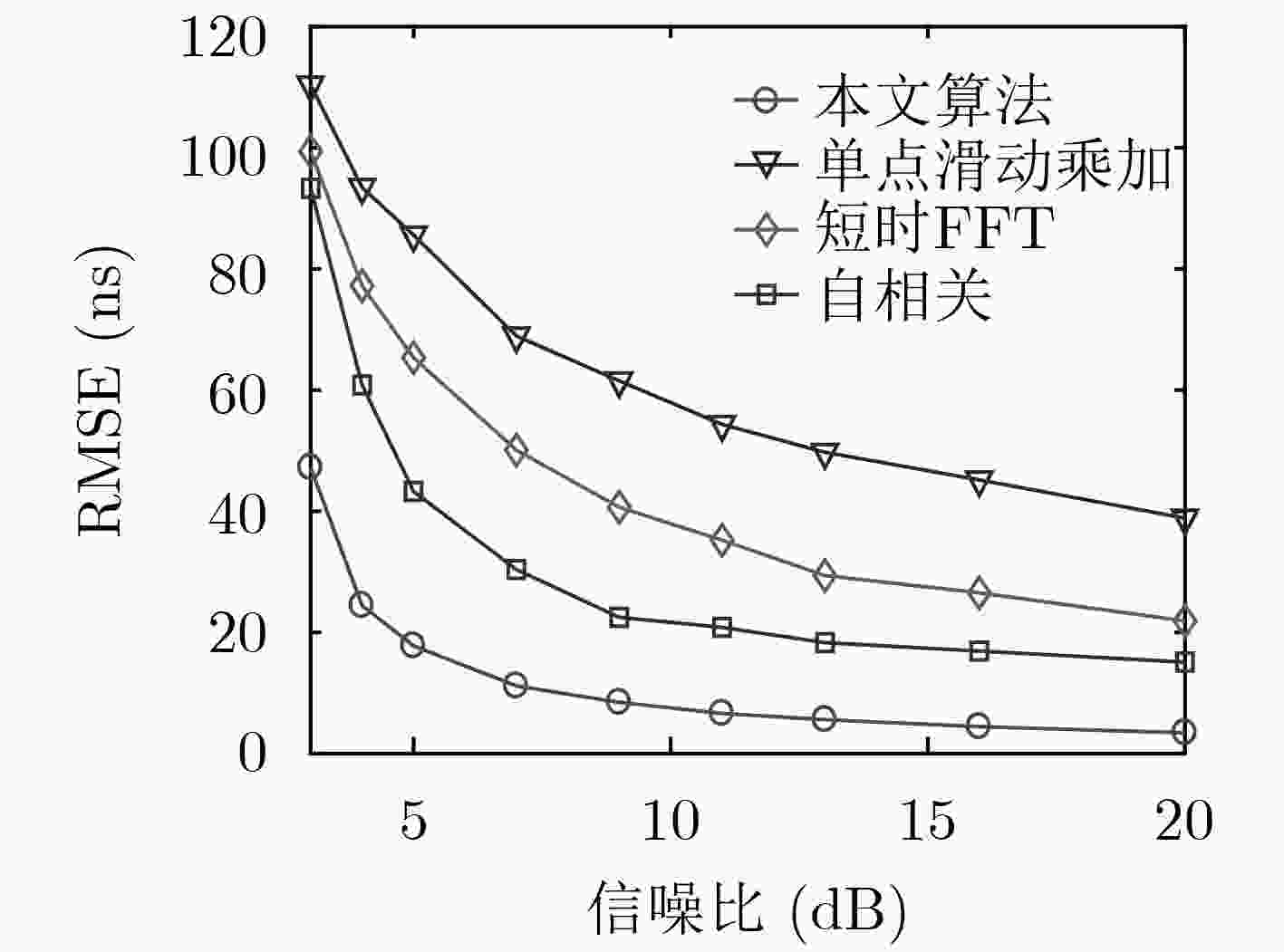
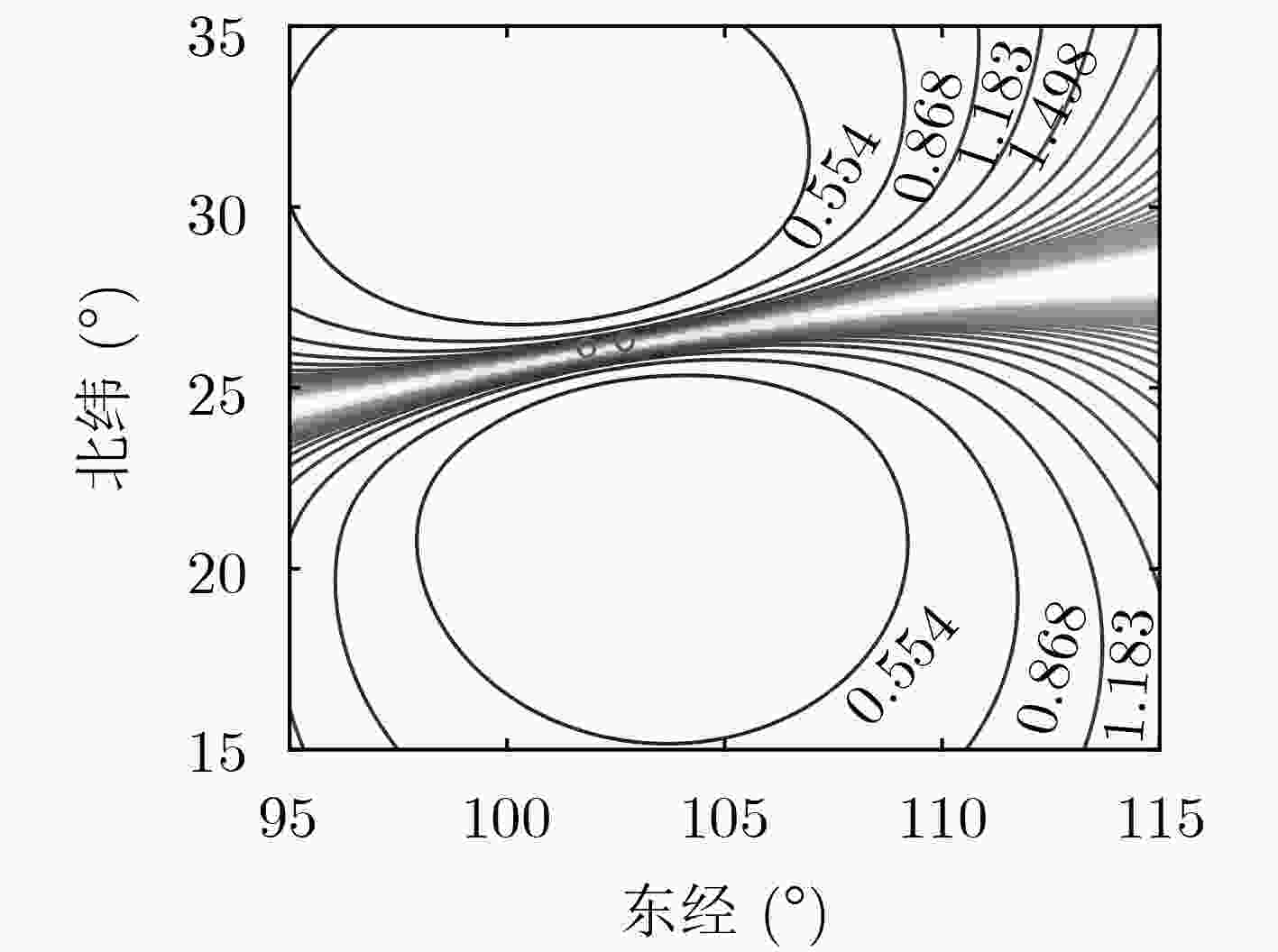
双星TDOA/FDOA联合定位通过时差曲面和频差曲面进行定位，定位的精度受时差/频差测量精度的影响。针对精确测量时差/频差的需求，该文提出一种基于短合成孔径的双星干涉测量时差/频差的方法，利用一定长度的合成孔径提高测量精度。对于窄带信号，该方法有估计单星多普勒频率的能力，通过两颗卫星单独估计的结果得到频差；对于宽带信号，通过双星数据干涉可以获得频差的高精度估计。对于短期稳定的雷达信号，STK仿真数据的处理结果证实了该方法在大范围内可以实现1 km的定位精度。
- 双星定位 /
- 短合成孔径 /
- 双星干涉 /
- 到达时差 /
- 到达频差
Abstract:
Dual satellite TDOA/FDOA localization is achieved by the TDOA hyperboloid and FDOA hyperboloid. The accuracy of localization is affected by TDOA/FDOA accuracy. In order to measure accurately the TDOA/FDOA, a method of TDOA/FDOA measurement based on short synthetic aperture is presented. This method improves the measurement accuracy by using a certain length of synthetic aperture. For narrowband signals, the method has the ability to estimate a single satellite Doppler frequency, and the frequency difference can be obtained from the results estimated by the two satellites. For wideband signals, high-precision estimates of frequency differences can be obtained by dual satellite data interference. For short-term stable radar signals, the processing results of STK simulation data confirm the effectiveness of the proposed method.
- Dual-satellite localization /
- Short synthetic aperture /
- Dual-satellite interferometry /
- TDOA /
- FDOA

HTML全文

图 1 双星信号模型

下载: 全尺寸图片幻灯片

图 2 频差测量算法流程

下载: 全尺寸图片幻灯片

图 3 双星定位模型

下载: 全尺寸图片幻灯片

图 4 有噪声的情况下干涉的相位图

下载: 全尺寸图片幻灯片

图 5 某个脉冲距离向剖面图

下载: 全尺寸图片幻灯片

图 6 LFM信号频差均方误差值随信噪比变化

下载: 全尺寸图片幻灯片

图 7 单频信号频差均方误差值随信噪比变化

下载: 全尺寸图片幻灯片

图 8 时差均值随信噪比的变化

下载: 全尺寸图片幻灯片

图 9 双星时差频差定位的GDOP (km)

下载: 全尺寸图片幻灯片

表 1 STK仿真数据处理结果

载频(GHz)	脉宽(μs)	脉冲重复周期(μs)	带宽(MHz)	基频测量结果(Hz)	基频测量误差(Hz)	相对误差(Hz)
1.59	10	100	10	–4781.5	33.9742	2.16
1.59	10	100	10	1710.5	31.8189	2.16
1.78	10	100	10	–1120.3	40.6852	4.20
1.78	10	100	10	2486.2	36.4891	4.20

下载: 导出CSV

表 2 时差频差定位结果

载频(GHz)	脉宽(μs)	脉冲重复周期(μs)	带宽(MHz)	目标真实位置(m)	目标测量位置(m)	相对误差(m)
1.59	10	100	10	(6303534, –92215, 968286)	(6303532, –92876, 968234)	662
1.59	10	100	10	(6348796, –27959, 610439)	(6348797, –27448, 610446)	510
1.78	10	100	10	(6339522, –16218, 700588)	(6339520, –16829, 700590)	611
1.78	10	100	10	(6374947, 30978, 199294)	(6374945, 30530, 199409)	462

下载: 导出CSV

表 3 常规方法定位结果

目标真实位置(m)	目标测量位置(m)	相对误差(m)
(6303534, –92215, 968286)	(6303808, –91936, 970069)	1825
(6348796, –27959, 610439)	(6348976, –28173, 612353)	1935
(6339522, –16218, 700588)	(6339615, –16496, 701470)	930
(6374947, 30978, 199294)	(6374905, 30788, 198028)	1280

下载: 导出CSV

参考文献(23)

陈涛, 王梦馨, 黄湘松. 基于樽海鞘群算法的无源时差定位[J]. 电子与信息学报, 2018, 40(7): 1591–1597. doi: 10.11999/JEIT170979

CHEN Tao, WANG Mengxin, and HUANG Xiangsong. Time difference of arrival passive location based on salp swarm algorithm[J]. Journal of Electronics &Information Technology, 2018, 40(7): 1591–1597. doi: 10.11999/JEIT170979

BAMLER R and JUST D. Phase statistics and decorrelation in SAR interferograms[C]. The IGARSS '93- IEEE International Geoscience and Remote Sensing Symposium, Tokyo, Japan, 1993: 980–984. doi: 10.1109/IGARSS.1993.322637.

KIM Y H, KIM D G, HAN J W, et al. Analysis of sensor-emitter geometry for emitter localisation using TDOA and FDOA measurements[J]. IET Radar, Sonar & Navigation, 2017, 11(2): 341–349. doi: 10.1049/iet-rsn.2016.0314

张磊, 陈艳玲, 陈俊平. SAR回波信号及InSAR干涉相位的统计特性研究[J]. 天文学进展, 2007, 25(2): 169–176. doi: 10.3969/j.issn.1000-8349.2007.02.006

ZHANG Lei, CHEN Yanling, and CHEN Junping. The statistic characteristics of SAR signals and interferometric phases[J]. Progress in Astronomy, 2007, 25(2): 169–176. doi: 10.3969/j.issn.1000-8349.2007.02.006

CAO H, CHAN Y T, and SO H C. Maximum likelihood TDOA estimation from compressed sensing samples without reconstruction[J]. IEEE Signal Processing Letters, 2017, 24(5): 564–568. doi: 10.1109/LSP.2017.2684185

PEPE A and MASTRO P. On the use of directional statistics for the adaptive spatial multi-looking of sequences of differential SAR interferograms[C]. 2017 IEEE International Geoscience and Remote Sensing Symposium, Fort Worth, USA, 2017: 3791–3794. doi: 10.1109/IGARSS.2017.8127825.

XU Huaping, CHEN Wei, LIU Wei, et al. Phase statistics for strong scatterers in SAR interferograms[J]. IEEE Geoscience and Remote Sensing Letters, 2014, 11(11): 1966–1970. doi: 10.1109/LGRS.2014.2315611

NOROOZI A, OVEIS A H, HOSSEINI S M R, et al. Improved algebraic solution for source localization from TDOA and FDOA measurements[J]. IEEE Wireless Communications Letters, 2018, 7(3): 352–355. doi: 10.1109/LWC.2017.2777995

QU Xiaomei, XIE Lihua, and TAN Wenrong. Iterative constrained weighted least squares source localization using TDOA and FDOA measurements[J]. IEEE Transactions on Signal Processing, 2017, 65(15): 3990–4003. doi: 10.1109/TSP.2017.2703667

冯明月, 何明浩, 徐璟, 等. 低信噪比条件下宽带欠定信号高精度DOA估计[J]. 电子与信息学报, 2017, 39(6): 1340–1347. doi: 10.11999/JEIT160921

FENG Mingyue, HE Minghao, XU Jing, et al. High accuracy DOA estimation under low SNR condition for wideband underdetermined signals[J]. Journal of Electronics &Information Technology, 2017, 39(6): 1340–1347. doi: 10.11999/JEIT160921

刘倩, 夏斌, 彭荣群, 等. 基于包络提取的TOA估计算法[J]. 山东大学学报: 工学版, 2012, 42(5): 24–29. doi: 10.6040/j.issn.1672-3961.2012.05.005

LIU Qian, XIA Bin, PENG Rongqun, et al. A TOA estimation algorithm based on envelope extraction[J]. Journal of Shandong University:Engineering Science, 2012, 42(5): 24–29. doi: 10.6040/j.issn.1672-3961.2012.05.005

王方秋, 张小飞, 汪飞. IR-UWB系统中基于root-MUSIC算法的TOA和DOA联合估计[J]. 通信学报, 2014, 35(2): 137–145. doi: 10.3969/j.issn.1000-436x.2014.02.018

WANG Fangqiu, ZHANG Xiaofei, and WANG Fei. Root-MUSIC-based joint TOA and DOA estimation in IR-UWB[J]. Journal on Communications, 2014, 35(2): 137–145. doi: 10.3969/j.issn.1000-436x.2014.02.018

刘德树. 空间谱估计及其应用[M]. 合肥: 中国科学技术大学出版社, 1997.

孙超, 王世练, 朱江. 基于自相关算法的TOA估计方法研究[J]. 微处理机, 2014, 35(4): 39–43. doi: 10.3969/j.issn.1002-2279.2014.04.013

SUN Chao, WANG Shilian, and ZHU Jiang. Research of TOA estimation based on auto -correlation algorithm[J]. Microprocessors, 2014, 35(4): 39–43. doi: 10.3969/j.issn.1002-2279.2014.04.013

杨小凤, 陈铁军, 刘峰. 基于TOA-DOA联合估计的无线定位新方法[J]. 数据采集与处理, 2014, 29(6): 1036–1040. doi: 10.16337/j.1004-9037.2014.06.024

YANG Xiaofeng, CHEN Tiejun, and LIU Feng. Novel wireless positioning method based on joint TOA-DOA estimation[J]. Journal of Data Acquisition and Processing, 2014, 29(6): 1036–1040. doi: 10.16337/j.1004-9037.2014.06.024

YEREDOR A and ANGEL E. Joint TDOA and FDOA estimation: A conditional bound and its use for optimally weighted localization[J]. IEEE Transactions on Signal Processing, 2011, 59(4): 1612–1623. doi: 10.1109/TSP.2010.2103069

张桀, 沈重. 联合TDOA改进算法和卡尔曼滤波的UWB室内定位研究[J]. 现代电子技术, 2016, 39(13): 1–5. doi: 10.16652/j.issn.1004-373x.2016.13.001

ZHANG Jie and SHEN Chong. Research on UWB indoor positioning in combination with TDOA improved algorithm and Kalman filtering[J]. Modern Electronics Technique, 2016, 39(13): 1–5. doi: 10.16652/j.issn.1004-373x.2016.13.001

林雪原, 何友, 史佩. 利用二星TDOA和FDOA测量及辅助高度信息对地面目标的定位算法及精度分析[J]. 空间科学学报, 2006, 26(4): 277–281. doi: 10.3969/j.issn.0254-6124.2006.04.006

LIN Xueyuan, HE You, and SHI Pei. Location algorithm and error analysis for earth object using TDOA, FDOA by dual-satellite and aided height information[J]. Chinese Journal of Space Science, 2006, 26(4): 277–281. doi: 10.3969/j.issn.0254-6124.2006.04.006

卢鑫. 无源定位多普勒频差测量方法研究[C]. 江苏省电子学会2010年学术年会论文集, 南京, 2010: 96-99.

LU Xin. Research on accurate FDOA measurement of radar coherent pulses in passive location[C]. 2010 Annual Conference of Jiangsu Institute of Electronics, Nanjing, China, 2010: 96-99.

STEIN S. Algorithms for ambiguity function processing[J]. IEEE Transactions on Acoustics, Speech, and Signal Processing, 1981, 29(3): 588–599. doi: 10.1109/TASSP.1981.1163621

吕宏春, 卢晓春, 武建锋. 星间链路体制下的星地双向时间同步方法[J]. 宇航学报, 2017, 38(7): 728–734. doi: 10.3873/j.issn.1000-1328.2017.07.008

LV Hongchun, LU Xiaochun, WU Jianfeng. A method of two-way satellite-ground time synchronization under inter-satellite links system[J]. Journal of Astronautics, 2017, 38(7): 728–734. doi: 10.3873/j.issn.1000-1328.2017.07.008

郭福成, 樊昀. 双星时差频差联合定位方法及其误差分析[J]. 宇航学报, 2008, 29(4): 1381–1386. doi: 10.3873/j.issn.1000-1328.2008.04.053

GUO Fucheng and FAN Yun. A method of dual-satellites geolocation using TDOA and FDOA and its precision analysis[J]. Journal of Astronautics, 2008, 29(4): 1381–1386. doi: 10.3873/j.issn.1000-1328.2008.04.053

马淑歌. 脉冲信号TOA估计方法研究[D]. [硕士论文], 哈尔滨工业大学, 2017.

MA Shuge. Research on pulse signal TOA estimation method[D]. [Master dissertation], Harbin Institute of Technology, 2017.

施引文献

资源附件(0)

访问统计