基于两步方位重采样的中轨SAR聚焦方法

刘文康; 景国彬; 孙光才; 陈权; 邢孟道

doi:10.11999/JEIT180238

基于两步方位重采样的中轨SAR聚焦方法

doi: 10.11999/JEIT180238

刘文康^{1, 2, ,},
景国彬³,
孙光才^{1, 2},
陈权^{1, 2},
邢孟道^{1, 2}

1.
西安电子科技大学雷达信号处理国家重点实验室西安 710071
2.
西安电子科技大学信息感知技术协同创新中心西安 710071
3.
上海卫星工程研究所毫米波遥感技术实验室上海 201109

基金项目: 国家重点研发计划(2017YFC1405600)，国家自然科学基金创新群体基金(61621005)，中央高校基本业务费(JB180213)

详细信息

作者简介:
刘文康：男，1994年生，博士生，研究方向为合成孔径雷达成像

景国彬：男，1990年生，博士，研究方向为合成孔径雷达成像

孙光才：男，1984年生，副教授，研究方向为新体制雷达成像、运动目标检测成像

陈权：男，1995年生，硕士生，研究方向为星载合成孔径雷达成像

邢孟道：男，1975年生，教授，研究方向为雷达探测、雷达成像、运动目标检测成像

通讯作者:
刘文康　wkliu@xidian.edu.cn

中图分类号: TN957.52
计量
- 文章访问数: 2113
- HTML全文浏览量: 447
- PDF下载量: 75
- 被引次数: 0
出版历程
- 收稿日期: 2018-03-14
- 修回日期: 2018-09-19
- 网络出版日期: 2018-10-08
- 刊出日期: 2019-01-01

Medium-earth-orbit SAR Data Focusing Method Based on Two-step Azimuth Resampling

Wenkang LIU^{1, 2
, ,},
Guobin JING³,
Guangcai SUN^{1, 2},
Quan CHEN^{1, 2},
Mengdao XING^{1, 2}

1.
National Key Laboratory of Radar Signal Processing, Xidian University, Xi’an 710071, China
2.
Collaborative Innovation Center of Information Sensing and Understanding, Xidian University, Xi’an 710071, China
3.
Milimeter Wave Imaging Technology Laboratory, Shanghai Institute of Satellite Engineering, Shanghai 201109, China

Funds: The National Key R&D Program of China (2017YFC1405600), The Foundation for Innovotive Research Groups of the National Natural Science Foundation of China (61621005), The Fundamental Research Funds for the Central Universities (JB180213)

摘要

摘要:
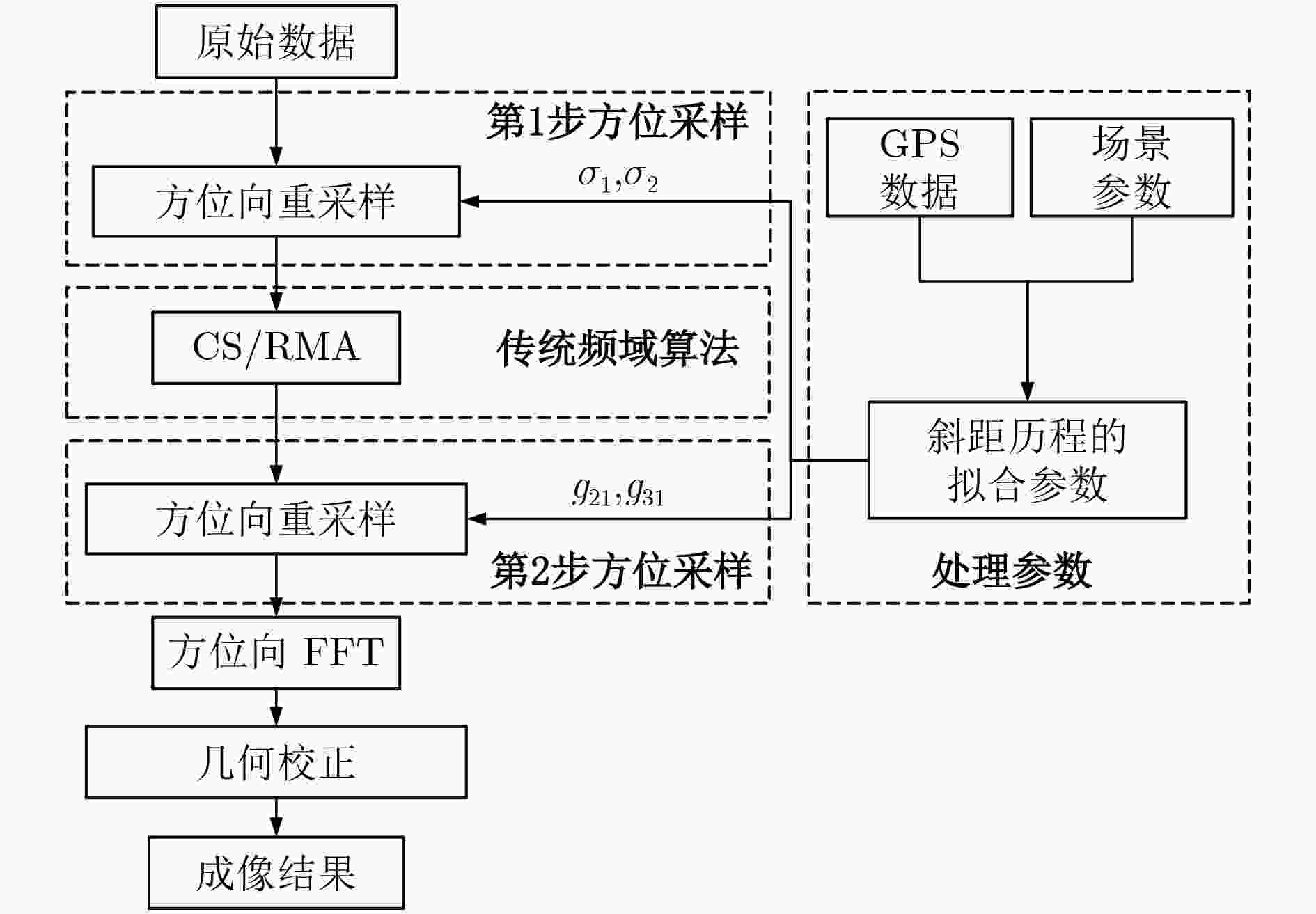
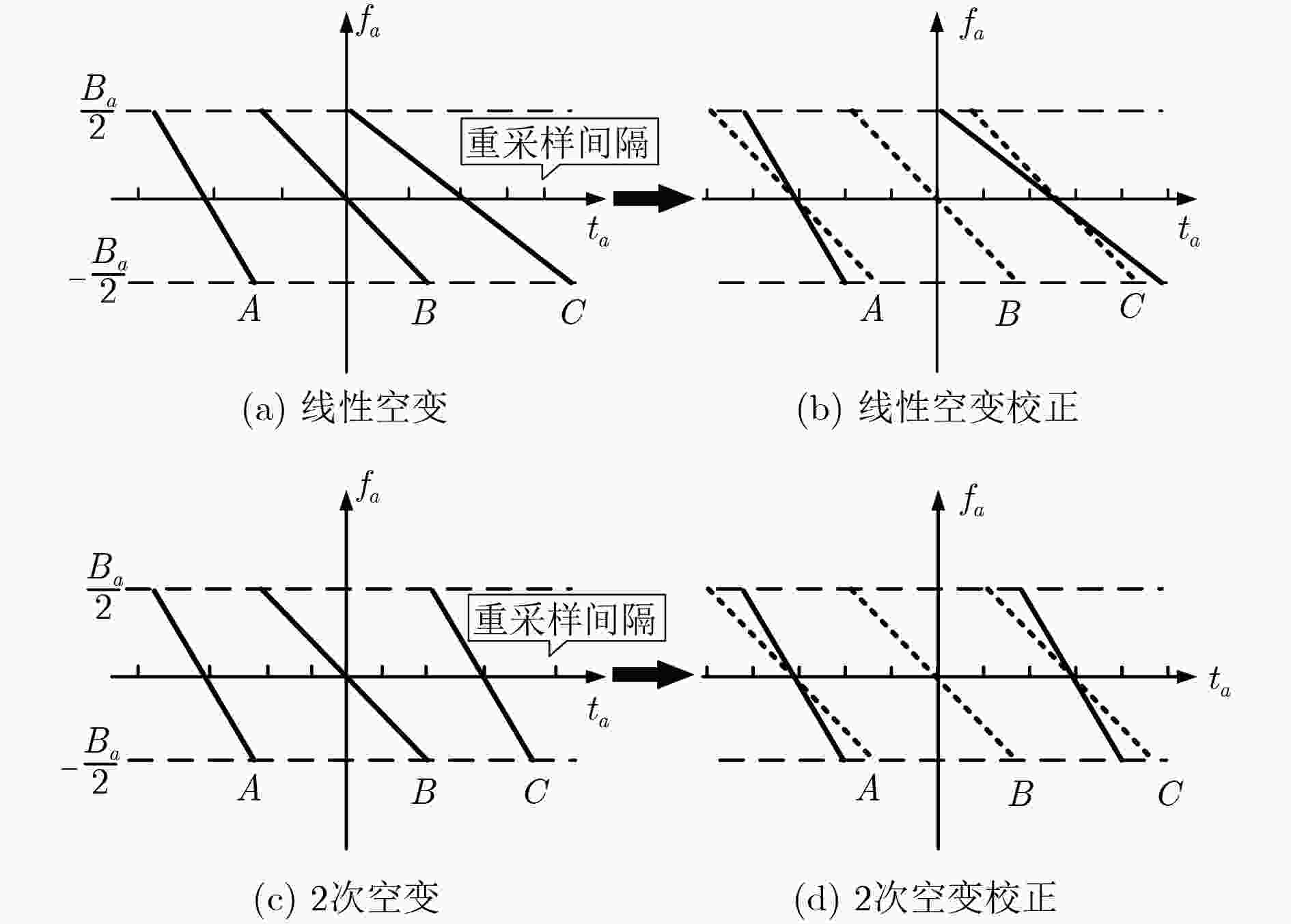
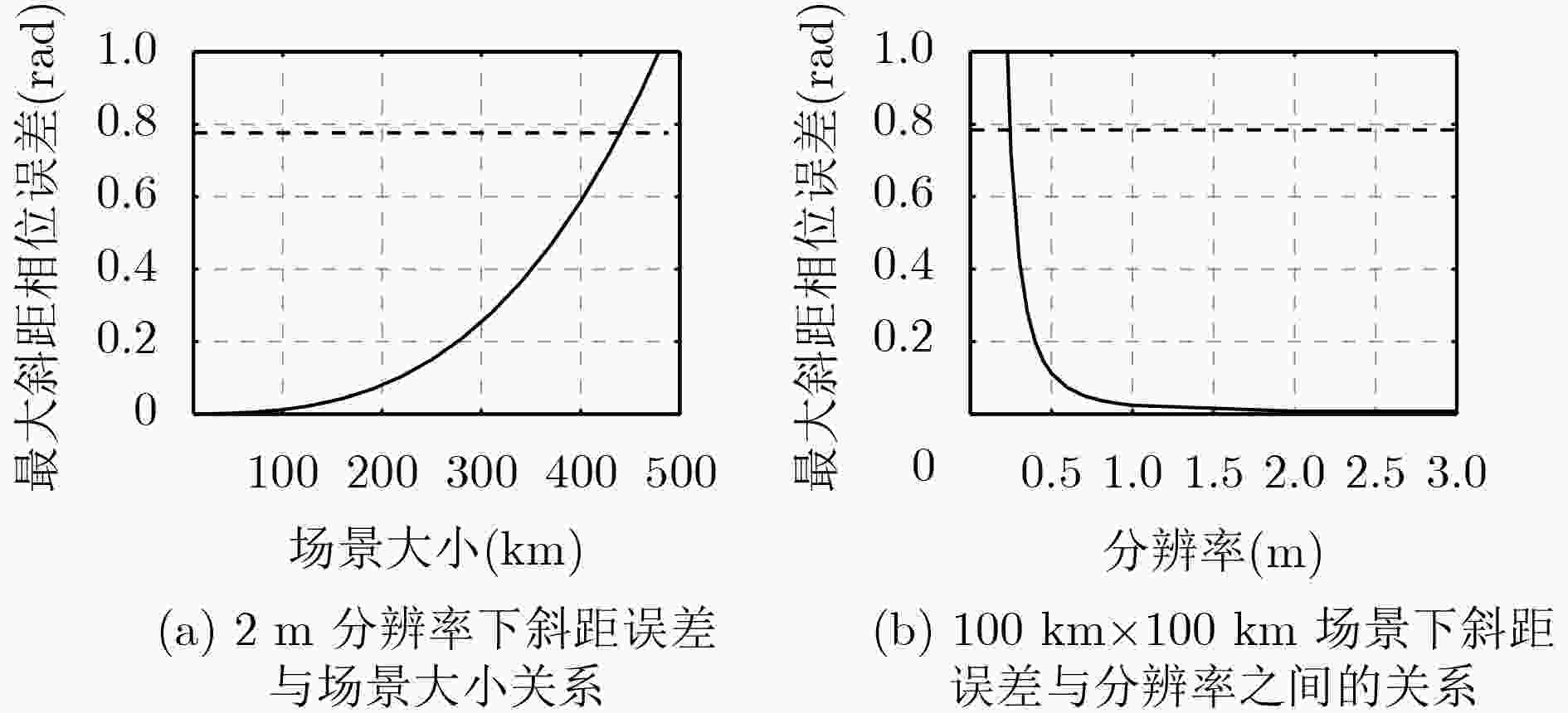
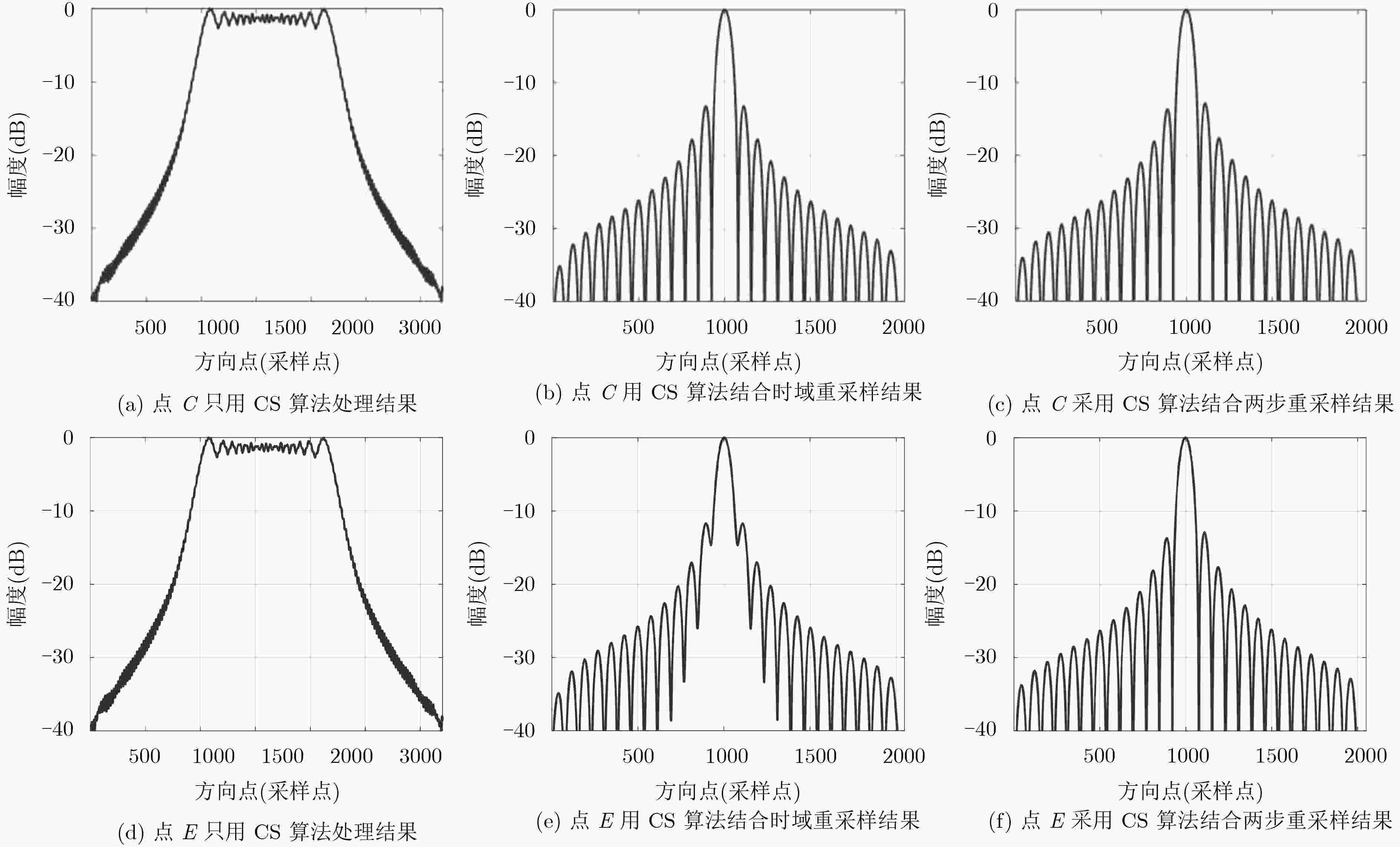
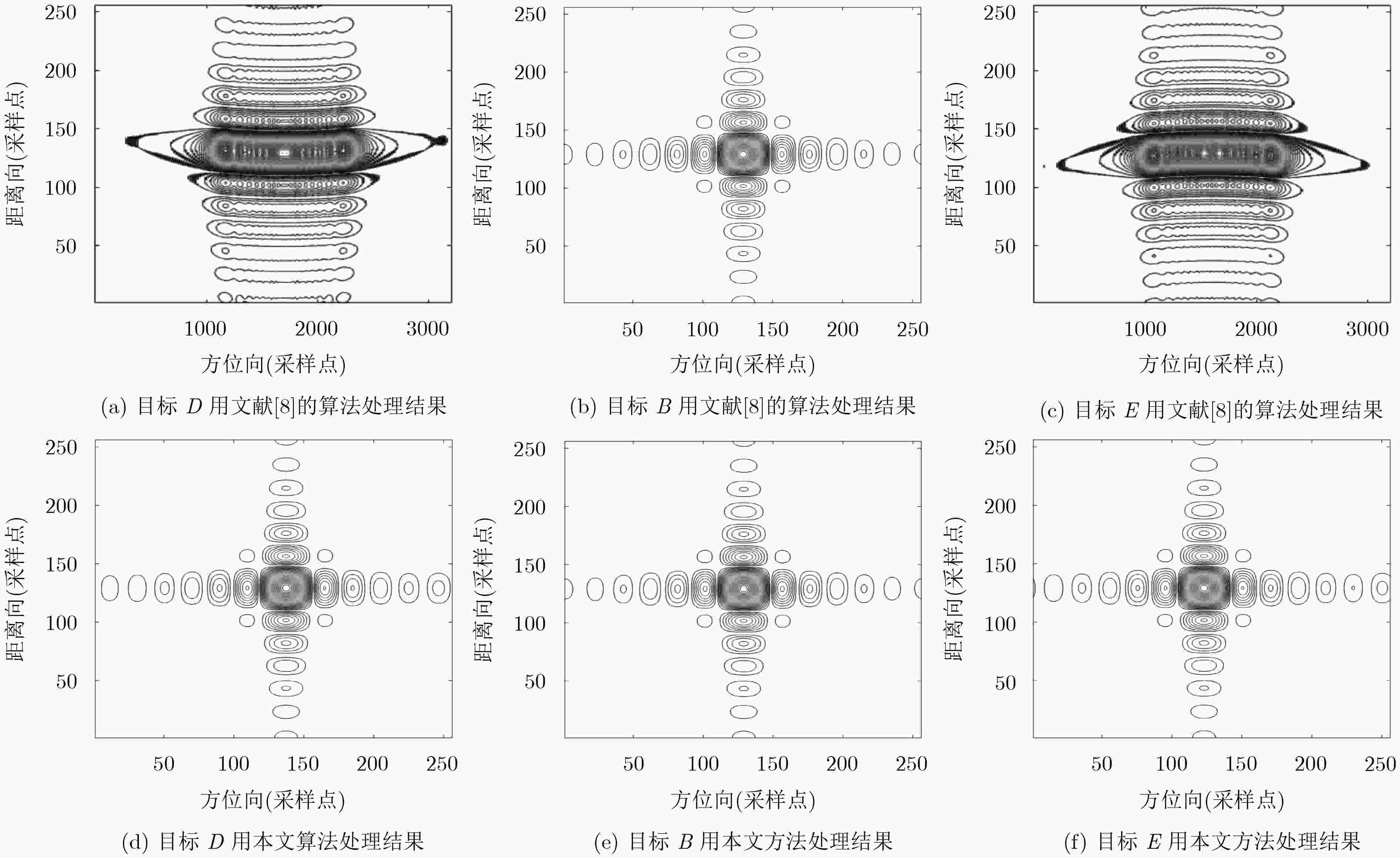
中轨轨道显著的弯曲特性导致中轨SAR信号存在2维空变，因此大场景成像对于中轨SAR仍然是个难题。该文使用参数2维空变的4阶多项式模型对信号进行建模。同时提出一种基于两步方位插值的信号方位空变校正方法，通过方位时域重采样可以校正参考距离上不同方位目标点的多普勒调频率的线性和2次空变，距离向利用CS/RMA算法即可校正场景中所有点目标的距离徙动，而第2步多普勒重采样则能够校正剩余的多普勒参数的空变特性，包括剩余的距离方位耦合空变，以及高阶多普勒参数空变。通过两步插值法能够完全校正整个场景目标信号的方位空变特性，使得传统频域成像算法可以应用于中轨SAR的大场景聚焦。最后通过所提方法与参考方法的仿真结果对比，验证了所提方法的有效性。
- 中轨SAR /
- 空变 /
- 两步重采样
Abstract:
The obvious orbit curvature of Medium Earth Orbit (MEO) results in severe two-dimensional space variance in the received signals. Thus, the focusing of MEO SAR data is still a problem to be solved. Fourth-order polynomial is used to model the range history. Also, an azimuth two-step resampling method is proposed to address the azimuth variance. The azimuth resampling in the time domain can adjust the azimuth chirp rate to be the same, then CS/RMA algorithm can be used to handle the space variance of the RCM. The second-step azimuth resampling can correct the left space variance of the Doppler parameters, including range-azimuth coupled space variance of the azimuth chirp rate, and the higher-order focusing parameters. The proposed method can well address the azimuth space variance of the whole scene, make the conventional frequency-domain focusing algorithms applicable to large scene focusing. Finally, the comparison results obtained by the proposed method and the reference method, validate the effectiveness of the proposed method.
- Medium Earth Orbit (MEO) SAR /
- Space variance /
- Two-step resampling

HTML全文

图 1 基于两步方位重采样的中轨SAR成像处理流程图

下载: 全尺寸图片幻灯片

图 2 调频率方位空变校正

下载: 全尺寸图片幻灯片

图 3 多普勒域重采样校正多普勒参数空变示意图

下载: 全尺寸图片幻灯片

图 4 斜距模型误差分析

下载: 全尺寸图片幻灯片

图 5 仿真场景中点目标布置

下载: 全尺寸图片幻灯片

图 6 点C, E有无各步骤的处理结果对比

下载: 全尺寸图片幻灯片

图 7 纬度幅角为180°时的成像仿真结果

下载: 全尺寸图片幻灯片

表 1 仿真参数

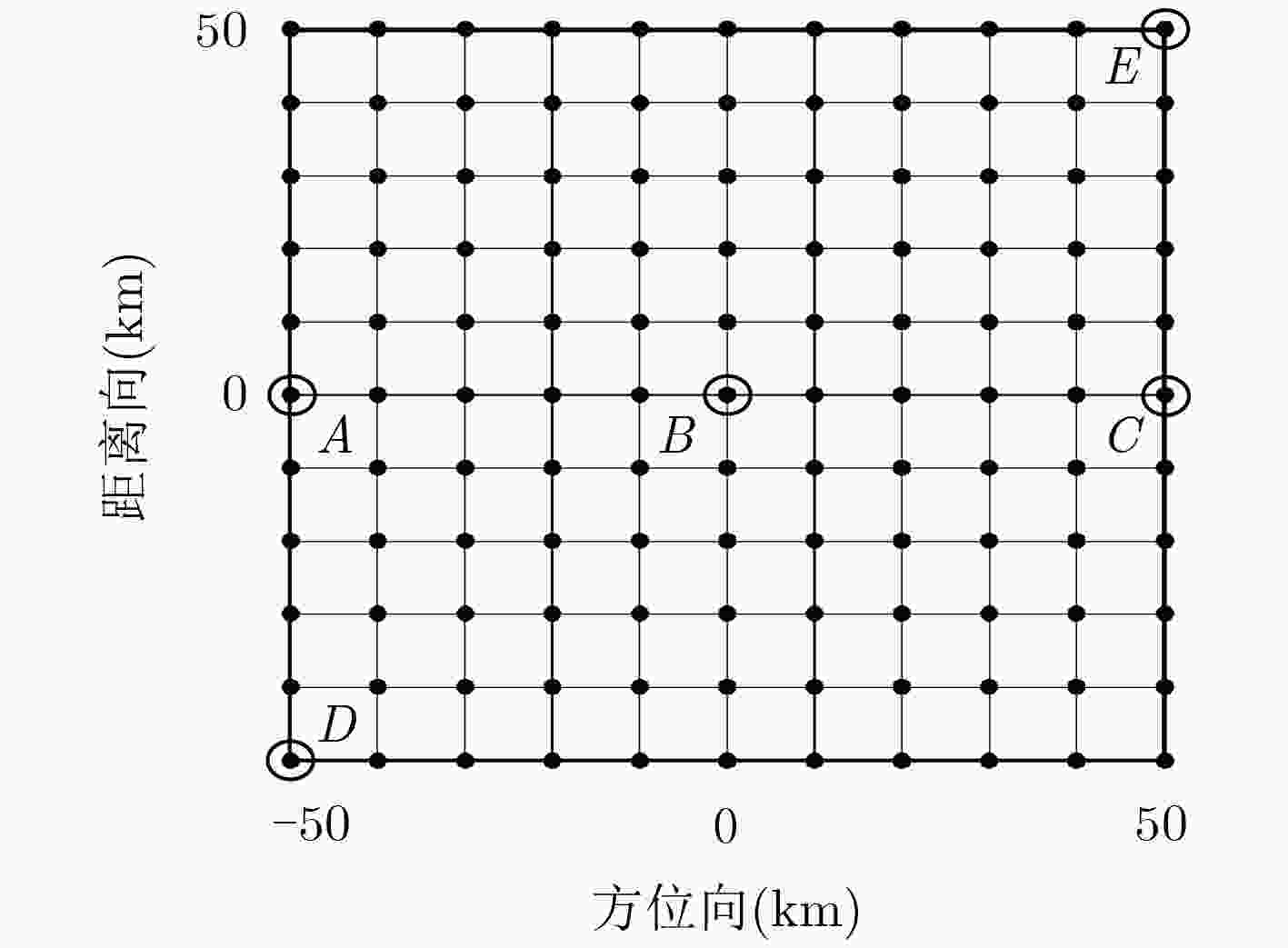
参数类型	参数名称	数值
轨道参数	轨道高度(km)	15000
	离心率	0
	倾角(°)	90
	近地点幅角(°)	0
雷达参数	频率(GHz)	5.4
	带宽(MHz)	103.4
	PRF(Hz)	4000
	入射角(°)	40
	合成孔径时间(s)	43.1
	地表距离分辨率(m)	2
	地表方位分辨率(m)	2
场景参数	场景方位宽度(km)	100
场景参数	场景距离宽度(km)	100

下载: 导出CSV

表 2 成像质量评估(dB)

目标(行，列)	PSLR		ISLR
目标(行，列)	距离向	方位向	距离向	方位向
D(11,1)	–13.27	–13.27	–10.06	–10.06
B(6,6)	–13.24	–13.26	–10.04	–10.06
E(1,11)	–13.27	–13.27	–10.06	–10.05

下载: 导出CSV

参考文献(21)

ROLF W and STEFAN B. The TerraSAR-X mission and system design[J]. IEEE Transactions on Geoscience and Remote Sensing, 2010, 48(2): 606–615. doi: 10.1109/TGRS.2009.2031062

ZHANG Tianyi, DING Zegang, TIAN Weiming, et al. A 2-D nonlinear chirp scaling algorithm for high squint GEO SAR imaging based on optimal azimuth polynomial compensation[J]. IEEE Journal of Selected Topics in Applied Earth Observations And Remote Sensing, 2017, 10(12): 5724–5735. doi: 10.1109/JSTARS.2017.2765353

STEPHEN H. System design for geosynchronous synthetic aperture radar missions[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(12): 7750–7763. doi: 10.1109/TGRS.2014.2318171

JALAL M, PACO L D, and GERHARD K. Potentials and limitations of MEO SAR[C]. European Conference on Synthetic Aperture Radar, Hamburg, Germany, 2016: 1–5.

TIAN Ye, HU Cheng, DONG Xichao, et al. Theoretical analysis and verification of time variation of background ionosphere on geosynchronous SAR imaging[J]. IEEE Geoscience and Remote Sensing Letters, 2015, 12(4): 721–725. doi: 10.1109/LGRS.2014.2360235

LI Liang, HONG Jun, and MING Feng. Study about ionospheric effects on medium-earth-orbit SAR imaging[C]. 2014 IEEE Radar Conference, Cincinnati, USA, 2014: 27–31.

温雪娇, 仇晓兰, 尤红建, 等. 高分辨率星载SAR起伏运动目标精聚焦与参数估计方法[J]. 雷达学报, 2017, 6(2): 213–220. doi: 10.12000/JR17005

WEN Xuejiao, QIU Xiaolan, YOU Hongjian, et al. Focusing and parameter estimation of fluctuating targets in high resolution space-borne SAR[J]. Journal of Radars, 2017, 6(2): 213–220. doi: 10.12000/JR17005

HUANG Lijia, QIU Xiaolan, HU Donghui, et al. Focusing of medium-earth-orbit SAR with advanced nonlinear chirp scaling algorithm[J]. IEEE Transactions on Geoscience and Remote Sensing, 2011, 49(1): 500–508. doi: 10.1109/TGRS.2010.2053211

BAO Ming, XING Mengdao, LI Yachao, et al. Two-dimensional spectrum for MEO SAR processing using a modified advanced hyperbolic range equation[J]. Electronics Letters, 2011, 47(18): 1043–1045. doi: 10.1049/el.2011.1322

TANG Shiyang, LIN Chunhui, ZHOU Yu, et al. Processing of long integration time spaceborne SAR data with curved orbit[J]. IEEE Transactions on Geoscience and Remote Sensing, 2018, 52(2): 888–904. doi: 10.1109/TGRS.2017.2756109

CHEN Jie, KUANG Hui, YANG Wei, et al. A novel imaging algorithm for focusing high-resolution spaceborne SAR data in squinted sliding-spotlight mode[J]. IEEE Geoscience and Remote Sensing Letters, 2016, 13(10): 1577–1581. doi: 10.1109/LGRS.2016.2598066

RICHARD B. A comparison of range-Doppler and wavenumber domain SAR focusing algorithms[J]. IEEE Transactions on Geoscience and Remote Sensing, 1992, 30(4): 706–713. doi: 10.1109/36.158864

HUANG Lijia, QIU Xiaolan, HU Donghui, et al. Medium-Earth-Orbit SAR focusing using range Doppler algorithm with integrated two-step azimuth perturbation[J]. IEEE Geoscience and Remote Sensing Letters, 2015, 12(3): 626–630. doi: 10.1109/LGRS.2014.2353674

WANG Yan, LI Jingwen, XU Feng, et al. A new nonlinear chirp scaling algorithm for high-squint high-resolution SAR imaging[J]. IEEE Geoscience and Remote Sensing Letters, 2017, 14(12): 2225–2229. doi: 10.1109/LGRS.2017.2758386

LI Zhenyu, XING Mengdao, LIANG Yi, et al. A frequency-domain imaging algorithm for highly squinted SAR mounted on maneuvering platforms with nonlinear trajectory[J]. IEEE Transactions on Geoscience and Remote Sensing, 2016, 54(7): 4023–4038. doi: 10.1109/TGRS.2016.2535391

LI Zhenyu, LIANG Yi, XING Mengdao, et al. An improved range model and omega-k-based imaging algorithm for high-squint SAR with curved trajectory and constant acceleration[J]. IEEE Geoscience and Remote Sensing Letters, 2016, 13(5): 656–660. doi: 10.1109/LGRS.2016.2533631

LI Dexin, WU Manqing, SUN Zaoyu, et al. Modeling and processing of two-dimensional spatial-variant geosynchronous SAR data[J]. IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing, 2015, 8(8): 3999–4009. doi: 10.1109/JSTARS.2015.2418814

DAVIDE D and ANDREA M G. High-resolution spaceborne SAR focusing by SVD-Stolt[J]. IEEE Geoscience and Remote Sensing Letters, 2007, 4(4): 639–643. doi: 10.1109/LGRS.2007.903081

CHEN Jianlai, SUN Guangcai, YANG Jun, et al. A TSVD-NCS algorithm in range-Doppler domain for geosynchronous synthetic aperture radar[J]. IEEE Geoscience and Remote Sensing Letters, 2016, 13(11): 1631–1635. doi: 10.1109/LGRS.2016.2599224

HAUKE F, ELKE B, and JOSEF M. Total zero Doppler steering—A new method for minimizing the Doppler centroid[J]. IEEE Geoscience and Remote Sensing Letters, 2005, 2(2): 141–145. doi: 10.1109/LGRS.2005.844591

DANIEL P S. Analytic yaw-pitch steering for side-looking SAR with numerical roll algorithm for incidence angle[J]. IEEE Transactions on Geoscience and Remote Sensing, 2012, 50(9): 3587–3594. doi: 10.1109/TGRS.2012.2183375

施引文献

资源附件(0)

访问统计