A Novel Method for Nonlinear Processing in Impulsive Noise Based on Gaussianization and Generalized Matching
-
摘要: 针对脉冲型噪声，该文提出一种新的非线性处理方法，即高斯化-广义匹配(GGM)处理。GGM方法基于高斯化处理与广义匹配滤波，可结合非参数的概率密度估计进行设计，解决噪声模型未知时的非线性处理问题。该文以脉冲型噪声
${\rm S\alpha S}$ 分布模型为例，分析GGM方法的特点和性能；再结合Class A噪声模型，讨论GGM设计作为非参数方法相比模型假设失配的优势；引入效能函数，验证GGM方法在恒虚警技术中的运用。结果表明，在已知噪声分布情况下，GGM方法具有次优检测性能；当噪声模型未知时，非参数GGM设计能保持稳健性能，优于模型失配下的处理。并且，GGM设计对样本数目要求不高，为噪声特性不明或时变的场景提供了一种新的信号处理方法。Abstract: A method based on Gaussianization and generalized matching, called Gaussianization-Generalized Matching (GGM) method is proposed, for nonlinear processing in impulsive noise. The GGM method can be designed based on noise samples, aided by nonparametric probability density estimation. Thus the GGM design is suitable for nonlinear processing in unknown noise models. The GGM method in the${\rm S\alpha S}$ model is analyzed, and also the comparison with another approach is presented based on unmatched noise model assumption in the Class A noise. The GGM method is applied to the constant false alarm rate technique via the efficacy function. Simulation and analysis results show that the GGM design is sub-optimal, works robustly when the noise model is unknown, and raises a low requirement on the sample number. Thus, the GGM method provides a promising choice when the noise model is unclear or time-varying.-
Key words:
- Impulsive noise /
- Nonlinear processing /
- Gaussianization /
- Generalized matching /
- Efficiency function
-
KAY S M. Fundamentals of Statistical Signal Processing, Volume II: Detection Theory[M]. Englewood Cliffs, US, Prentice-Hall, Inc., 1993: 94–115. LACHOS V H, ANGOLINI T, and ABANTO-VALLE C A. On estimation and local influence analysis for measurement errors models under heavy-tailed distributions[J]. Statistical Papers, 2011, 52(3): 567–590 doi: 10.1007/s00362-009-0270-4 DAVIS R R and CLAVIER O. Impulsive noise: A brief review[J]. Elsevier Hearing Research, 2017, 349: 34–36 doi: 10.1016/j.heares.2016.10.020 LI Xutao, JIN Lianwen, and WANG Shouyong. A simplified non-Gaussian mixture model for signal LO detection in -stable interference[C]. IEEE Congress on Image and Signal Processing, Beijing, China, 2008: 403–407. SHAO M and NIKIAS C L. Signal processing with fractional lower order moments: stable processes and their applications[J]. Proceedings of the IEEE, 1993, 81(7): 986–1010 doi: 10.1109/5.231338 ZHANG Guoyong, WANG Jun, YANG Guosheng, et al. Nonlinear processing for correlation detection in symmetric alpha-stable noise[J]. IEEE Signal Processing Letters, 2018, 25(1): 120–124 doi: 10.1109/LSP.2017.2776317 MIDDLETON D. Procedures for determining the parameters of the first-order canonical models of Class A and Class B electromagnetic interference[J]. IEEE Transactions on Electromagnetic Compatibility, 2007, 21(3): 190–208 doi: 10.1109/TEMC.1979.303731 TSIHRINTZIS G A and NIKIAS C L. Performance of optimum and suboptimum receivers in the presence of impulsive noise modeled as an alpha-stable process[J]. IEEE Transactions on Communications, 1995, 43(2/3/4): 904–914 doi: 10.1109/26.380123 VADALI S R K, RAY P, MULA S, et al. Linear detection of a weak signal in additive Cauchy noise[J]. IEEE Transactions on Communications, 2017, 65(3): 1061–1076 doi: 10.1109/TCOMM.2016.2647599 OH H and NAM H. Design and performance analysis of nonlinearity preprocessors in an impulsive noise environment[J]. IEEE Transactions on Vehicular Technology, 2017, 66(1): 364–376 doi: 10.1109/TVT.2016.2547889 LI Xutao, SUN Jun, WANG Shouyong, et al. Near-optimal detection with constant false alarm ratio in varying impulsive interference[J]. IET Signal Processing, 2013, 7(9): 824–832 doi: 10.1049/iet-spr.2013.0024 LI Xutao, CHEN Peng, FAN Lisheng, et al. Normalization-based receiver using BCGM approximation for -stable noise channels[J]. Electronics Letters, 2013, 49(15): 965–967 doi: 10.1049/el.2013.1289 SWAMI A and SADLER B M. On some detection and estimation problems in heavy-tailed noise[J]. Elsevier Signal Processing, 2002, 82(12): 1829–1846 doi: 10.1016/S0165-1684(02)00314-6 张杨勇, 刘勇. 低频段大气噪声及处理技术[J]. 舰船科学技术, 2008, 30(S1): 85–88 doi: 10.3404/j.issn.1672-7649.2008.S021ZHANG Yangyong and LIU Yong. Atmospheric-noise at low frequency and its processing technique[J]. Ship Science&Technology, 2008, 30(S1): 85–88 doi: 10.3404/j.issn.1672-7649.2008.S021 WANG Pingbo, LIU Feng, CAI Zhiming, et al. G-Filter's Gaussianization function for interference background[C]. International Conference on Signal Acquisition and Processing, Nanjing, China, 2010: 76–79. SAMIUDDIN M and EL-SAYYAD G M. On nonparametric kernel density estimates[J]. Biometrika, 1990, 77(4): 865–874 doi: 10.1093/77.4.865 SILVERMAN B W. Density Estimation for Statistics and Data Analysis[M]. London, UK, Chapman & Hall, 1986: 45–48. HASHEMIFARD Z and AMINDAVAR H. PDF approximations to estimation and detection in time-correlated alpha-stable channels[J]. Elsevier Signal Processing, 2017, 133: 97–106 doi: 10.1016/j.sigpro.2016.10.021 BIBALAN M H, AMINDAVAR H, and AMIRMAZLAGHANI M. Characteristic function based parameter estimation of skewed alpha-stable distribution: An analytical approach[J]. Elsevier Signal Processing, 2017, 130: 323–336 doi: 10.1016/j.sigpro.2016.07.020 KOLODZIEJSKI K R and BETZ J W. Detection of weak random signals in IID non-Gaussian noise[J]. IEEE Transactions on Communications, 2000, 48(2): 222–230 doi: 10.1109/26.823555 ARIF M, NASEEM I, MOINUDDIN M, et al. Design of optimum error nonlinearity for channel estimation in the presence of Class-A impulsive noise[C]. IEEE International Conference on Intelligent and Advanced Systems, Kuala Lumpur, Malaysia, 2017: 1–6. WEINBERG G V. On the construction of CFAR decision rules via transformations[J]. IEEE Transactions on Geoscience and Remote Sensing, 2017, 55(2): 1140–1146 doi: 10.1109/TGRS.2016.2620138 -
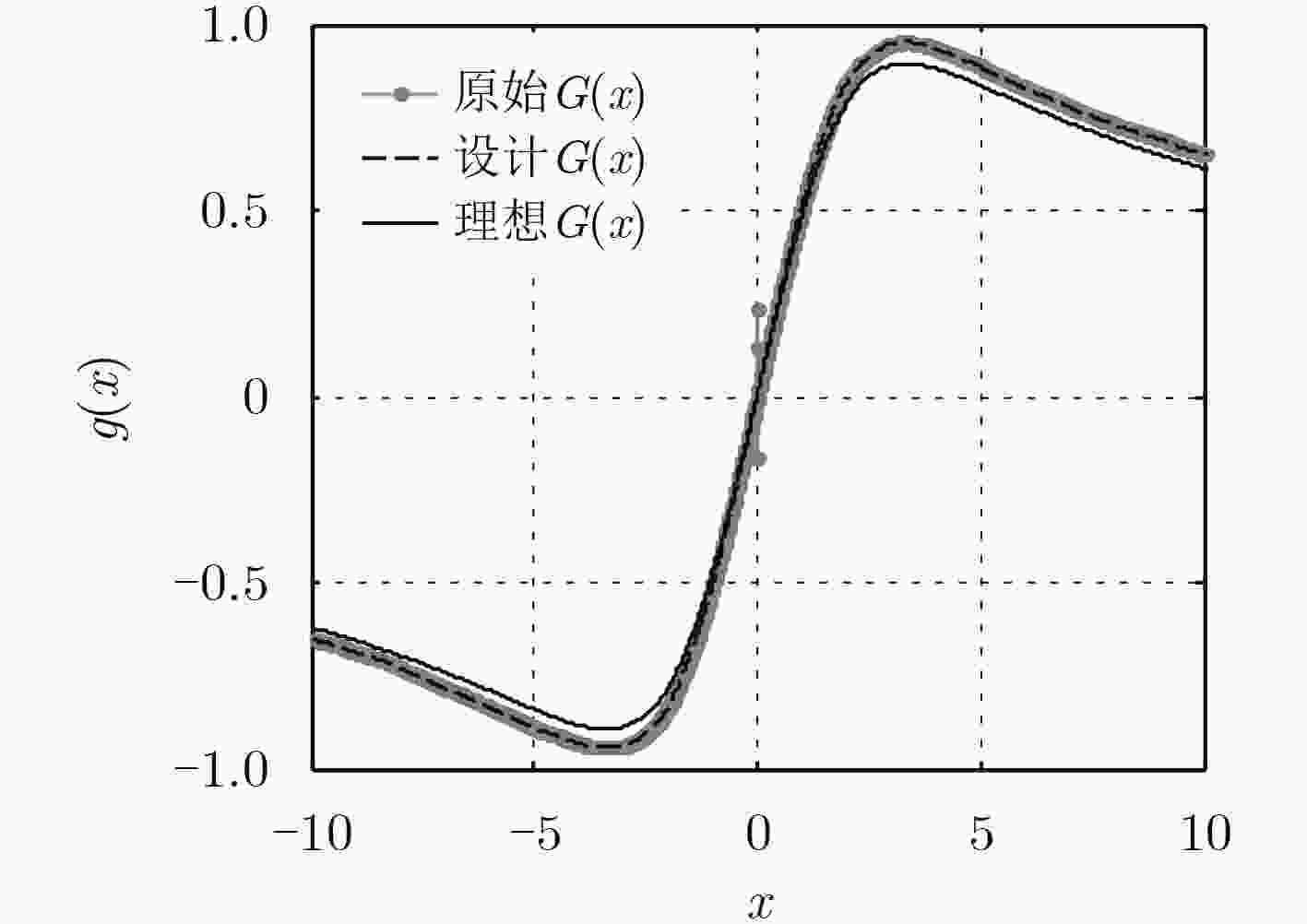
下载:
图(7)
计量
- 文章访问数: 1675
- HTML全文浏览量: 840
- PDF下载量: 64
- 被引次数: 0
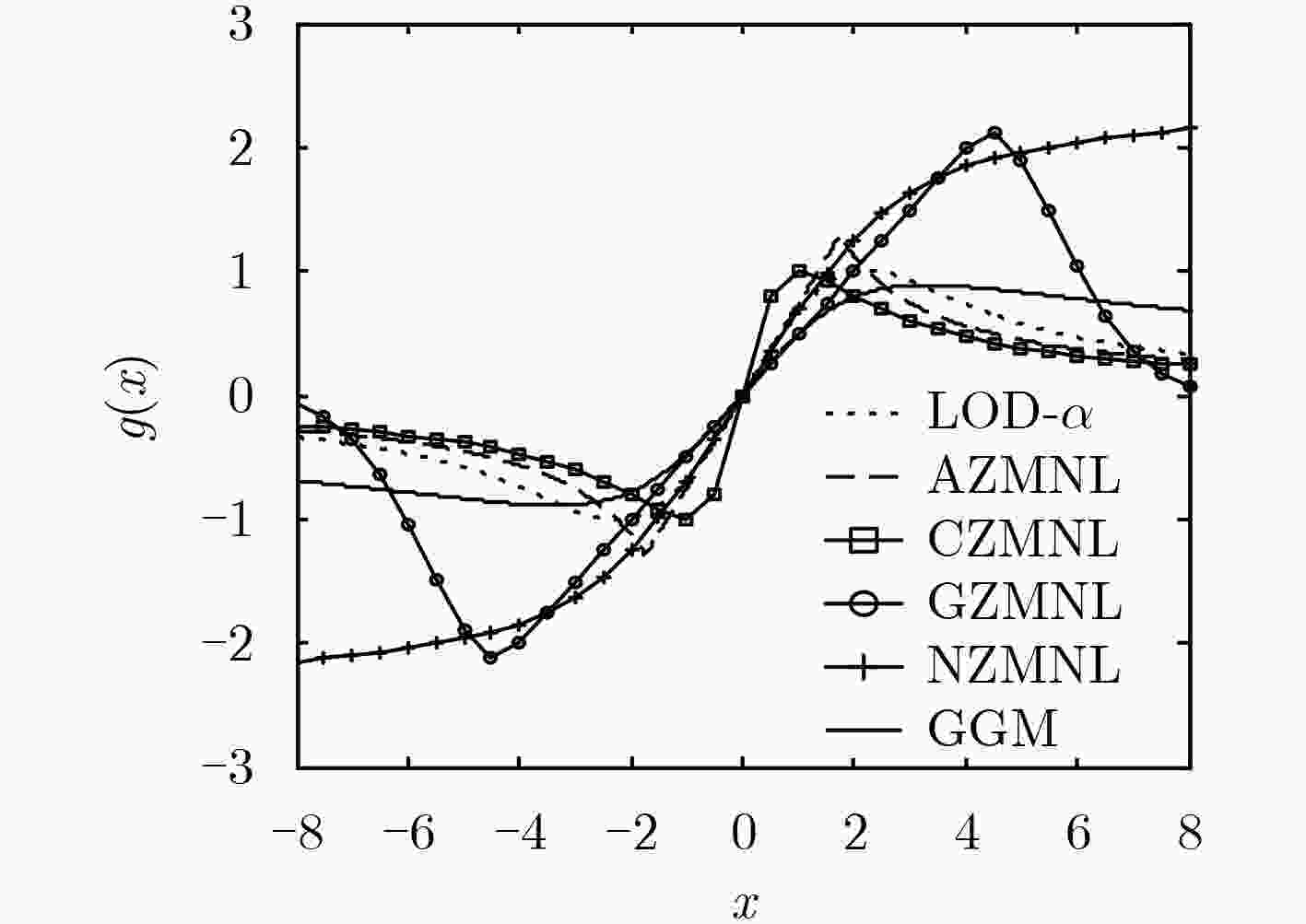
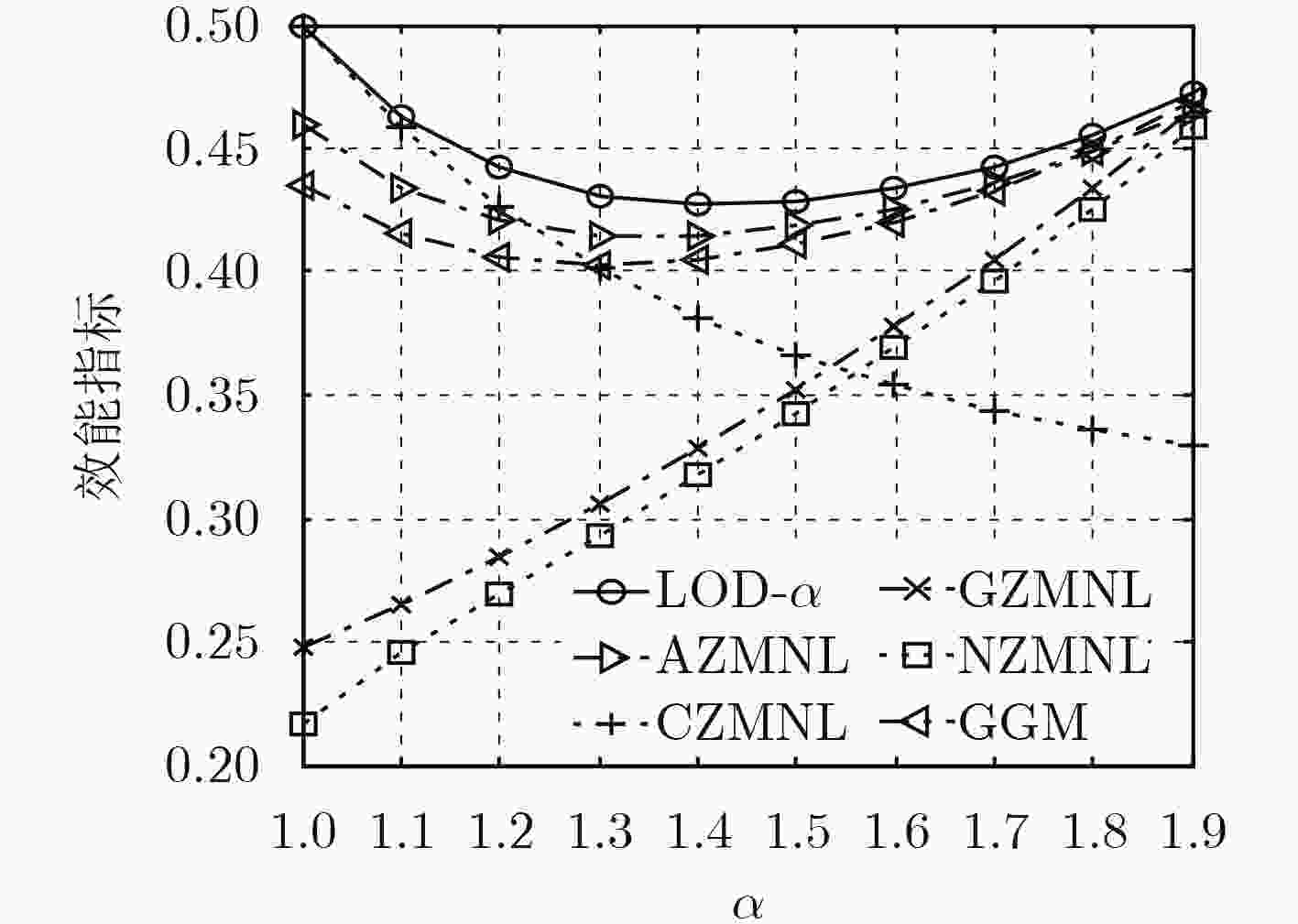
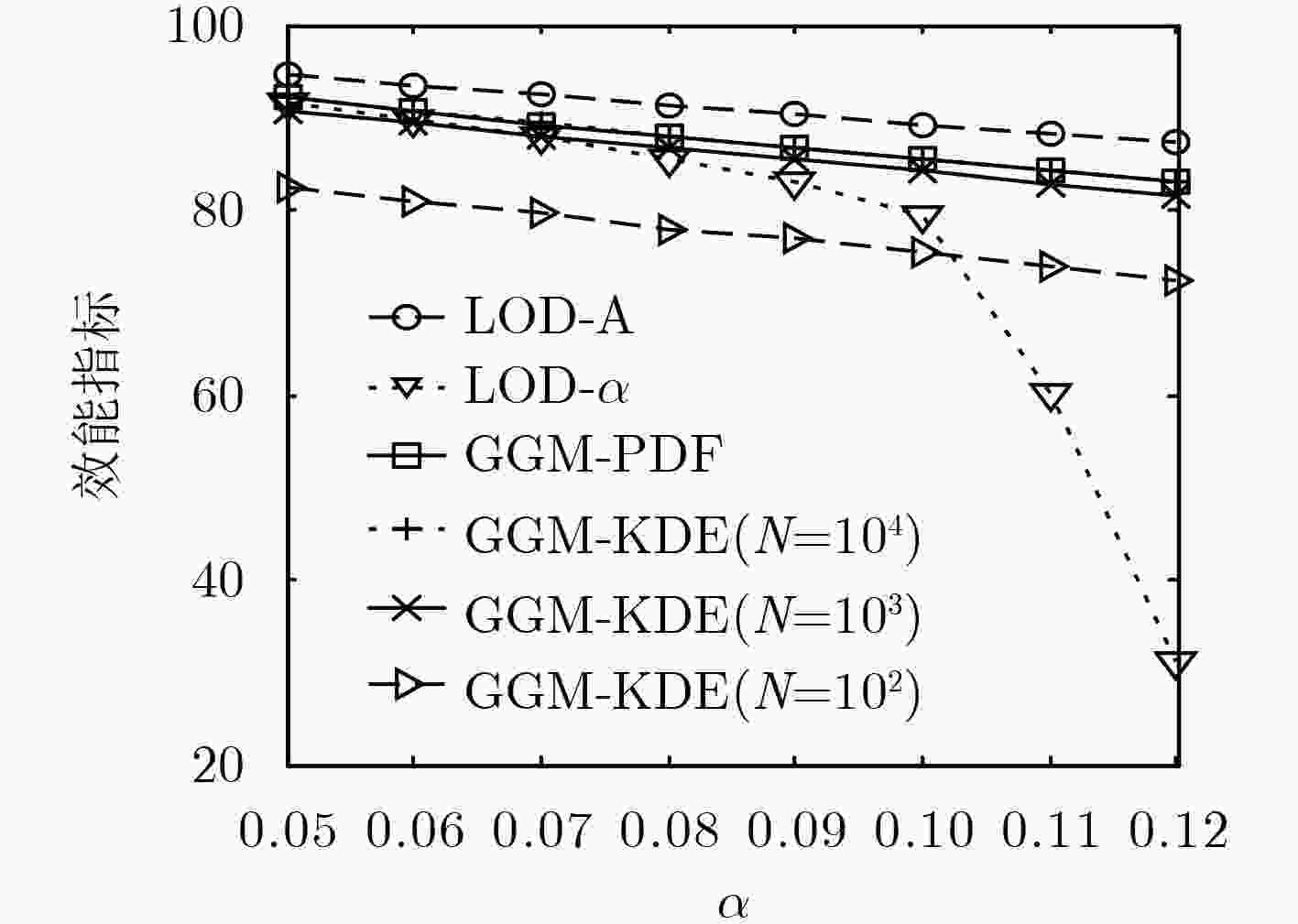
下载:
