Maximum Eigenvalue Based Radar Signal Detection Method for K Distribution Sea Clutter Environment
-
摘要: 针对K分布海杂波背景下的恒虚警检测问题,基于信息几何的矩阵CFAR检测器具有较好的检测性能,但其计算复杂度较高,从而影响其实际应用。该文根据奈曼-皮尔逊准则,推导了似然比检测统计量与最大特征值之间的关系,进而提出了基于最大特征值的矩阵CFAR检测方法(M-MED)。最后通过对所提方法的计算复杂度及仿真实验结果的分析表明了所提方法不仅计算复杂度低且具有较好的检测性能。Abstract: Information geometry based matrix Constant False Alarm Rate (CFAR) detector is an efficient solution to the intractable issue of target detection for K-distributed sea clutter environment. However, most existing matrix CFAR detectors cost heavy computation complexity, which leads to a limitation in practical application. Based on the Neyman-Pearson criterion, the Likelihood Ratio Test (LRT) is analyzed, the relationship between LRT statistic and the Maximum Eigenvalue is derived, and Matrix CFAR Detection method based on the Maximum Eigenvalue (M-MED) is designed. Simulation results verify that the proposed method can achieve better detection performance with relatively lower computational complexity.
-
Key words:
- CFAR detection /
- K-distribution /
- Maximum eigenvalue
-
表 1 不同方法的计算复杂度比较
算法 协方差矩阵 指数运算 对数运算 幂运算 特征值分解O(M3) 基本运算量 本文M-MED √ × × × √ $N$ KLD √ × × × × $O(M! + (N + 3){M^3} + 2{M^2} + M)$ REM √ √ √ √ √ $O({n_t}((N + 2){M^3} + 3N{M^2}) + {M^3} + 2{M^2} + 2M - 1)$ LD √ × × × × $M! + (N + 4){M^3} + 2{M^2} + M$ LE √ √ √ × √ $(N + 2){M^2} + 2M - 1$ Bha √ × × × × $O({n_t}((N + 1){M^3} + (2N + 1){M^2}) + 3M! + 2{M^2} + 3)$ HEL √ × × × × $O({n_t}(3NM! + (N + 3){M^3} + 4N{M^2}) + 3M! + 2{M^2} + 4)$ -
WARD K D, WATTS S, and TOUGH R JA. Sea Clutter: Scattering, the K Distribution and Radar Performance[M]. London, United Kingdom: 2006: 101–134. WANG Bing, CUI Guolong, YI Wei, et al. Constant false alarm rate performance prediction for non-independent and non-identically distributed gamma fluctuating targets[J].IET Radar, Sonar&Navigation, 2016, 10(5): 992–1000 doi: 10.1049/iet-rsn.2014.0563 CHEN Xiaolong, GUAN Jian, BAO Zhonghua, et al. Detection and extraction of target with micro-motion in spiky sea clutter via short-time fractional Fourier transform[J]. IEEE Transactions on Geoscience and Remote Sensing, 2014, 52(2): 1002–1018 doi: 10.1109/TGRS.2013.2246574 陈小龙, 关键, 董云龙, 等. 稀疏域海杂波抑制与微动目标检测方法[J]. 电子学报, 2016, 44(4): 860–867 doi: 10.3969/j.issn.0372-2112.2016.04.015CHEN Xiaolong, GUAN Jian, DONG Yunlong, et al. Sea clutter suppression and micromotion target detection in sparse domain[J]. Acta Electronica Sinica, 2016, 44(4): 860–867 doi: 10.3969/j.issn.0372-2112.2016.04.015 ARNAUDON M, BARBARESCO F, and YANG Le. Riemannian medians and means with applications to radar signal processing[J]. IEEE Journal of Selected Topics in Signal Processing, 2013, 7(4): 595–604 doi: 10.1109/JSTSP.2013.2261798 CHENG Yongqiang, HUA Xiaoqiang, WANG Hongqiang, et al. The geometry of signal detection with applications to radar signal processing[J]. Entropy, 2016, 18(381): 1–17 doi: 10.3390/e18110381 赵兴刚, 王首勇. 一种基于KL分离度的改进矩阵CFAR检测方法[J]. 电子与信息学报, 2016, 38(4): 934–940 doi: 10.11999/JEIT150711ZHAO Xinggang and WANG Shouyong. An improved matrix CFAR detection method base on KL divergence[J]. Journal of Electronics&Information Technology, 2016, 38(4): 934–940 doi: 10.11999/JEIT150711 HUA Xiaoqiang, CHENG Yongqiang, WANG Hongqiang, et al. Geometric means and medians with applications to target detection[J]. IET Signal Processing, 2017, 11(6): 711–720 doi: 10.1049/iet-spr.2016.0547 赵兴刚, 王首勇. 基于K-L散度和散度均值的改进矩阵CFAR检测器[J]. 中国科学: 信息科学, 2017, 47(2): 247–259 doi: 10.1360/N112016-00105ZHAO Xinggang and WANG Shouyong. Improved matrix CFAR detector based on K-L divergence and divergence mean (in Chinese)[J]. Scientia Sinica Informationis, 2017, 47(2): 247–259 doi: 10.1360/N112016-00105 ALI S S, LIU Chang, LIU Jialong, et al. On the eigenvalue based detection for multiantenna cognitive radio system[J]. Mobile Information Systems, 2016, 2016: 1–8 doi: 10.1155/2016/3848734 LIU Chang, LI Husheng, WANG Jie, et al. Optimal eigenvalue weighting detection for multi-antenna cognitive radio networks[J]. IEEE Transactions on Wireless Communications, 2017, 16(4): 2083–2096 doi: 10.1109/TWC.2016.2632711 LIU Jun, LI Hongbin, and BRAHAM H. Two target detection algorithms for passive multistatic radar[J]. IEEE Transactions on Signal Processing, 2014, 62(22): 5930–5939 doi: 10.1109/TSP.2014.2359637 陈远征, 陈建军, 付强. 复合高斯杂波下基于GLRT的扩展目标检测[J]. 电子与信息学报, 2010, 32(6): 1327–1331 doi: 10.3724/SP.J.1146.2009.00832CHEN Yuanzheng, CHEN Jianjun, and FU Qiang. Detection of range spread target based on GLRT in compound-Gaussian clutter[J]. Journal of Electronics&Information Technology, 2010, 32(6): 1327–1331 doi: 10.3724/SP.J.1146.2009.00832 王松贵, 吴密霞, 贾忠贞. 矩阵不等式[M]. 北京: 科学出版社, 2006: 96–103.WANG Songgui, WU Mixia, and JIA Zhongzhen. Matrix Inequalities [M]. Beijing: Science Press, 2006: 96–103. WATKINS D. Fundamentals of Matrix Computation [M]. Hoboken, New Jersey: John Wiley & Sons, Inc., 2010: 71–105. IPIX radar file, IPIX radar dataset files in Dartmouth [OL]. http://soma.ece.cmaster.ca/ipix/dartmouth/cdfhowto.html, 1993. -






 下载:
下载:
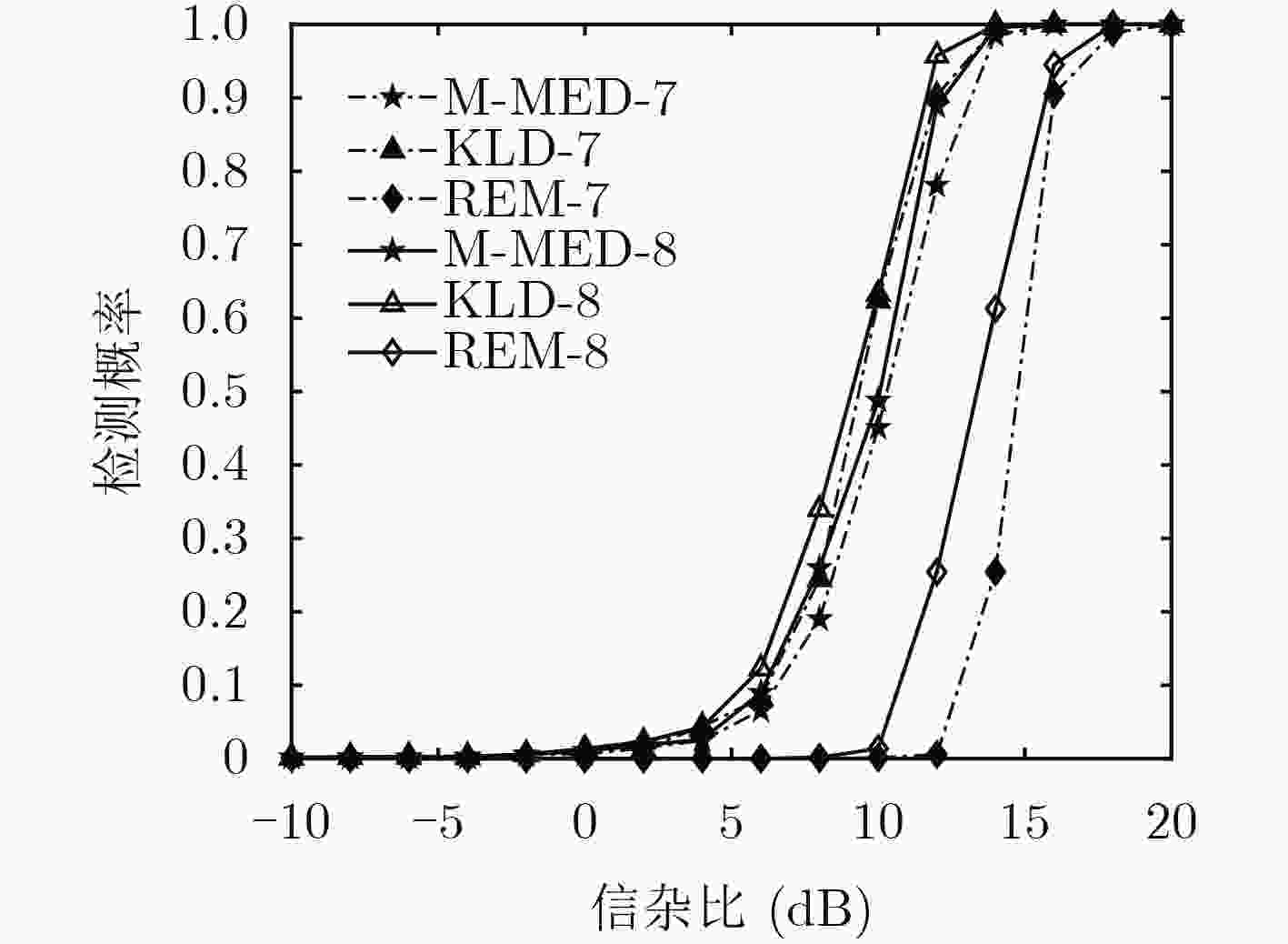
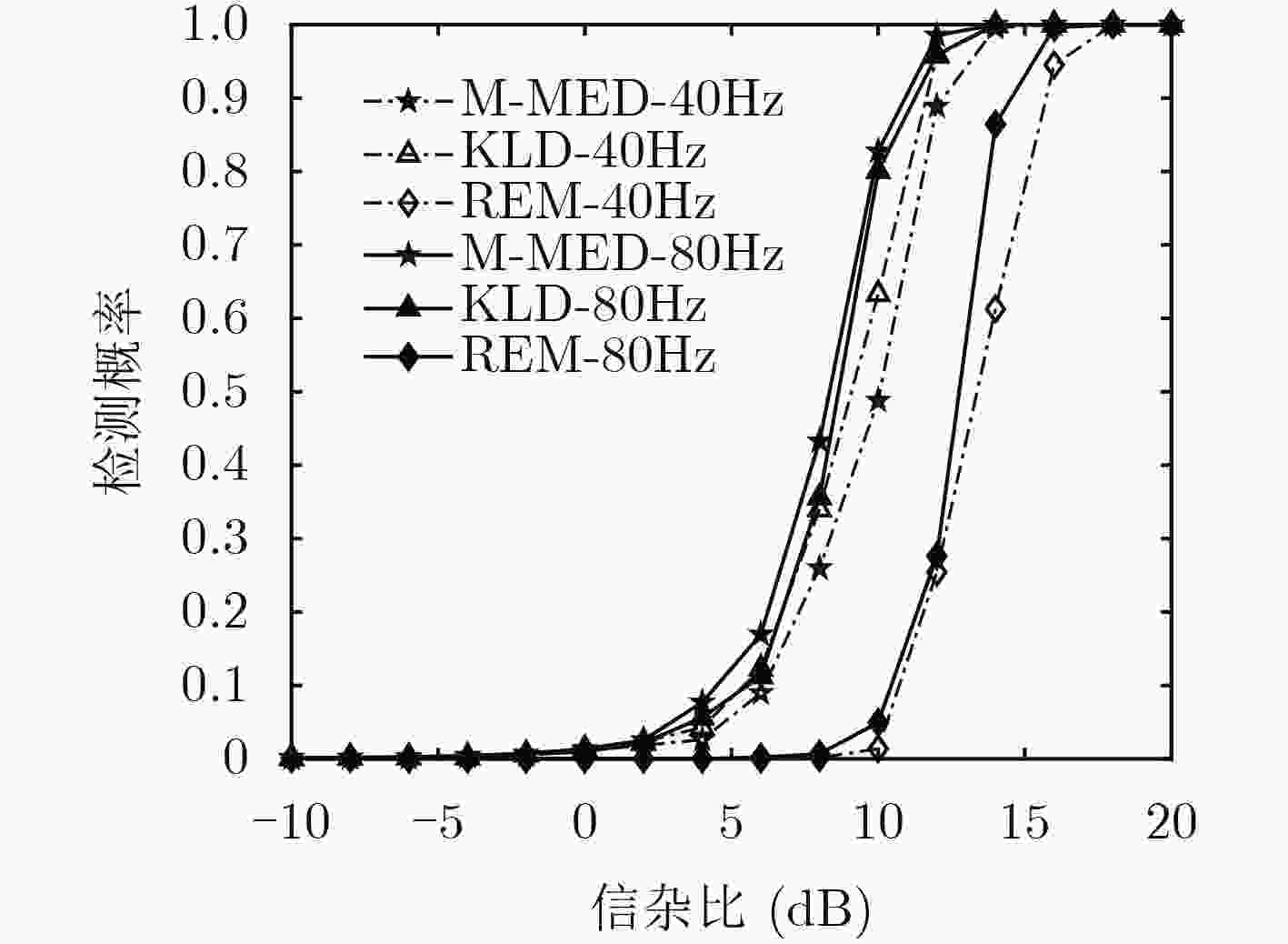
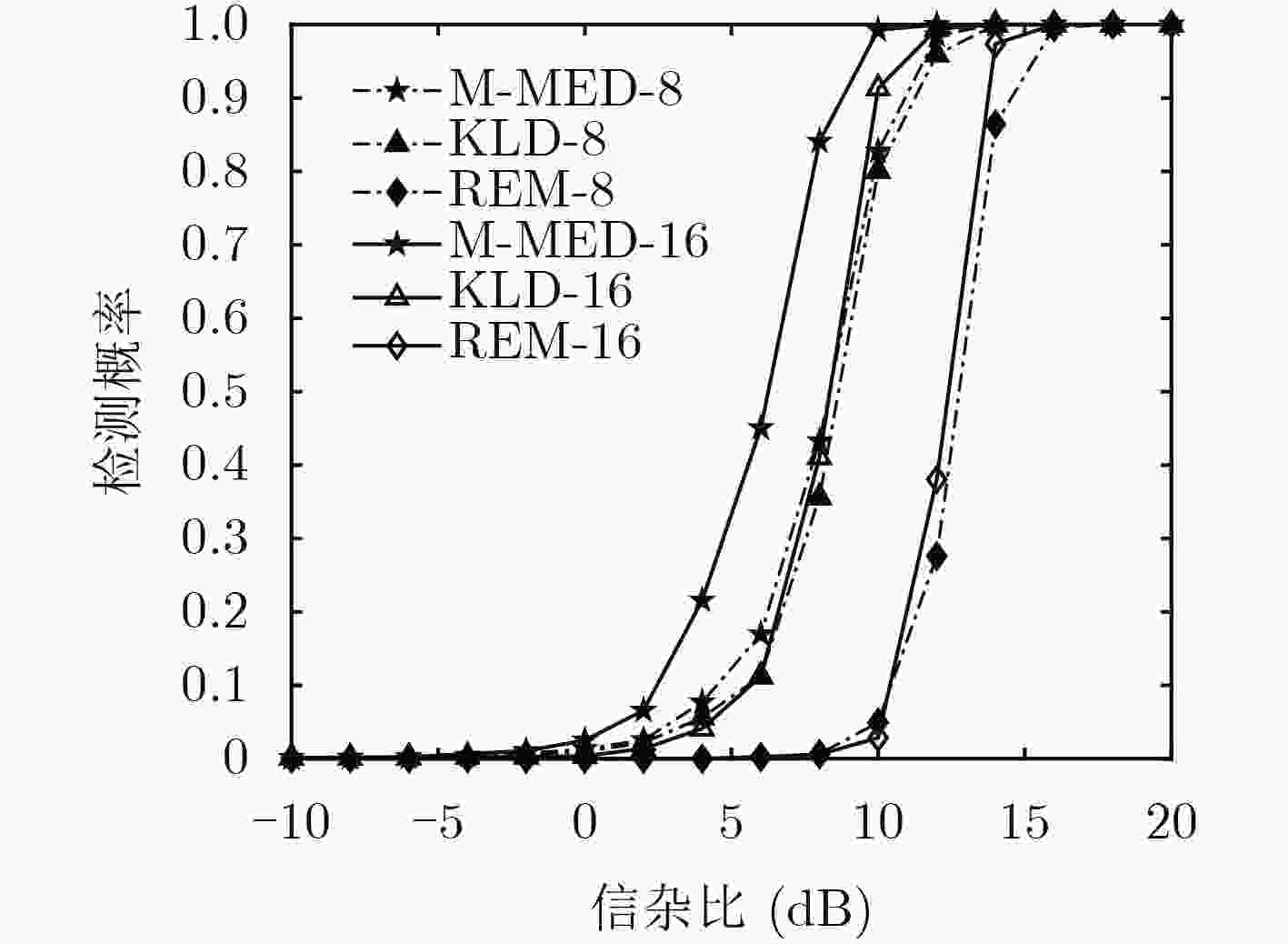

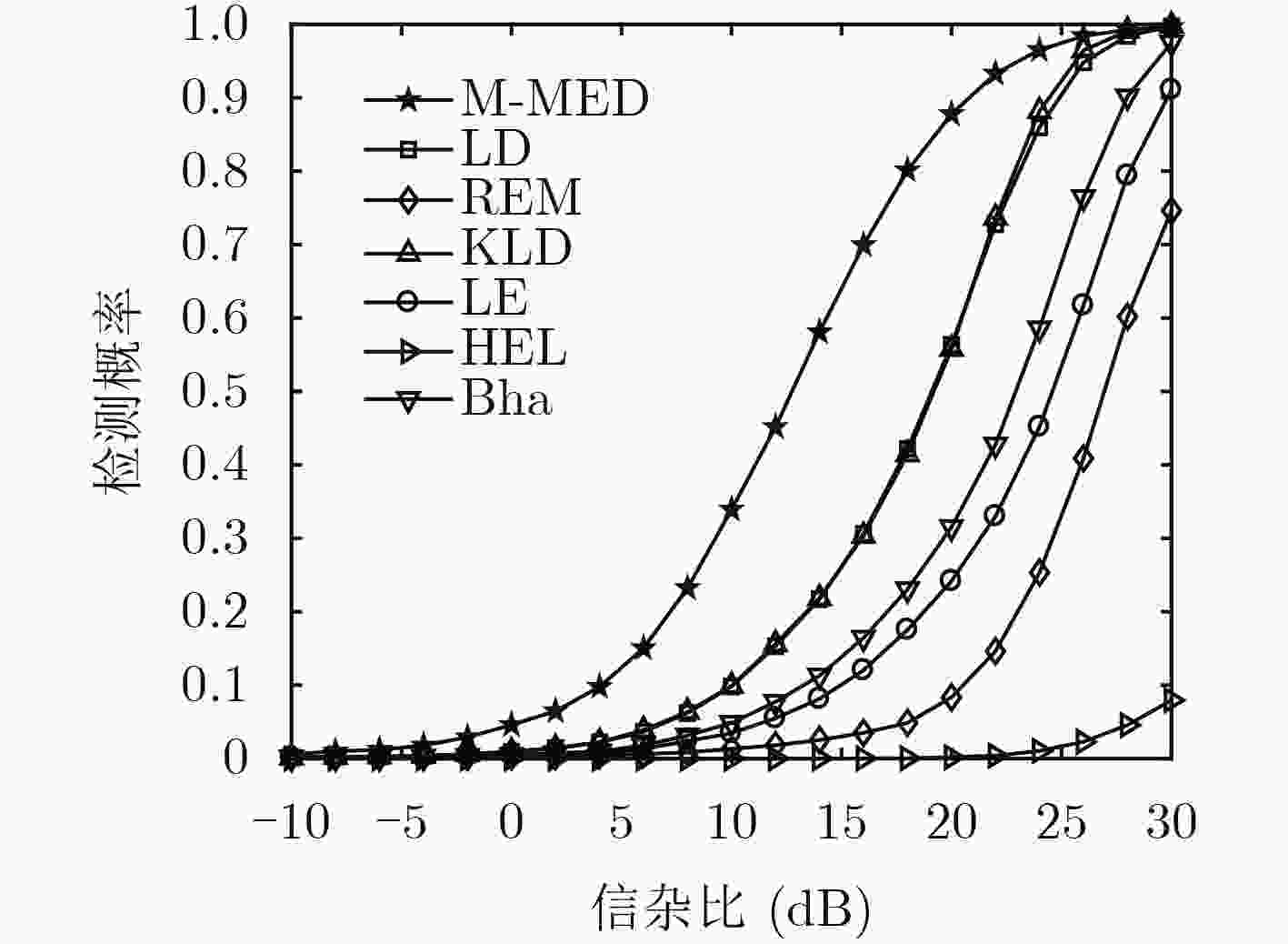


 下载:
下载:
