A Class of Repeated-root Constacyclic Codes over the Ring Fq+uFq++uk-1Fq

1.   引言

近年来，随着UHF RFID技术的成熟^[1]，近场通信应用已不再是高频(HF)标签所主导的领域。普遍预期UHF RFID标签将在未来数十年主导近场(NF)应用市场^[2]；另一方面，无源RFID标签与标签之间的近距离通信^[3]及标签间的无线功率传输^[4]等研究在UHF频段中开辟出新的应用前景，特别是在物联网领域。但是在UHF RFID系统NF应用中，仍有许多问题亟待解决，如密集放置的标签彼此之间产生的互阻抗会导致系统的能量传输效率降低、频率偏移等问题^[5]。

UHF RFID近场具有切向和径向的电场和磁场分量，对应于磁场(电感)耦合和电场(电容)耦合。由于超高频近场主要应用在单品识别中，经常要识别一些附着于液体环境周围的标签，而磁场抗液体环境干扰性较强，因此UHF RFID系统近场天线更多的是利用磁场耦合。在标签密集放置的情况下，标签间也存在磁场耦合，这对RFID系统有着重要影响。佐磊等人^[6,7]推导出密集条件下标签间的互阻抗表达式，并分析了互偶效应对系统的影响。但以上几篇文献都是研究分析干扰标签位于工作标签天线的辐射远场区，未考虑感应近场区的标签密集放置情况。当任意两个标签位于彼此的近场区时，标签的增益将发生质的变化，上述公式已不再适用。彭章友等人^[8,9]研究了基于电感耦合原理工作的标签，密集放置在UHF RFID感应近场区内时，标签天线增益的变化情况以及天线自阻抗的频移特性。上述文献研究的标签天线具有特定性，且未能进一步地就影响标签间互阻抗的环境因素，来分析系统的互耦效应。文献[10]基于毕奥—萨伐尔定律解析出了UHF RFID近场系统中两个天线线圈之间的互阻抗，但这类方法只适用于结构单一、形状规则的线圈天线。微带标签天线，因结构复杂等特点，较难获得标准线圈的有关等效参数。

针对上述问题，本文首先结合变压器模型，从无线电能传输的角度，研究分析电感耦合型标签密集放置在UHF RFID感应近场区时，标签天线间的互阻抗表达式。然后对标签建模间接提取参数，代入推导的公式与传统公式相比较，并将标签间的互阻抗值引入标签的谐振频率偏移计算中，对推导出的互阻抗表达式进行验证。最后从标签间互阻抗为出发点，探究UHF RFID近场互耦效应中频率偏移的影响因素。

2.   典型UHF RFID系统近场链路模型

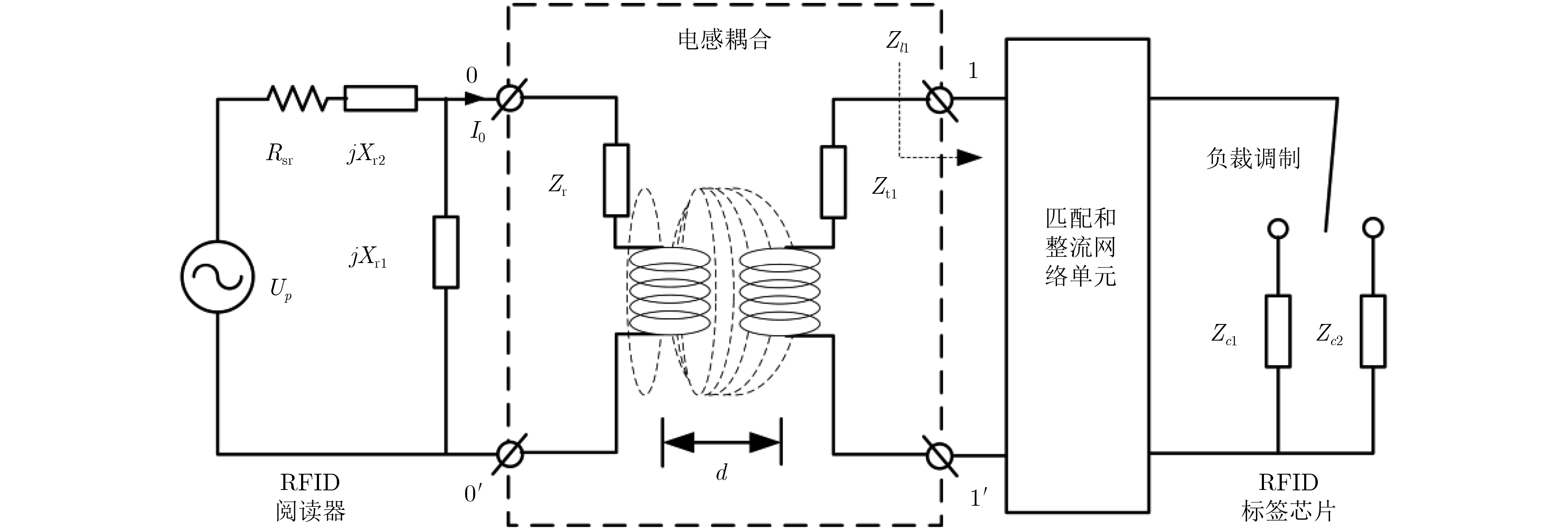
UHF RFID近场系统是一种变压器型电感耦合式原理工作的系统^[10]，如图1所示。读写器天线视为其初级线圈，标签天线视为其次级线圈。对于普通的近场天线，只要线圈间的距离$d \le 0.16\lambda$(5 cm左右)，系统就是有效的。但是这个距离已经远远不能够满足实用需求。目前，许多近场应用的阅读器通过采用分段环型天线等，可将其有效读取距离提高至十几个厘米，甚至更远^[11]。

图  1  UHF RFID近场磁场耦合电路模型

下载: 全尺寸图片 幻灯片

图1中R_sr为阅读器电源电阻(通常为${\rm{50}}\ \Omega$)；Z_r为阅读器天线阻抗，为了实现阅读器电源与负载之间的最大功率传输，需要设置天线阻抗匹配网络，X_r1, X_r2为阅读器天线阻抗L型匹配网络的电抗元件；Z_t1为标签天线阻抗；Z_l1为$11'$端口阻抗，包括匹配网络、整流单元和芯片负载的阻抗。当读写器阻抗匹配系数$\rho = 1$，即R_sr–jX_r2=Z_r//jX_r1时(其中，“//”表示阻抗并联)，阅读器天线线圈的电流I₀及阅读器天线对标签芯片的耦合功率P_01c分别为^[12]

式中，U_p为阅读器的电源电压；阅读器天线阻抗Z_r=R_r+jwML_r；标签天线阻抗Z_t1=R_t1+jwML_t1；P_01c为标签芯片功率；M为阅读器天线线圈与标签天线线圈之间的耦合互感。

整流网络及匹配网络都是由电抗元件组成，因此不消耗有功功率，故标签芯片消耗的有功功率P_01c即为负载的有功功率P_01l。故标签天线获得耦合功率P_01t为

对于微带标签，其天线等效线圈之间为弱耦合^[11]，即有${{{{(wM)}^2}} / {(4{R_{\rm{r}}}{R_{\rm{t}}})}} \ll 1$，则式(3)可简化为

在UHF RFID系统中，一方面当标签近距离地放置在阅读器天线感应近场区时，阅读器天线与标签天线之间存在电感耦合，如UHF RFID近场应用等；另一方面，当多标签密集放置在阅读器天线读取区域内，且标签位于彼此的感应近场区，其间也存在电感耦合，这对UHF RFID的阅读器的发射功率，工作频率等有着重要的影响。当标签间距$d \le 0.16\lambda$，阅读器天线传输给工作标签天线的无线电能公式式(4)，也同样适用于干扰标签耦合给工作标签的能量^[6,7]。

3.   UHF RFID近场互偶效应中频率偏移

3.1   标签天线间互阻抗的推导

为了简化推导过程，本文以双标签为例，对密集标签间的互阻抗表达式进行推导。图2为UHF感应近场区内双标签耦合等效二端口网络。其中，标签1为工作标签，标签2为干扰标签，两者相距为d₁₂。U₁, U₂分别为标签1, 2单独放置在阅读器天线感应近场区时的感应电压；I₁, I₂分别为标签1, 2独立工作时的电流。U₁₂, U₂₁为标签1, 2之间产生的互感应电压。记Z₁₁=Z_t1+Z_l1, Z₂₂=Z_t2+Z_l2分别为标签1, 2的自阻抗；Z₁₂, Z₂₁为标签1, 2间的互阻抗，且有U₁₂=Z₁₂I₁, U₂₁=Z₂₁I₂。则有^[6]

图  2  双标签互耦等效二端口网络

下载: 全尺寸图片 幻灯片

由式(4)可得，标签2对标签1天线部分的耦合功率为

标签2对标签1天线部分的耦合有功功率被其天线电阻消耗，故P₂₁也可以表示为

结合式(6)，式(7)可得

式中，标签1天线与标签2天线之间的互感M₂₁为

Z₂₁相位记为

当阅读器天线感应近场区密集放置n个标签时，而且任意两个标签都位于彼此的感应近场区，由式(5)可得

由式(9)～式(11)可得任意两个标签之间的互阻抗Z_ij为

(12)

其中，任意两个标签天线间的互感为

式中，k_ij为任意两标签之间的耦合系数。对于微带标签，小间隔标签天线间的耦合系数可以通过3.2节的方法来提取。

3.2   互阻抗中耦合系数提取方法

为了便于理论公式推导，仅以两标签间的耦合系数提取为例。两个微带标签天线等效电路之间的磁耦合可以用阻抗逆变器$K = \omega {L_{\rm{m}}}$表示^[13]，如图3所示。其中，R_ti, L_ti, C_ti分别为微带标签天线i(i=1, 2)的等效电感、电阻及电容，L_m为标签天线间耦合电感。该电路发生谐振的条件为${Y_L} = - {Y_R}$，即有

图  3  双标签电感耦合等效电路

下载: 全尺寸图片 幻灯片

当两个标签类型相同时，双标签间的耦合系数^[13]为

式中，f₁, f₂为双标签系统的谐振频率(工作频率)，可以借助HFSS的S₁₂曲线图获得f₁, f₂。此时来自标签2的耦合能量完全被标签1接收，即S₁₂=0 dB。该方法的局限性在于，两标签需为同种类型，且标签间距${d_{12}} < 0.16\lambda$。

3.3   互阻抗引起的工作标签频率偏移

标签间的互耦效应会导致标签1的工作频率向低频偏移^[14]，由式(5)易得：

文献[15]指出当两个标签独立工作时I₁=I₂，故式(16)可化简为

记${Z'_{\rm t1}} = {Z_{\rm t1}} + {Z_{12}}$为双标签互耦时标签1的天线部分总阻抗。标签1独立工作时的工作频率为$f = 1 /$${ {2{{π}} \sqrt {{L_{\rm t1}}C} }} = 915\ {\rm{MHz}}$，则互耦时的工作频率为

式中，${L'_{\rm t1}} = {{\operatorname{Im} ({{Z'}_{\rm t1}})} / {2{{π}} f}}$, C为标签天线的寄生电容与并联电容的等效电容，由于本文没有考虑标签间的电场耦合，故假定C不变。记标签1的频率偏移量为$\Delta f = f - f'$。

4.   实验研究准备

4.1   标签及其参数提取

UHF RFID近场标签通常有传统型偶极子标签、环形标签和T型匹配偶极子标签等，本文以超宽带环形标签Impinj J41为研究对象。因微带标签天线的结构特点，本文通过在ANSYS HFSS 15.0软件中，对标签建立3D初始化模型来间接地提取其各电气参数值，如图4所示。标签模型参数如表1所示，其中h为标签基质厚度，基质为理想导体(PET：${\cal E}_ {\rm{r}}=3.0, \tan \theta = 0.02$)。

图  4  标签

下载: 全尺寸图片 幻灯片

表  1  标签模型参数(mm)

参数名称	$h$	${r_0}$	${r_1}$	${r_2}$	${a_1}$	${a_2}$	$l$
数值	0.05	8.00	5.90	5.20	$2\sqrt 2$	$2\sqrt 2 - 1.4$0	4.20

下载: 导出CSV 

| 显示表格

由仿真结果可得，标签天线的阻抗Z_t1=(10.2039+j143.3921) $\Omega$，又由J41的Datasheet可知，其芯片Monza 4E的并联电阻为$1650\ \Omega$，并联电容为2.48 pF(915 MHz)，则标签的负载阻抗Z_l1=(11–j143) $\Omega$，可认为建立的标签模型基本上实现了共轭阻抗匹配的要求。又经Agilent网络分析仪对标签实物进行回波损耗测试分析，与仿真值进行对比，结果如图5所示。结果表明，仿真模型性能与实物的性能相差无几，即本文通过对标签实物建立仿真模型，来克服实际操作中难以获得标签电气参数值的困难，间接提取电气参数值的方法是可行的。

图  5  标签J41的回波损耗性能

下载: 全尺寸图片 幻灯片

4.2   双标签间耦合系数的提取

在RFID实际应用中，标签密集放置形式具有随机性，且场景较为复杂^[16]。本文仅选取两种较为典型的场景来建模提取耦合系数，其中自由空间为边长120 mm的正方体，工作标签位于正方体中心，如图6所示。

图  6  HFSS下的双标签互耦仿真模型图

下载: 全尺寸图片 幻灯片

当d=5, 25 mm时，标签间的插入损耗曲线图S₁₂，如图7所示。理论上，当两标签天线完全耦合时，来自标签2的耦合能量全部被标签1接收，即S₁₂=0 dB；当两标签天线耦合较弱时，标签1接收到来自标签2的耦合能量较小，通常S₁₂<20 dB。但在仿真实验中，很难实现S₁₂=0 dB，故以图7中S₁₂曲线上两个波峰值对应的频率点分别为式(17)中的f₁, f₂。更多耦合系数数据如图8所示。随着标签间距的增大，双标签系统的两个谐振频率点f₁，f₂逐渐趋近，直至汇聚为一个频率点。当两标签间距超过某一临界值(小于$0.16\lambda$)时，谐振频率点甚至不出现，耦合系数为0。

图  8  双标签布放不同时耦合系数与距离的关系

下载: 全尺寸图片 幻灯片

图  7  标签间插入损耗曲线图(垂直叠放)

下载: 全尺寸图片 幻灯片

5.   实验结果与分析

实验测试平台是上海聚星仪器有限公司研发的型号为VISN-R1200射频识别综合测试仪，该仪器可实时发射并采集射频信号，其具备一个信号发射天线、一个信号采集天线。被测试标签1, 2均为环形标签Impinj J41，单端口连接，响应频率为915 MHz，最大被读取距离为22 cm。实验中，阅读器发射天线与标签1的距离设定为定值d₀₁=15 cm，仿真测试软件为ANSYS HFSS 15.0。文献[17]的研究表明，当标签密集放置时，一个干扰标签对工作标签的耦合影响与多个干扰标签对工作标签造成的耦合影响的趋势是一致的，因此两枚标签系统已经能够反映多枚标签系统的性能。

5.1   本文公式及与传统公式的比较

由图9可知，两标签之间的互阻抗实部几乎为零。微带标签密集放置时，标签间的互耦更多的是影响标签天线的虚部。因此，互阻抗虚部大小能够反映出标签间的互耦强度。微带标签天线间的互阻抗虚部为

图  9  不同距离下的标签间互阻抗仿真值

下载: 全尺寸图片 幻灯片

RFID近场系统中两个天线线圈之间的互阻抗虚部表达式(传统公式)为^[18]

易得式(18)较式(19)，多了一个校正系数。将前文得到的双标签间互耦时的相关参数值代入式(18)，式(19)计算，且与仿真值相比较，结果如图10所示。结果表明，本文公式计算值较传统公式的平均误差明显较小。原因在于微带标签天线较传统线圈天线结构复杂、体积小，两微带标签天线间的电感耦合情况较RFID近场两线圈间电感耦合更为复杂，故传统公式不宜用于UHF RFID近场密集标签间的互阻抗计算。

图  10  标签间的互阻抗虚部理论值与仿真值对比

下载: 全尺寸图片 幻灯片

5.2   标签间互阻抗导致的频率偏移

标签密集放置时，标签1受到标签2的互耦影响会导致其工作频率的偏移，仅以标签的垂直叠放为例，改变标签2与标签1的间距d₁₂，经测试即可获得不同间距下工作标签受到互耦时的工作频率，如图11所示。

图  11  标签1受到互耦时的工作频率偏移

下载: 全尺寸图片 幻灯片

图11(a)，11(b)分别为当标签1受到不同距离下标签2的耦合影响时的回波损耗S₁₁的仿真值和测量值，且其最佳工作频率(S₁₁曲线对应的最低点，简称工作频率)向低频偏移，这与文献[14]的结论是一致的。提取图11(a)，11(b)中的最佳工作频率与式(17)计算得到的标签1工作频率理论值如图11(c)所示。图11(c)表明，在实际的环境中，标签1的工作频率测量值要大于理论值与仿真值，原因在于测试环境非自由空间，来自标签2天线产生电磁波受环境因素衰落较多，对工作标签耦合作用减弱。当标签间距大于30 mm，标签间的互耦电磁波衰落的愈加严重，彼此间的互阻抗很小，标签1的频率几乎没有发生偏移，其间的互耦效应很弱。当间距小于30 mm时，标签1的工作频率测试值与本文公式计算的理论值变化趋势接近，误差较小。标签1工作频率的理论值小于实验测量值和HFSS软件仿真值的原因，在于本文计算忽略了标签间的电场耦合引起的C 值变小。

5.3   频率偏移影响因素分析

影响标签间互阻抗的影响因素有很多，限于文章篇幅，本文仅讨论标签间的相对位置、标签的附着物等主要影响因素。标签附着物尺寸为8.5 cm×5.4 cm×1 mm。其中标签间距d₁₂从5 mm变化到50 mm，步进值为5 mm。当d₁₂=15 mm时，标签夹角$\theta$从–${75^ \circ }$变化到${75^ \circ }$，步进值为${15^ \circ }$。实验布置如图12所示。

图  12  实验布置示意图

下载: 全尺寸图片 幻灯片

5.3.1   相对位置对工作标签频率偏移量的影响

图13给出了标签2与标签1的相对位置不同时，标签1的频率偏移量变化情况。当$\theta$=${0^ \circ }$时，Df 随着d₁₂增加而减小，但非简单的线性关系，d₁₂较小时，Df 变化率较大；d₁₂较大时，Df 变化率较小，且d₁₂>30 mm时，Df 几乎没有变化。当d₁₂=15 mm时，Df 与$\theta$的变化表现出一种对称性质，即Df 在(–${75^ \circ }$,${0^ \circ }$]内变化情况与[${0^ \circ }$,${75^ \circ }$)内变化情况一样(如图12所示，顺时针偏转角为正)。当$\theta$接近${0^ \circ }$时，Df较大；当$\theta$>${75^ \circ }$时，Df几乎没有变化，原因在于此时，标签2产生的变化磁感线几乎不通过与之近乎垂直的标签1，故对其影响可忽略不计。由图14数据可得，理论值与实验值的误差在1.6～7.3 MHz之间；当标签间距d₁₂>30 mm，夹角|$\theta$|>${60^ \circ }$时，标签1的频率偏移量约小于13.05 MHz，对于本文选择的超宽带J41标签而言，标签2对标签1的互耦影响较小。

图  13  $Δf$ 随d₁₂及$\theta$的变化图

下载: 全尺寸图片 幻灯片

图  14  $Δf$ 随标签间相对位置的变化

下载: 全尺寸图片 幻灯片

5.3.2   标签附着物对工作标签频率偏移量的影响

仿真实验中，为了便于提取不同标签间距下标签间的耦合系数，本文假定标签天线的基质类同于其附着的物体。其基质，依次设定为环氧树脂(FR4)、胶木(bakelite)、玻璃(glass)；测试实验中分别将标签粘贴于上述材料的物体上，测试不同材料下的标签1的频率偏移量Δf，结果如15所示。

图15(a)仿真结果表明，对于J41标签，两标签密集放置时，其间的耦合系数小于0.015。间距相同时，当标签附着于高介电常数的物体时，标签间的耦合系数较低。原因是对于微带标签，低介电常数基质可以增强产生辐射的边缘场，因此密集标签间的电磁波衰落的较少，标签2对标签1的磁场耦合效果较强。图15(b)，15(c)，15(d)实验结果也表明，间距相同时，标签的附着物为高介电常数物体时，标签1的频率偏移较小。综合图15可得，双标签间的耦合系数与Δf变化趋势一致。因此，在物品级标签领域，可以将标签附着于高介电常数物体，降低阅读器的误读率；在标签间通信等方面，可使用低介电常数附着物，提高系统的能量利用率等。由图15(b)，15(c)，15(d)易得，当标签受到互耦时，本文推导的互阻抗表达式较传统公式在计算其工作频率偏移量上误差明显较小，更加适用于UHF RFID近场互耦效应问题中的频率偏移预估。

图  15  $Δf$ 随 ${\cal E}_ {\rm{r}}$的变化($\theta={0^ \circ }$)

下载: 全尺寸图片 幻灯片

6.   结束语

本文基于变压器模型，从无线电能传输的角度，推导出密集放置在UHF RFID感应近场区内的电感耦合型双标签之间的互阻抗表达式，以电感耦合型标签J41为例，通过仿真与实验验证了该互阻抗表达式在d₁₂<30 mm时，较传统互阻抗公式，更加适用于UHF RFID近场系统中存在电感耦合的双环形标签天线间的互耦效应问题中的频率偏移预估研究，误差小，精确性高。并得出了以下结论：(1)当密集放置的标签类型确定时，标签间的耦合系数唯一影响互阻抗；(2)本文Df 理论值与实验值的误差范围为(1.6～7.3 MHz)，且当标签间距d₁₂>30 mm，夹角|$\theta$|>${60^ \circ }$时，工作标签的频率偏移量约小于13.05 MHz；Df与标签附着物的介电常数成负相关。研究结果对于UHF RFID近场中多个物品级标签应用具有指导意义。本文方法仍有一定的不足，推导的互阻抗表达式是基于UHF近场电感耦合型标签，实际中UHF近场标签天线之间还存在微弱的电场耦合。

下一步的工作，将验证本文的结论对于形状复杂的UHF RFID近场标签是否适用，并结合标签间的电容耦合进一步地优化本文提出的UHF RFID近场标签天线互阻抗表达式；探究影响标签间互阻抗的其他因素，为研究UHF RFID近场互耦效应提供更加有力的理论支撑。